Elementary Algebra

Elementary Algebra is a foundational topic in mathematics, crucial for success in the ACT exam. This guide will cover essential concepts, definitions, and examples to help you achieve a high score.

Learning Objectives

In Elementary Algebra for the ACT Math exam, you should focus on mastering the basic operations involving integers, fractions, and decimals. Understand and solve linear equations, inequalities, and systems of equations. Become proficient in working with polynomials, including factoring and expanding expressions. Gain a solid grasp of rational expressions, ratios, proportions, and quadratic equations. Additionally, practice translating word problems into algebraic expressions and equations to develop problem-solving skills.

Variables and Expressions

Variables: Symbols (usually letters) that represent numbers.

Expressions: Combinations of variables, numbers, and operations (e.g., 2x+3).

Operations with Real Numbers

• Addition and Subtraction: Combining like terms.
• Multiplication and Division: Applying distributive property a(b+c) = ab+ac.
• Order of Operations: PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction).

Solving Linear Equations

A linear equation is an equation of the first degree, meaning it has no exponents higher than one. The general form is:

ax+b = c

Steps to solve linear equations:

1. Simplify both sides of the equation (expand, combine like terms).
2. Isolate the variable on one side (add or subtract terms).
3. Solve for the variable (divide or multiply).

Example: 2x+3 = 7
2x = 4
x = 2

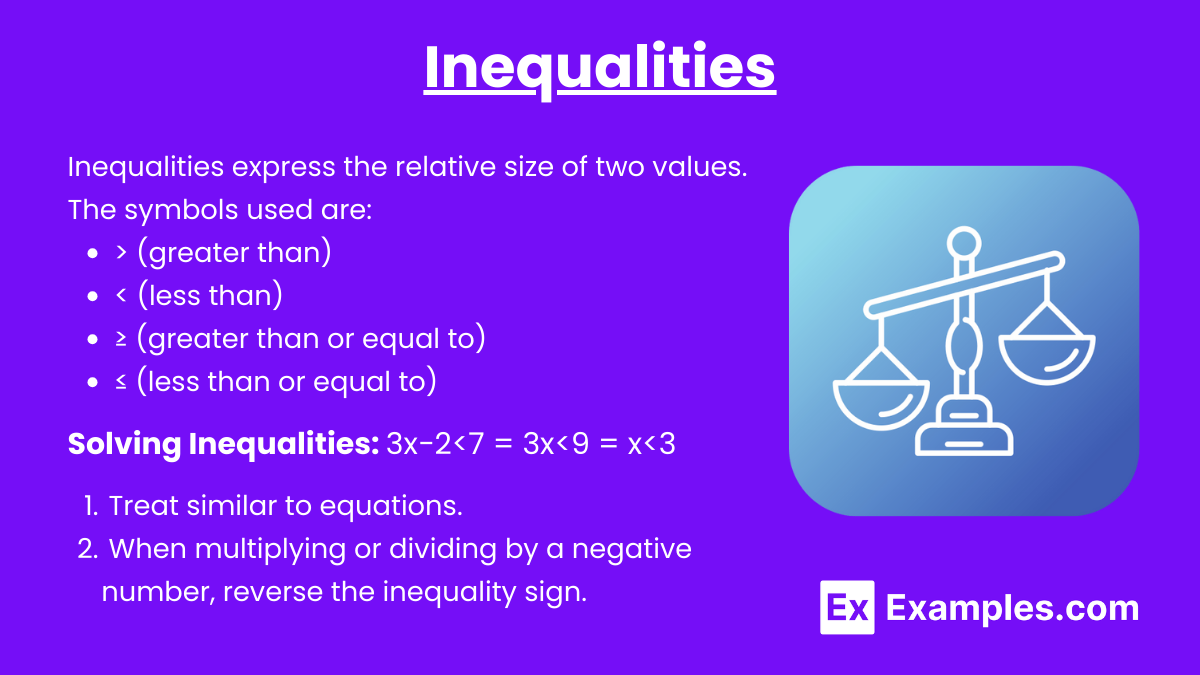
Inequalities

Inequalities express the relative size of two values. The symbols used are:

• > (greater than)
• < (less than)
• ≥ (greater than or equal to)
• ≤ (less than or equal to)

Solving Inequalities:

1. Treat similar to equations.
2. When multiplying or dividing by a negative number, reverse the inequality sign.

Example: 3x−2<7 = 3x<9 = x<3

Functions

A function f(x) is a relation where each input x has exactly one output f(x).

Example: f(x) = 2x+1
If x = 3,
f(3) = 2(3)+1 = 7

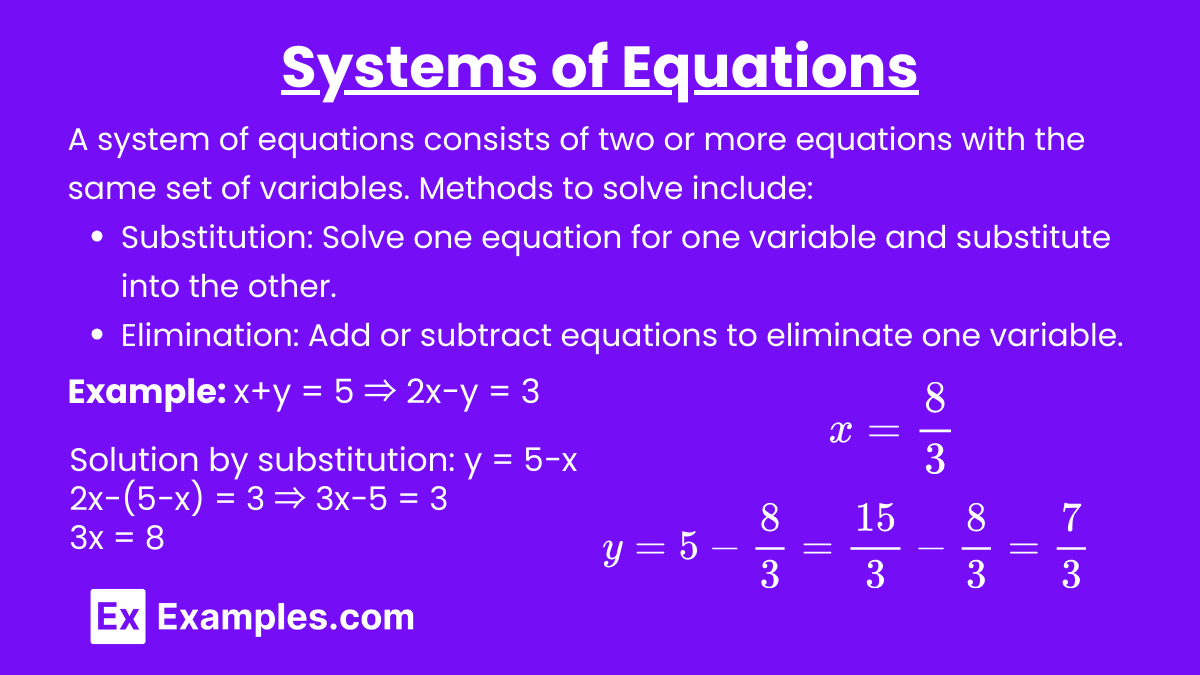
Systems of Equations

A system of equations consists of two or more equations with the same set of variables. Methods to solve include:

• Substitution: Solve one equation for one variable and substitute into the other.
• Elimination: Add or subtract equations to eliminate one variable.

Example: x+y = 5
2x−y = 3

Solution by substitution: y = 5−x
2x−(5−x) = 3
3x−5 = 3
3x = 8

   

   

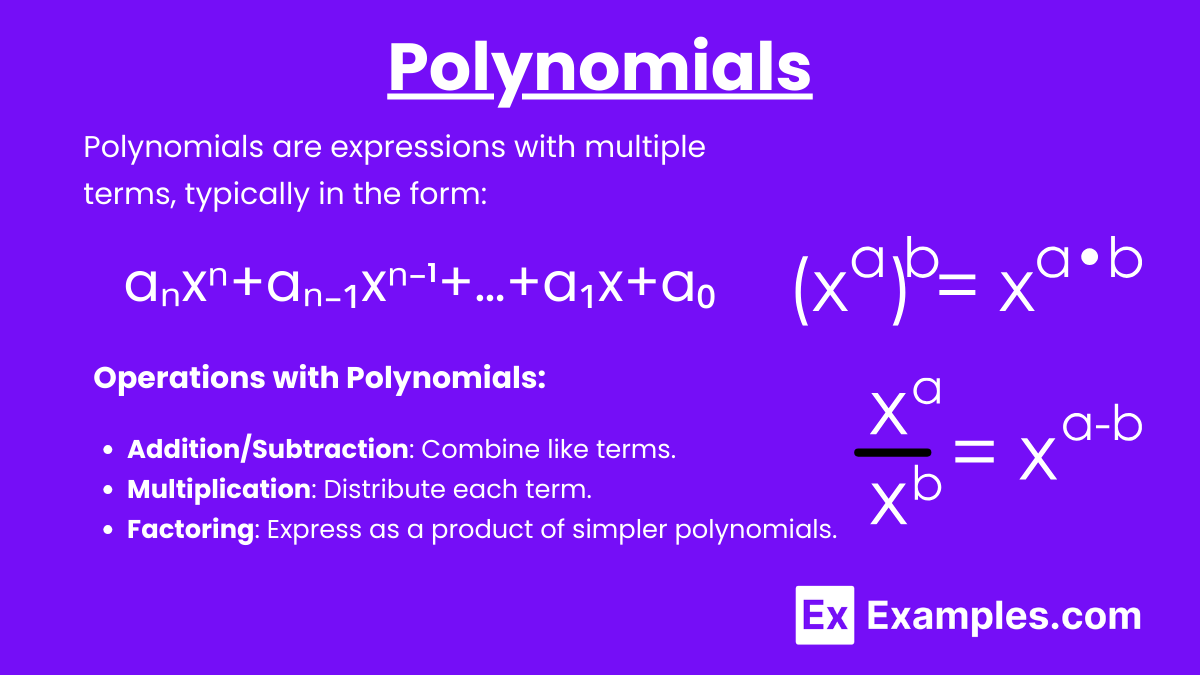
Polynomials

Polynomials are expressions with multiple terms, typically in the form:

aₙ​xⁿ+aₙ₋₁xⁿ⁻¹+…+a₁​x+a₀

Operations with Polynomials:

• Addition/Subtraction: Combine like terms.
• Multiplication: Distribute each term.
• Factoring: Express as a product of simpler polynomials.

Example: x²−5x+6 = (x−2)(x−3)

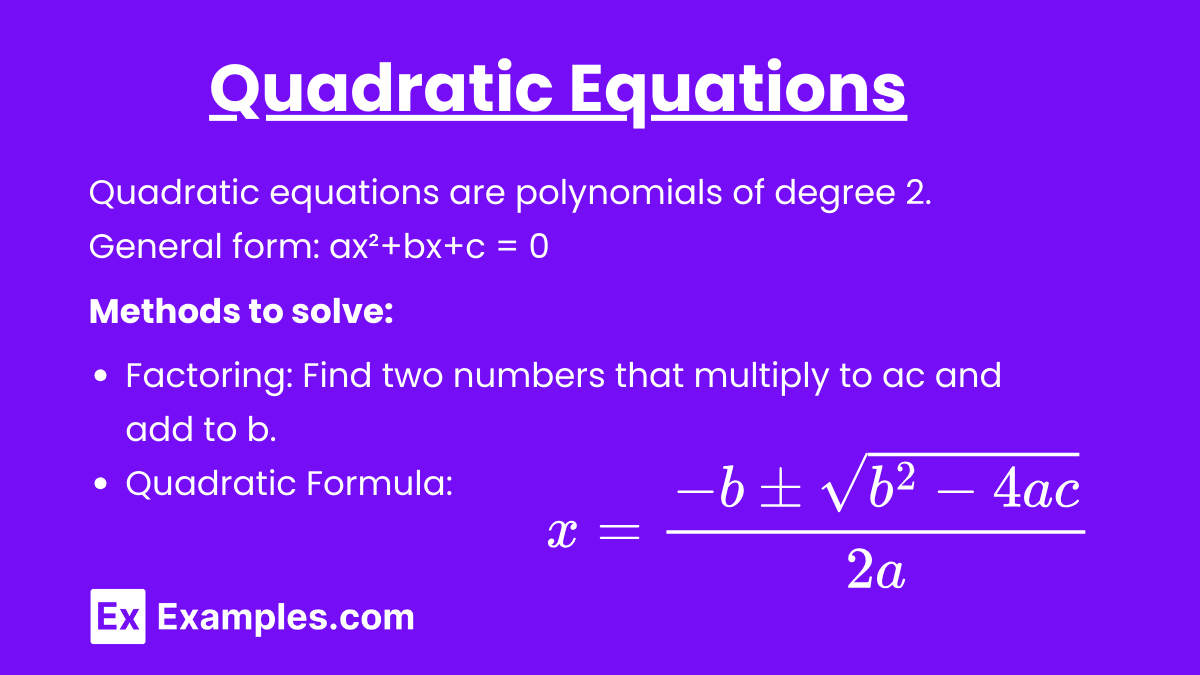
Quadratic Equations

Quadratic equations are polynomials of degree 2. General form: ax²+bx+c = 0

Methods to solve:

• Factoring: Find two numbers that multiply to ac and add to b.
• Quadratic Formula:
  

     

  
  

Example: x²−3x+2 = 0
Factoring: (x−1)(x−2) = 0
x = 1 or x = 2

Elementary Algebra Multiple Choice Questions

Question 1:

Solve for x in the equation: 3x−7 = 2x+5.

A. x = 1
B. x = 2
C. x = 3
D. x = 4

Answer: D. x = 4

Explanation:

To solve the equation 3x−7 = 2x+5, follow these steps:

• Subtract 2x from both sides: 3x−2x−7 = 2x−2x+5
  x−7 = 5
• Add 7 to both sides: x−7+7 = 5+7
  x=12

So, the correct answer is D. x = 4.

Question 2:

Which of the following is a factor of x²−9?

A. x+3
B. x−9
C. x−1
D. x+9

Answer: A. x+3

Explanation:

The expression x²−9 can be factored using the difference of squares formula, which states a²−b² = (a+b)(a−b). Here, a = x and b = 3:

• Apply the difference of squares formula: x²−9 = (x+3)(x−3)

So, the factors of x²−9 are (x+3) and (x−3). Therefore, the correct answer is A. x+3.

Question 3:

Simplify the expression 4(x+2)−3x.

A. x+8
B. x+2
C. x+4
D. x+6

Answer: A. x+8

Explanation:

To simplify 4(x+2)−3x, follow these steps:

• Distribute 4 into (x+2): 4x+8
• Subtract 3x: 4x+8−3x
• Combine like terms: 4x−3x+8 = x+8

So, the correct answer is A. x+8.