Intermediate Algebra builds on the concepts of Elementary Algebra and is essential for achieving a high score on the ACT exam. This guide covers key topics, definitions, and examples to enhance your understanding and performance.
Learning Objectives
In Intermediate Algebra for the ACT Math exam, you should focus on understanding and solving quadratic equations, including using the quadratic formula and completing the square. Master the properties and operations of exponents and radicals. Learn about functions, including evaluating, interpreting, and graphing them. Study complex numbers and their operations. Gain proficiency in solving systems of equations, both linear and non-linear. Additionally, explore sequences, series, and logarithms to enhance problem-solving skills and algebraic understanding.
Rational Expressions
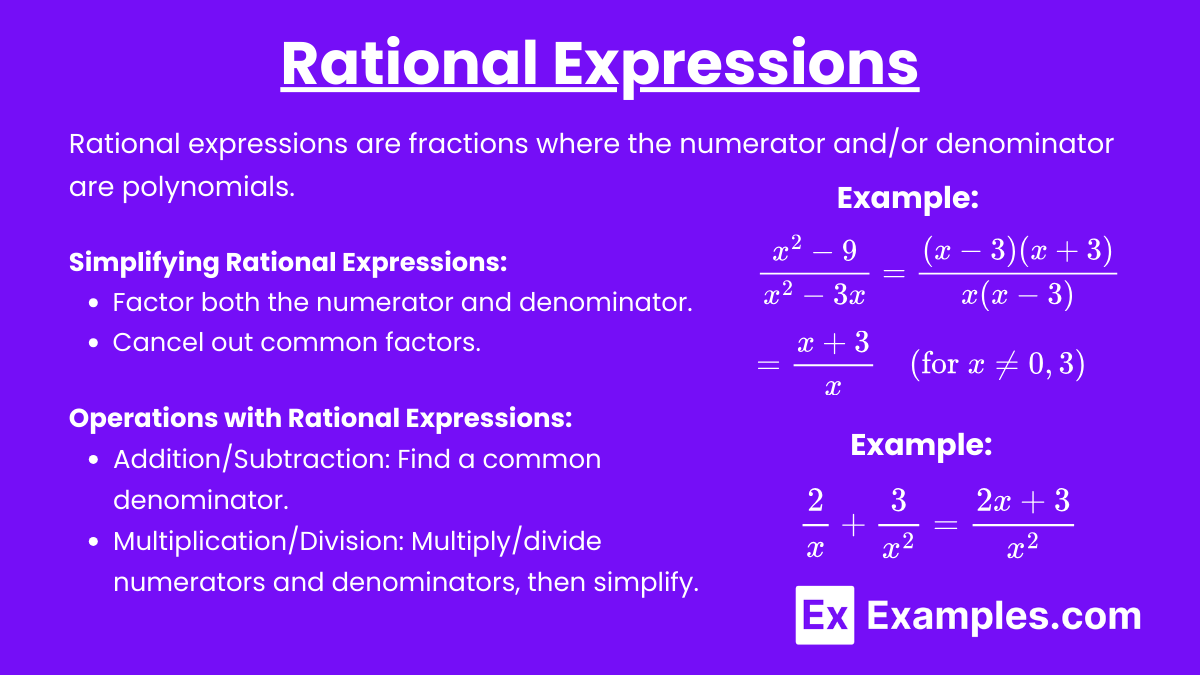
Rational expressions are fractions where the numerator and/or denominator are polynomials.
Simplifying Rational Expressions:
- Factor both the numerator and denominator.
- Cancel out common factors.
Example:
![]()
Operations with Rational Expressions:
- Addition/Subtraction: Find a common denominator.
- Multiplication/Division: Multiply/divide numerators and denominators, then simplify.
Example:
![]()
Radical Expressions and Equations
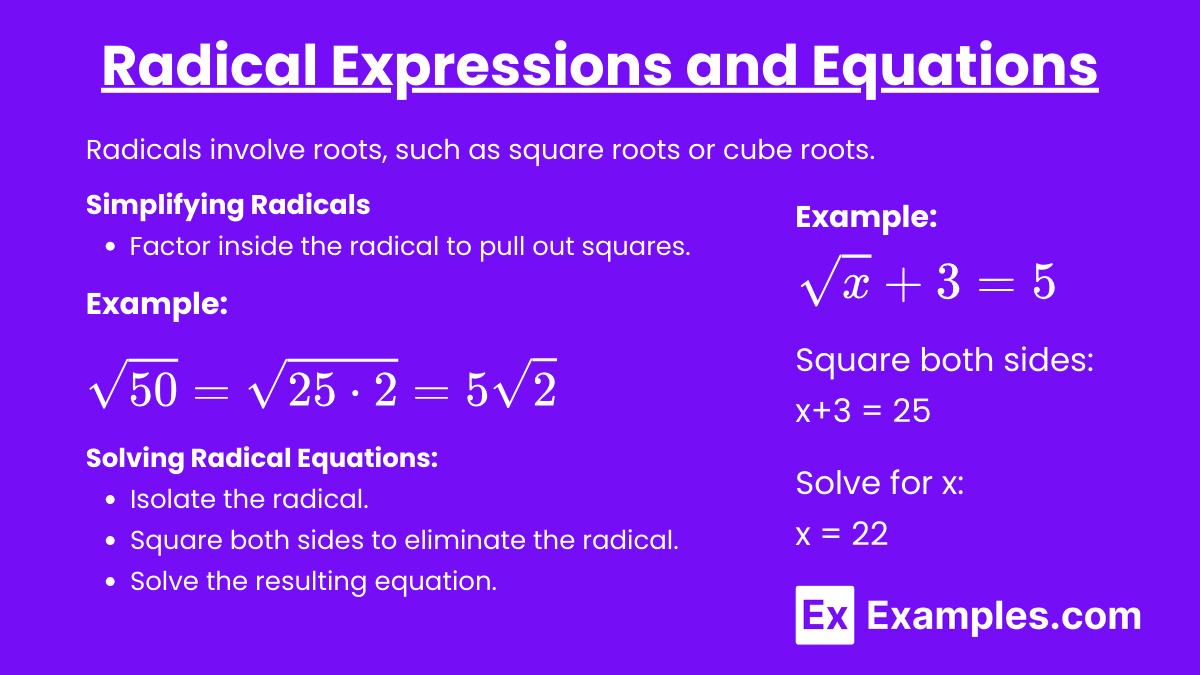
Radicals involve roots, such as square roots or cube roots.
Simplifying Radicals:
- Factor inside the radical to pull out squares.
Example:
![]()
Solving Radical Equations:
- Isolate the radical.
- Square both sides to eliminate the radical.
- Solve the resulting equation.
Example:
![]()
Square both sides: x+3 = 25
Solve for x: x = 22
Quadratic Functions and Equations
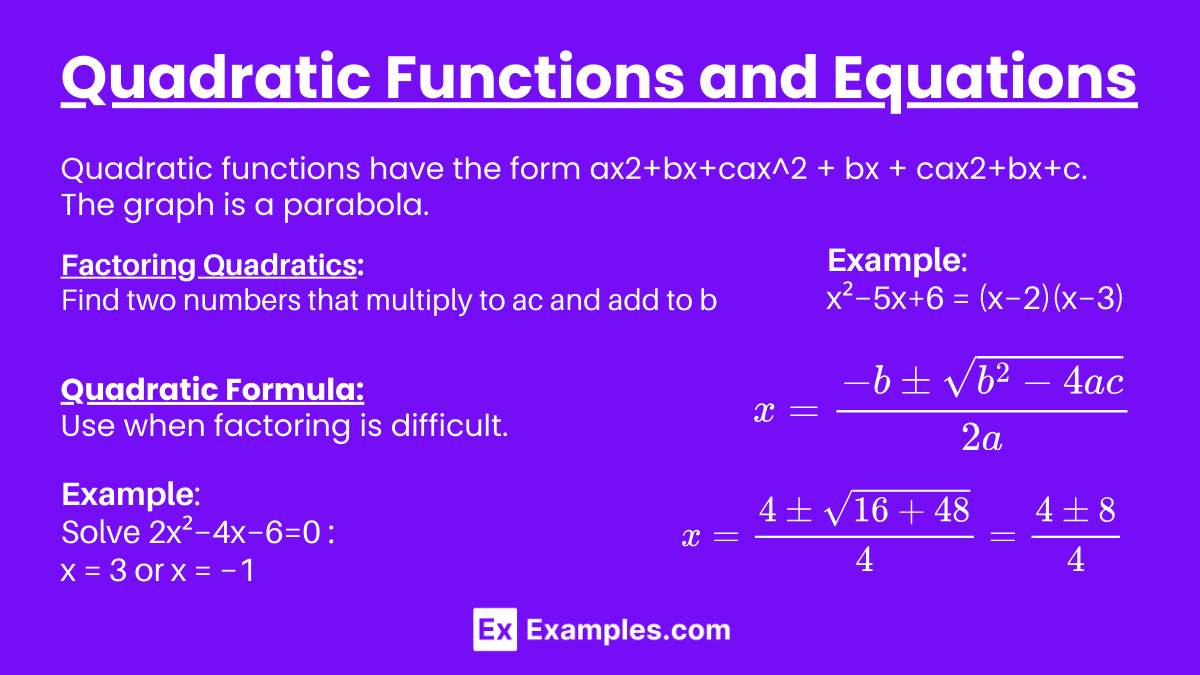
Quadratic functions have the form ax²+bx+c. The graph is a parabola.
Factoring Quadratics:
Find two numbers that multiply to ac and add to b.
Example:
x²−5x+6 = (x−2)(x−3)
Quadratic Formula:
Use when factoring is difficult.
![]()
Example:
Solve 2x²−4x−6 = 0:
![]()
x = 3 or x = −1
Exponential and Logarithmic Functions
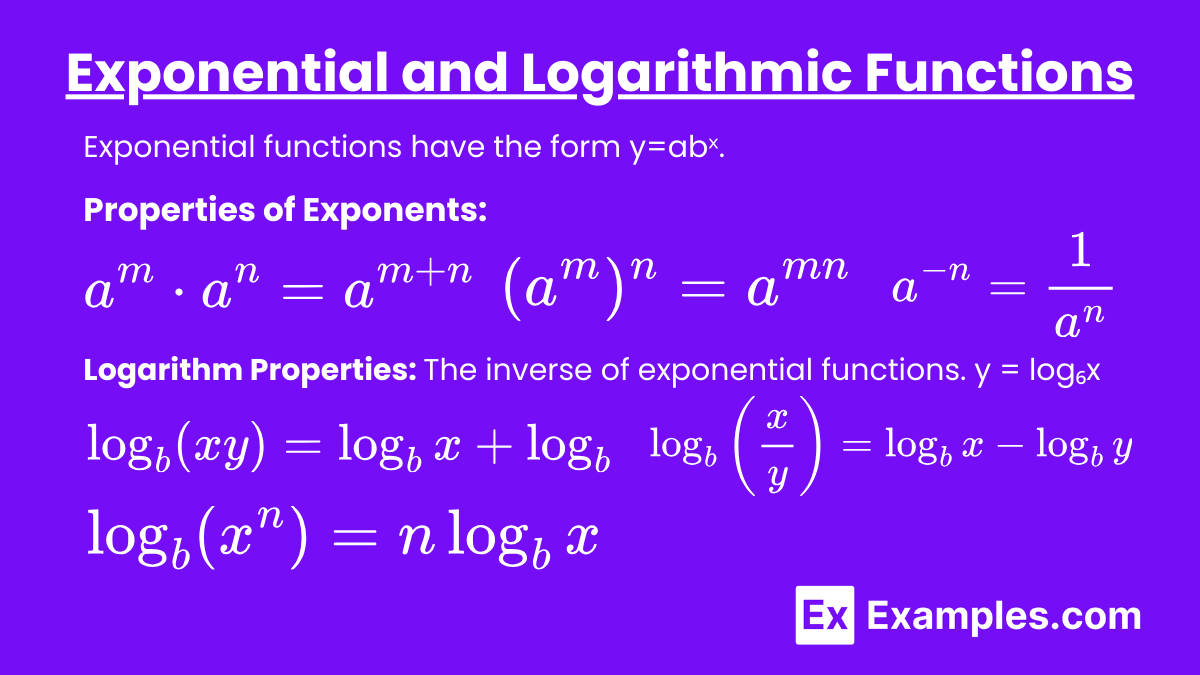
Exponential functions have the form y = abˣ.
Properties of Exponents:
Logarithms:
The inverse of exponential functions. y = log₆x means bᵧ = x.
Example:
log₂8 = 3(since 2³ = 8)
Logarithm Properties:
Functions and Their Graphs
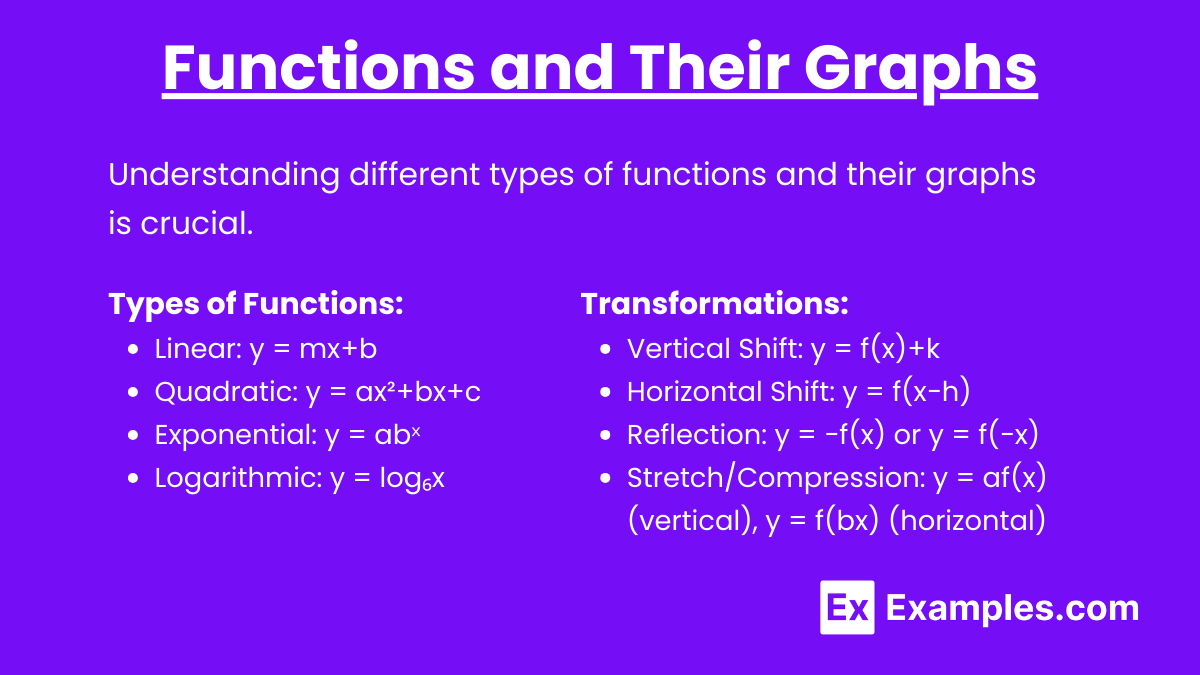
Understanding different types of functions and their graphs is crucial.
Types of Functions:
- Linear: y = mx+b
- Quadratic: y = ax²+bx+c
- Exponential: y = abˣ
- Logarithmic: y = log₆x
Transformations:
- Vertical Shift: y = f(x)+k
- Horizontal Shift: y = f(x−h)
- Reflection: y = −f(x) or y = f(−x)
- Stretch/Compression: y = af(x) (vertical), y = f(bx) (horizontal)
Systems of Equations and Inequalities
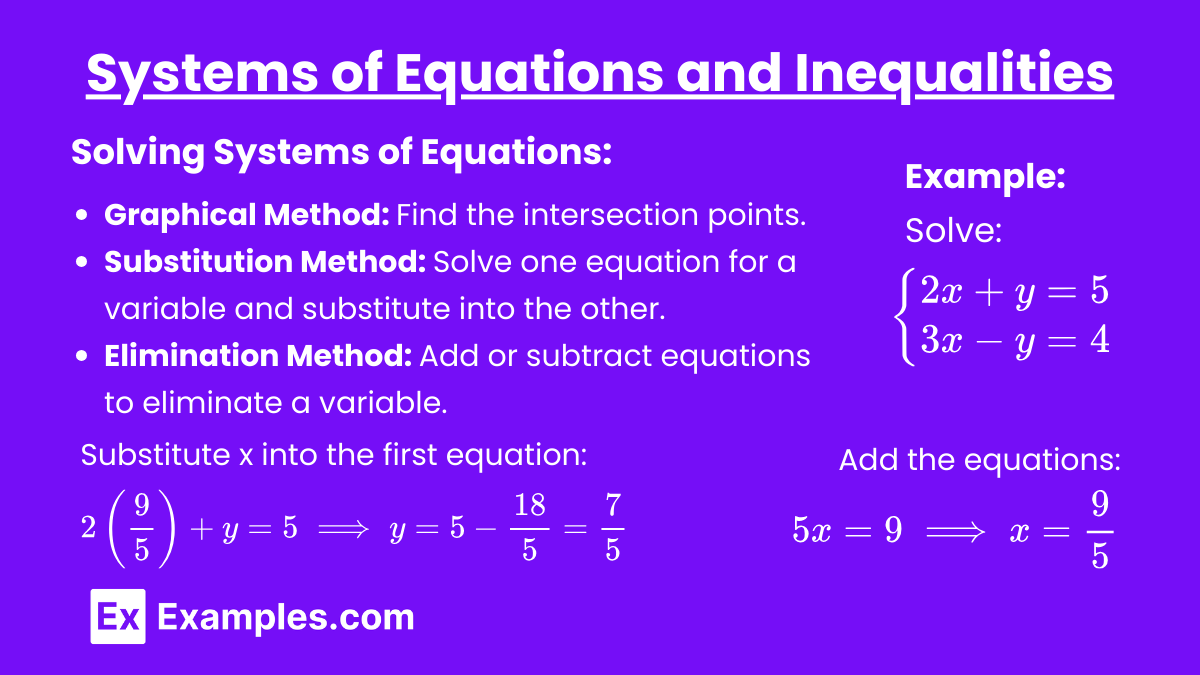
Solving Systems of Equations:
- Graphical Method: Find the intersection points.
- Substitution Method: Solve one equation for a variable and substitute into the other.
- Elimination Method: Add or subtract equations to eliminate a variable.
Example:
Solve:
![Rendered by QuickLaTeX.com \[\begin{cases}2x + y = 5 \\ 3x - y = 4\end{cases}\]](https://www.examples.com/wp-content/ql-cache/quicklatex.com-f733da5ae774c4066fec7dcc7b148aa9_l3.png)
Add the equations:
![]()
Substitute x into the first equation:
![]()
Intermediate Algebra Multiple Choice Questions
Question 1:
Solve for x in the quadratic equation: x²−5x+6 = 0.
A. x = 2 and x = 3
B. x = −2 and x = −3
C. x = 1 and x = 6
D. x = −1 and x = −6
Answer: A. x = 2 and x = 3
Explanation:
To solve the quadratic equation x²−5x+6 = 0, we can factorize it:
- Factorize the quadratic equation:
x²−5x+6 = (x−2)(x−3) = 0 - Set each factor to zero and solve for x:
x−2 = 0 ⟹ x = 2
x−3 = 0 ⟹ x = 3
So, the correct answer is A. x = 2 and x = 3.
Question 2:
Solve the system of equations:
2x+3y = 7
x−y = 1
A. x = 1, y = 2
B. x = 2, y = 1
C. x = 3, y = 1
D. x = 1, y = 3
Answer: B. x = 2, y = 1
Explanation:
To solve the system of equations 2x+3y = 7 and x−y = 1:
- Solve the second equation for x:
x−y = 1 ⟹ x = y+1 - Substitute x=y+1 into the first equation:
2(y+1)+3y = 7
2y+2+3y = 7
5y+2 = 7 - Solve for y:
5y = 5
y = 1 - Substitute y=1 back into x = y+1:
x = 1+1
x = 2
So, the correct answer is B. x = 2, y = 1.
Question 3:
Simplify the expression:
![]()
A. x−3
B. x−2
C. x−1
D. x−4
Answer: C. x−1
Explanation:
To simplify
![]()
- Factor the numerator:
3x²−9x = 3x(x−3) - Rewrite the expression:
![Rendered by QuickLaTeX.com \[\frac{3x(x - 3)}{3x}\]](https://www.examples.com/wp-content/ql-cache/quicklatex.com-3faf7379ccc2f385929ccc56d5802749_l3.png)
- Cancel the common factor 3x:
x−3
So, the correct answer is C. x−1.