Antiderivatives and Indefinite Integrals
Antiderivatives and indefinite integrals are core topics in AP Calculus AB and BC, essential for understanding the reverse process of differentiation. They allow us to find the original function from its derivative, providing a fundamental tool for solving various calculus problems, including those involving motion, area, and accumulation. Mastery of antiderivatives, along with key integration techniques, is crucial for both AB and BC students, as these concepts are heavily tested and form the basis for more advanced topics in calculus.
Learning Objectives
When studying Antiderivatives and Indefinite Integrals for the AP Calculus AB and BC exams, you should focus on understanding the process of reversing differentiation, mastering the basic integration rules, and applying these rules to find antiderivatives of various functions. Additionally, learn to use integration techniques such as substitution and, for BC, integration by parts and trigonometric substitution. Finally, apply these concepts to solve problems related to motion, area, and accumulation to ensure a comprehensive grasp of the topic.
Definition of Antiderivatives
An antiderivative of a function f(x) is a function F(x) such that:
\(F'(x) = f(x) \)
This means that the derivative of F(x) with respect to x gives us back the original function f(x). The process of finding an antiderivative is essentially the reverse of differentiation.
Formal Definition
Given a function f(x), a function F(x) is called an antiderivative of f(x) if:
\(\frac{d}{dx} \left[ F(x) \right] = f(x) \)
Antiderivatives are not unique. Instead, they form a family of functions that differ by a constant. This is because the derivative of a constant is zero, so adding any constant C to a function F(x) does not change its derivative.
Example:
If f(x) = 2x, then an antiderivative F(x) can be found by reversing the differentiation:
\(F(x) = x^2 + C \)
where C is the constant of integration. The constant C represents an infinite family of functions that differ only by a constant.
Indefinite Integrals
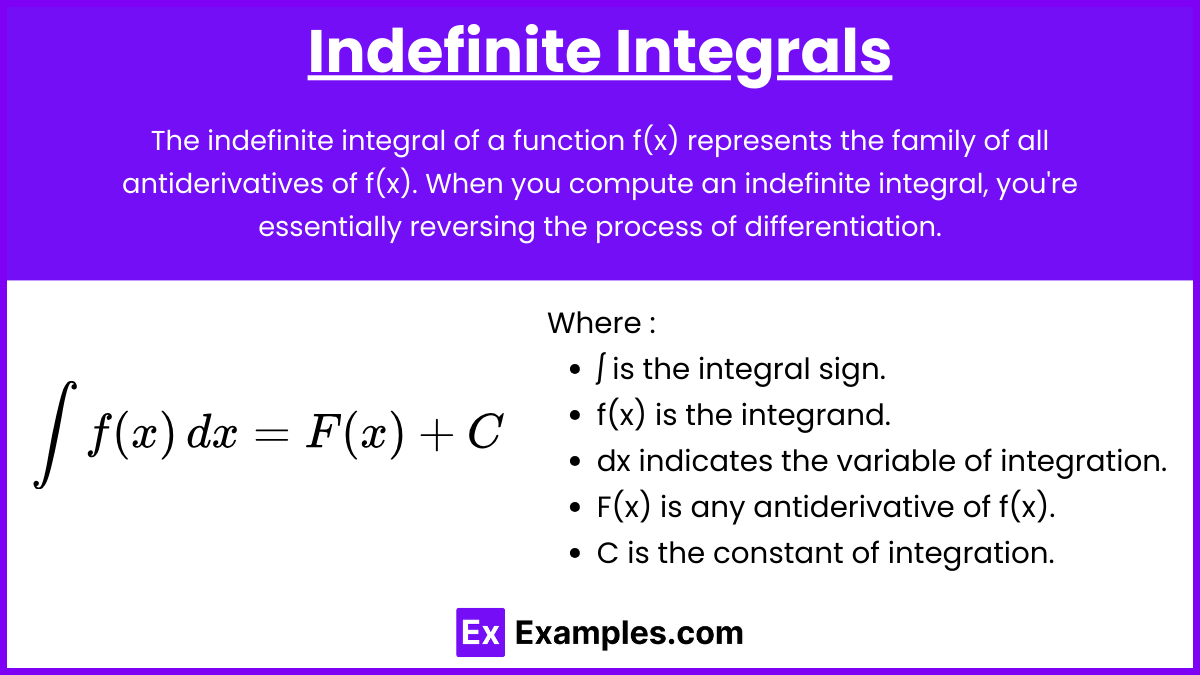
The indefinite integral of a function f(x) represents the family of all antiderivatives of f(x). When you compute an indefinite integral, you’re essentially reversing the process of differentiation. Unlike definite integrals, which yield a specific numerical value, indefinite integrals yield a general expression, which includes a constant of integration, C, to account for the fact that any constant added to a function does not affect its derivative.
\(\int f(x) \, dx = F(x) + C \)
where:
- ∫ is the integral sign.
- f(x) is the integrand.
- dx indicates the variable of integration.
- F(x) is any antiderivative of f(x).
- C is the constant of integration.
Basic Rules of Integration
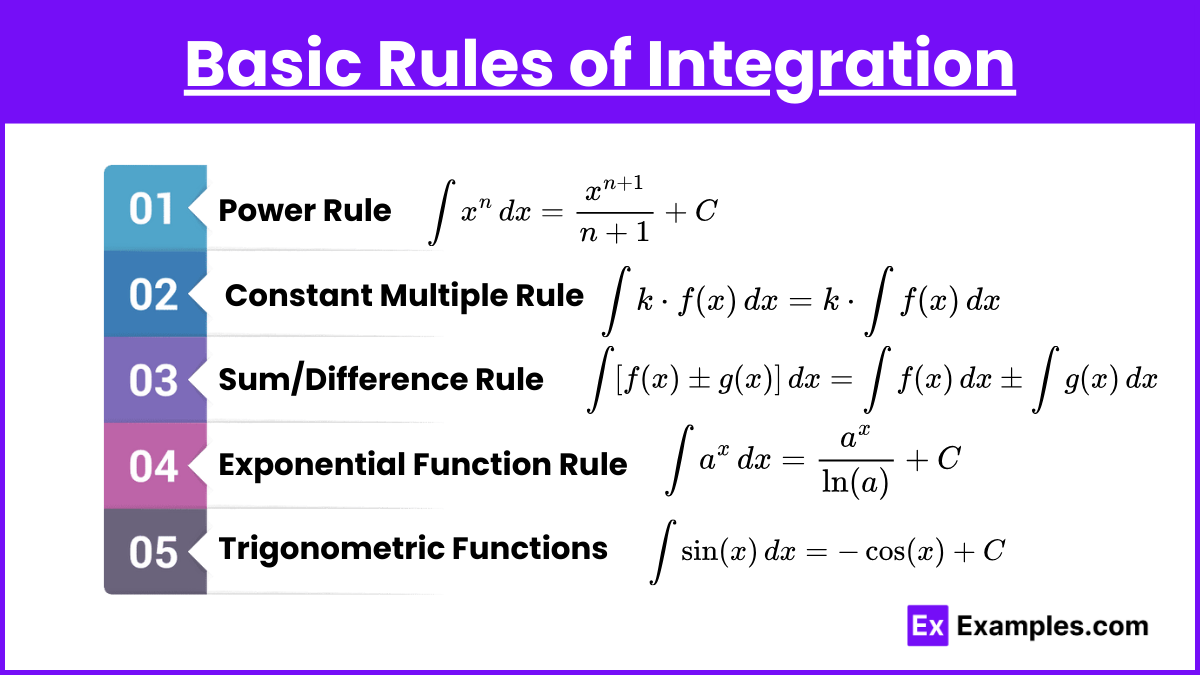
To efficiently find indefinite integrals, understanding and applying a set of fundamental integration rules is crucial. These rules are the building blocks for more complex integrals and allow you to solve a wide variety of problems quickly and accurately. Below are the key rules, along with examples to illustrate their application.
a. Power Rule : The Power Rule is one of the most frequently used rules for finding the indefinite integral of polynomial functions.
For any real number \( n \neq -1 \),
\(\int x^n \, dx = \frac{x^{n+1}}{n+1} + C \)
Example: \(\int x^3 \, dx = \frac{x^{4}}{4} + C \)
b. Constant Multiple Rule : This rule states that a constant factor can be pulled out of the integral.
For a constant k, \(\int k \cdot f(x) \, dx = k \cdot \int f(x) \, dx \)
Example: \(\int 5x^2 \, dx = 5 \cdot \int x^2 \, dx = 5 \cdot \frac{x^3}{3} + C = \frac{5x^3}{3} + C \)
c. Sum/Difference Rule : This rule allows the integration of sums or differences of functions term by term.
For functions f(x) and g(x),
\(\int [f(x) \pm g(x)] \, dx = \int f(x) \, dx \pm \int g(x) \, dx \)
Example: \(\int (x^2 + 2x) \, dx = \int x^2 \, dx + \int 2x \, dx = \frac{x^3}{3} + x^2 + C \)
d. Exponential Function Rule : When dealing with exponential functions, there are specific rules depending on the base of the exponential.
For a > 0 and \( a \neq 1 \),
\(\int a^x \, dx = \frac{a^x}{\ln(a)} + C \)
For the natural exponential function ex:
\(\int e^x \, dx = e^x + C \)
e. Trigonometric Functions : Integrating trigonometric functions is essential in calculus. Each basic trigonometric function has a corresponding integral that you need to memorize.
\(\int \sin(x) \, dx = -\cos(x) + C \)
\(\int \cos(x) \, dx = \sin(x) + C \)
\(\int \sec^2(x) \, dx = \tan(x) + C \)
\(\int \csc^2(x) \, dx = -\cot(x) + C\)
\(\int \sec(x)\tan(x) \, dx = \sec(x) + C\)
\(\int \csc(x)\cot(x) \, dx = -\csc(x) + C \)
Integration by Substitution
Integration by substitution is a method used when an integral involves a composite function. The goal is to simplify the integrand by introducing a new variable.Steps:
- Identify a part of the integrand as u such that du (the derivative of u) appears elsewhere in the integrand.
- Rewrite the integral in terms of u and du.
- Integrate with respect to u.
- Substitute back the original variable x.
Example:
Evaluate \( \int 2x \sqrt{x^2+1} \, dx \).
- Let u = x2+1, then \( du = 2x \, dx \).
- The integral becomes \( \int \sqrt{u} \, du \).
- Integrate: \( \frac{2}{3} u^{3/2} + C \).
- Substitute u back: \( \frac{2}{3} (x^2 + 1)^{3/2} + C \).
Applications of Indefinite Integrals
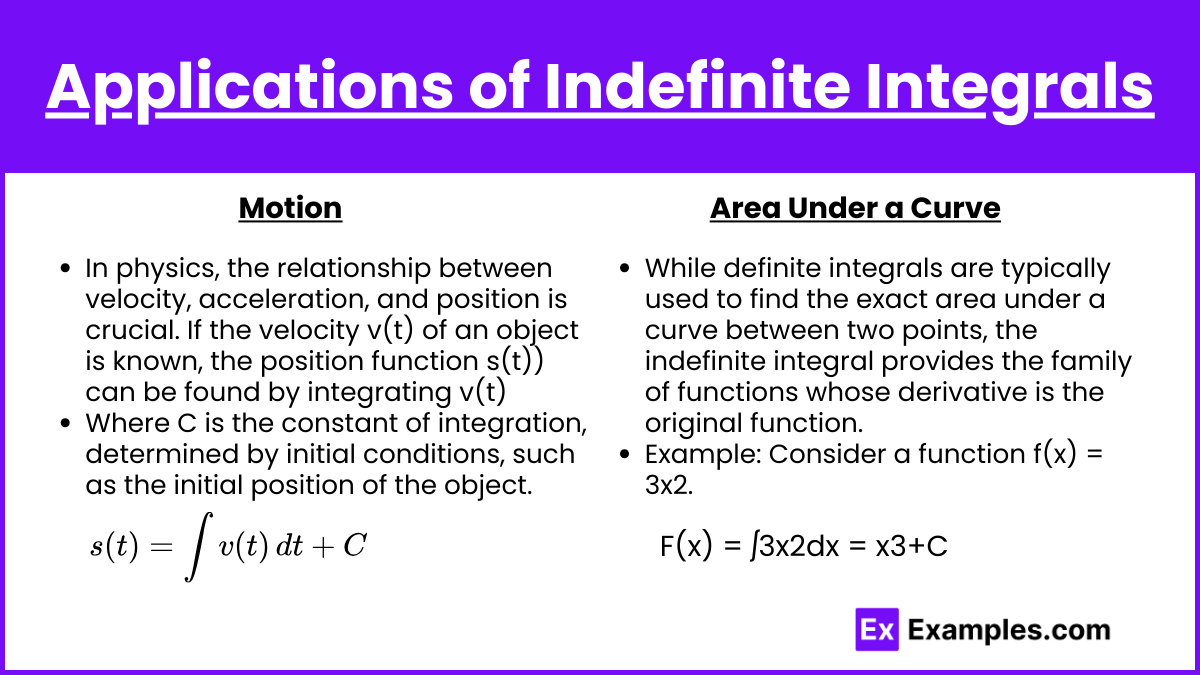
Indefinite integrals have several applications, particularly in determining the family of functions that describe various physical situations, such as motion, area under curves, and accumulation problems.
a. Motion
In physics, the relationship between velocity, acceleration, and position is crucial. If the velocity v(t) of an object is known, the position function s(t)) can be found by integrating v(t)
\(s(t) = \int v(t) \, dt + C \)
Where C is the constant of integration, determined by initial conditions, such as the initial position of the object.
b. Area Under a Curve
While definite integrals are typically used to find the exact area under a curve between two points, the indefinite integral provides the family of functions whose derivative is the original function. This can be useful in understanding the accumulation of area as a function changes.
Example: Consider a function f(x) = 3x2. The indefinite integral gives:
F(x) = ∫3x2dx = x3+C
This function F(x) represents the area under the curve f(x) = 3x2 from an arbitrary point aaa to any point xxx, plus a constant.
Examples
Example 1: Antiderivative of a Polynomial Function
Consider the function f(x) = 4x3−3x2+2x−5. The antiderivative, or indefinite integral, of this function can be found by applying the power rule to each term. The result is: \(\int (4x^3 – 3x^2 + 2x – 5) \, dx = x^4 – x^3 + x^2 – 5x + C \) . Here, C is the constant of integration, representing the infinite set of functions that differ only by a constant.
Example 2: Antiderivative of an Exponential Function
For the function f(x) = e2x, the antiderivative is found by using the integration rule for exponential functions. Since the derivative of e2x is 2e2x, the antiderivative involves dividing by the constant multiple: \(\int e^{2x} \, dx = \frac{1}{2}e^{2x} + C \). The constant C is added to account for the general solution of the antiderivative.
Example 3: Antiderivative of a Trigonometric Function
For the function f(x) = cos(x), the antiderivative is straightforward, as it is a basic trigonometric integral. The integral of cos(x) is: \(\int \cos(x) \, dx = \sin(x) + C \). This result follows from the fact that the derivative of sin(x) is cos(x), making sin(x) the antiderivative.
Example 4: Antiderivative Involving Substitution
Consider the function \( f(x) = 2x \sqrt{x^2 + 1} \). To find the antiderivative, substitution is used. Let u = x2+1, so du = 2x dx. The integral then becomes: \(\int 2x \sqrt{x^2 + 1} \, dx = \int \sqrt{u} \, du = \frac{2}{3} u^{3/2} + C = \frac{2}{3} (x^2 + 1)^{3/2} + C \). Substitution simplifies the integral, making it easier to solve.
Example 5: Antiderivative Using Integration by Parts
For the function f(x) = xln(x), the integration by parts technique is required. Let u = ln(x) and dv = x dx, so \( du = \frac{1}{x} \, dx \) and \( v = \frac{x^2}{2} \). The antiderivative is: \(\int x \ln(x) \, dx = \frac{x^2}{2} \ln(x) – \int \frac{x^2}{2} \cdot \frac{1}{x} \, dx = \frac{x^2}{2} \ln(x) – \frac{x^2}{4} + C \).This process illustrates the application of integration by parts to find antiderivatives that involve products of functions.
Multiple Choice Questions
Question 1
What is the antiderivative of f(x) = 6x?
A) 3x2+C
B) 6x2+C
C) x2+C
D) 12x2+C
Answer: A) 3x2+C
Explanation: To find the antiderivative of f(x) = 6x, use the power rule for integration:
\(\int 6x \, dx = \frac{6x^2}{2} + C = 3x^2 + C \)
So, the correct answer is 3x2+C.
Question 2
Which of the following is the antiderivative of f(x) = 5?
A) 5x+C
B) 5x2+C
C) 1+C
D) 5+C
Answer: A) 5x+C
Explanation: The antiderivative of a constant 5 is 5x+C because:
\(\int 5 \, dx = 5x + C \)
This is because the derivative of 5x is 5.
Question 3
What is the antiderivative of f(x) = x3?
A) \( \frac{x^4}{4} + C \)
B) \( 3x^2 + C \)
C) \( 4x + C \)
D) \( \frac{3x^4}{4} + C \)
Answer: A) \( \frac{x^4}{4} + C \)
Explanation: To find the antiderivative of f(x) = x3, use the power rule:
\(\int x^3 \, dx = \frac{x^{3+1}}{3+1} + C = \frac{x^4}{4} + C \)
So, the correct answer is \( \frac{x^4}{4} + C \).