Applying Understandings of Differentiation to Problems Involving Motion

Last Updated: September 23, 2024

Notes

In AP Calculus AB and AP Calculus BC, applying differentiation to problems involving motion is a fundamental skill that connects mathematical concepts with real-world phenomena. By analyzing the position, velocity, and acceleration of objects, students can understand how these quantities evolve over time. Differentiation allows us to determine an object’s speed, direction, and how these change, providing insights into various physical scenarios. Mastery of these concepts is essential.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

By studying “Applying Understandings of Differentiation to Problems Involving Motion” for AP Calculus AB, you should learn how to differentiate position functions to find velocity and acceleration, analyze motion using these derivatives, and solve problems involving changing direction, speed, and total distance traveled. For AP Calculus BC, you should also understand higher-order derivatives like jerk. Additionally, you will develop the ability to interpret the graphical representations of these functions and apply these concepts to real-world motion scenarios, ensuring a deep understanding for the AP Calculus exam.

Key Concepts in Motion and Differentiation1. Position, Velocity, and Acceleration

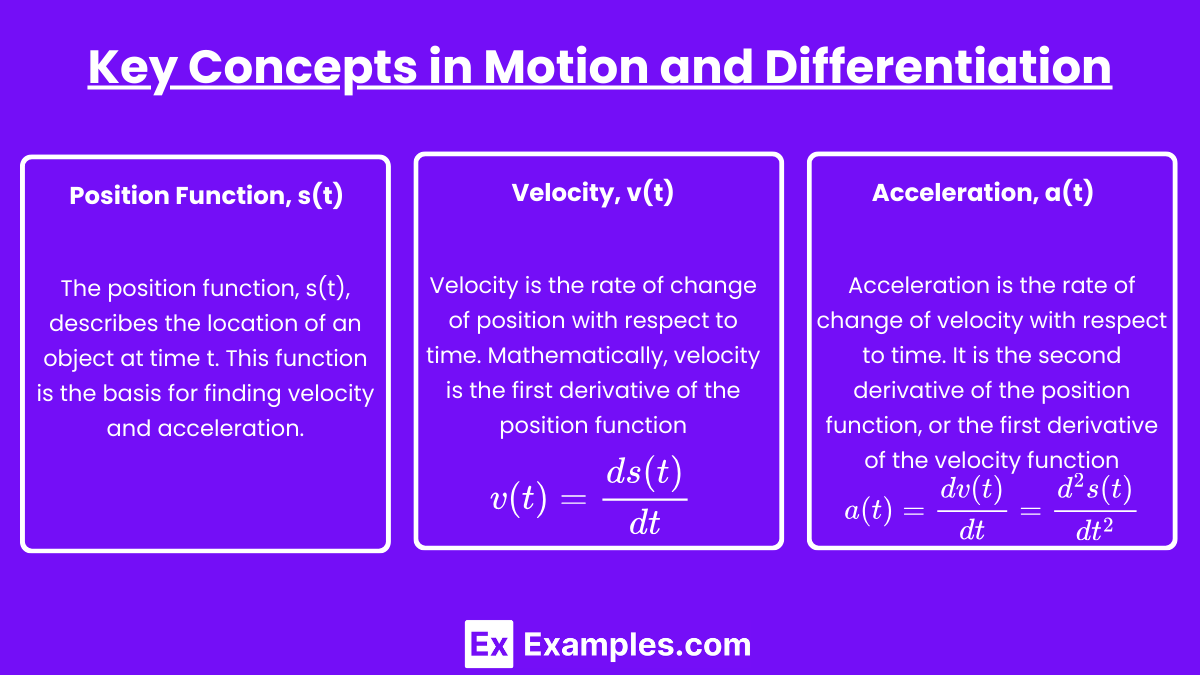
Position Function, s(t): The position function, s(t), describes the location of an object at time t. This function is the basis for finding velocity and acceleration.
Velocity, v(t): Velocity is the rate of change of position with respect to time. Mathematically, velocity is the first derivative of the position function:
$v(t) = \frac{ds(t)}{dt}$
Velocity gives the speed and direction of the object.
Acceleration, a(t): Acceleration is the rate of change of velocity with respect to time. It is the second derivative of the position function, or the first derivative of the velocity function: $a(t) = \frac{dv(t)}{dt} = \frac{d^2s(t)}{dt^2}$
Acceleration indicates how quickly the velocity of the object is changing.
Jerk, j(t)( AP Calculus BC Only ): Jerk is the rate of change of acceleration with respect to time. It is the third derivative of the position function:
$j(t) = \frac{da(t)}{dt} = \frac{d^3s(t)}{dt^3}$
Jerk describes how acceleration is changing over time, often in situations involving higher-level dynamics.

Motion Along a Line

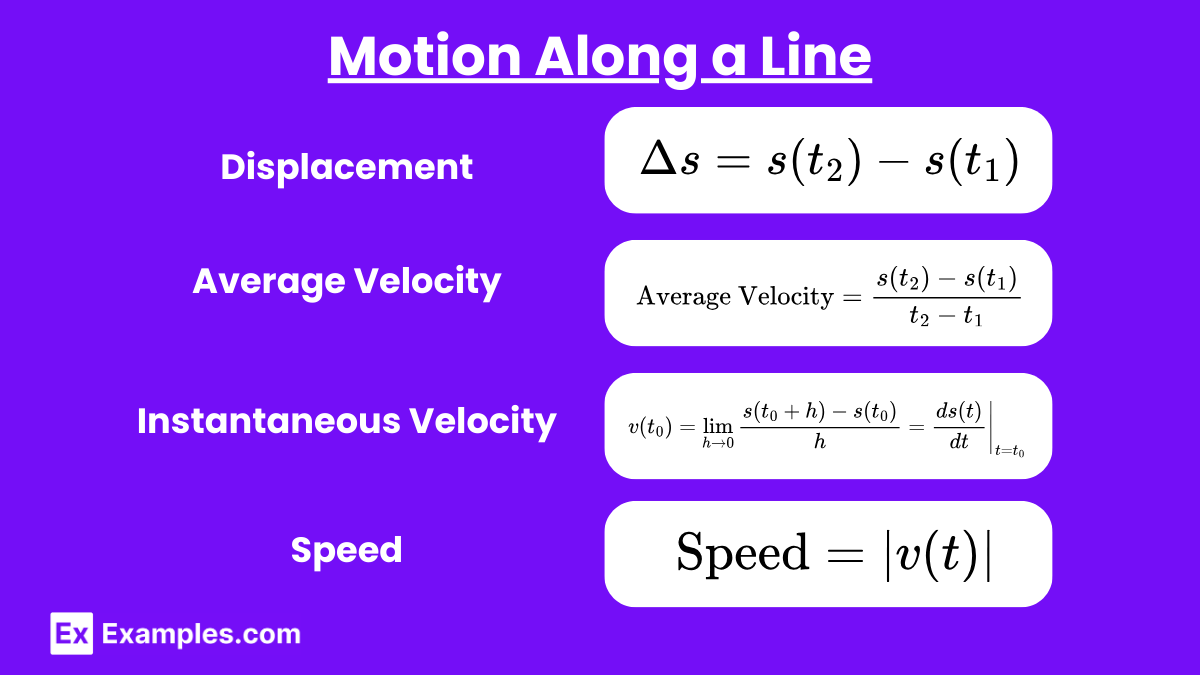
In AP Calculus, you’ll encounter problems where an object moves along a straight line (often referred to as motion in one dimension). Here are the essential ideas:

Displacement: Displacement is the change in position of an object. For an interval from t1 to t2, the displacement is: $\Delta s = s(t_2) - s(t_1)$
Average Velocity: The average velocity over a time interval [t1,t2] is: $\text{Average Velocity} = \frac{s(t_2) - s(t_1)}{t_2 - t_1}$
Instantaneous Velocity: Instantaneous velocity at a specific time t=t0 is given by: $v(t_0) = \lim_{h \to 0} \frac{s(t_0 + h) - s(t_0)}{h} = \left. \frac{ds(t)}{dt} \right|_{t = t_0}$
Speed: Speed is the absolute value of velocity: $\text{Speed} = |v(t)|$
Acceleration and Deceleration: Positive acceleration indicates an increase in velocity, while negative acceleration (deceleration) indicates a decrease.

Graphical Interpretation

Understanding the graphical relationship between position, velocity, and acceleration is key for analyzing motion in AP Calculus.

1.Position vs. Time Graph: The slope of the tangent line at any point gives the instantaneous velocity. Upward slopes indicate forward motion, while downward slopes show backward motion.

Instantaneous Velocity: The slope of the tangent line at any point on a position vs. time graph represents the instantaneous velocity of the object at that moment.
Direction of Motion: If the graph slopes upward, the object is moving forward (positive velocity). If the graph slopes downward, the object is moving backward (negative velocity).
Overall Displacement: The difference in the y-values (position) between two points on the graph gives the displacement of the object over that time interval.

2. Velocity vs. Time Graph: The slope at any point represents instantaneous acceleration, and the area under the curve gives the displacement. The graph crossing the time axis indicates a change in direction.

Instantaneous Acceleration: The slope of the tangent line at any point on a velocity vs. time graph gives the instantaneous acceleration of the object. A positive slope indicates that the object is speeding up, while a negative slope indicates that it is slowing down.
Area Under the Curve: The area between the velocity graph and the time axis over a given interval represents the displacement of the object during that time. A positive area corresponds to forward movement, while a negative area indicates backward movement.
Crossing the Time Axis: When the velocity graph crosses the time axis (where v(t)=0), the object changes direction. Identifying these points helps in understanding when the object is at rest and when it reverses its motion.

3. Acceleration vs. Time Graph: The area under the graph shows the change in velocity. A horizontal line indicates constant acceleration, while the slope represents jerk (rate of change of acceleration).

Change in Velocity: The area under the acceleration vs. time graph over a given interval represents the change in velocity of the object during that time. A positive area indicates an increase in velocity (speeding up), while a negative area indicates a decrease (slowing down).
Constant Acceleration: If the acceleration graph is a horizontal line, the object is experiencing constant acceleration. If the line is at zero, the velocity is constant, meaning no acceleration is occurring.
Jerk (AP Calculus BC): The slope of the tangent line on an acceleration vs. time graph represents the jerk, or the rate at which acceleration is changing. This can be critical in understanding more complex motions where acceleration isn’t constant.

Problem Solving Strategies

When solving motion problems on the AP Calculus exam:

Identify the Given Function: Determine if you are given the position, velocity, or acceleration function.
Find Derivatives: Differentiate the position function to find velocity, and differentiate velocity to find acceleration.
Analyze Critical Points: Set the velocity function equal to zero to find when the object is at rest, and use the acceleration function to determine the nature of these points (i.e., whether the object is speeding up or slowing down).
Interpret Physical Meaning: Relate your mathematical findings (like derivatives and critical points) to the physical motion of the object.

Examples

Example 1: Tracking a Rocket’s Ascent

A rocket’s height above the ground is given by the function s(t) = 5t³−20t²+30t, where s(t) is the height in meters and t is time in seconds. To determine when the rocket reaches its maximum height, differentiate s(t) to find the velocity function v(t), set v(t) = 0, and solve for t. This tells you the time when the rocket stops ascending and begins to descend.

Example 2: Finding When a Car Stops

A car’s position is described by s(t) = t⁴−8t²+16. To determine when the car comes to a stop, find the velocity function $v(t) = \frac{ds(t)}{dt} = 4t^3 - 16t$ and set it equal to zero. Solving for t reveals the times when the car’s velocity is zero, indicating that it is momentarily at rest.

Example 3: Calculating Total Distance Traveled

A runner’s position is modeled by s(t) = t³−6t²+9t. To calculate the total distance traveled from t = 0 to t = 4, first find the velocity function and determine when it changes sign (indicating a change in direction). Then, integrate the absolute value of velocity over each interval to find the total distance.

Example 4: Determining Acceleration in a Bouncing Ball

The height of a bouncing ball is given by s(t) = −16t²+64t+10. To find the acceleration at any time t, differentiate twice to get a(t) = −32. This shows that the acceleration is constant and directed downward due to gravity, regardless of the ball’s height or velocity.

Example 5: Analyzing a Particle’s Motion with Jerk (AP Calculus BC)

For a particle moving according to s(t)=2t⁵−5t⁴+t³, differentiate to find the velocity, then differentiate again to find acceleration, and finally differentiate to find the jerk. This allows you to understand not only how the particle’s velocity and acceleration change but also how the rate of change of acceleration (jerk) affects the motion.

Multiple Choice Questions

Question 1

The position of a car moving along a straight road is given by s(t) = t²+ 2t, where s(t) is in meters and t is in seconds. What is the velocity of the car at t = 3 seconds?

A) 5 m/s
B) 8 m/s
C) 10 m/s
D) 12 m/s

Answer: D) 12 m/s

Explanation: The velocity is the derivative of the position function with respect to time. The position function is s(t) = t²+2t.

Differentiate s(t): v(t) = dtds(t) = 2t+2

Substitute t=3: v(3) = 2(3)+2 =6+2 =8 m/s

So, the velocity of the car at t = 3 seconds is 8 m/s.

Question 2

A particle moves along a line, and its velocity at time t is given by v(t)=3t−1. What is the acceleration of the particle?

A) 0 m/s²
B) 1 m/s²
C) 3 m/s²
D) 6 m/s²

Answer: C) 3 m/s²

Explanation: The acceleration is the derivative of the velocity function with respect to time. The velocity function is v(t)=3t−1.

Differentiate v(t): a(t)= dv(t)/dt =3

Since the derivative is a constant, the acceleration of the particle is 3 m/s².

Question 3

The velocity of a particle is given by v(t) = 4 m/s. What is the total distance traveled by the particle from t = 0 to t = 5 seconds?

A) 10 meters
B) 15 meters
C) 20 meters
D) 25 meters

Answer: D) 20 meters

Explanation: The velocity is constant at 4 m/s. To find the distance traveled over the time interval, multiply the velocity by the time:

Distance = v(t) × Δt = 4m/s×5seconds =20meters

So, the total distance traveled by the particle is 20 meters.