Connecting Differentiability and Continuity

The relationship between differentiability and continuity is a fundamental concept in calculus, crucial for mastering both AP Calculus AB and BC. Understanding this connection helps in analyzing the behavior of functions at specific points. While continuity ensures that a function has no breaks or jumps, differentiability goes a step further, requiring a smooth, non-cornered curve. A key principle is that differentiability implies continuity, but the reverse isn’t always true. Grasping this distinction is essential for solving various problems involving limits, derivatives, and the overall behavior of functions.

Learning Objectives

By studying Connecting Differentiability and Continuity for the AP Calculus AB and BC exams, you should aim to understand the precise relationship between these two concepts. You will learn that differentiability at a point implies continuity at that point, but continuity does not necessarily imply differentiability. You should also be able to identify points of non-differentiability on a graph, such as sharp corners or cusps, and explain why these points are continuous but not differentiable, using both graphical and algebraic methods.

Continuity

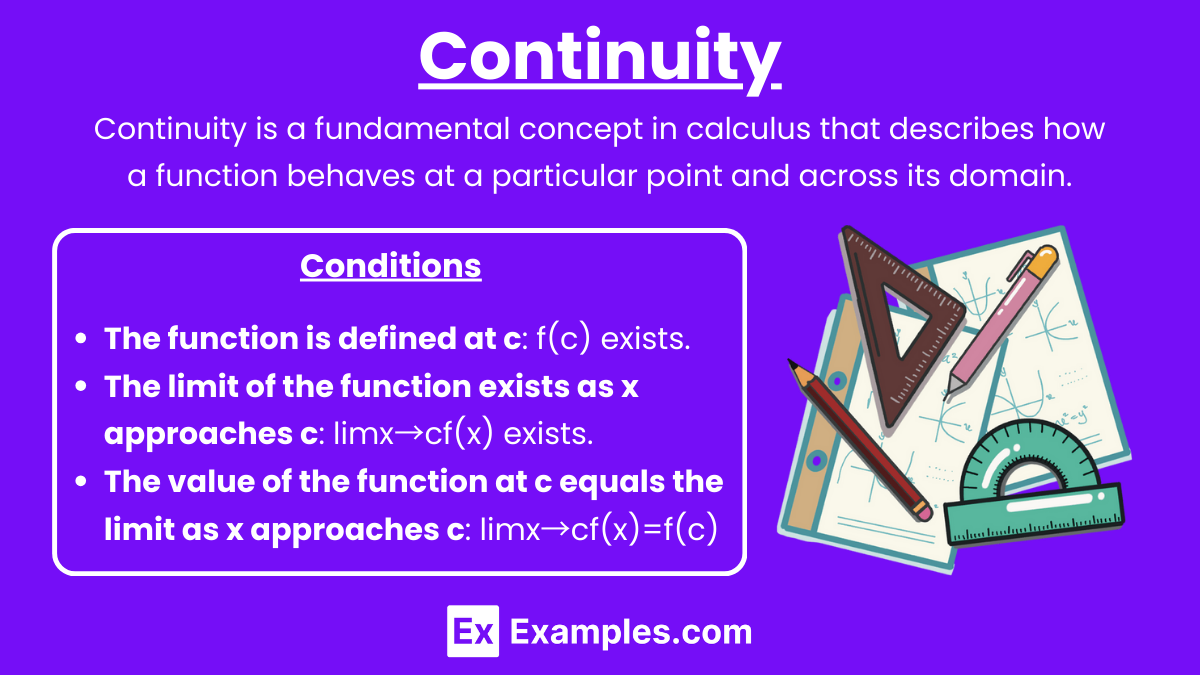
Continuity is a fundamental concept in calculus that describes how a function behaves at a particular point and across its domain. A function is continuous if its graph can be drawn without lifting your pen from the paper, meaning there are no breaks, holes, jumps, or interruptions in the graph at that point. A function is said to be continuous at a point x=c if the following three conditions are met:

• The function is defined at c: f(c) exists.
• The limit of the function exists as x approaches c: lim_x→c​f(x) exists.
• The value of the function at c equals the limit as x approaches c: lim_x→c​f(x)=f(c).

In simpler terms, a function is continuous at a point if there are no holes, jumps, or breaks in the graph at that point. A function is continuous over an interval if it is continuous at every point in that interval.

Differentiability

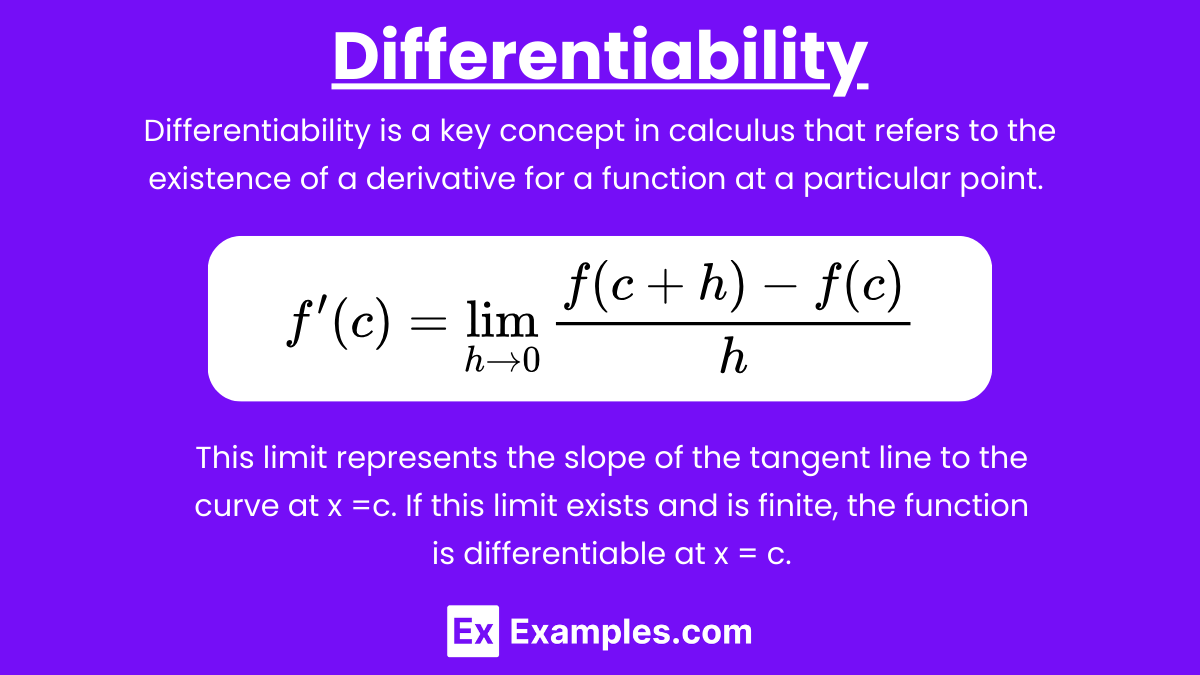
Differentiability is a key concept in calculus that refers to the existence of a derivative for a function at a particular point. If a function is differentiable at a point, it means the function has a well-defined tangent at that point, and the function’s graph is “smooth” without any sharp corners, cusps, or vertical tangents at that point. A function f(x) is differentiable at a point x=c if the derivative f′(c) exists at that point. Mathematically, this means:

This limit represents the slope of the tangent line to the curve at x =c. If this limit exists and is finite, the function is differentiable at x = c.

Relationship Between Differentiability and Continuity

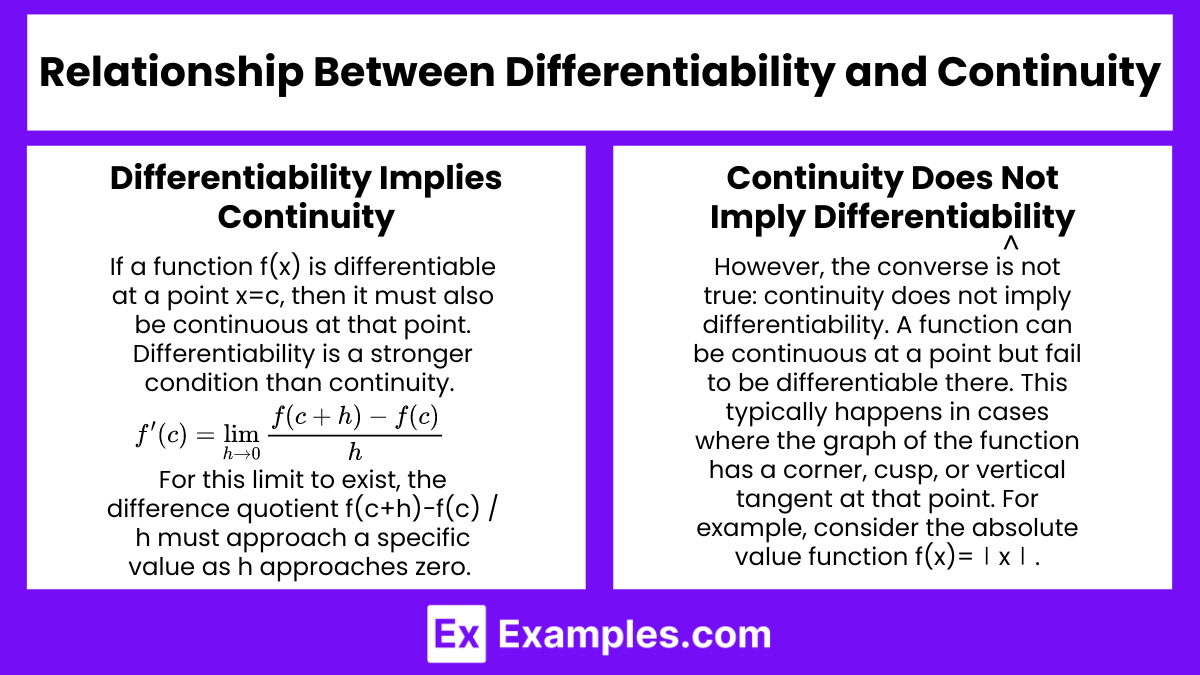
Differentiability Implies Continuity

If a function f(x) is differentiable at a point x=c, then it must also be continuous at that point. Differentiability is a stronger condition than continuity. To be differentiable at x=c, the derivative of f(x) at x=c must exist, which is defined as:

For this limit to exist, the difference quotient f(c+h)−f(c)​ / h must approach a specific value as h approaches zero. This requires that f(c+h) gets arbitrarily close to f(c) as h approaches zero. In other words, the function must not have any jumps, breaks, or discontinuities at x=c.

Mathematically, this implies that:

which is the condition for continuity at x=c. Therefore, differentiability at a point guarantees continuity at that point.

Continuity Does Not Imply Differentiability

However, the converse is not true: continuity does not imply differentiability. A function can be continuous at a point but fail to be differentiable there. This typically happens in cases where the graph of the function has a corner, cusp, or vertical tangent at that point.

For example, consider the absolute value function f(x)=∣x∣. This function is continuous at x=0 because there is no break or jump in the graph, and the left-hand limit and right-hand limit both equal f(0)=0. However, f(x) is not differentiable at x=0 because the slope of the graph changes abruptly from negative to positive as x moves through zero. The left-hand derivative (as x approaches 0 from the left) is −1, while the right-hand derivative (as x approaches 0 from the right) is +1. Since these two one-sided derivatives do not match, the overall derivative at x=0 does not exist.

Examples

Example 1: Polynomials

Consider the polynomial function f(x) = x². Since polynomials are smooth and do not have any sharp corners or breaks, they are both continuous and differentiable at every point on their domain. For instance, at x = 1, the function is continuous, and the derivative f′(x) = 2x exists and is also continuous. This demonstrates that the function’s differentiability guarantees its continuity.

Example 2: Absolute Value Function

The function f(x) = ∣x∣ is continuous everywhere but not differentiable at x = 0. At this point, the graph of f(x) has a sharp corner, causing the left-hand derivative f′(−0) = −1 and the right-hand derivative f′(+0) = 1 to differ. Since the derivative does not exist at x = 0, the function is not differentiable there. However, the function remains continuous at that point, illustrating that continuity does not necessarily imply differentiability.

Example 3: Sine Function

The function f(x) = sin(x) is continuous and differentiable for all real numbers. The derivative f′(x) = cos(x) also exists and is continuous. For instance, at x = π/4, sin(π/4) is continuous, and the derivative cos(π/4) is well-defined and continuous. This example shows that differentiability leads to continuity across the entire domain of the sine function.

Example 4: Piecewise Function

Consider a piecewise function defined as f(x) = x² for x≥0 and f(x)=−x² for x<0. Although this function is continuous at x = 0, it is not differentiable there. The derivative from the left-hand side does not equal the derivative from the right-hand side, meaning the derivative does not exist at x = 0. This highlights how a function can be continuous at a point but not differentiable.

Example 5: Cubic Root Function

The function f(x) = x^1/3 is continuous everywhere, including at x = 0. However, its derivative f′(x) = 1/3 ​x^−2/3 becomes infinite as x approaches zero, indicating that while the function is continuous at x = 0, it is not differentiable there. This example shows that while continuity is a necessary condition for differentiability, it is not sufficient; the function must also have a finite derivative at that point.

Multiple Choice Questions

Question 1

If a function f(x) is differentiable at x=c, which of the following statements is true?

A) f(x) must be continuous at x=c.
B) f(x) is not necessarily continuous at x=c.
C) f(x) must be discontinuous at x=c.
D) f(x) must have a sharp corner at x=c.

Correct Answer: A) f(x) must be continuous at x=c.

Explanation: Differentiability implies continuity. If a function is differentiable at a point x=c, it must also be continuous at that point. Differentiability is a stronger condition than continuity, meaning that while every differentiable function is continuous, not every continuous function is differentiable. Therefore, the correct answer is A.

Question 2

Which of the following functions is an example of a function that is continuous at x=0 but not differentiable at x=0?

A) f(x) = x2
B) f(x) = ∣x∣
C) f(x) = sin(x)
D) f(x) = x3

Correct Answer: B) f(x) = ∣x∣

Explanation: The function f(x) = ∣x∣ is continuous everywhere, including at x = 0, because there is no jump, gap, or break in the graph of the function. However, f(x) = ∣x∣ is not differentiable at x = 0 because the slope of the graph changes abruptly at that point, resulting in a sharp corner. The derivative from the left-hand side does not equal the derivative from the right-hand side at x = 0, meaning the derivative does not exist there. Therefore, the correct answer is B.

Question 3

If a function f(x) is continuous but not differentiable at x=c, what can be inferred about f(x) at x=c?

A) f(x) has a jump discontinuity at x=c.
B) f(x) has a vertical tangent at x=c.
C) f(x) has a sharp corner or cusp at x=c.
D) f(x) is differentiable at x=c.

Correct Answer: C) f(x) has a sharp corner or cusp at x=c.

Explanation: A function can be continuous but not differentiable at a point if there is a sharp corner or cusp at that point. A sharp corner means the function has different slopes approaching the point from the left and right, so the derivative does not exist there. This is a common scenario where continuity does not imply differentiability. Hence, the correct answer is C.