Definitions of Continuity of a Function at a Point and over a Domain

Last Updated: September 23, 2024

Notes

Continuity is a fundamental concept in AP Calculus that ensures a function behaves predictably without any abrupt jumps or gaps. For a function to be continuous at a point, it must be well-defined, its limit must exist as the input approaches that point, and the function’s value at that point must match the limit. Understanding continuity over a domain extends this idea, ensuring the function is smooth and uninterrupted across an entire interval, which is crucial in both AP Calculus AB and BC.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

When studying the Definitions of Continuity of a Function at a Point and over a Domain for the AP Calculus AB exam, you should aim to understand how to determine if a function is continuous at a specific point and over an interval. Focus on learning the three key conditions for continuity at a point, identifying different types of discontinuities (removable, jump, and infinite), and applying the Intermediate Value Theorem. Additionally, practice evaluating the continuity of piecewise, polynomial, and rational functions to solidify your understanding.

Continuity of a Function: Definitions at a Point and Over a Domain

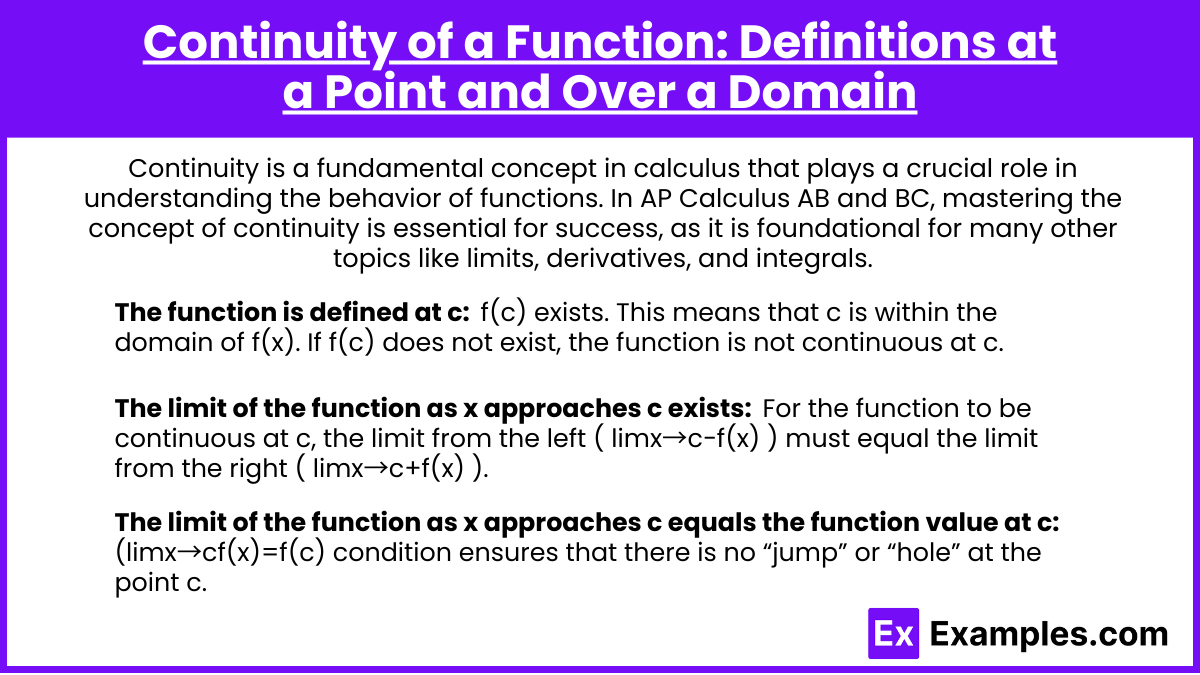
Continuity is a fundamental concept in calculus that plays a crucial role in understanding the behavior of functions. In AP Calculus AB and BC, mastering the concept of continuity is essential for success, as it is foundational for many other topics like limits, derivatives, and integrals. Here’s a detailed explanation of continuity, both at a point and over a domain.Continuity of a Function at a Point

A function f(x) is said to be continuous at a point c if the following three conditions are met:

The function is defined at c: f(c) exists. This means that c is within the domain of f(x). If f(c) does not exist, the function is not continuous at c.
The limit of the function as x approaches c exists: $\left(\lim_{x \to c} f(x) \text{ exists.}\right)$
For the function to be continuous at c, the limit from the left ( limx→c−f(x) ) must equal the limit from the right ( limx→c+f(x) ).
The limit of the function as x approaches c equals the function value at c: $\left(\lim_{x \to c} f(x) = f(c)\right)$ . This condition ensures that there is no “jump” or “hole” at the point c.

Summary of Continuity at a Point

For a function f(x) to be continuous at a point c:

f(c) is defined.
limx→cf(x) exists.
limx→cf(x)=f(c).

If any of these conditions are not satisfied, the function is not continuous at c, and we say the function has a discontinuity at that point.

Types of Discontinuities

Understanding different types of discontinuities is important, as it helps to identify where and why a function is not continuous.

Removable Discontinuity:
Occurs when the limit limx→cf(x) exists, but f(c) is either undefined or not equal to this limit. This type of discontinuity can be “fixed” by redefining f(c).
Jump Discontinuity:
Occurs when the left-hand limit limx→c−f(x) and the right-hand limit limx→c+f(x) exist but are not equal. The function has an abrupt change or “jump” at c.
Infinite Discontinuity:
Occurs when limx→cf(x) approaches infinity or negative infinity, meaning the function grows without bound as it approaches c.

Continuity of a Function Over a Domain

A function is continuous over a domain if it is continuous at every point in that domain. For the AP Calculus exam, you’ll need to understand what this means for various types of domains, particularly on open intervals, closed intervals, and entire real lines. Continuity on an Interval.

Continuous on an Open Interval (a,b):
A function f(x) is continuous on the open interval (a,b) if it is continuous at every point c within the interval a<c<b.
The function is defined at c: f(c) exists. The limit of the function as it approaches c exists: limx→cf(x) exists. The limit equals the function value at c: limx→cf(x)=f(c).
Continuous on a Closed Interval [a,b]:
A function f(x) is continuous on the closed interval [a,b] if:
- It is continuous at every point within the interval (a,b),
- limx→a+f(x)=f(a) (right-hand limit equals the function value at a),
- limx→b−f(x)=f(b) (left-hand limit equals the function value at b).

Special Cases and Theorems Related to Continuity

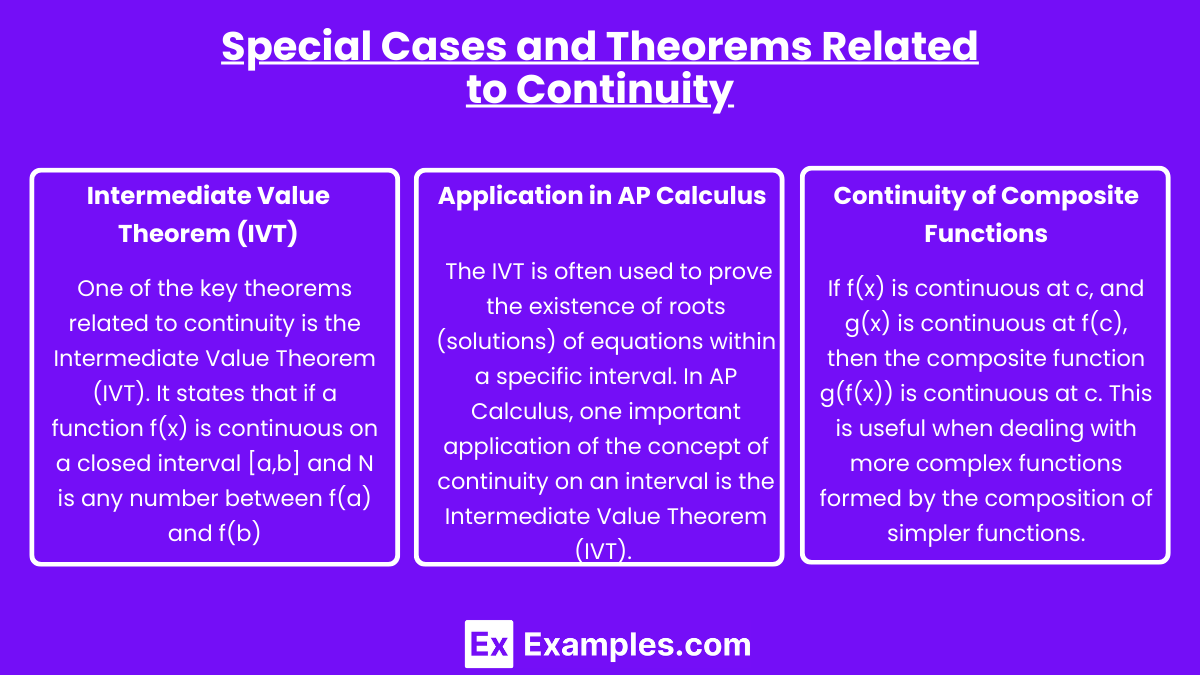
Intermediate Value Theorem (IVT) : One of the key theorems related to continuity is the Intermediate Value Theorem (IVT). It states that if a function f(x) is continuous on a closed interval [a,b] and N is any number between f(a) and f(b), then there exists at least one point c in (a,b) such that f(c)=N.

Application in AP Calculus: The IVT is often used to prove the existence of roots (solutions) of equations within a specific interval. In AP Calculus, one important application of the concept of continuity on an interval is the Intermediate Value Theorem (IVT). The IVT states that if a function f(x) is continuous on a closed interval [a,b] and if f(a) and f(b) have opposite signs, then there exists at least one point ccc in the open interval (a,b) such that f(c)=0.

Continuity of Composite Functions : If f(x) is continuous at c, and g(x) is continuous at f(c), then the composite function g(f(x)) is continuous at c. This is useful when dealing with more complex functions formed by the composition of simpler functions.

Examples

Example 1: Continuity at a Point c:

A function f(x) is continuous at a point c if the limit of f(x) as x approaches c exists and equals the function’s value at that point. Mathematically, this means limx→cf(x)=f(c). This ensures there is no sudden jump, break, or hole in the function at c.

Example 2: Continuity over an Open Interval

A function f(x) is continuous over an open interval (a,b) if it is continuous at every point within that interval. This implies that as x moves within (a,b), the function smoothly transitions from one value to another without any interruptions or undefined points.

Example 3: Continuity over a Closed Interval

For a function to be continuous over a closed interval [a,b], it must be continuous at every point within the open interval (a,b), continuous from the right at x=a, and continuous from the left at x=b. This ensures the function behaves consistently across the entire domain, including the endpoints.

Example 4: Continuity at a Removable Discontinuity

A function f(x) may have a removable discontinuity at a point c if the limit limx→cf(x) exists, but f(c) is either undefined or does not equal the limit. Continuity can be restored by redefining f(c) to equal the limit, thereby “removing” the discontinuity.

Example 5: Continuity on the Entire Real Line

A function f(x) is said to be continuous on the entire real line if it is continuous at every point x in (−∞,∞). This means there are no breaks, jumps, or points of discontinuity anywhere on the graph of the function, ensuring smooth, unbroken behavior across all real numbers.

Multiple Choice Questions

Question 1

Which of the following conditions must be satisfied for a function f(x) to be continuous at a point x=c?

A) f(c) is defined, limx→cf(x) exists, and f(c)=limx→cf(x).
B) f(c) is undefined, but limx→cf(x) exists.
C) f(c) is defined, limx→cf(x) does not exist, and f(c) equals some finite value.
D) f(c) is defined, but limx→cf(x) does not exist.

Answer: A)

Explanation: For a function f(x) to be continuous at a point x=c, the following three conditions must be met:

f(c) is defined.
The limit limx→cf(x) exists.
The value of the function at c is equal to the limit, i.e., f(c)=limx→cf(x).

Option A is correct because it includes all these three essential conditions. Options B, C, and D are incorrect because they each miss at least one of these conditions, which are necessary for continuity at a point.

Question 2

Which of the following statements is true regarding the continuity of a function over an interval?

A) A function is continuous over an interval if it is continuous at every point within the interval.
B) A function is continuous over an interval if it is continuous at the endpoints of the interval only.
C) A function is continuous over an interval if it is discontinuous at only one point within the interval.
D) A function is continuous over an interval if it is differentiable at every point within the interval.

Answer: A)

Explanation: A function is continuous over an interval if it is continuous at every point within that interval. This means that for every point c within the interval, the function satisfies the three conditions for continuity (as described in Question 1).

Option A is correct because it accurately describes the condition for continuity over an interval. Option B is incorrect because continuity at just the endpoints does not guarantee continuity over the entire interval. Option C is incorrect because a single discontinuity within the interval means the function is not continuous over the interval. Option D is incorrect because differentiability implies continuity, but continuity does not necessarily imply differentiability.

Question 3

If a function f(x) is not continuous at a point x=a, which of the following could be true?

A) f(a) is not defined.
B) limx→af(x) does not exist.
C) limx→af(x) exists, but f(a)=limx→af(x).
D) All of the above.

Answer: D)

Explanation: A function f(x) can fail to be continuous at a point x=a for several reasons:

Option A: f(a) is not defined. If the function is not defined at the point, it cannot be continuous there.
Option B: limx→af(x) does not exist. If the limit doesn’t exist, the function cannot be continuous at a.
Option C: limx→af(x) exists, but f(a)=limx→af(x). If the function’s value at a doesn’t match the limit, continuity at a is violated.

Therefore, Option D is correct because any of these scenarios would result in the function being discontinuous at x=a.