Derivatives and Properties of Functions

Last Updated: September 23, 2024

Notes

Derivatives are fundamental in calculus, representing the rate of change of a function with respect to its variable. They provide insight into the behavior of functions, including their slopes, rates of change, and concavity. Understanding derivatives is essential for analyzing the properties of functions, such as identifying critical points, determining maxima and minima, and solving real-world problems involving motion, growth, and optimization. Mastery of derivative concepts is crucial for success in both AP Calculus AB and BC exams.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

By studying Derivatives and Properties of Functions for the AP Calculus exam, you should aim to master the basic derivative rules (power, product, quotient, chain), understand the derivatives of trigonometric functions, and apply implicit differentiation. You should also focus on analyzing the behavior of functions using first and second derivatives to determine critical points, extrema, concavity, and points of inflection. Additionally, you need to develop problem-solving skills for optimization and related rates problems, which are crucial for both AP Calculus AB and BC.

Derivatives and Properties of Functions: AP Calculus AB & BCIntroduction

Derivatives form the foundation of calculus and play a crucial role in understanding the behavior of functions. In both AP Calculus AB and AP Calculus BC, mastering derivatives and their properties is essential for success. This guide covers key concepts, rules, and applications of derivatives relevant to both courses, providing the depth needed to excel in the AP Calculus exam.

What is a Derivative?

Derivative of a function represents the rate at which the function’s value changes concerning its independent variable. It provides information on the function’s slope or steepness at any given point.

Mathematically, if y=f(x), the derivative of f with respect to x is denoted as dxdy or f′(x), and is defined as:

$f'(x) = \lim_{\Delta x \to 0} \frac{f(x + \Delta x) - f(x)}{\Delta x}$

Basic Derivative Rules

1. Power Rule

For any function of the form f(x) = xⁿ, where n is a real number: $f'(x) = nx^{n-1}$

2. Constant Rule

The derivative of a constant function f(x) = c, where c is a constant: f′(x) = 0

3. Constant Multiple Rule

If f(x) = c⋅g(x), where c is a constant and g(x) is a differentiable function: f′(x) = c⋅g′(x)

4. Sum and Difference Rule

If f(x) = g(x)±h(x), where g(x) and h(x) are differentiable: f′(x) = g′(x)±h′(x) Advanced Derivative Rules (AP Calculus BC Focus)

5. Product Rule

If f(x) = g(x)⋅h(x), where g(x) and h(x) are differentiable: f′(x)=g′(x)⋅h(x)+g(x)⋅h′(x)

6. Quotient Rule

If f(x)=h(x)g(x), where g(x) and h(x) are differentiable and $h(x) = \frac{f(x)}{g(x)}$

7. Chain Rule

If a function is composed of two functions f(x) = g(h(x)), then: f′(x) = g′(h(x))⋅h′(x)

This rule is particularly important in dealing with composite functions and implicit differentiation.Derivatives of Trigonometric Functions

Understanding the derivatives of trigonometric functions is essential in both AP Calculus AB and BC:

$\frac{d}{dx} (\sin x) = \cos x$
$\frac{d}{dx} (\cos x) = -\sin x$
$\frac{d}{dx} (\tan x) = \sec^2 x$
$\frac{d}{dx} (\csc x) = -\csc x \cot x$
$\frac{d}{dx} (\sec x) = \sec x \tan x$
$\frac{d}{dx} (\cot x) = -\csc^2 x$

Implicit Differentiation

Implicit differentiation is used when the function is not explicitly solved for one variable. For example, for the equation x²+y² = 1 (the equation of a circle):

Differentiate both sides with respect to x, treating y as a function of x (i.e., apply the chain rule to y).
Solve for dxdy:

$\frac{d}{dx} \left( x^2 + y^2 \right) = \frac{d}{dx} (1)$

$2x + 2y \frac{dy}{dx} = 0$

$\frac{dy}{dx} = \frac{-x}{y}$

Higher-Order Derivatives

Higher-order derivatives are derivatives of derivatives. The second derivative, denoted as f′′(x) or dx2d2y, provides information about the concavity of the function. For example:

First derivative f′(x): Indicates the slope of the function.
Second derivative f′′(x): Indicates the concavity (concave up or down).

Applications of Derivatives

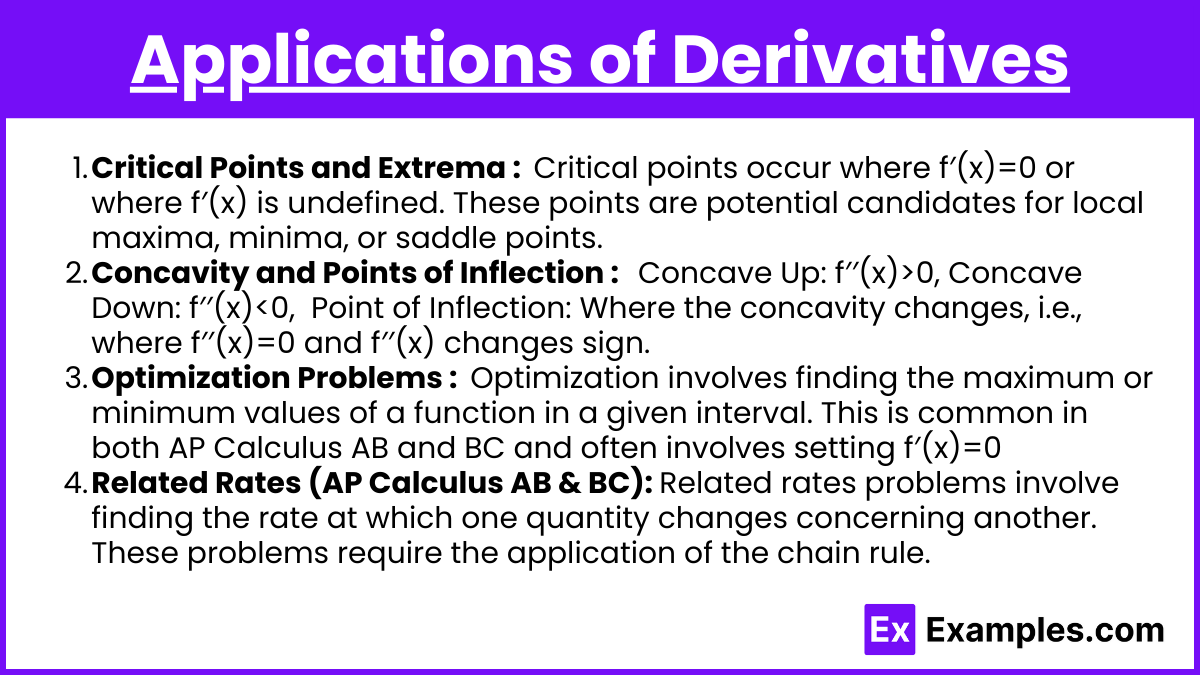
1. Critical Points and Extrema

Critical points occur where f′(x)=0 or where f′(x) is undefined. These points are potential candidates for local maxima, minima, or saddle points.

Local Maximum: Occurs if f′(x) changes from positive to negative.
Local Minimum: Occurs if f′(x) changes from negative to positive.

2. Concavity and Points of Inflection

Concave Up: f′′(x)>0
Concave Down: f′′(x)<0
Point of Inflection: Where the concavity changes, i.e., where f′′(x)=0 and f′′(x) changes sign.

3. Optimization Problems

Optimization involves finding the maximum or minimum values of a function in a given interval. This is common in both AP Calculus AB and BC and often involves setting f′(x)=0 to find critical points and using the second derivative test to determine the nature of these points.

4. Related Rates (AP Calculus AB & BC)

Related rates problems involve finding the rate at which one quantity changes concerning another. These problems require the application of the chain rule.

Example: If a balloon is being inflated such that its radius r is increasing at a rate of 2 cm/s, find the rate at which the volume V of the balloon is increasing when the radius is 5 cm.

Given V = 4/3 πr³, differentiate both sides with respect to time t:

$\frac{dV}{dt} = 4\pi r^2 \frac{dr}{dt}$

Substitute r = 5 and dr/dt = 2:

$\frac{dV}{dt} = 4\pi (5^2)(2) = 200\pi \ \text{cm}^3/\text{s}$

Examples

Example 1: Derivative of a Constant Function

Consider the function f(x) = 5. Since the value of f(x) is constant for all x, the derivative is zero. This is because a constant function has no slope or rate of change. Therefore, f′(x) = 0.

Example 2: Derivative of a Linear Function

Take the function f(x) = 3x+2. The derivative of a linear function is simply the coefficient of x. Here, the derivative f′(x) = 3, meaning the slope of the line is 3.

Example 3: Power Rule

For the function f(x) = x⁴, use the power rule to find the derivative. According to the power rule, bring down the exponent and subtract one from it. The derivative is f′(x) = 4x³.

Example 4: Sum Rule

Consider the function f(x) = x²+4x+1. The sum rule states that the derivative of a sum of functions is the sum of their derivatives. So, f′(x) = 2x+4.

Example 5: Product Rule

For two functions multiplied together, such as f(x) = x² × sin(x), the derivative is found using the product rule. The derivative is f′(x) = 2x × sin(x) + x² × cos(x).

Multiple Choice Questions

Question 1

What is the derivative of the function f(x) = 5x³?

A) 15x²
B) 15x³
C) 5x²
D) 10x²

Answer: A) 15x²

Explanation: To find the derivative of f(x) = 5x³, apply the power rule. The derivative is f′(x) = 3⋅5x³⁻¹ = 15x².

Question 2

If f(x) = x²+3x, what is the value of f′(2)?

A) 7
B) 10
C) 11
D) 14

Answer:
B) 7

Explanation: First, find the derivative f′(x) = 2x+3. Then, substitute x = 2 into the derivative: f′(2) = 2(2) + 3 = 4 + 3 = 7.

Question 3

Which of the following functions has a derivative of 4x?

A) x⁴
B) 2x²
C) 4x²
D) x²

Answer: B) 2x²

Explanation: The derivative of 2x² is found by applying the power rule: d/dx (2x²) = 2⋅2x²⁻¹ = 4x.