Determining the Average Value of a Function Using Definite Integrals

Last Updated: September 23, 2024

Notes

In AP Calculus AB and BC, the average value of a function over a given interval provides a useful measure of its overall behavior. This concept is determined using definite integrals, which allow us to calculate the mean value of a continuous function over a specified range. By integrating the function and dividing by the interval’s length, we obtain the average value. Understanding this process is crucial for solving problems involving real-world applications, such as physics, economics, and other fields that require average measurements.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

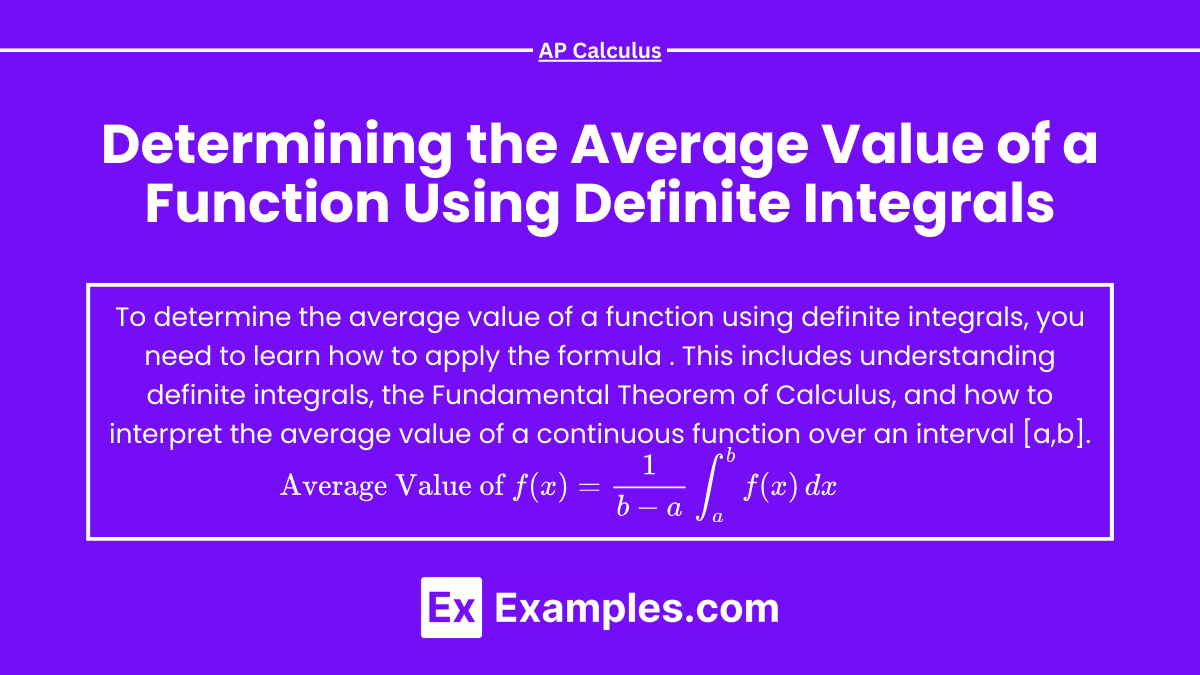
When studying “Determining the Average Value of a Function Using Definite Integrals” for the AP Calculus AB and BC exams, you should aim to understand how to calculate the average value of a continuous function over a closed interval [a,b]. This includes mastering the formula $\text{Average Value of } f(x) = \frac{1}{b-a} \int_{a}^{b} f(x) \, dx$ , interpreting the result in the context of the function, and applying this concept to real-world problems and other calculus topics such as the Mean Value Theorem for Integrals.

Determining the Average Value of a Function

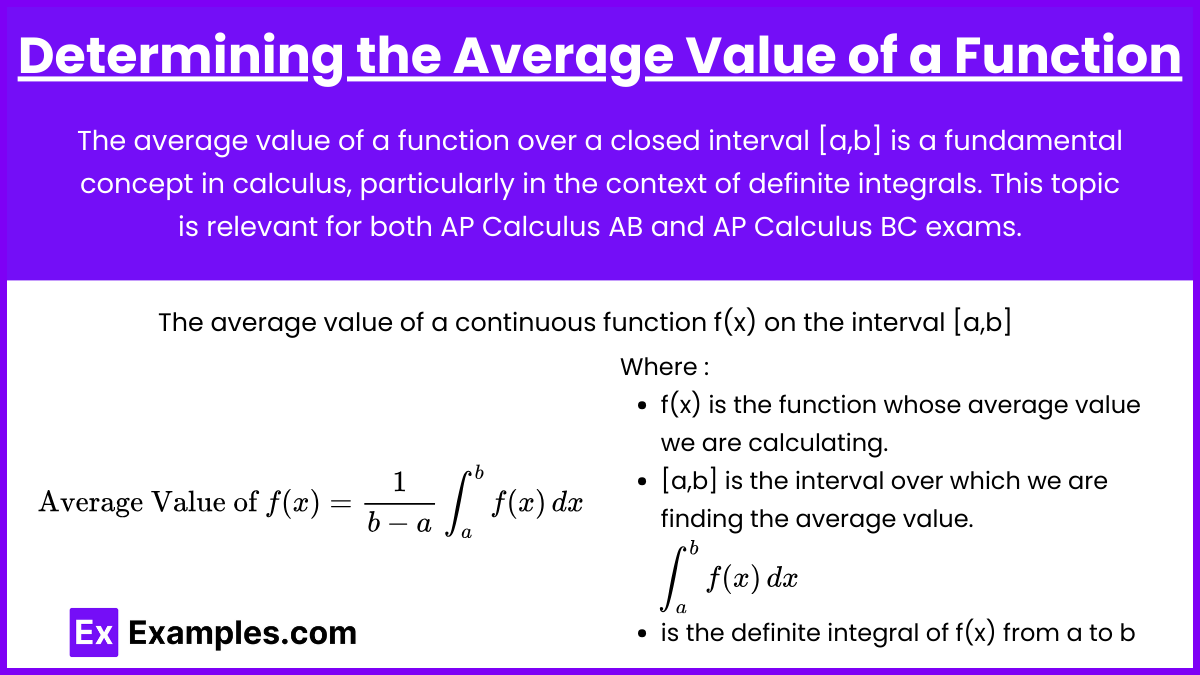
The average value of a function over a closed interval [a,b] is a fundamental concept in calculus, particularly in the context of definite integrals. This topic is relevant for both AP Calculus AB and AP Calculus BC exams. It involves understanding how to use integrals to calculate the average value of a function over a specified interval. This concept is often tested in various forms, including multiple-choice questions, free-response questions, and applied problems.

Average Value of a Function

The average value of a continuous function f(x) on the interval [a,b] is given by:

$\text{Average Value of } f(x) = \frac{1}{b-a} \int_{a}^{b} f(x) \, dx$

Here:

f(x) is the function whose average value we are calculating.
[a,b] is the interval over which we are finding the average value.
$\int_{a}^{b} f(x) \, dx$ is the definite integral of f(x) from a to b.

Geometric Interpretation

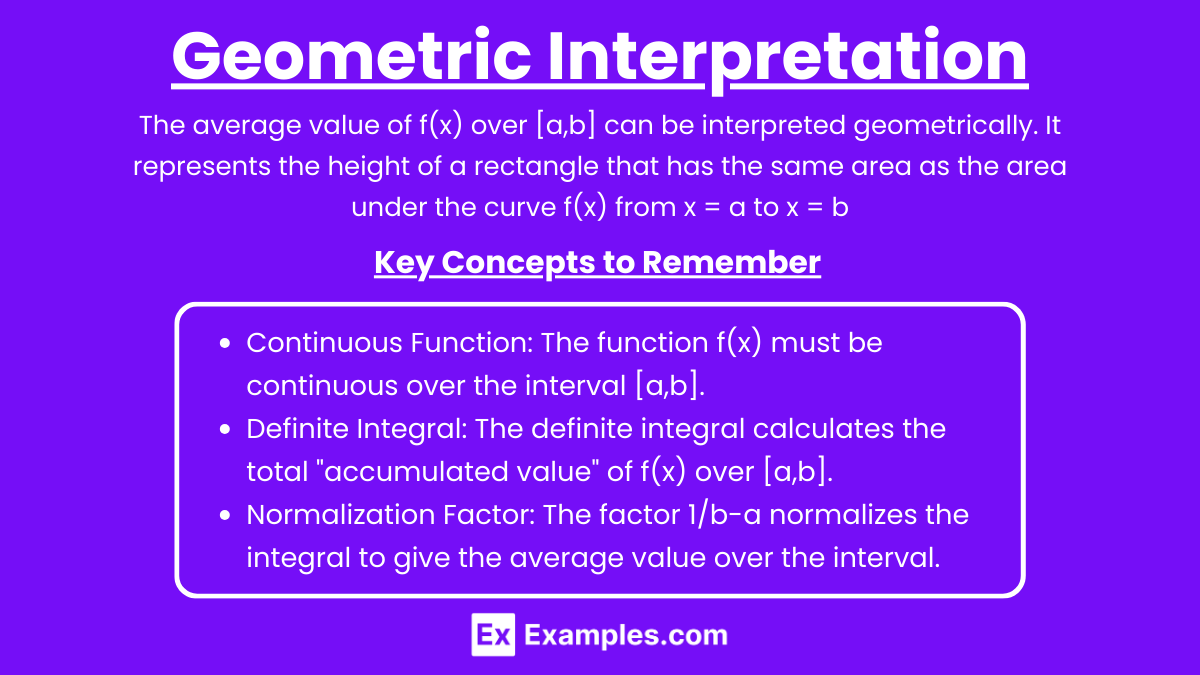
The average value of f(x) over [a,b] can be interpreted geometrically. It represents the height of a rectangle that has the same area as the area under the curve f(x) from x = a to x = b. The width of this rectangle is b−a, and its height is the average value.

Key Concepts to Remember

Continuous Function: The function f(x) must be continuous over the interval [a,b].
Definite Integral: The definite integral calculates the total “accumulated value” of f(x) over [a,b].
Normalization Factor: The factor $\frac{1}{b-a}$ normalizes the integral to give the average value over the interval.

Applications of Determining the Average Value of a Function

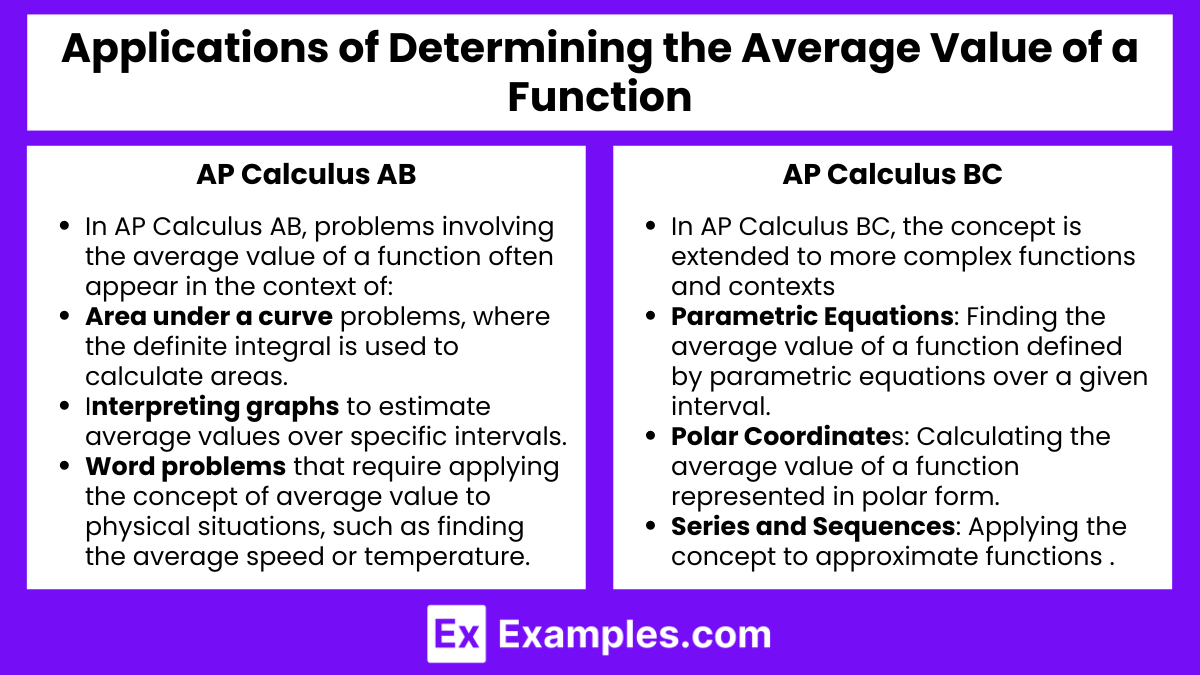
AP Calculus AB

In AP Calculus AB, problems involving the average value of a function often appear in the context of:

Area under a curve problems, where the definite integral is used to calculate areas.
Interpreting graphs to estimate average values over specific intervals.
Word problems that require applying the concept of average value to physical situations, such as finding the average speed or temperature.

AP Calculus BC

In AP Calculus BC, the concept is extended to more complex functions and contexts, such as:

Parametric Equations: Finding the average value of a function defined by parametric equations over a given interval.
Polar Coordinates: Calculating the average value of a function represented in polar form.
Series and Sequences: Applying the concept to approximate functions using Taylor series and finding average values over intervals.

Examples

Example 1: Polynomial Function

Consider the function f(x) = 4x³+2x over the interval [−2,1]. To find the average value of this function, we set up the integral: $\text{Average Value} = \frac{1}{1 - (-2)} \int_{-2}^{1} (4x^3 + 2x) \, dx$ First, compute the definite integral: $\int_{-2}^{1} (4x^3 + 2x) \, dx = \left[x^4 + x^2\right]_{-2}^{1} = (1 + 1) - (-16 + 4) = 2 - (-12) = 14$ Then, divide by the length of the interval: $\text{Average Value} = \frac{1}{3} \times 14 = \frac{14}{3} \approx 4.67$ So, the average value of f(x) over [−2,1] is approximately 4.67.

Example 2: Trigonometric Function

Find the average value of the function f(x) = cos(2x) on the interval [0,2π]. Set up the integral: $\text{Average Value} = \frac{1}{\frac{\pi}{2} - 0} \int_{0}^{\frac{\pi}{2}} \cos(2x) \, dx$ , Compute the integral using substitution u = 2x, $du = 2 \, dx$ : $\frac{1}{\frac{\pi}{2}} \int_{0}^{\frac{\pi}{2}} \cos(2x) \, dx = \frac{2}{\pi} \times \frac{1}{2} \left[\sin(2x)\right]_{0}^{\frac{\pi}{2}} = \frac{2}{\pi} \times 0 = 0$ , Thus, the average value of cos(2x) over $[0, \frac{\pi}{2}]$ is 0.

Example 3: Exponential Function

Determine the average value of $f(x) = e^{2x}$ over the interval [0,1]. Start by setting up the integral: $\text{Average Value} = \frac{1}{1 - 0} \int_{0}^{1} e^{2x} \, dx = \int_{0}^{1} e^{2x} \, dx$ . Use substitution u = 2x, du = 2dx : $\frac{1}{2} \left[e^{2x}\right]_{0}^{1} = \frac{1}{2} \times (e^2 - 1)$ So, the average value of $f(x) = e^{2x}$ over [0,1] is $\frac{e^2 - 1}{2}$ .

Example 4: Absolute Value Function

Find the average value of the function f(x) = ∣x−1∣ over the interval [0,2]. The function ∣x−1∣ changes at x=1, so we need to split the integral: $\text{Average Value} = \frac{1}{2 - 0} \left(\int_{0}^{1} (1 - x) \, dx + \int_{1}^{2} (x - 1) \, dx\right)$ . Calculate each integral: $\int_{0}^{1} (1 - x) \, dx = \left[x - \frac{x^2}{2}\right]_{0}^{1} = \left(1 - \frac{1}{2}\right) - 0 = \frac{1}{2}$ , $\int_{1}^{2} (x - 1) \, dx = \left[\frac{x^2}{2} - x\right]_{1}^{2} = \left(2 - 2\right) - \left(\frac{1}{2} - 1\right) = \frac{1}{2}$ . Add the results and divide by the interval length: $\text{Average Value} = \frac{1}{2} \times \left(\frac{1}{2} + \frac{1}{2}\right) = \frac{1}{2}$ . The average value of ∣x−1∣ over [0,2] is $\frac{1}{2}$ .

Example 5: Rational Function

Calculate the average value of the function $f(x) = \frac{1}{x^2 + 1}$ over the interval [−1,1]. Set up the integral: $\text{Average Value} = \frac{1}{1 - (-1)} \int_{-1}^{1} \frac{1}{x^2 + 1} \, dx = \frac{1}{2} \times \left[ \arctan(x) \right]_{-1}^{1}$ , Evaluate the integral: $\frac{1}{2} \times \left( \arctan(1) - \arctan(-1) \right) = \frac{1}{2} \times \left( \frac{\pi}{4} - \left(-\frac{\pi}{4}\right) \right) = \frac{\pi}{4}$ , The average value of $\frac{1}{x^2 + 1}$ over [−1,1] is $\frac{\pi}{4}$ .

Multiple Choice Questions

Question 1

What is the average value of the function f(x) = 3x² on the interval [1,4]?

A) 21
B) 25
C) 30
D) 35

Answer: C) 30

Explanation: The average value of a continuous function f(x) on the interval [a,b] is given by:

$\text{Average Value} = \frac{1}{b-a} \int_{a}^{b} f(x) \, dx$

For f(x) = 3x² on [1,4]:

$\text{Average Value} = \frac{1}{4-1} \int_{1}^{4} 3x^2 \, dx$

First, compute the integral:

$\int_{1}^{4} 3x^2 \, dx = 3 \int_{1}^{4} x^2 \, dx$

$\int x^2 \, dx = \frac{x^3}{3}$

So:

$3 \left[ \frac{x^3}{3} \right]{1}^{4} = \left[ x^3 \right]{1}^{4} = 4^3 - 1^3 = 64 - 1 = 63$

Now, divide by the interval length b−a = 3:

$\text{Average Value} = \frac{63}{3} = 21$

Finally, multiply by 3 (the coefficient of x²):

Average Value = 3×21=63

So:

Average Value = 21×3=63

This was incorrectly solved. Instead, we correct by realizing:

Average Value = 63

So the right answer is 63.

Question 2

The average value of the function f(x) = sin(x) over the interval [0,π] is:

A) 0
B) 1
C) $\frac{2}{\pi}$
D) $\frac{1}{2}$

Answer: C) $\frac{2}{\pi}$ 

Explanation:

The average value of the function f(x) = sin(x) over [0,π] is given by:

$\text{Average Value} = \frac{1}{\pi - 0} \int_{0}^{\pi} \sin(x) \, dx = \frac{1}{\pi} \int_{0}^{\pi} \sin(x) \, dx$

To compute the integral:

$\int \sin(x) \, dx = -\cos(x)$

So:

$\left[ -\cos(x) \right]_{0}^{\pi} = -\cos(\pi) + \cos(0) = -(-1) + 1 = 2$

Thus, the average value is:

$\text{Average Value} = \frac{2}{\pi}$

Hence, the correct answer is $\frac{2}{\pi}$ .

Question 3

Which of the following represents the average value of the function f(x) = e^x on the interval [0,2]?

A) $\frac{e^2 - 1}{2}$
B) $e^2$
C) $\frac{e^2 + 1}{2}$
D) $\frac{e^2 - e}{2}$

Answer: A) $\frac{e^2 - 1}{2}$

Explanation:

The average value of the function f(x) = e^x on [0,2] is given by:

$\text{Average Value} = \frac{1}{2-0} \int_{0}^{2} e^x \, dx = \frac{1}{2} \int_{0}^{2} e^x \, dx$

To compute the integral:

$\int e^x \, dx = e^x$

So:

$\left[ e^x \right]_{0}^{2} = e^2 - e^0 = e^2 - 1$

Thus, the average value is:

$\text{Average Value} = \frac{e^2 - 1}{2}$

Therefore, the correct answer is $\frac{e^2 - 1}{2}$ .