Finding the Area Between Curves
In AP Calculus AB and BC, finding the area between curves is a key application of definite integrals. This process involves calculating the region enclosed between two functions over a given interval. By integrating the difference between the top and bottom functions, students can determine the exact area. This technique is essential for solving problems involving physical areas, economic models, and more complex applications in calculus. Mastering this concept is crucial for success in both AP Calculus AB and BC exams.
Learning Objectives
When learning to find the area between curves for the AP Calculus AB and BC exams, you should focus on understanding how to set up and evaluate definite integrals to calculate the area between two functions. This includes determining the points of intersection, integrating the difference of the functions over the relevant interval, and handling cases where the curves intersect multiple times or require horizontal slicing. Mastering these skills is crucial for solving a variety of problems involving areas bounded by curves in both AP Calculus AB and BC.
Finding the Area Between Curves: AP Calculus AB and BC
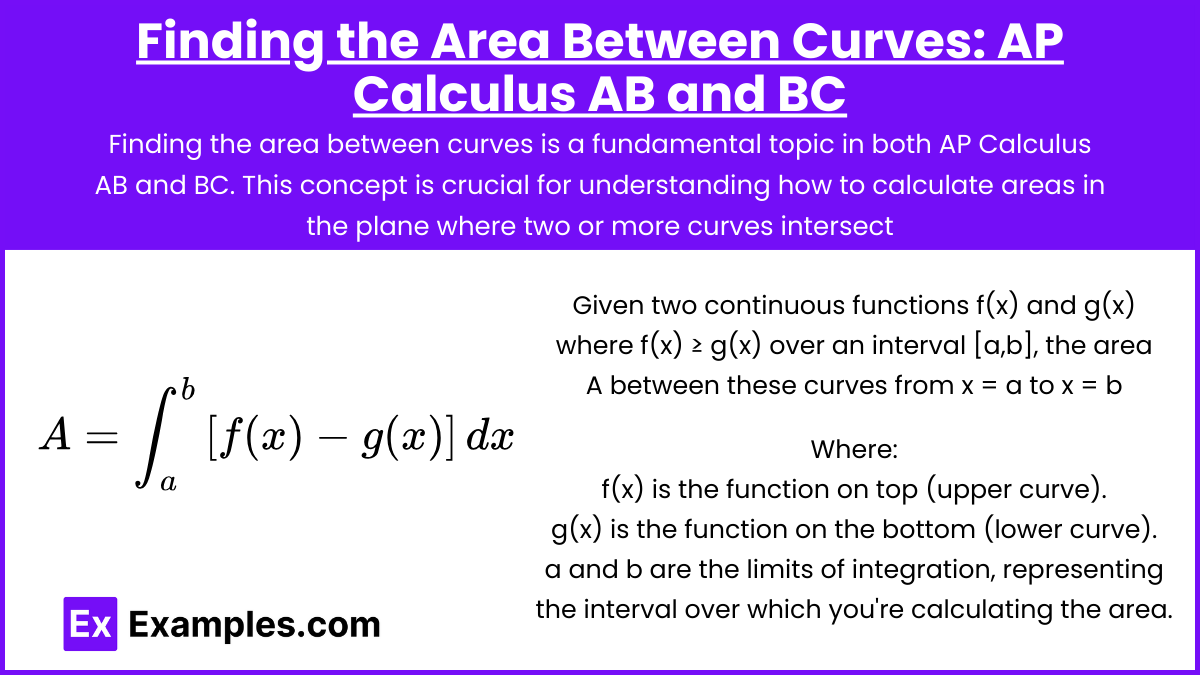
Finding the area between curves is a fundamental topic in both AP Calculus AB and BC. This concept is crucial for understanding how to calculate areas in the plane where two or more curves intersect, forming bounded regions.
Understanding the Concept
When you find the area between curves, you’re essentially computing the area of the region that lies between two functions over a specific interval. This involves integrating the difference between the top function (upper curve) and the bottom function (lower curve) with respect to a variable, typically x or y.
Basic Idea
Given two continuous functions f(x) and g(x) where f(x) ≥ g(x) over an interval [a,b], the area A between these curves from x = a to x = b is given by:
\(A = \int_{a}^{b} \left[ f(x) – g(x) \right] \, dx \)
- f(x) is the function on top (upper curve).
- g(x) is the function on the bottom (lower curve).
- a and b are the limits of integration, representing the interval over which you’re calculating the area.
Step-by-Step Process of Finding the Area Between Curves
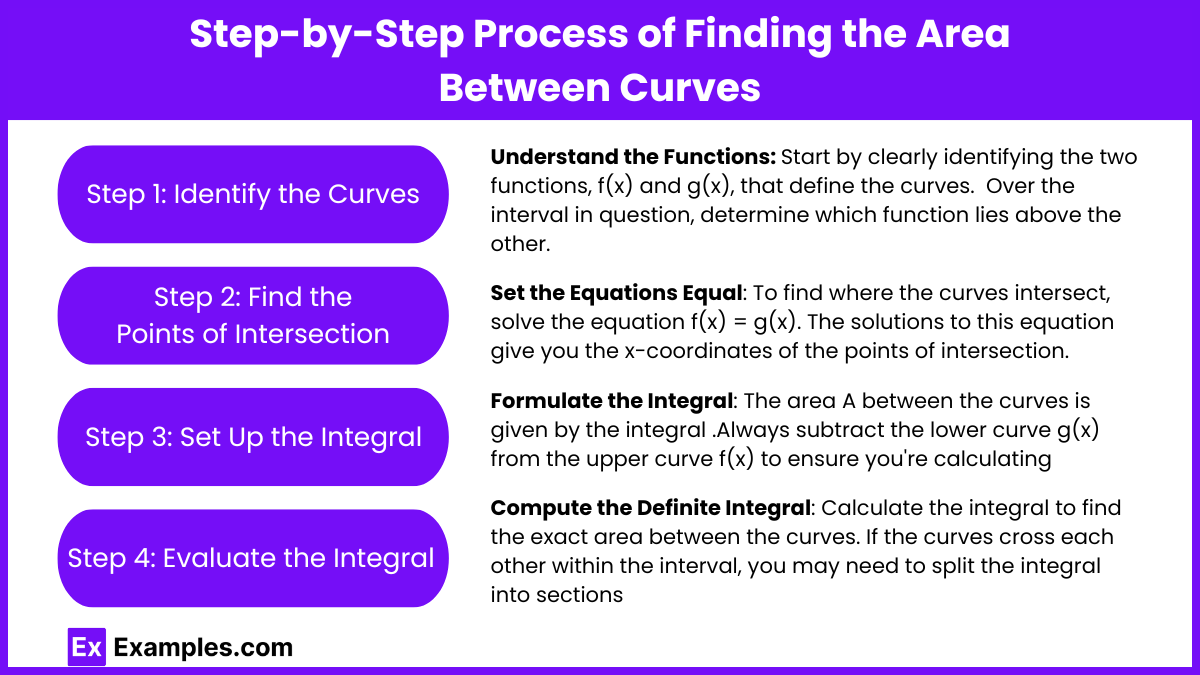
Step 1: Identify the Curves
- Understand the Functions: Start by clearly identifying the two functions, f(x) and g(x), that define the curves. The goal is to find the area of the region bounded by these curves.
- Determine Upper and Lower Curves: Over the interval in question, determine which function lies above the other. Typically, f(x) is considered the upper curve, and g(x) is the lower curve. You can check this by comparing the values of f(x) and g(x) at specific points or by sketching the graphs.
Step 2: Find the Points of Intersection
- Set the Equations Equal: To find where the curves intersect, solve the equation f(x) = g(x). The solutions to this equation give you the x-coordinates of the points of intersection.
- Check the Intersection Points: These intersection points will serve as the limits of integration. Ensure that you correctly identify all relevant points, as missing one could lead to an incorrect calculation of the area.
Step 3: Set Up the Integral
- Formulate the Integral: The area A between the curves is given by the integral: \(A = \int_{a}^{b} \left[ f(x) – g(x) \right] \, dx \)
- Here, a and b are the x-coordinates of the points of intersection found in Step 2.
- Subtract the Lower Curve: Always subtract the lower curve g(x) from the upper curve f(x) to ensure you’re calculating the positive area between them.
Step 4: Evaluate the Integral
- Compute the Definite Integral: Calculate the integral to find the exact area between the curves. This can often be done using basic integration techniques, but in more complex cases, you might need to use substitution or integration by parts.
- Consider Multiple Intervals: If the curves cross each other within the interval, you may need to split the integral into sections where the roles of the upper and lower curves switch. Evaluate each section separately and then sum the results to get the total area.
Tips for Success on the AP Calculus Exam
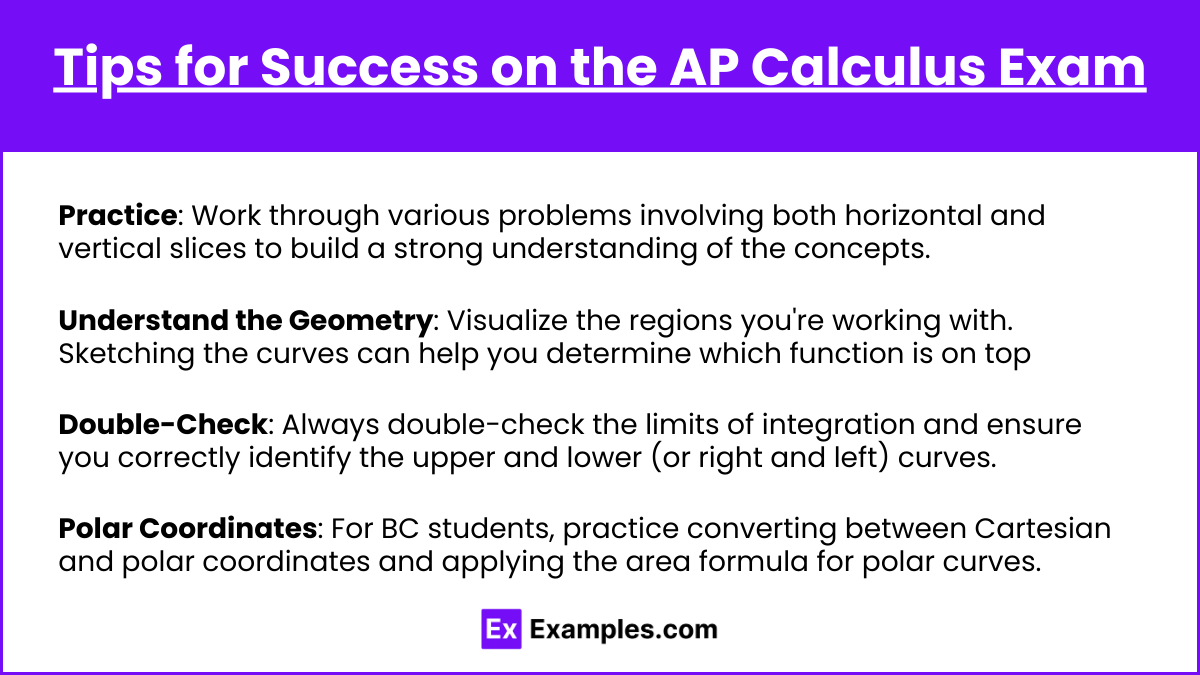
- Practice: Work through various problems involving both horizontal and vertical slices to build a strong understanding of the concepts.
- Understand the Geometry: Visualize the regions you’re working with. Sketching the curves can help you determine which function is on top (or on the right, if using vertical integration).
- Double-Check: Always double-check the limits of integration and ensure you correctly identify the upper and lower (or right and left) curves.
- Polar Coordinates: For BC students, practice converting between Cartesian and polar coordinates and applying the area formula for polar curves.
Examples
Example 1: Area Between a Parabola and a Line
Find the area between the curves f(x) = x2 and g(x) = 4x−x2 over the interval where they intersect. First, solve x2 = 4x−x2 to find the points of intersection. These curves intersect at x = 0 and x = 2. Set up the integral \(\int_{0}^{2} \left[(4x – x^2) – x^2 \right] \, dx, \), and evaluate to find the area.
Example 2: Area Between Sine and Cosine Functions
Calculate the area between the curves f(x) = sin(x) and g(x) = cos(x) from x = 0 to \( x = \frac{\pi}{2} \). These curves intersect at \( x = \frac{\pi}{4} \). To find the area, split the integral into two parts: \(\int_{0}^{\frac{\pi}{4}} \left[\cos(x) – \sin(x)\right] \, dx \) and \(\int_{\frac{\pi}{4}}^{\frac{\pi}{2}} \left[\sin(x) – \cos(x)\right] \, dx. \). Add these results to get the total area.
Example 3: Area Between an Exponential Function and a Line
Determine the area between the curves f(x) = ex and g(x) = x+1 over the interval where they intersect. Solve ex = x+1 numerically to find the intersection points. Let’s assume they intersect at x = 0 and x = 1.31. Set up the integral \(\int_{0}^{1.31} \left[(e^x) – (x + 1)\right] \, dx \) and evaluate it to find the exact area.
Example 4: Area Between a Polynomial and a Quadratic
Find the area between the curves f(x) = x3−3x+2 and g(x) = −x2+2x+3. First, solve the equation x3−3x+2 = −x2+2x+3 to determine the intersection points. After finding these points, set up and evaluate the integral over the appropriate interval to calculate the area.
Example 5: Area Between Hyperbola and Line
Calculate the area between the hyperbola \( f(x) = \frac{1}{x} \) and the line g(x) = 2x−3 over the interval x = 1 to x = 3. Find where these functions intersect by solving \( \frac{1}{x} = 2x – 3 \). After identifying the intersection points, set up the integral \(\int_{1}^{3} \left[(2x – 3) – \frac{1}{x}\right] \, dx, \), and evaluate it to find the area between these curves.
Multiple Choice Questions
Question 1
Let f(x) = 3x+1 and g(x) = x2. What is the area between these curves from x = 0 to x = 1?
a) \( \frac{5}{6} \)
b) 2
c) \( \frac{7}{6} \)
d) 1
Answer: c) \( \frac{7}{6} \)
Explanation:
Set up the integral for the area:
\(A = \int_{0}^{1} \left[ (3x + 1) – x^2 \right] \, dx \)
Simplify and integrate:
\(A = \int_{0}^{1} \left( 3x + 1 – x^2 \right) \, dx = \left[ \frac{3x^2}{2} + x – \frac{x^3}{3} \right]_{0}^{1} \)
Substitute the limits:
\(A = \left( \frac{3(1)^2}{2} + 1 – \frac{(1)^3}{3} \right) – 0 = \frac{3}{2} + 1 – \frac{1}{3} = \frac{9}{6} + \frac{6}{6} – \frac{2}{6} = \frac{7}{6} \)
Question 2
Given the curves y = x and y = 2x, what is the area between these curves from x = 0 to x = 1?
a) \( \frac{1}{2} \)
b) 1
c) \( \frac{3}{2} \)
d) \( \frac{1}{4} \)
Answer: a) \( \frac{1}{2} \)
Explanation:
Set up the integral for the area:
\(A = \int_{0}^{1} \left[ 2x – x \right] \, dx = \int_{0}^{1} x \, dx \)
Integrate:
\(A = \left[ \frac{x^2}{2} \right]_{0}^{1} = \frac{1^2}{2} – \frac{0^2}{2} = \frac{1}{2} \)
Question 3
Which of the following correctly represents the area between the curves y = 4 and y = x2 from x = −1 to x = 1?
a) \( \int_{-1}^{1} \left[ 4 – x^2 \right] \, dx \)
b) \( \int_{-1}^{1} \left[ x^2 – 4 \right] \, dx \)
c) \( \int_{0}^{1} \left[ 4 + x^2 \right] \, dx \)
d) \( \int_{-1}^{1} \left[ 4 \cdot x^2 \right] \, dx \)
Answer: a) \( \int_{-1}^{1} \left[ 4 – x^2 \right] \, dx \)
Explanation:
The area between the curves y=4 and y=x2 is found by subtracting the lower curve (y = x2) from the upper curve (y = 4):
\(A = \int_{-1}^{1} \left[ 4 – x^2 \right] \, dx \)