How Limits Help us to Handle Change at an Instant
In AP Calculus AB and BC, limits are a key concept that helps us understand how functions behave as they approach specific points, enabling us to handle change at an exact instant. By using limits, we can define derivatives, which measure the instantaneous rate of change of a function. This ability to analyze change at a precise moment is crucial for solving complex problems involving motion, growth, and other dynamic processes, making limits an essential tool in both courses.
Learning Objectives
By studying “How Limits Help Us to Handle Change at an Instant” for the AP Calculus exam, you should aim to understand how limits define the instantaneous rate of change, leading to the concept of derivatives. You should learn how to calculate limits, interpret their significance in real-world contexts, and apply them to determine the behavior of functions near specific points. Additionally, you should be able to analyze continuity, evaluate one-sided and two-sided limits, and solve problems involving instantaneous change in various scenarios.
AP Calculus AB: Introduction to Limits and Instantaneous Change
1. Understanding Limits
In AP Calculus AB, students are introduced to the concept of limits as a fundamental tool to understand how functions behave as they approach specific points. A limit answers the question: What value does the function approach as the input gets closer to a particular point?
Why are limits important?
- Behavior Near a Point: Limits help us understand what a function is doing as it gets close to a certain point, even if it doesn’t actually reach that point.
- Handling Undefined Points: They allow us to deal with functions that have points of discontinuity (like a hole) or where direct computation might result in undefined expressions, such as division by zero.
- Approximating Values: In many real-world scenarios, we are interested in how a system behaves as a certain variable approaches a particular value rather than just at the value itself.
2. The Formal Definition of a Limit
The formal definition of a limit in AP Calculus AB is expressed as:
\( \left(\lim_{x \to a} f(x) = L\right) \)
This means that as x approaches a, the function f(x) gets closer and closer to L.
Notation and Interpretation: The expression limx→af(x)=L reads as: “The limit of f(x) as x approaches a is L.” This means that as x gets arbitrarily close to a (from either side), the values of f(x) get arbitrarily close to L.
3. Instantaneous Rate of Change and the Derivative
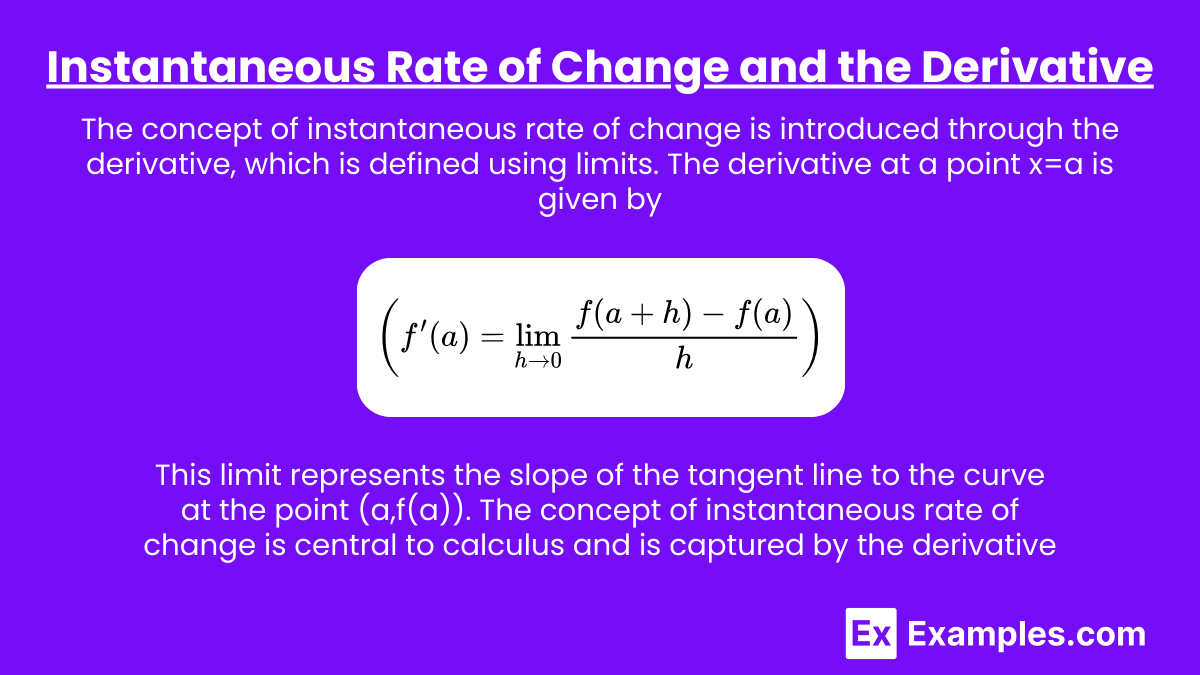
The concept of instantaneous rate of change is introduced through the derivative, which is defined using limits. The derivative at a point x=a is given by:
\( \left(f'(a) = \lim_{h \to 0} \frac{f(a+h) – f(a)}{h}\right) \)
This limit represents the slope of the tangent line to the curve at the point (a,f(a)).
The concept of instantaneous rate of change is central to calculus and is captured by the derivative.
- Average vs. Instantaneous Rate of Change: The average rate of change of a function over an interval gives a broad sense of how the function behaves, similar to average speed. However, the instantaneous rate of change, akin to speed at a precise moment, gives a more specific and localized measure.
4. Example: Derivative of a Polynomial Function
Consider the function f(x) = 3x2. To find the derivative at x=2
\( \left(f'(2) = \lim_{h \to 0} \frac{3(2+h)^2 – 3(2)^2}{h} = \lim_{h \to 0} \frac{12h + 3h^2}{h} = \lim_{h \to 0} (12 + 3h) = 12\right) \)
Thus, the instantaneous rate of change of f(x)=3x2 at x=2 is 12.
5. Continuity and Differentiability
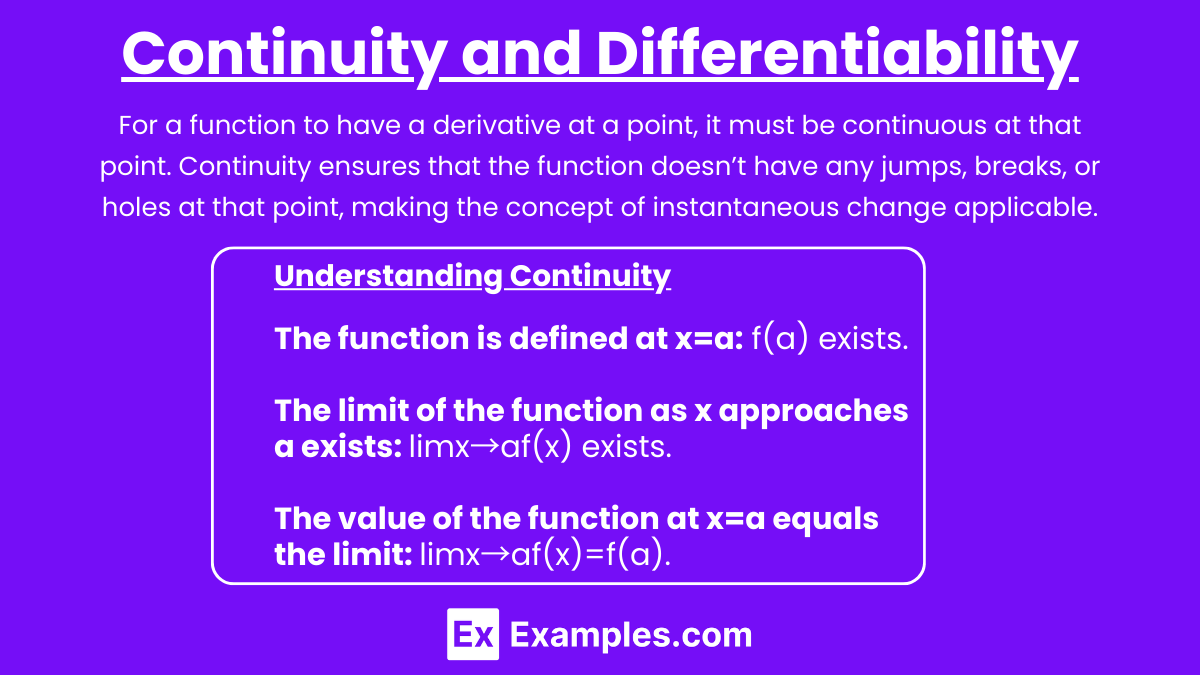
For a function to have a derivative at a point, it must be continuous at that point. Continuity ensures that the function doesn’t have any jumps, breaks, or holes at that point, making the concept of instantaneous change (via the derivative) applicable.
Understanding Continuity
A function is said to be continuous at a point x=a if the following three conditions are met:
- The function is defined at x=a: f(a) exists.
- The limit of the function as x approaches a exists: limx→af(x) exists.
- The value of the function at x=a equals the limit: limx→af(x)=f(a).
AP Calculus BC: Extending Limits and Instantaneous Change
In AP Calculus BC, the concept of limits is extended to more complex scenarios, including infinite limits, limits at infinity, and series.
1. Limits Involving Infinity
In AP Calculus BC, students explore how limits can describe the behavior of functions as x approaches infinity or negative infinity. This is critical for understanding asymptotic behavior.
For example, for the function f(x) =1/ x2
\( \left(\lim_{x \to \infty} \frac{1}{x^2} = 0\right) \)
This indicates that as x becomes very large, f(x) approaches 0, showing that the graph of the function has a horizontal asymptote at y=0.
2. Derivatives of Parametric and Polar Functions
AP Calculus BC extends the concept of derivatives to parametric and polar functions, requiring a deep understanding of limits. For a parametric curve defined by x(t) and y(t), the derivative dy/dx is found using the limits of the derivatives of the parametric equations:
\( \left(\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}}\right) \)
This application of limits helps in understanding the instantaneous rate of change along more complex curves.
3. Series and Convergence
Another advanced topic in AP Calculus BC is the study of infinite series. Limits play a crucial role in determining whether an infinite series converges (approaches a finite value) or diverges (grows without bound). For example, the sum of an infinite geometric series ∑n=0∞arn converges if:
\( \left(\lim_{n \to \infty} r^n = 0 \quad \text{for } |r| < 1\right) \)
This limit is fundamental in determining the sum of the series.
4. L’Hôpital’s Rule
L’Hôpital’s Rule, an important tool in AP Calculus BC, uses limits to evaluate indeterminate forms like 00\frac{0}{0}00 or ∞∞\frac{\infty}{\infty}∞∞. The rule states:
\( \left(\lim_{x \to a} \frac{f(x)}{g(x)} = \lim_{x \to a} \frac{f'(x)}{g'(x)}\right) \)
if the original limit produces an indeterminate form and certain conditions are met.
Examples
Example 1: Instantaneous Velocity
In physics, the velocity of an object at a specific moment is the instantaneous rate of change of its position. For example, if the position of a car is given by s(t)=t3−4t2+5t, the instantaneous velocity at any time t is found by taking the derivative using limits. The derivative s′(t) gives the exact speed of the car at any particular second, which is crucial for understanding motion.
Example 2: Marginal Cost in Economics
In economics, the marginal cost of producing one additional unit of a product is derived from the cost function C(x). If C(x) represents the total cost to produce x units, then the marginal cost at x = a is C′(a), found by applying limits to calculate the derivative. This helps businesses make decisions about production levels by understanding how costs change instantaneously as output changes.
Example 3: Slope of a Tangent Line
The slope of a tangent line to a curve at a point represents the instantaneous rate of change of the function at that point. For example, if you have the curve y = f(x) = x2+3x+2, the slope of the ta ngent at any point x=a is given by f′(a). This slope, calculated using limits, gives the best linear approximation to the curve at that specific point, which is vital for many applications in calculus.
Example 4: Instantaneous Rate of Population Growth
In biology, the rate at which a population is growing at a specific moment can be modeled using limits. If P(t) represents the population at time t, the instantaneous rate of growth is P′(t), found by taking the limit as the time interval approaches zero. This helps biologists understand how fast a population is growing or declining at any given instant, which is essential for managing ecosystems.
Example 5: Electrical Current at an Instant
In electrical engineering, the current I(t) at a specific time t can be described as the derivative of the charge Q(t) with respect to time. By applying limits, engineers can determine the instantaneous current flowing through a circuit at any moment. This is crucial for designing and analyzing circuits where current may change rapidly, such as in alternating current (AC) systems.
Multiple Choice Questions
Question 1
Which of the following best explains how limits are used to understand instantaneous change?
A) Limits allow us to evaluate the exact value of a function at a point.
B) Limits help us find the slope of a curve at a specific point, representing the instantaneous rate of change.
C) Limits determine whether a function is continuous at a certain point.
D) Limits only apply to functions that are linear and have a constant rate of change.
Answer: B) Limits help us find the slope of a curve at a specific point, representing the instantaneous rate of change.
Explanation: The concept of limits is fundamental in calculus for understanding instantaneous change. Specifically, the limit is used to calculate the derivative, which represents the slope of the tangent line to a curve at a given point. This slope corresponds to the instantaneous rate of change of the function at that point. Option B correctly identifies this relationship.
Question 2
How does the limit of a function as the input approaches a specific value relate to real-world problems involving change?
A) It predicts the average change over a specific interval.
B) It determines the future value of a function over time.
C) It provides the exact rate of change at a single moment in time.
D) It shows how the function behaves over a large interval.
Answer: C) It provides the exact rate of change at a single moment in time.
Explanation: In real-world problems, understanding how something changes instantaneously is crucial, whether it’s the speed of a car at a specific moment or the rate at which water flows out of a tap. The limit allows us to pinpoint this exact rate of change by considering what happens to the function’s output as the input gets arbitrarily close to a particular value. This is the essence of finding the derivative and understanding instantaneous change.
Question 3
Why is the concept of a limit essential when calculating the derivative of a function at a point?
A) The limit ensures that the function is differentiable everywhere.
B) The limit allows us to approximate the slope of the tangent by comparing values from a large interval.
C) The limit is used to formally define the exact slope of the tangent line to the curve at the given point.
D) The limit helps to simplify the function before taking the derivative.
Answer: C) The limit is used to formally define the exact slope of the tangent line to the curve at the given point.
Explanation: The derivative of a function at a point is defined as the limit of the difference quotient as the interval between two points approaches zero. This limit gives the slope of the tangent line, representing the instantaneous rate of change at that point. Without the limit, we would not be able to precisely determine this slope, which is crucial for understanding how a function behaves at any given moment.