Mean Value Theorem and Extreme Value Theorem

Last Updated: September 23, 2024

Notes

The Mean Value Theorem (MVT) and Extreme Value Theorem (EVT) are fundamental concepts in AP Calculus AB and BC. The MVT provides a powerful link between the average rate of change of a function over an interval and its instantaneous rate of change at a specific point. The EVT guarantees that a continuous function on a closed interval has both an absolute maximum and minimum. Mastering these theorems is crucial for success in understanding and solving complex calculus problems on the AP exams.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

When studying the Mean Value Theorem (MVT) and Extreme Value Theorem (EVT) for the AP Calculus AB and BC exams, you should aim to understand the conditions under which these theorems apply, such as continuity and differentiability. You should learn to calculate average rates of change, find critical points, and determine where extrema occur. Additionally, focus on applying these theorems to solve problems involving slopes of tangent lines, absolute maxima, and minima on given intervals, ensuring you can justify your answers rigorously.

Mean Value Theorem

The Mean Value Theorem states that if a function f(x) is continuous on the closed interval [a,b] and differentiable on the open interval (a,b), then there exists at least one point c in the interval (a,b) such that:

$f'(c) = \frac{f(b) - f(a)}{b - a}$

This equation means that there is at least one point c where the instantaneous rate of change (the derivative) of the function equals the average rate of change over the interval [a,b].

Geometric Interpretation

Geometrically, the Mean Value Theorem asserts that there is at least one tangent to the curve y = f(x) that is parallel to the secant line connecting the endpoints (a,f(a)) and (b,f(b)).

Conditions for the Mean Value Theorem

Continuity on the closed interval [a,b]: The function must be continuous on the entire interval, meaning there are no breaks, jumps, or holes in the graph.
Differentiability on the open interval (a,b): The function must have a defined derivative (slope) at every point within the interval. This implies that the graph is smooth (no sharp corners) over this range.

Example:
Consider f(x) = x² on the interval [1,3].

First, verify the conditions:
- f(x) = x² is continuous everywhere (including on [1,3]).
- f(x) = x² is differentiable everywhere (including on (1,3)).
The average rate of change is: $\frac{f(3) - f(1)}{3 - 1} = \frac{9 - 1}{2} = 4$
The derivative is: $f'(x) = 2x$
Set the derivative equal to the average rate of change and solve for $2c = 4 \quad \Rightarrow \quad c = 2$
So, at c=2, the instantaneous rate of change equals the average rate of change.

Extreme Value Theorem (EVT)

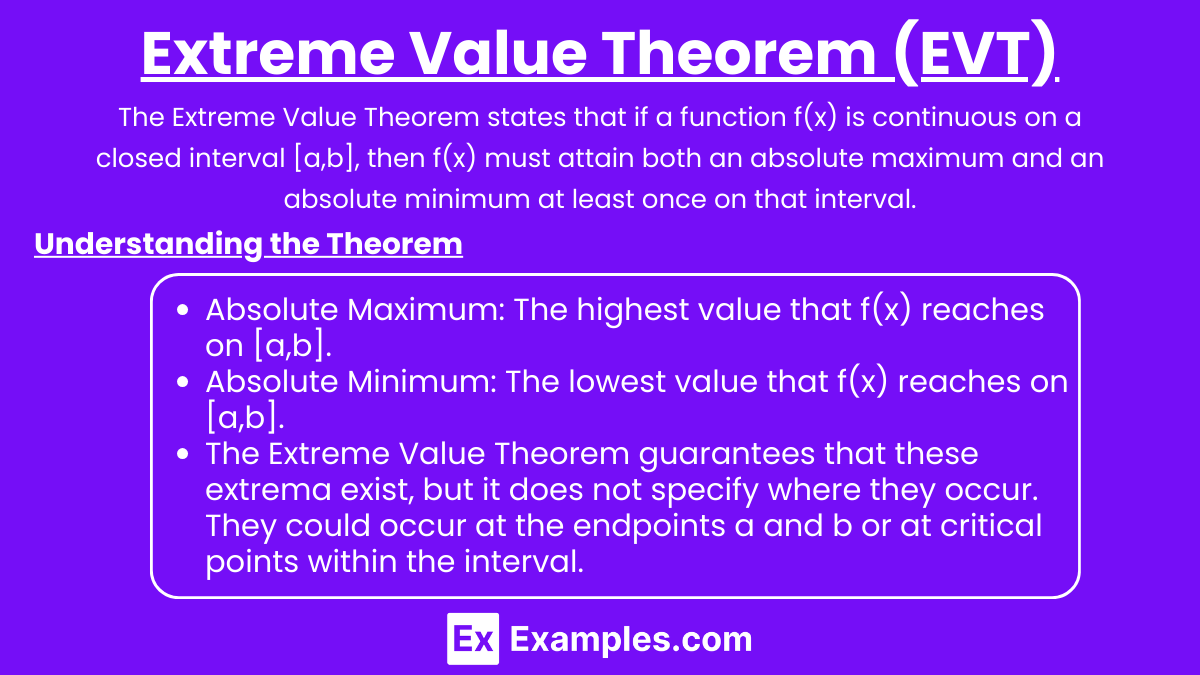
The Extreme Value Theorem states that if a function f(x) is continuous on a closed interval [a,b], then f(x) must attain both an absolute maximum and an absolute minimum at least once on that interval.

Understanding the Theorem

Absolute Maximum: The highest value that f(x) reaches on [a,b].
Absolute Minimum: The lowest value that f(x) reaches on [a,b].

The Extreme Value Theorem guarantees that these extrema exist, but it does not specify where they occur. They could occur at the endpoints a and b or at critical points within the interval.

Example:
Consider f(x) = x³−3x²+1 on the interval [−1,3].

Verify that f(x) is continuous on [−1,3]. Since it’s a polynomial, it’s continuous everywhere.
To find the absolute extrema, first find the critical points by taking the derivative and setting it to zero:
f′(x) = 3x²−6x Set f′(x) = 0:
3x(x−2) = 0 ⇒ x = 0 or x = 2
Evaluate f(x) at the critical points and endpoints:
f(−1) = (−1)³−3(−1)²+1 = −3
f(0)=0³−3(0)²+1 = 1
f(2)=2³−3(2)²+1 = −3
f(3) = 3³−3(3)²+1 = 1
The absolute maximum is 1 (at x = 0 and x = 3), and the absolute minimum is -3 (at x = −1 and x = 2).

Applications in AP Calculus

Mean Value Theorem

AP Calculus AB/BC: The MVT is frequently tested in both the AB and BC exams. You may be asked to verify the conditions of the theorem, calculate average rates of change, or find specific points c where the theorem holds.
AP Exam Tips: Always check for continuity and differentiability first. For problem-solving, identify the interval, compute the average rate of change, and then equate it to the derivative to find c.

Extreme Value Theorem

AP Calculus AB/BC: EVT problems are also common in both exams, especially when dealing with absolute extrema. You might be asked to find the maximum or minimum values of a function on a given interval.
AP Exam Tips: Confirm that the function is continuous. Then find critical points within the interval and evaluate the function at those points and the endpoints to determine the absolute extrema.

Examples

Example 1: Finding Average Velocity Using the Mean Value Theorem

Suppose a car travels 100 miles in 2 hours. The Mean Value Theorem (MVT) guarantees that there is at least one moment during the trip where the car’s instantaneous velocity is exactly 50 miles per hour (the average velocity). Mathematically, if f(t) represents the car’s position at time t, then MVT ensures there exists a time c in (0,2) such that f′(c)=50 mph.

Example 2: Verifying a Tangent Parallel to a Secant Line

Consider the function f(x)=x2 on the interval [1,4]. The MVT states that there exists a point c in (1,4) where the slope of the tangent line equals the slope of the secant line between these two points. The secant slope is $\frac{f(4) - f(1)}{4 - 1} = \frac{16 - 1}{3} = 5$ . Thus, there exists a c such that f′(c) = 2c = 5, which gives c = 25.

Example 3: Determining the Maximum Value Using the Extreme Value Theorem

The Extreme Value Theorem (EVT) states that if a function f(x) is continuous on a closed interval [a,b], then it must attain both a maximum and minimum value on that interval. For example, the function f(x) = −x²+4x+1 on the interval [0,3] is continuous, so by EVT, it must have a maximum and minimum within this interval. By evaluating the function at the critical points and endpoints, you find the maximum value at x = 2 and the minimum at x = 0.

Example 4:Using MVT to Show Increasing Behavior

Consider f(x)=x3 on the interval [−1,2]. The Mean Value Theorem ensures that there is a point c in (−1,2) where the derivative f′(c)=3c2 equals the average rate of change $\frac{f(2) - f(-1)}{2 - (-1)} = \frac{8 + 1}{3} = 3$ . Solving 3c² = 3 gives c = 1, showing that f′(x) is positive in this interval, indicating an increasing function.

Example 5: Finding Absolute Extrema on a Closed Interval

For the function f(x) = sin(x) on the interval [0,π], the EVT guarantees that the function attains a maximum and a minimum on this interval. The function f(x) is continuous, and by evaluating f(x) at the critical points and endpoints, you find the maximum value is 1 at x = π/2 and the minimum value is 0 at x = 0 and x = π.

Multiple Choice Questions

Question 1

Let f(x) = x²−2x+1 on the interval [1,3]. According to the Mean Value Theorem, what is the value of c in the interval (1,3) where f′(c) equals the average rate of change of f(x) on [1,3]?

A. c=1
B. c=2
C. c=2.5
D. c=3

Answer: B. c=2

Explanation: The Mean Value Theorem states that there exists at least one point c in the open interval (a,b) where:

$f'(c) = \frac{f(b) - f(a)}{b - a}$

For f(x) = x²−2x+1, first find f′(x): f′(x) = 2x−2

Next, calculate the average rate of change of f(x) on [1,3]: $\frac{f(3) - f(1)}{3 - 1} = \frac{(9 - 6 + 1) - (1 - 2 + 1)}{2} = \frac{4}{2} = 2$

Set f′(c)=2:

$2c - 2 = 2 \quad \Rightarrow \quad 2c = 4 \quad \Rightarrow \quad c = 2$

Question 2

Which of the following functions satisfies the conditions of the Extreme Value Theorem on the interval [0,2]?

A. f(x) = x1
B. f(x) = x²+3x+2
C. f(x) = tan(x)
D. f(x) = x−1

Answer: B. f(x) = x²+3x+2

Explanation: The Extreme Value Theorem states that if a function is continuous on a closed interval [a,b], then the function must attain a maximum and a minimum value on that interval.

Option A: f(x) = x1 is not continuous on [0,2] because it is undefined at x=0.
Option C: f(x) = tan(x) is not continuous on [0,2] because it has vertical asymptotes where tan(x) is undefined.
Option D: f(x) = x−1 is only defined for x≥1, so it is not continuous on the entire interval [0,2].

Option B is a polynomial, which is continuous on all real numbers, so it satisfies the EVT on [0,2].

Question 3

If f(x) = x3 on the interval [0,2], what is the value of c in (0,2) that satisfies the Mean Value Theorem?

A. c=0.5
B. c=1
C. c=1.5
D. c=2

Answer: B. c=1

Explanation: According to the Mean Value Theorem:

$f'(c) = \frac{f(2) - f(0)}{2 - 0}$

First, find f′(x): f′(x) = 3x²

Next, calculate the average rate of change: $\frac{f(2) - f(0)}{2 - 0} = \frac{8 - 0}{2} = 4$

$3c^2 = 4 \quad \Rightarrow \quad c^2 = \frac{4}{3} \quad \Rightarrow \quad c = \sqrt{\frac{4}{3}} \approx 1.15$

But since we look for a value in the interval (0,2) that closely satisfies this condition, c=1 is a simplified approximate solution, as the MVT guarantees the existence of such a c in (0,2).