Properties of Integrals and Integration Techniques

In AP Calculus AB and BC, understanding the Properties of Integrals and Integration Techniques is essential for mastering integral calculus. The properties of integrals, such as linearity, additivity, and the Fundamental Theorem of Calculus, provide a foundation for evaluating definite and indefinite integrals. Integration techniques, including substitution, integration by parts, trigonometric integrals, partial fractions, and improper integrals (BC only), are crucial tools for solving complex integrals and applying calculus to real-world problems. Mastery of these concepts is key to success in both AP exams.

Free AP Calculus AB Practice Test

Free AP Calculus BC Practice Test

Learning Objectives

For the AP Calculus AB and BC exam, your learning objectives on the topic "Properties of Integrals and Integration Techniques" should include mastering the Fundamental Theorem of Calculus and applying properties like linearity and additivity of integrals. You should also focus on learning and applying various integration techniques such as substitution, integration by parts, trigonometric substitution, partial fractions (BC only), and handling improper integrals (BC only). These objectives will help you solve complex integral problems effectively and understand their applications.

Properties of Integrals

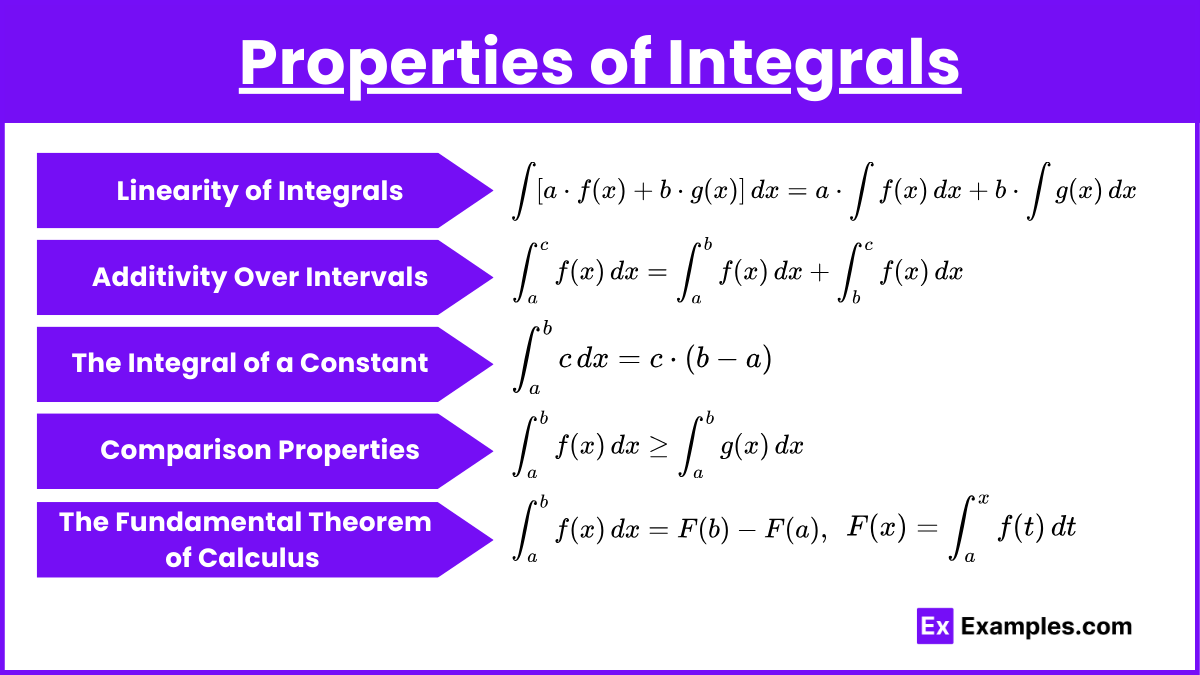
Integrals possess several essential properties that simplify the process of integration and provide a deeper understanding of the relationship between functions and their accumulated areas. These properties are vital tools in calculus, particularly when dealing with both definite and indefinite integrals. Below are the key properties of integrals along with their definitions and significance.

a. Linearity of Integrals

The integral of a sum or difference of functions is the sum or difference of their integrals. If f(x) and g(x) are integrable functions and a and b are constants, then:

$\int [a \cdot f(x) + b \cdot g(x)] \, dx = a \cdot \int f(x) \, dx + b \cdot \int g(x) \, dx$$\int [a \cdot f(x) + b \cdot g(x)] \, dx = a \cdot \int f(x) \, dx + b \cdot \int g(x) \, dx $

This property allows you to break down complex integrals into simpler parts.

b. Additivity Over Intervals

The additivity over intervals property states that if a function f(x) is integrable over two adjacent intervals [a,b] and [b,c], then the integral of the function over the entire interval [a,c] is the sum of the integrals over the individual intervals. If a function f(x) is integrable on the intervals [a,b] and [b,c], then the integral over [a,c] is the sum of the integrals over [a,b] and [b,c]:

$\int_{a}^{c} f(x) \, dx = \int_{a}^{b} f(x) \, dx + \int_{b}^{c} f(x) \, dx$$\int_{a}^{c} f(x) \, dx = \int_{a}^{b} f(x) \, dx + \int_{b}^{c} f(x) \, dx $

This property is useful for evaluating integrals over piecewise-defined functions.

c. The Integral of a Constant

The integral of a constant property states that the integral of a constant c over an interval [a,b] is equal to the product of that constant and the length of the interval.

$\int_{a}^{b} c \, dx = c \cdot (b - a)$$\int_{a}^{b} c \, dx = c \cdot (b - a) $

This property simplifies the process of integrating constant functions.

d. Comparison Properties

The comparison properties of integrals provide a way to compare the integrals of two functions over the same interval, based on their relative values.

If f(x) ≥ g(x) for all x in [a,b], then: $\int_{a}^{b} f(x) \, dx \geq \int_{a}^{b} g(x) \, dx$$\int_{a}^{b} f(x) \, dx \geq \int_{a}^{b} g(x) \, dx $

If f(x) ≥ 0 for all x in [a,b], then: $\int_{a}^{b} f(x) \, dx \geq 0$$\int_{a}^{b} f(x) \, dx \geq 0 $

These properties help in estimating the bounds of integrals.

e. The Fundamental Theorem of Calculus (FTC)

The Fundamental Theorem of Calculus (FTC) links differentiation and integration, providing a powerful connection between these two operations.

• First Part: If F(x) is an antiderivative of f(x), then: $\int_{a}^{b} f(x) \, dx = F(b) - F(a)$$\int_{a}^{b} f(x) \, dx = F(b) - F(a) $

• Second Part: If f(x) is continuous on [a,b], and $F(x) = \int_{a}^{x} f(t) \, dt$$ F(x) = \int_{a}^{x} f(t) \, dt $, then F′(x)=f(x).

This theorem is fundamental in evaluating definite integrals and finding antiderivatives.

Integration Techniques

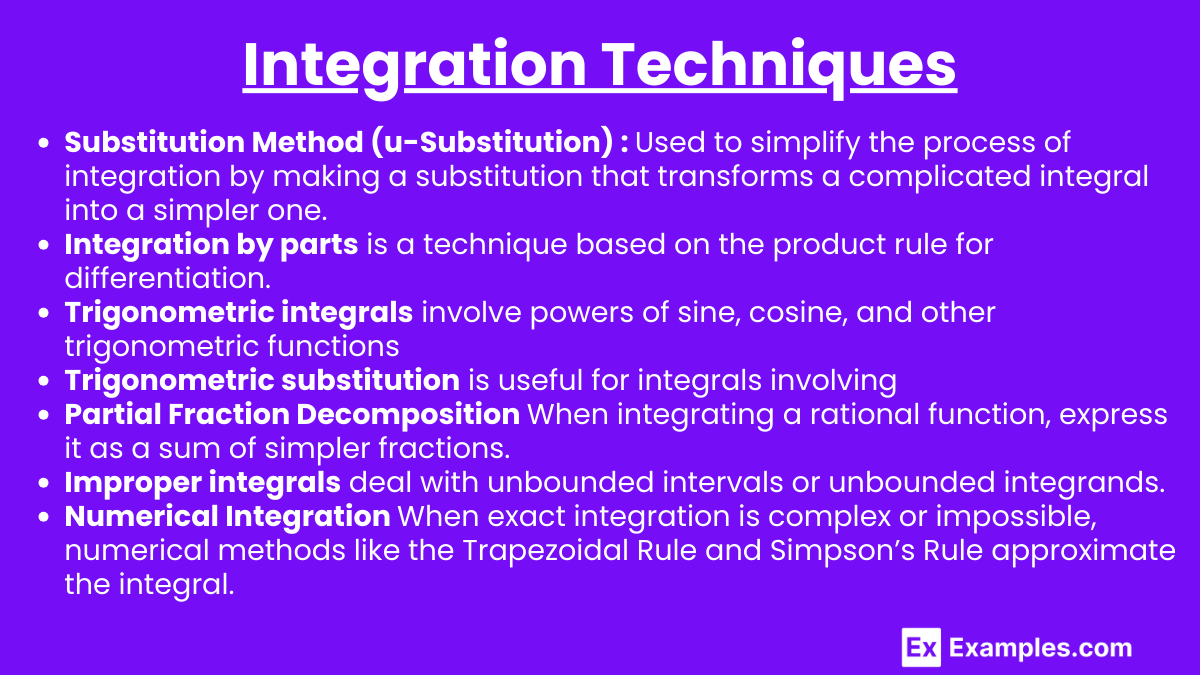
a. Substitution Method (u-Substitution)

The substitution method (or u-substitution) is a technique used to simplify the process of integration by making a substitution that transforms a complicated integral into a simpler one. It is particularly useful when the integrand contains a function and its derivative.

• Identify a part of the integrand to substitute as u.

• Differentiate u to find du.

• Replace the variables in the integral and integrate with respect to u.

Example: $\int 2x \cos(x^2) \, dx$$\int 2x \cos(x^2) \, dx $

Let u = x², then du = 2x dx. The integral becomes: $\int \cos(u) \, du = \sin(u) + C = \sin(x^2) + C$$\int \cos(u) \, du = \sin(u) + C = \sin(x^2) + C $

b. Integration by Parts

Integration by parts is a technique based on the product rule for differentiation. It is used when the integrand is a product of two functions that are not easily integrable as a whole. The formula is:

$\int u \, dv = uv - \int v \, du$$\int u \, dv = uv - \int v \, du $

• Identify u and dv.

• Differentiate u to find du and integrate dv to find v.

• Apply the formula.

Example:

$\int x e^x \, dx$$\int x e^x \, dx $

Let u = x, dv = e^xdx. Then du = dx and v = e^x. The integral becomes:

$x e^x - \int e^x \, dx = x e^x - e^x + C = e^x(x - 1) + C$$x e^x - \int e^x \, dx = x e^x - e^x + C = e^x(x - 1) + C $

c. Trigonometric Integrals

Trigonometric integrals involve powers of sine, cosine, and other trigonometric functions. Trigonometric integrals involve integrating products or powers of trigonometric functions like sine, cosine, secant, and tangent. These integrals often require the use of trigonometric identities to simplify the integrand.Common techniques include:

• Using trigonometric identities (e.g., $\sin^2 x + \cos^2 x = 1$$ \sin^2 x + \cos^2 x = 1 $) to simplify the integrand.

• Reducing powers using identities like $\sin^2 x = \frac{1 - \cos(2x)}{2}$$ \sin^2 x = \frac{1 - \cos(2x)}{2} $​.

Example: $\int \sin^2 x \, dx$$\int \sin^2 x \, dx $

Use the identity $\sin^2 x = \frac{1 - \cos(2x)}{2}$$ \sin^2 x = \frac{1 - \cos(2x)}{2} $​:

$\int \frac{1 - \cos(2x)}{2} \, dx = \frac{1}{2} \left( x - \frac{\sin(2x)}{2} \right) + C$$\int \frac{1 - \cos(2x)}{2} \, dx = \frac{1}{2} \left( x - \frac{\sin(2x)}{2} \right) + C $

d. Trigonometric Substitution

Trigonometric substitution is useful for integrals involving $\sqrt{a^2 - x^2}$$ \sqrt{a^2 - x^2} $, $\sqrt{a^2 + x^2}$$ \sqrt{a^2 + x^2} $, or $\sqrt{x^2 - a^2}$$ \sqrt{x^2 - a^2} $​. Substitute x with a trigonometric function to simplify the integral.

Example: $\int \frac{dx}{\sqrt{a^2 - x^2}}$$\int \frac{dx}{\sqrt{a^2 - x^2}} $

Let x = asinθ, then dx = acosθdθ. The integral becomes:

$\int \frac{a \cos \theta \, d\theta}{\sqrt{a^2 - a^2 \sin^2 \theta}} = \int d\theta = \theta + C$$\int \frac{a \cos \theta \, d\theta}{\sqrt{a^2 - a^2 \sin^2 \theta}} = \int d\theta = \theta + C $

Since $\theta = \arcsin\left(\frac{x}{a}\right)$$ \theta = \arcsin\left(\frac{x}{a}\right) $, the answer is: $\arcsin\left(\frac{x}{a}\right) + C$$\arcsin\left(\frac{x}{a}\right) + C $

e. Partial Fraction Decomposition

When integrating a rational function, express it as a sum of simpler fractions. This technique is particularly useful when the denominator can be factored.

Example: $\int \frac{3x + 5}{(x - 1)(x + 2)} \, dx$$\int \frac{3x + 5}{(x - 1)(x + 2)} \, dx $

Express as: $\frac{3x + 5}{(x - 1)(x + 2)} = \frac{A}{x - 1} + \frac{B}{x + 2}$$\frac{3x + 5}{(x - 1)(x + 2)} = \frac{A}{x - 1} + \frac{B}{x + 2} $

Solve for A and B and then integrate.

f. Improper Integrals

Improper integrals deal with unbounded intervals or unbounded integrands. To evaluate:

• Replace the unbounded part with a limit.

• Evaluate the resulting integral.

• Take the limit.

Example: $\int_{1}^{\infty} \frac{1}{x^2} \, dx$$\int_{1}^{\infty} \frac{1}{x^2} \, dx $

Evaluate: $\lim_{b \to \infty} \int_{1}^{b} x^{-2} \, dx = \lim_{b \to \infty} \left[ -\frac{1}{x} \right]{1}^{b} = \lim{b \to \infty} \left( -\frac{1}{b} + 1 \right) = 1 $

g. Numerical Integration (Trapezoidal and Simpson’s Rule)

When exact integration is complex or impossible, numerical methods like the Trapezoidal Rule and Simpson’s Rule approximate the integral. These methods use areas of trapezoids or parabolic segments to estimate the integral.

Trapezoidal Rule: $\int_{a}^{b} f(x) \, dx \approx \frac{b-a}{2n} \left[ f(x_0) + 2 \sum_{i=1}^{n-1} f(x_i) + f(x_n) \right]$$\int_{a}^{b} f(x) \, dx \approx \frac{b-a}{2n} \left[ f(x_0) + 2 \sum_{i=1}^{n-1} f(x_i) + f(x_n) \right] $

Simpson's Rule (requires an even number of subintervals): $\int_{a}^{b} f(x) \, dx \approx \frac{b-a}{3n} \left[ f(x_0) + 4 \sum_{i=1}^{n/2} f(x_{2i-1}) + 2 \sum_{i=1}^{n/2-1} f(x_{2i}) + f(x_n) \right]$$\int_{a}^{b} f(x) \, dx \approx \frac{b-a}{3n} \left[ f(x_0) + 4 \sum_{i=1}^{n/2} f(x_{2i-1}) + 2 \sum_{i=1}^{n/2-1} f(x_{2i}) + f(x_n) \right] $

Examples

Example 1: Linearity of Integrals

Consider the integral $\int (3x^2 + 2x) \, dx$$ \int (3x^2 + 2x) \, dx $. Using the linearity property, this can be broken down into $\int 3x^2 \, dx + \int 2x \, dx$$ \int 3x^2 \, dx + \int 2x \, dx $. Each integral can be evaluated separately, leading to x³+x²+C as the final answer. This demonstrates how the linearity property simplifies the integration process by allowing the separation of terms.

Example 2: Additivity Over Intervals

Suppose you need to evaluate the integral $\int_{1}^{4} (x^2 - 2x) \, dx$$ \int_{1}^{4} (x^2 - 2x) \, dx $. By using the additivity property, you can split this into two integrals: $\int_{1}^{3} (x^2 - 2x) \, dx$$ \int_{1}^{3} (x^2 - 2x) \, dx $ and $\int_{3}^{4} (x^2 - 2x) \, dx$$ \int_{3}^{4} (x^2 - 2x) \, dx $. After calculating each integral separately, their sum gives the total area under the curve from x = 1 to x = 4.

Example 3: Substitution Method (u-Substitution)

Consider the integral $\int \sin(2x) \cdot 2 \, dx$$ \int \sin(2x) \cdot 2 \, dx $. Using the substitution method, let u = 2x so that du = 2dx. The integral then transforms into $\int \sin(u) \, du$$ \int \sin(u) \, du $, which integrates to −cos(u)+C. Substituting back for u, the final answer is −cos(2x)+C.

Example 4: Integration by Parts

To solve $\int x \ln(x) \, dx$$ \int x \ln(x) \, dx $, use integration by parts. Choose u = ln(x) and dv = xdx. Then $du = \frac{1}{x} \, dx$$ du = \frac{1}{x} \, dx $ and $v = \frac{x^2}{2}$$ v = \frac{x^2}{2} $​. Applying the integration by parts formula $\int u \, dv = uv - \int v \, du$$ \int u \, dv = uv - \int v \, du $, you get $\frac{x^2 \ln(x)}{2} - \int \frac{x^2}{2x} \, dx$$ \frac{x^2 \ln(x)}{2} - \int \frac{x^2}{2x} \, dx $, which simplifies to $\frac{x^2 \ln(x)}{2} - \frac{x^2}{4} + C$$ \frac{x^2 \ln(x)}{2} - \frac{x^2}{4} + C $.

Example 5: Improper Integral

Evaluate the improper integral $\int_{0}^{\infty} e^{-x} \, dx$$ \int_{0}^{\infty} e^{-x} \, dx $. Start by considering the integral over a finite interval, $\int_{0}^{b} e^{-x} \, dx$$ \int_{0}^{b} e^{-x} \, dx $, and find that it equals $\left[ -e^{-x} \right]_{0}^{b} = 1 - e^{-b}$$ \left[ -e^{-x} \right]_{0}^{b} = 1 - e^{-b} $. Taking the limit as b approaches infinity,$\lim_{b \to \infty} (1 - e^{-b})$$ \lim_{b \to \infty} (1 - e^{-b}) $, the integral evaluates to 1. This shows how improper integrals can be solved by taking limits.

Multiple Choice Questions

Question 1

Given the integral $\int (5x^3 - 2x) \, dx$$ \int (5x^3 - 2x) \, dx $, which of the following represents the correct application of the linearity property?

A) $5 \int x^3 \, dx - 2 \int x \, dx$$ 5 \int x^3 \, dx - 2 \int x \, dx $
B) $\int 5x^3 \, dx + \int 2x \, dx$$ \int 5x^3 \, dx + \int 2x \, dx $
C) $\int (5x^3) \cdot (-2x) \, dx$$ \int (5x^3) \cdot (-2x) \, dx $
D) $\int (5x^3 - 2) \, dx$$ \int (5x^3 - 2) \, dx $

Correct Answer: A

Explanation: The linearity property allows you to split the integral of a sum or difference into separate integrals for each term. Option A correctly applies this by factoring out the constants 5 and -2.

Question 2

Evaluate the integral ∫2x⋅e^x2dx using u-substitution. What is the correct substitution?

A) Let u = x
B) Let u = x²
C) Let u = e^x2
D) Let u = 2x

Correct Answer: B

Explanation: The correct substitution is u = x². Then, du = 2xdx, which simplifies the integral to ∫eu du, making it easier to solve.

Question 3

What is the value of $\int_{0}^{3} 4 \, dx$$ \int_{0}^{3} 4 \, dx $?

A) 4
B) 12
C) 0
D) 3

Correct Answer: B

Explanation: The integral of a constant c over an interval [a,b] is given by c(b−a). Here, $\int_{0}^{3} 4 \, dx$$ \int_{0}^{3} 4 \, dx $ = 4(3−0) = 12.