The Squeeze Theorem and the Intermediate Value Theorem are essential tools in both AP Calculus AB and AP Calculus BC for understanding limits and the behavior of continuous functions. The Squeeze Theorem helps determine limits of functions that are difficult to evaluate directly, while the Intermediate Value Theorem is crucial for proving the existence of roots within an interval. Mastering these theorems is vital for success in AP Calculus, as they are frequently used to solve complex problems involving limits and continuity.
Learning Objectives
By studying the Squeeze Theorem and the Intermediate Value Theorem for the AP Calculus AB, BC exam, you should aim to master the ability to reason about limits and continuity. Specifically, learn how to apply the Squeeze Theorem to evaluate challenging limits by bounding functions and the Intermediate Value Theorem to prove the existence of roots within an interval for continuous functions. Understanding these theorems will strengthen your problem-solving skills in calculus, especially in proving limits and finding solutions to equations.
Reasoning Using the Squeeze Theorem and the Intermediate Value TheoremIntroduction
In AP Calculus AB, the Squeeze Theorem and the Intermediate Value Theorem are two essential tools for understanding the behavior of functions. These theorems are often used to prove the existence of limits and roots, making them crucial for solving a wide range of calculus problems. This guide will provide a detailed explanation of these theorems, how they are used, and some examples to help you master their application.
The Squeeze Theorem
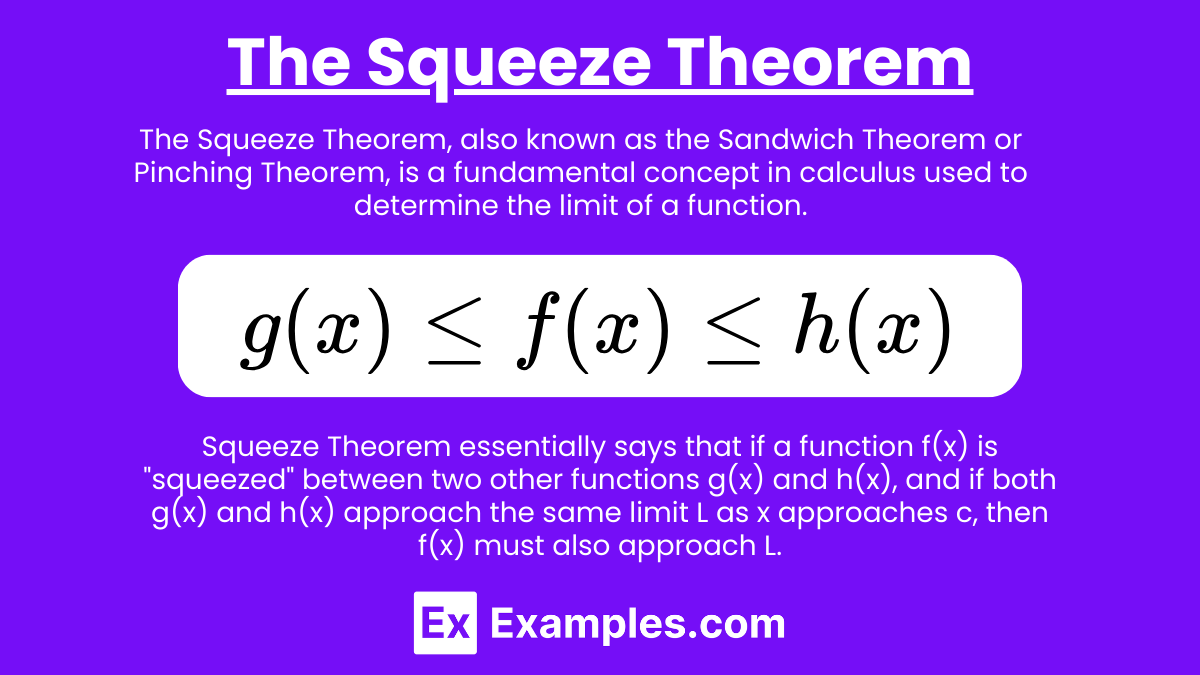
The Squeeze Theorem, also known as the Sandwich Theorem or Pinching Theorem, is a fundamental concept in calculus used to determine the limit of a function. The theorem is applied in situations where direct evaluation of the limit is challenging or impossible. The Squeeze Theorem states that if you have three functions f(x), g(x), and h(x), such that:
g(x)≤f(x)≤h(x)
for all x in an interval around c (except possibly at c itself), and if:
x→climg(x) = x→climh(x) = L
then:
x→climf(x) = L
Explanation:
The Squeeze Theorem essentially says that if a function f(x) is “squeezed” between two other functions g(x) and h(x), and if both g(x) and h(x) approach the same limit L as x approaches c, then f(x) must also approach L.
Why It’s Important:
The Squeeze Theorem is particularly useful for finding the limits of functions that are difficult to evaluate directly. It allows you to use simpler functions that “trap” the more complex function and show that their limits must match.
Example Using the Squeeze Theorem
Evaluate the limit:
x→0limx2sin(x1)
Step-by-Step Solution:
- Identify the bounding functions:
We know that −1≤sin(x1)≤1 for all x, so:−x2≤x2sin(x1)≤x2 - Apply the Squeeze Theorem:
As x approaches 0:x→0lim−x2=0andx→0limx2=0Therefore, by the Squeeze Theorem:x→0limx2sin(x1)=0
The Intermediate Value Theorem
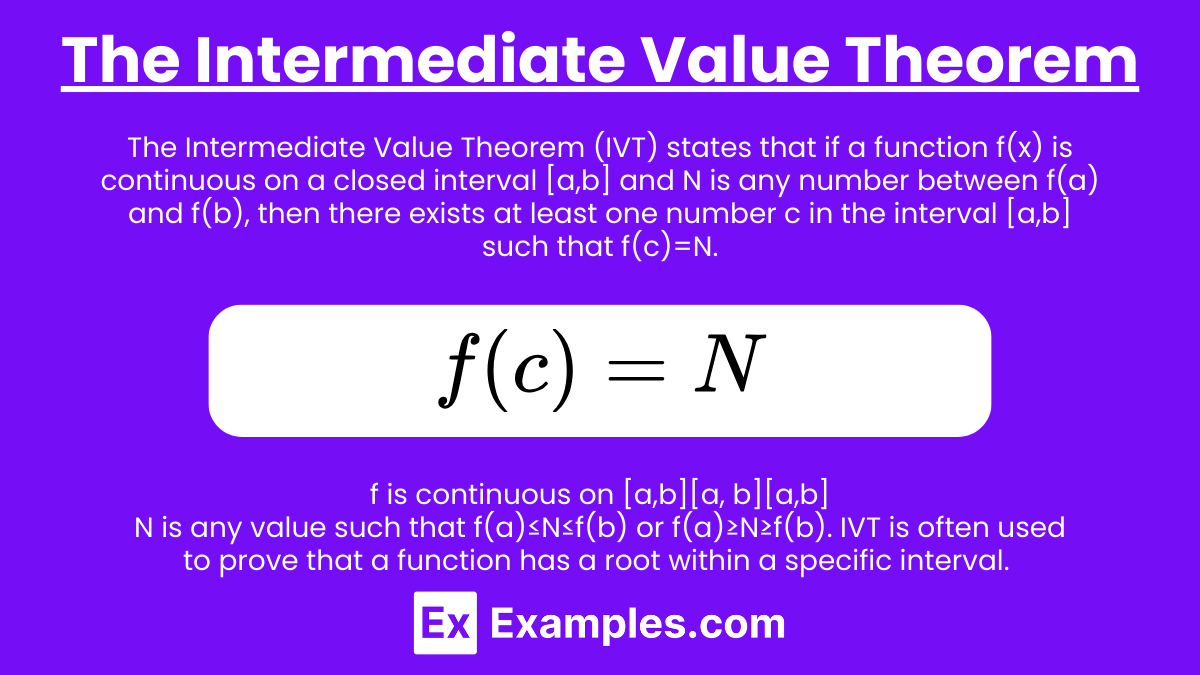
The Intermediate Value Theorem (IVT) states that if a function f(x) is continuous on a closed interval [a,b] and N is any number between f(a) and f(b), then there exists at least one number c in the interval [a,b] such that f(c)=N.
Mathematically:
If f is continuous on [a,b], and N is between f(a) and f(b), then there exists a c in [a,b] such that:
Given:
- f is continuous on [a,b][a, b][a,b]
- N is any value such that f(a)≤N≤f(b) or f(a)≥N≥f(b)
f(c) = N
Explanation:
The Intermediate Value Theorem guarantees that a continuous function will take on every value between f(a) and f(b) at least once. This is a powerful tool for proving the existence of roots within a given interval.
Why It’s Important:
IVT is often used to prove that a function has a root within a specific interval. It’s also used to show that certain equations have solutions.
Example Using the Intermediate Value Theorem
Prove that the equation f(x)=x3−x−2=0 has a root in the interval [1,2].
Step-by-Step Solution:
- Check the function at the endpoints:
Calculate f(1) and f(2):f(1)=13−1−2=−2 f(2)=23−2−2=4 - Apply the Intermediate Value Theorem:
Since f(x) is continuous (as it is a polynomial), and f(1)=−2 and f(2)=4, and 0 is between -2 and 4, by the Intermediate Value Theorem, there must be some c in the interval [1,2] such that f(c)=0. - Conclusion:
Thus, the equation f(x)=x3−x−2=0 has at least one root in the interval [1,2].
Examples
Example 1: Squeeze Theorem: Approximating Trigonometric Limits
Consider the limit limx→0x2sin(x1). Since −1≤sin(x1)≤1, we can squeeze the function as −x2≤x2sin(x1)≤x2. As x approaches 0, both −x2 and x2 approach 0. By the Squeeze Theorem, limx→0x2sin(x1)=0.
Example 2: Squeeze Theorem: Estimating Limits Involving Oscillations
Suppose you need to evaluate limx→0x2cos(x21). The cosine function oscillates between -1 and 1, so we have −x2≤x2cos(x21)≤x2. As x approaches 0, both bounds approach 0. By the Squeeze Theorem, the limit is limx→0x2cos(x21)=0.
Example 3: Intermediate Value Theorem: Finding Roots
Consider the function f(x)=x3−4x+1. To show there is a root between x=1 and x=2, evaluate f(1)=−2 and f(2)=3. Since f(x) is continuous, by the Intermediate Value Theorem, there must be some c in (1,2) where f(c)=0, meaning a root exists in this interval.
Example 4: Intermediate Value Theorem: Proving Existence of Solutions
Given g(x)=ex−3x, show that a solution exists between x=0 and x=1. Calculate g(0)=1 and g(1)=e−3 (which is approximately -0.28). Since g(x) is continuous, by the Intermediate Value Theorem, there must be some c in (0,1) where g(c)=0.
Example 5: Squeeze Theorem: Handling Absolute Values
Evaluate limx→0x2⋅∣sin(x1)∣. Knowing that 0≤∣sin(x1)∣≤1, we can squeeze the function: 0≤x2⋅∣sin(x1)∣≤x2. As x approaches 0, the upper bound x2 approaches 0. By the Squeeze Theorem, limx→0x2⋅∣sin(x1)∣=0.
Multiple Choice Questions
Question 1
Given functions f(x), g(x), and h(x), assume that f(x)≤g(x)≤h(x) for all x in some interval around c (except possibly at c). If limx→cf(x)=limx→ch(x)=L, what can we conclude about limx→cg(x)?
A) limx→cg(x) does not exist.
B) limx→cg(x)=L.
C) limx→cg(x) could be any value.
D) limx→cg(x) equals either L or does not exist.
Answer: B) limx→cg(x)=L
Explanation: The Squeeze Theorem states that if f(x)≤g(x)≤h(x) for all x near c and both limx→cf(x)=limx→ch(x)=L, then limx→cg(x) must also equal L. Since g(x) is “squeezed” between f(x) and h(x), and both f(x) and h(x) approach L as x approaches c, g(x) is forced to approach L as well. Therefore, the correct answer is B.
Question 2
Consider the continuous function f(x) on the closed interval [a,b] where f(a)=−3 and f(b)=5. Which of the following can we guarantee by the Intermediate Value Theorem?
A) There exists a value c in (a,b) such that f(c)=0.
B) There exists a value c in (a,b) such that f(c)=−4.
C) There exists a value c in (a,b) such that f(c)=7.
D) The function f(x) must have a maximum at some point in (a,b).
Answer: A) There exists a value c in (a,b) such that f(c)=0
Explanation: The Intermediate Value Theorem (IVT) states that if f(x) is continuous on the interval [a,b], and f(a) and f(b) have opposite signs, then there must be some point c in the open interval (a,b) where f(c)=0. Since f(a)=−3 and f(b)=5, the function must cross the x-axis somewhere between a and b, implying that f(c)=0 for some c in (a,b). Option A is the correct answer. Options B and C cannot be guaranteed by the IVT because −4 and 7 are outside the range [−3,5]. Option D relates to the Extreme Value Theorem, not the IVT.
Question 3
Let f(x) be a continuous function on [−1,1] with f(−1)=2 and f(1)=4. Suppose g(x) is a function such that 2+sin(x)≤g(x)≤f(x) for all x in [−1,1]. What can be concluded about g(x) on this interval?
A) g(x) must equal 3 at some point in [−1,1].
B) g(x) must be continuous on [−1,1].
C) There exists a c in (−1,1) such that g(c)=3.
D) g(x) must equal 2+sin(x) at some point in [−1,1].
Answer: A) g(x) must equal 3 at some point in [−1,1]
Explanation: This question combines elements of the Squeeze Theorem and the Intermediate Value Theorem. Since g(x) is squeezed between 2+sin(x) and f(x), and f(x) is continuous on [−1,1], by the IVT, g(x) must take on every value between g(−1) and g(1) at least once. Given 2+sin(x) oscillates between 1 and 3, and f(x) takes on values between 2 and 4, g(x) must equal 3 at some point in [−1,1]. This makes option A the correct answer. Option C might seem correct, but the Intermediate Value Theorem only guarantees the value 3 will be taken on somewhere in the interval, not specifically within the open interval (−1,1).