The chain rule for differentiating composite functions

The Chain Rule is a fundamental technique in calculus for differentiating composite functions, where one function is nested within another. It is essential for solving complex problems in AP Calculus AB and BC, allowing you to find the derivative of a function by differentiating the outer function and multiplying it by the derivative of the inner function. Mastery of the Chain Rule is crucial for success on the AP exam, as it applies to a wide range of functions, including trigonometric, exponential, and logarithmic forms.

Learning Objectives

When studying the Chain Rule for Differentiating Composite Functions for the AP Calculus exam, you should aim to master the ability to identify and differentiate composite functions accurately. You should learn to apply the Chain Rule in conjunction with other differentiation rules, such as the Product Rule and Quotient Rule in AP Calculus AB. Additionally, focus on understanding how to differentiate more complex functions, including those involving trigonometric, exponential, and logarithmic functions, and how to extend this knowledge to parametric equations and implicit differentiation, especially for AP Calculus BC.

What is the Chain Rule?

The Chain Rule provides a method for differentiating composite functions. If you have two functions, say f(x) and g(x), and you form a new function by composing them, h(x) = f(g(x)), the Chain Rule allows you to find the derivative of h(x) with respect to x.

Chain Rule Formula

If h(x) = f(g(x)), then the derivative of h(x) with respect to x is given by:

h′(x) = f′(g(x))⋅g′(x)

This means you:

• Differentiate the outer function f with respect to its argument g(x).
• Multiply this result by the derivative of the inner function g(x).

Intuition Behind the Chain Rule

Imagine a process where one action depends on another. For example, suppose you are driving a car, and your speed depends on how hard you press the accelerator. However, the distance you travel depends on your speed. To find how your distance traveled depends on how hard you press the accelerator, you multiply the rate of change of distance with respect to speed by the rate of change of speed with respect to the accelerator. This is analogous to the Chain Rule in calculus.

Step-by-Step Application of the Chain Rule

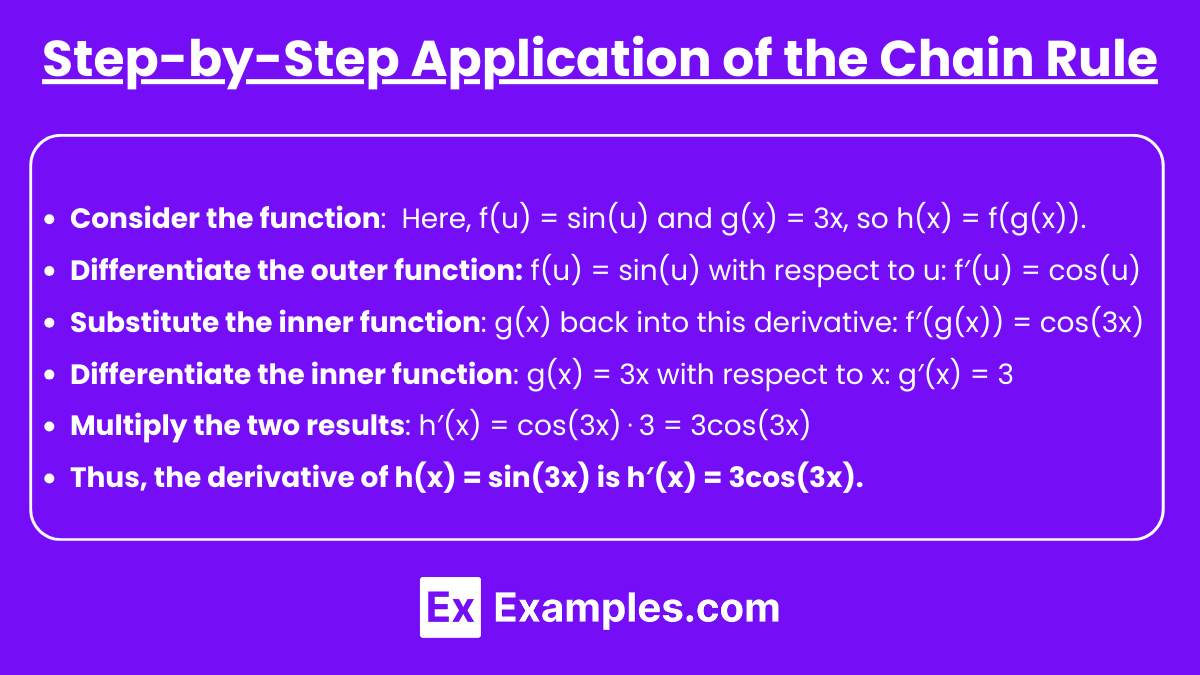
Let’s break down how to apply the Chain Rule in a step-by-step manner:

Example 1: Simple Composite Function

Consider the function:

h(x)=sin(3x)

Here, f(u) = sin(u) and g(x) = 3x, so h(x) = f(g(x)).

• Differentiate the outer function f(u) = sin(u) with respect to u: f′(u) = cos(u)
• Substitute the inner function g(x) back into this derivative: f′(g(x)) = cos(3x)
• Differentiate the inner function g(x) = 3x with respect to x: g′(x) = 3
• Multiply the two results: h′(x) = cos(3x)⋅3 = 3cos(3x)

Thus, the derivative of h(x) = sin(3x) is h′(x) = 3cos(3x).

The Chain Rule in the Context of AP Calculus AB and BC

In AP Calculus AB, the Chain Rule is a foundational tool for differentiating composite functions that involve basic trigonometric, exponential, logarithmic, and polynomial functions. You’ll be required to apply the Chain Rule to functions where one function is nested inside another, such as sin(2x) or e3x2. Often, these problems will require the Chain Rule to be used in combination with other differentiation techniques like the Product Rule (used when multiplying functions) or the Quotient Rule (used when dividing functions). For instance, differentiating a function like x2sin(3x) would involve both the Product Rule and the Chain Rule. Mastery of these techniques is essential for solving problems on the AP Calculus AB exam.

AP Calculus BC

In AP Calculus BC, the Chain Rule is applied in more advanced contexts, reflecting the broader scope of the curriculum. Here are some specific areas where the Chain Rule is essential:

• Parametric Equations: These equations express functions in terms of a third variable (often t). The Chain Rule is crucial when differentiating with respect to one variable while the function is defined in terms of another. For example, given x(t)=t2 and y(t)=sin(t), you need to find dxdy​ using the Chain Rule.
• Implicit Differentiation: This technique is used when functions are not explicitly solved for one variable in terms of another, such as in the equation x2+y2=1. The Chain Rule is applied when differentiating each term with respect to x to account for the presence of y(x).
• Differentiation of Inverse Functions: The Chain Rule plays a crucial role in differentiating inverse trigonometric functions and other inverses. For instance, if y=sin−1(x), then applying the Chain Rule helps find dy/dx​.
• Series Expansions: In the context of Taylor and Maclaurin series, the Chain Rule is often used to differentiate terms within the series. For example, when expanding esin(x) into a series, each term’s differentiation might involve the Chain Rule.

Examples

Example 1

Consider f(x)=(2x+3)2. Let u=2x+3, so f(x)=u2.
The derivative is f′(x)=2u⋅dxdu​=2(2x+3)⋅2=4(2x+3).

Example 2

For g(x)=cos(3x), let u=3x, so g(x)=cos(u).
The derivative is g′(x)=−sin(u)⋅dxdu​=−sin(3x)⋅3=−3sin(3x).

Example 3

If g(x)=sin(5x), let u=5x, so g(x)=sin(u).
The derivative is g′(x)=cos(u)⋅dxdu​=cos(5x)⋅5=5cos(5x).

Example 4

For h(x)=e2x2, let u=2×2, so h(x)=eu.
The derivative is h′(x)=eu⋅dxdu​=e2x2⋅4x=4x⋅e2x2.

Example 5

Consider f(x)=(3x+2)4. To differentiate this, let u=3x+2, so f(x)=u4.
The derivative is f′(x)=4u3⋅dxdu​=4(3x+2)3⋅3=12(3x+2)3.

Multiple Choice Questions

Question 1

Given f(x) = sin(3x²+2), what is the derivative f′(x)?

A) cos(3x²+2)
B) 6xcos(3x²+2)
C) 3cos(3x²+2)
D) 6xsin(3x²+2)

Answer: B) 6xcos(3x²+2)

Explanation: To find the derivative, first recognize that the outer function is sin(u) and the inner function is 3x²+2. The derivative of sin(u) is cos(u), and the derivative of 3x²+2 is 6x. Multiply these together to get 6xcos(3x²+2).

Question 2

If y=(2x+3)5, what is the derivative dy/dx​?

A) 5(2x+3)⁴
B) 10(2x+3)⁴
C) 10x(2x+3)⁵
D) 10(2x+3)⁴

Answer: B) 10(2x+3)⁴

Explanation: Here, the outer function is u5 and the inner function is 2x+3. The derivative of u⁵ is 5u⁴, and the derivative of 2x+3 is 2. Multiply these together to get 10(2x+3)⁴.

Question 3

Given h(x)=ln(4×2+1), what is the derivative h′(x)?

A) 1 / 4×2+1​
B) 8x / 4x²+1​
C) 8x​ / (4x²+1)²
D) 4x​ / 4x²+1

Answer: B) 8x / 4×2+1​

Explanation:
The outer function is ln(u) and the inner function is 4x²+1. The derivative of ln(u) is u1​, and the derivative of 4x²+1 is 8x. Multiply these together to get 8x / 4x²+1​.