Calculating the Equilibrium Constant

Learning Objectives

For the AP Chemistry exam, when learning about calculating the equilibrium constant, focus on understanding the concept of dynamic equilibrium and the law of mass action. Be able to write equilibrium constant expressions for chemical equations and calculate K using equilibrium concentrations of reactants and products. Learn how to interpret the magnitude of K in terms of the relative amounts of reactants and products at equilibrium. Additionally, grasp the impact of changes in temperature on the value of K and the distinction between K꜀ (using concentrations) and Kₚ​ (using partial pressures). This understanding will help you predict the direction of the reaction and the extent to which it proceeds under various conditions.

Introduction

Calculating the equilibrium constant (K) quantifies the balance between reactants and products in a chemical reaction at equilibrium. Derived from the law of mass action, it is the ratio of the concentrations of products to reactants, each raised to their stoichiometric coefficients. This constant helps predict the extent of a reaction and is essential for understanding and controlling chemical processes.

What is the Equilibrium Constant?

The equilibrium constant (K) is a numerical value that defines the ratio of the concentrations of products to reactants at chemical equilibrium, with each concentration raised to the power of its coefficient in the balanced chemical equation. It quantifies the position of equilibrium and indicates the extent to which reactants are converted into products under given conditions. This constant is crucial in determining the direction and extent of chemical reactions at equilibrium.

Steps to Calculating the Equilibrium Constant

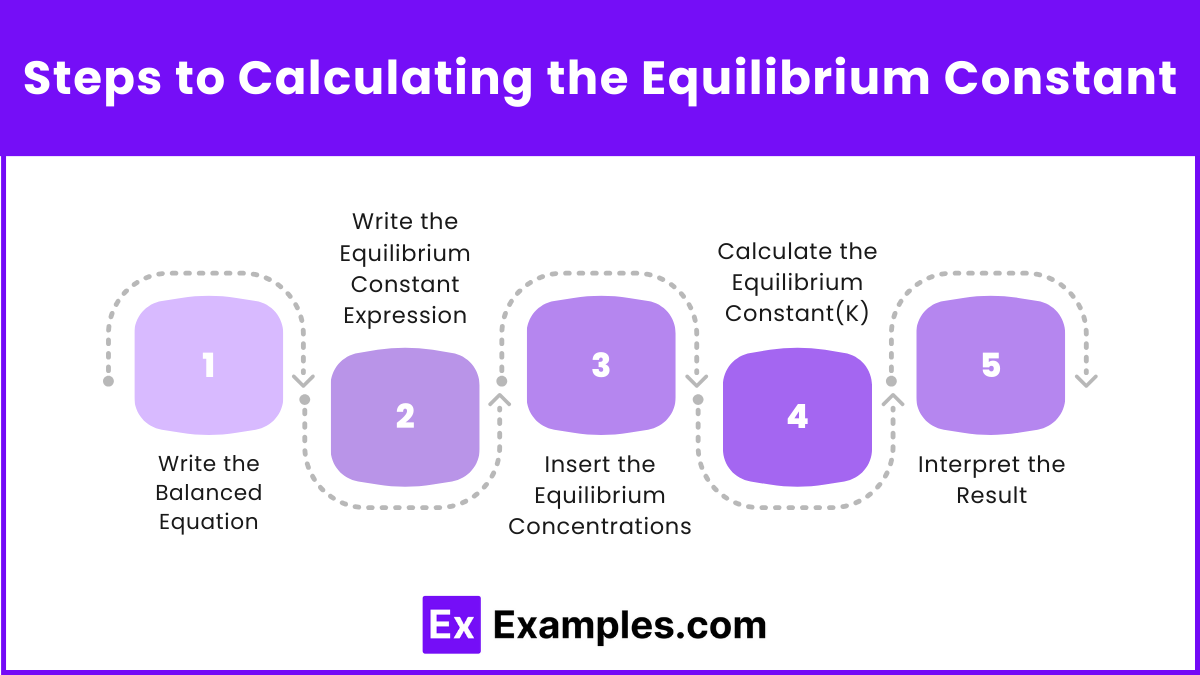
Step 1: Write the Balanced Equation

First, ensure the chemical equation is correctly balanced. The stoichiometric coefficients from this balanced equation are critical as they dictate the exponents for the concentrations of reactants and products in the equilibrium constant expression.

Step 2: Write the Equilibrium Constant Expression

Based on the law of mass action, the equilibrium constant expression for any chemical reaction can be formulated. It involves setting up a ratio where the numerator is the product of the concentrations of the products, each raised to the power of its respective stoichiometric coefficient, and the denominator is the product of the concentrations of the reactants, similarly raised to their coefficients. This expression quantifies the relationship between the concentrations of reactants and products at equilibrium.

For a general reaction:

aA + bB ⇌ cC + dDa

The equilibrium constant expression would be:

K =

​where [A], [B], [C] and [D] are the molar concentrations of the reactants and products at equilibrium.

Step 3: Insert the Equilibrium Concentrations

This step involves substituting the molar concentrations of all reactants and products into the equilibrium constant expression. These concentrations are those that are present at equilibrium. It’s important to note that these values need to be obtained from experimental data or provided within a problem scenario.

Step 4: Calculate the Equilibrium Constant(K)

After inserting the equilibrium concentrations into the equilibrium expression, calculate K by performing the necessary multiplications and divisions as dictated by the expression. This calculation yields a numerical value for K, which represents the ratio of the product of product concentrations to the product of reactant concentrations, each raised to their respective powers as per the balanced equation.

Step 5: Interpret the Result

The numerical value of K provides insight into the chemical behavior at equilibrium:

• A high K (much greater than 1) implies that the equilibrium mixture is product-heavy, indicating that the forward reaction is favored.
• A low K (much less than 1) implies that the equilibrium mixture contains mostly reactants, indicating that the reverse reaction is favored.
• A K close to 1 indicates that neither reactants nor products are favored, and significant amounts of both are present at equilibrium.

Practice Problems

Problem 1: Consider the following reaction at equilibrium: CO(g) + Cl₂(g) ⇌ COCl₂(g)

At a certain temperature, the equilibrium concentrations are found to be:

• [CO] = 0.030 M
• [Cl₂] = 0.045 M
• [COCl₂] = 0.015 M

Calculate the equilibrium constant K for this reaction.

Given reaction:

CO(g) + Cl₂(g) ⇌ COCl₂(g)

The equilibrium constant expression is:

K =

Substituting the given equilibrium concentrations:

• [CO]=0.030 M
• [Cl₂]=0.045 M
• [COCl₂]=0.015 M

K =

So, the equilibrium constant K for this reaction is approximately 11.11.

Problem 2: For the decomposition of calcium carbonate:

CaCO₃(s) ⇌ CaO(s) + CO₂(g)

At equilibrium, the pressure of CO₂​ is 0.90 atm. Calculate the equilibrium constant Kₚ for this reaction.

Given decomposition reaction:

CaCO₃(s) ⇌ CaO(s) + CO₂(g)

For this reaction, only CO₂(g) contributes to Kₚ​ since the other products and reactants are solids and do not appear in the equilibrium expression: Kₚ = [P꜀ₒ₂]

Given the equilibrium pressure of CO₂​:

• P꜀ₒ₂=0.90 atm

So, Kₚ = 0.90

The equilibrium constant Kₚ​ for this reaction is 0.90 atm.