Elemental composition of pure substances

Last Updated: October 10, 2024

Notes

Learning Objectives

Understand how to determine the molar mass of compounds and calculate the mass percent composition of each element. Learn to derive and molecular formulas from percent composition data and relate these formulas to real-world compounds. Master stoichiometric calculations and their applications in predicting chemical reaction outcomes. Develop proficiency in analytical techniques for identifying substances based on their elemental composition. Gain the ability to simplify complex ratios to find the simplest whole-number ratios of elements in compounds. Practice converting mass data to moles and using this information to solve various chemistry problems effectively.

Free AP Chemistry Practice Test

Introduction for Elemental Composition of Pure Substances

The elemental composition of pure substances involves understanding the percentage by mass of each element within a compound. By calculating the percent composition, you can determine the empirical formula, which shows the simplest whole-number ratio of elements, and the molecular formula, which reveals the actual number of atoms in a molecule. This knowledge helps predict properties, reactions, and behaviors of substances, making it crucial for mastering chemistry concepts. Understanding these calculations and relationships allows you to analyze compounds accurately and solve related problems effectively.

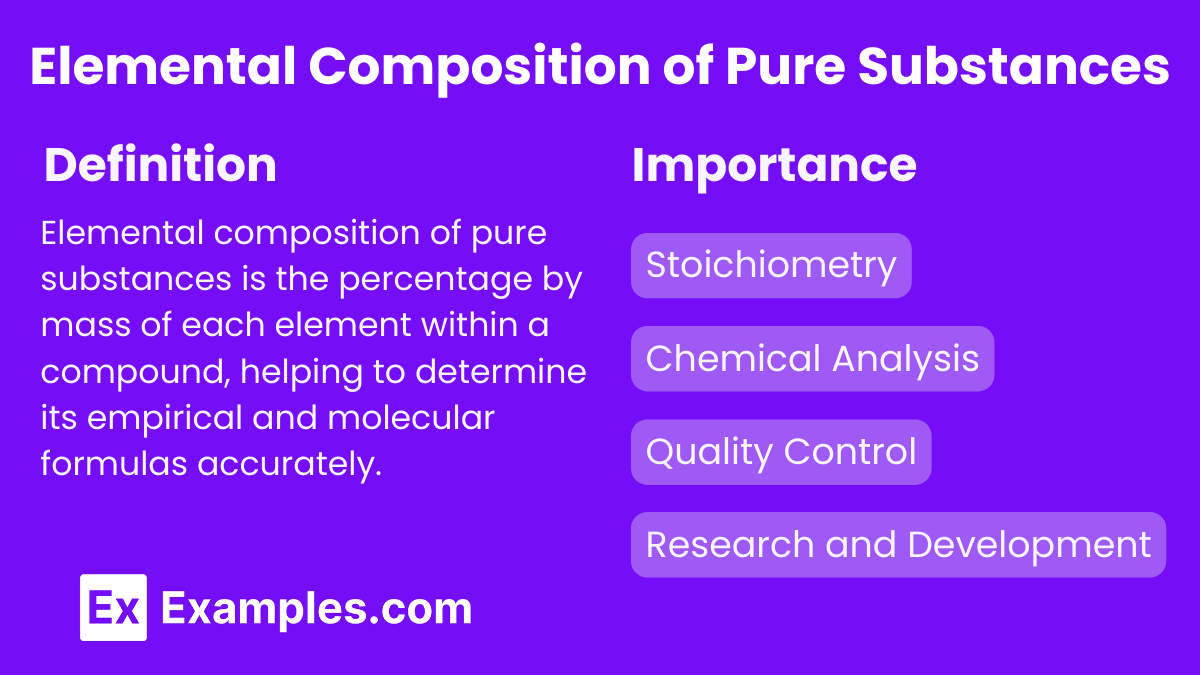
What is Elemental Composition of Pure Substances?

Elemental composition of pure substances is the percentage by mass of each element within a compound, helping to determine its empirical and molecular formulas accurately.

Empirical Formula

The empirical formula of a compound represents the simplest whole-number ratio of the elements present in the compound. It does not necessarily reflect the actual number of atoms in a molecule but gives the lowest ratio of the elements.

Steps to Determine Empirical Formula:

Convert Percent to Mass: Assume 100 g of the compound, so the percentage becomes the mass in grams.
Convert Mass to Moles: Moles of Element = $\frac{\text{Mass of Element (g)}}{\text{Atomic Mass of Element (g/mol)}}$
Find the Simplest Ratio: Ratio = $\frac{\text{Moles of Each Element}}{\text{Smallest Number of Moles}}$
Write the Empirical Formula using the simplest whole-number ratio.

Molecular Formula

The molecular formula shows the actual number of atoms of each element in a molecule. It is a whole-number multiple of the empirical formula.

Steps to Determine Molecular Formula:

Find the Empirical Formula Mass: $\text{Empirical Formula Mass} = \sum (\text{Atomic Mass of Each Element} \times \text{Number of Atoms of Each Element in Empirical Formula})$
Determine the Multiple (n): n = $\frac{\text{Molar Mass of Compound}}{\text{Empirical Formula Mass}}d$
Calculate the Molecular Formula: Molecular Formula = (Empirical Formula) × n

Percent Composition by Mass

Percent composition by mass is the percentage by mass of each element in a compound.

Formula to Calculate Percent Composition

$\text{Percent Composition} = \left( \frac{\text{Mass of Element}}{\text{Total Mass of Compound}} \right) \times 100$

Steps:

Find the molar mass of the compound.
Divide the mass of each element by the total molar mass.
Multiply by 100 to get the percentage.

Determining Empirical Formula from Percent Composition

Convert Percentages to Masses: Assume a 100 g sample of the compound, so the percentage of each element becomes the mass in grams.
Convert Masses to Moles: Use the atomic masses of the elements to convert the masses to moles.
Find the Simplest Ratio: Divide the number of moles of each element by the smallest number of moles calculated.
Write the Empirical Formula: Use the simplest whole-number ratio of the moles of each element to write the empirical formula.

Example: A compound is 40.0% carbon, 6.7% hydrogen, and 53.3% oxygen by mass.

Assume 100 g of the compound:
- Carbon: 40.0 g
- Hydrogen: 6.7 g
- Oxygen: 53.3 g
Convert to moles:
- Carbon: $\frac{40.0 \text{ g}}{12.01 \text{ g/mol}} = 3.33 \text{ moles}$
- Hydrogen: $\frac{6.7 \text{ g}}{1.01 \text{ g/mol}} = 6.63 \text{ moles}$
- Oxygen: $\frac{53.3 \text{ g}}{16.00 \text{ g/mol}} = 3.33 \text{ moles}$
Divide by the smallest number of moles (3.33):
- Carbon: $\frac{3.33}{3.33}$ = 13.333.33=1
- Hydrogen: $\frac{6.63}{3.33}$ = 23.336.63=2
- Oxygen: $\frac{3.33}{3.33}$ = 13.333.33=1
Empirical formula: CH₂O

Determining Molecular Formula from Empirical Formula

Steps:

Find the Empirical Formula: Follow the steps outlined above.
Calculate the Empirical Formula Mass: Sum the atomic masses of all atoms in the empirical formula.
Divide the Molar Mass by the Empirical Formula Mass: This gives the multiple nnn.
Multiply the Empirical Formula by nnn: Obtain the molecular formula.

Example: Given empirical formula CH₂O and a molar mass of 180 g/mol:

Empirical formula mass:
- Carbon: 12.01 g/mol
- Hydrogen: 2 × 1.01 g/mol = 2.02 g/mol
- Oxygen: 16.00 g/mol
- Total: 30.03 g/mol
Divide molar mass by empirical formula mass:
- $\frac{180 \text{ g/mol}}{30.03 \text{ g/mol}}$ = 6
Multiply empirical formula by 6:
- Molecular formula: C₆H₁₂O₆

Steps to Calculate Elemental Composition

Determine the Molecular Formula: Identify the molecular formula of the compound, which indicates the number and types of atoms present.
Find the Atomic Masses: Obtain the atomic masses of each element from the periodic table.
Calculate the Molar Mass: Add the atomic masses of all the atoms in the molecular formula to get the molar mass of the compound.
Calculate the Mass Contribution of Each Element:
- Multiply the number of atoms of each element by its atomic mass.
- This gives the total mass of each element in one mole of the compound.
Find the Percent Composition:
- Divide the mass contribution of each element by the molar mass of the compound.
- Multiply by 100 to convert it to a percentage.

Example: Calculation for Water (H₂O)

Molecular Formula: H₂O
Atomic Masses:
- Hydrogen: 1.01 g/mol
- O xygen: 16.00 g/mol
Molar Mass Calculation:
- Hydrogen: 2×1.01 g/mol=2.02 g/mol,
- Oxygen: 1×16.00 g/mol=16.00 g/mol
- Total Molar Mass: 2.02+16.00=18.02 g/mol
Mass Contribution:
- Hydrogen: 2.02 g
- Oxygen: 16.00 g
Percent Composition:
- Hydrogen: $\left(\frac{2.02}{18.02}\right) \times 100 = 11.21%$
- Oxygen: $\left(\frac{16.00}{18.02}\right) \times 100 = 88.79%$

Importance of Elemental Composition of Pure Substances

Stoichiometry: It is fundamental for stoichiometric calculations, which are essential for predicting the outcomes and yields of chemical reactions.
Chemical Analysis: Determining the elemental composition is vital in analytical chemistry for identifying and quantifying substances.
Formulating Compounds: It helps in deriving empirical and molecular formulas from experimental data, which is necessary for understanding the structure and properties of compounds.
Quality Control: In industries such as pharmaceuticals, materials science, and food chemistry, knowing the precise elemental composition ensures product quality and safety.
Environmental Monitoring: Analyzing the elemental composition of environmental samples helps in assessing pollution levels and tracking the sources of contaminants.
Research and Development: In scientific research, accurate knowledge of elemental composition aids in the synthesis of new materials and compounds with desired properties.
Educational Foundation: It provides a fundamental basis for advanced studies in chemistry and related fields, building a strong conceptual understanding necessary for higher-level coursework and research.

Practice Problems

Q) A compound contains 26.7% phosphorus (P) and 73.3% chlorine (Cl) by mass. Determine the empirical formula?

Solution:

Convert percentages to masses (assume 100 g of the compound):
- Phosphorus: 26.7 g
- Chlorine: 73.3 g
Convert masses to moles:
- Phosphorus: $\frac{26.7 \text{ g}}{30.97 \text{ g/mol}} = 0.862 \text{ moles}$
- Chlorine: $\frac{73.3 \text{ g}}{35.45 \text{ g/mol}} = 2.07 \text{ moles}$
Find the simplest ratio:
- Phosphorus: $\frac{0.862}{0.862}$ = 1
- Chlorine: $\frac{2.07}{0.862} = 2.4 \approx 2$
Empirical formula: PCl₂

Q) A compound with an empirical formula of C₂H₄O has a molar mass of 88 g/mol. Determine the molecular formula?

Solution:

Calculate the empirical formula mass:
- Carbon: 2 × 12.01 g/mol = 24.02 g/mol
- Hydrogen: 4 × 1.01 g/mol = 4.04 g/mol
- Oxygen: 1 × 16.00 g/mol = 16.00 g/mol
- Total: 44.06 g/mol
Divide the molar mass by the empirical formula mass:
- $\frac{88 \text{ g/mol}}{44.06 \text{ g/mol}}$ = 2
Multiply the empirical formula by 2:
- Molecular formula: C₄H₈O₂