Free Energy of Dissolution

Last Updated: September 24, 2024

Notes

Learning Objectives

By studying the topic of Free Energy of Dissolution for the AP Chemistry exam, you should be able to understand and calculate the Gibbs free energy change (ΔG) associated with the dissolution process, recognize the factors that influence the spontaneity of dissolution, and differentiate between the enthalpy (ΔH) and entropy (ΔS) components of the dissolution process. You should also be able to apply the concepts to predict whether a given dissolution will occur spontaneously at a specific temperature, use real-world examples such as the dissolution of ionic compounds in water, and explain the thermodynamic principles governing these processes.

Free AP Chemistry Practice Test

Introduction

Free energy of dissolution quantifies the spontaneity of a solute dissolving in a solvent, governed by the Gibbs free energy change (ΔG). This change combines the system’s enthalpy (ΔH) and entropy (ΔS). A negative ΔG indicates a spontaneous dissolution process, essential for predicting solubility and designing pharmaceuticals. Understanding this concept is crucial for applications involving solute-solvent interactions.

What is Free Energy of Dissolution?

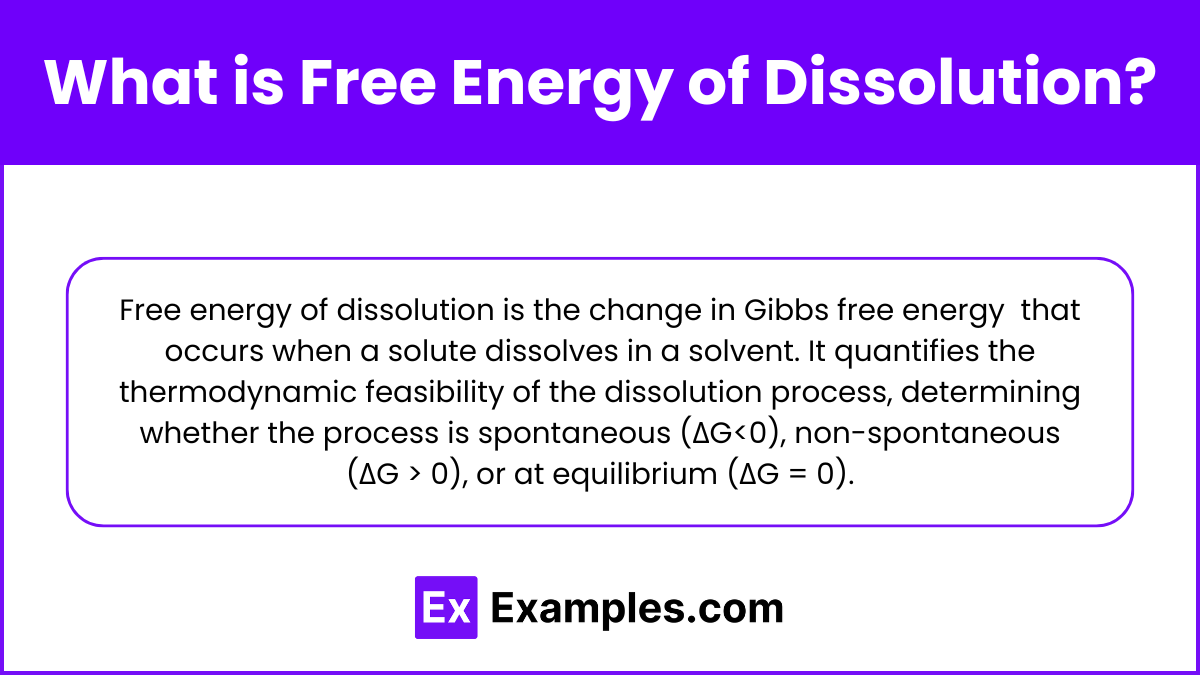
Free energy of dissolution is the change in Gibbs free energy (ΔG_dissolution) that occurs when a solute dissolves in a solvent. It quantifies the thermodynamic feasibility of the dissolution process, determining whether the process is spontaneous (ΔG<0), non-spontaneous (ΔG > 0), or at equilibrium (ΔG = 0).

Gibbs Free Energy (ΔG)

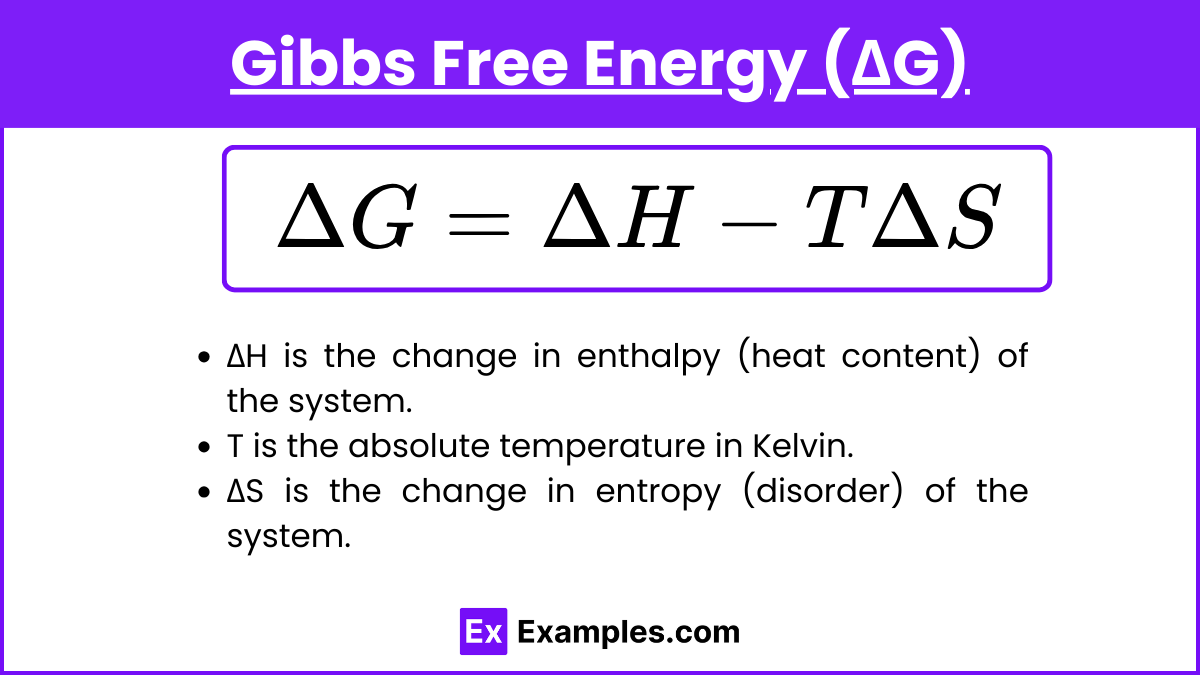
Gibbs free energy (ΔG) is a thermodynamic potential that measures the maximum amount of work that can be performed by a thermodynamic system at constant temperature and pressure. It is a crucial concept for predicting the spontaneity of chemical reactions and processes. The Gibbs free energy change for a process is given by the equation:

ΔG = ΔH − TΔS

where:

ΔH is the change in enthalpy (heat content) of the system.
T is the absolute temperature in Kelvin.
ΔS is the change in entropy (disorder) of the system.

Spontaneity of Dissolution

The spontaneity of dissolution refers to whether a solute will dissolve in a solvent without the input of external energy. This is determined by the change in Gibbs free energy (ΔG_dissolution) for the dissolution process.

Spontaneous Dissolution: If ΔG_dissolution < 0, the dissolution process is spontaneous.
Non-Spontaneous Dissolution: If ΔG_dissolution > 0, the dissolution process is non-spontaneous.
Equilibrium: If ΔG_dissolution = 0, the system is at equilibrium, and the dissolution process is neither spontaneous nor non-spontaneous.

Enthalpy of Solution (ΔH_solution)

Enthalpy of solution (ΔH_solution) is the total heat change that occurs when a solute dissolves in a solvent to form a solution. It is the sum of the energy changes involved in breaking the intermolecular forces within the solute and solvent, as well as the energy change when new interactions form between the solute and solvent molecules. The enthalpy of solution can be either positive (endothermic) or negative (exothermic) depending on the nature of the interactions.

Components of Enthalpy of Solution

Lattice Energy (ΔH_lattice):
- The energy required to break the ionic bonds in the solid solute. This process is always endothermic (positive ΔH) because energy is needed to overcome the electrostatic forces holding the ions together in the crystal lattice.
Hydration Energy (ΔH_hydration):
- The energy released when ions are surrounded by solvent molecules, usually water in aqueous solutions. This process is typically exothermic (negative ΔH) because new attractions are formed between the ions and the solvent molecules.

Calculation of Enthalpy of Solution

The enthalpy of solution can be expressed as:

ΔH_solution = ΔH_lattice + ΔH_hydration

Endothermic vs. Exothermic Dissolution

Endothermic Dissolution: When ΔH_solution is positive, the dissolution process absorbs heat from the surroundings. This happens when the energy required to break the solute-solvent interactions is greater than the energy released during the solvation process.
Exothermic Dissolution: When ΔH_solution is negative, the dissolution process releases heat to the surroundings. This occurs when the energy released during solvation is greater than the energy required to break the solute-solvent interactions.

Entropy of Solution (ΔS_solution)

Entropy of solution (ΔS_solution) is the change in entropy when a solute dissolves in a solvent to form a solution. Entropy (S) is a measure of the disorder or randomness in a system. When a solute dissolves, the overall disorder of the system typically changes, influencing the spontaneity of the dissolution process.

Key Points about Entropy of Solution

Increase in Disorder:
- When a solid solute dissolves in a solvent, the solute particles spread out and become more randomly distributed, usually increasing the entropy of the system.
- For ionic compounds, the ions separate and disperse throughout the solvent, significantly increasing disorder.
Factors Affecting ΔS_solution:
- Nature of Solute and Solvent: The degree of increase in entropy depends on the types of solute and solvent involved.
- Temperature: Higher temperatures generally increase the entropy of the solution because particles move more vigorously and spread out more.

Calculation of ΔS_solution

ΔS_solution can be determined by comparing the entropy of the solution to the entropy of the solute and solvent before mixing:

ΔS_solution = S_solution − (S_solute + S_solvent)

Spontaneity and Entropy

A positive ΔS_solution favors the spontaneity of the dissolution process because it contributes to a negative ΔG_dissolution (since ΔG = ΔH − TΔS).

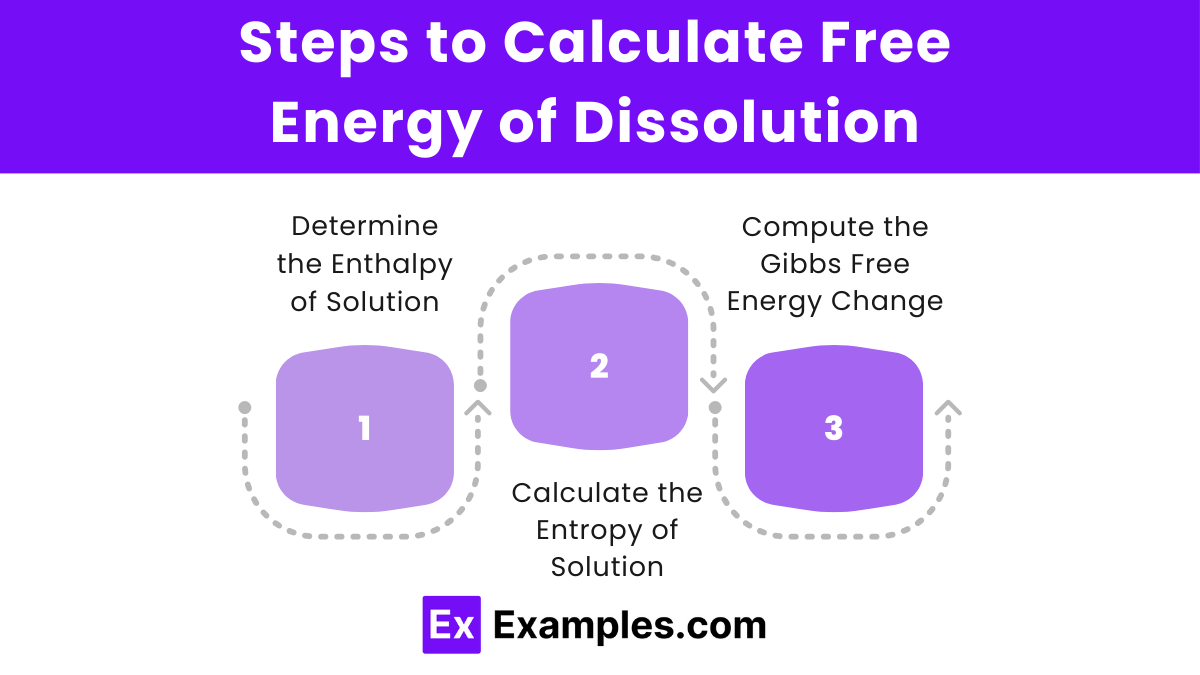
Steps to Calculate Free Energy of Dissolution

Determine the Enthalpy of Solution (ΔH_solution):
- Identify the lattice energy (ΔH_lattice), which is the energy required to break the bonds in the solid solute. This value is usually positive (endothermic).
- Identify the hydration (or solvation) energy (ΔH_hydration), which is the energy released when the solute particles interact with the solvent molecules. This value is usually negative (exothermic).
- Calculate ΔH_solution: ΔH_solution = ΔH_lattice + ΔH_hydration
Calculate the Entropy of Solution (ΔS_solution):
- Determine the entropy change associated with the dissolution process. This can often be found in tables or given in the problem statement.
- ΔS_solution is typically positive because the solute particles spread out and increase disorder in the solution.
Compute the Gibbs Free Energy Change (ΔG_dissolution):
- Use the Gibbs free energy equation to calculate ΔG_dissolution = ΔH_solution − TΔS_solution
- Here, T is the absolute temperature in Kelvin.

Factors Affecting Free Energy of Dissolution

Temperature (T): Higher temperatures can favor dissolution if ΔS_solution is positive, as TΔS_solution becomes larger.
Nature of Solute and Solvent: The type of solute and solvent affects ΔH_solution and ΔS_solution; ionic compounds often dissolve spontaneously in polar solvents.
Concentration: The initial concentration of solute and solvent can shift the equilibrium and affect ΔG_dissolution.
Pressure: Primarily affects gases; higher pressure increases solubility of gases in liquids, impacting ΔG_dissolution.
Intermolecular Forces: Stronger solute-solvent interactions (e.g., hydrogen bonding) can lower ΔH_solution, making dissolution more favorable.

Practice Problems

1. Dissolution of Potassium Nitrate (KNO₃) in Water

Determine $\Delta H_{\text{solution}}$ :
- Given: $\Delta H_{\text{lattice}} (KNO₃) = +34.9 kJ/mol$
- Given: $\Delta H_{\text{hydration}} (K⁺ and NO₃⁻) = -33.9 kJ/mol$
- Calculate $\Delta H_{\text{solution}}:$
- $\Delta H_{\text{solution}} = +34.9 \text{ kJ/mol} - 33.9 \text{ kJ/mol} = +1 \text{ kJ/mol}$
Calculate $\Delta S_{\text{solution}}</strong>: <ul> <li>Given: \(\Delta S_{\text{solution}} = +133 \text{ J/mol·K}$

Compute $\Delta G_{\text{dissolution}}</strong> at 298 K:  <ul> <li>\(\Delta G_{\text{dissolution}} = \Delta H_{\text{solution}} - T\Delta S_{\text{solution}}$

$\Delta G_{\text{dissolution}} = 1 \text{ kJ/mol} - 298 \text{ K} \times 0.133 \text{ kJ/mol·K}$

$\Delta G_{\text{dissolution}} = 1 \text{ kJ/mol} - 39.634 \text{ kJ/mol}$

$\Delta G_{\text{dissolution}} = -38.634 \text{ kJ/mol}$

Since $\Delta G_{\text{dissolution}}$ n is negative, the dissolution of KNO₃ in water is spontaneous at 298 K.

2: Dissolution of Ammonium Chloride (NH₄Cl) in Water

Determine $\Delta H_{\text{solution}}$ :

Given: $\Delta H_{\text{lattice}} (NH₄Cl) = +16.2 kJ/mol$

Given: $\Delta H_{\text{hydration}} (NH₄⁺ and Cl⁻) = -37.3 kJ/mol$

Calculate $\Delta H_{\text{solution}}:$

$\Delta H_{\text{solution}} = +16.2 \text{ kJ/mol} - 37.3 \text{ kJ/mol} = -21.1 \text{ kJ/mol}$

Calculate $\Delta S_{\text{solution}}$ :

Given: $\Delta S_{\text{solution}} = +108 \text{ J/mol·K}$

Compute $\Delta G_{\text{dissolution}}$  at 298 K:

$\Delta G_{\text{dissolution}} = \Delta H_{\text{solution}} - T\Delta S_{\text{solution}}$

$\Delta G_{\text{dissolution}} = -21.1 \text{ kJ/mol} - 298 \text{ K} \times 0.108 \text{ kJ/mol·K}$

$\Delta G_{\text{dissolution}} = -21.1 \text{ kJ/mol} - 32.184 \text{ kJ/mol}$

$\Delta G_{\text{dissolution}} = -53.284 \text{ kJ/mol}$

Since $\Delta G_{\text{dissolution}}$ is negative, the dissolution of NH₄Cl in water is spontaneous at 298 K.