Introduction to Rate Law
Learning Objectives
In AP Chemistry, the topic “Introduction to Rate Law” focuses on understanding how the rate of a chemical reaction depends on the concentration of reactants, determining the rate law from experimental data, calculating reaction order, using graphical methods to identify reaction orders, and comprehending the impact of temperature on the rate constant through the Arrhenius equation.
Introduction
the rate law is a fundamental concept that describes the relationship between the rate of a chemical reaction and the concentrations of its reactants. By understanding the rate law, students can determine how changes in reactant concentrations affect the reaction rate, identify the order of reactions through experimental data, and use mathematical and graphical methods to analyze reaction kinetics. This knowledge is crucial for predicting reaction behavior and for manipulating conditions to achieve desired reaction rates in both laboratory and industrial settings.
What is Rate Law?
The rate law is an equation that expresses the rate of a chemical reaction as a function of the concentrations of its reactants, raised to specific powers, known as reaction orders, which are determined experimentally.
General Form of a Rate Law
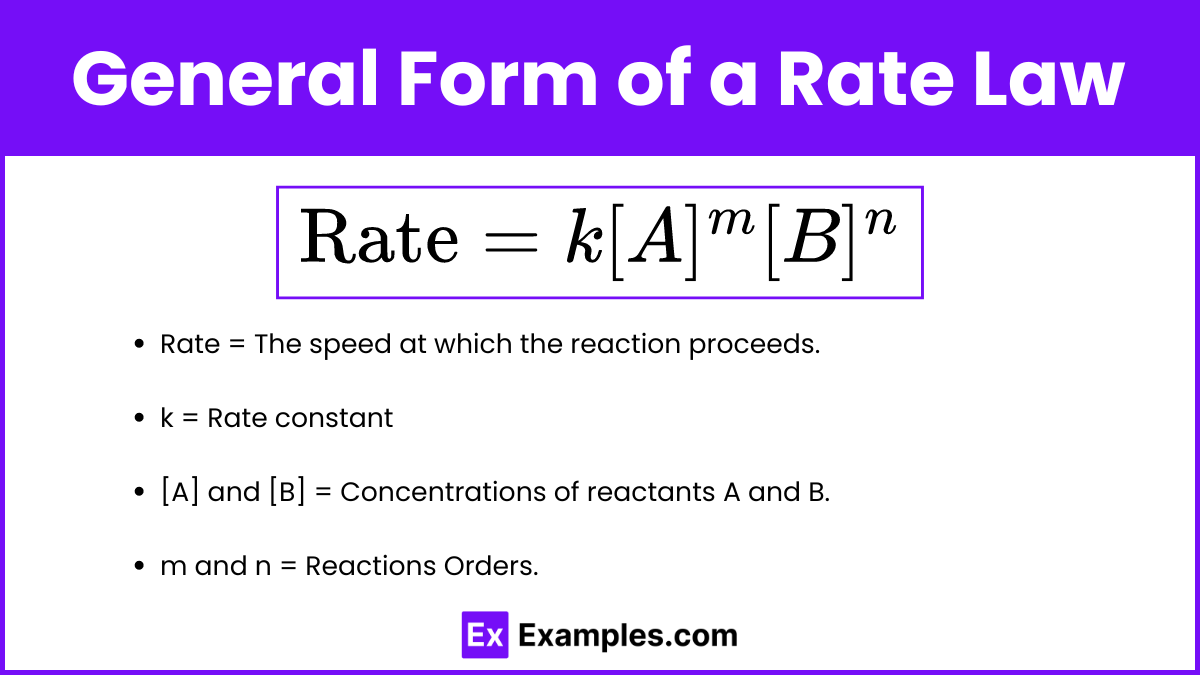
The general form of a rate law for a reaction involving reactants A and B can be expressed as:
\(\text{Rate} = k[A]^m[B]^n\)
- Rate: The speed at which the reaction proceeds.
- k: The rate constant, a proportionality factor specific to the reaction at a given temperature.
- [A] and [B]: The molar concentrations of reactants A and B.
- m and n: The reaction orders with respect to reactants A and B, respectively, which are determined experimentally and indicate how the rate is affected by changes in concentration of each reactant.
Types of Rate Laws
Zero-Order Reactions
Definition: A zero-order reaction has a rate that is independent of the concentration of the reactant(s).
Rate Law: Rate = k
Characteristics:
- The reaction rate remains constant regardless of changes in reactant concentration.
- Common in reactions where a catalyst is saturated by the reactant.
Graphical Representation:
- A plot of reactant concentration [A] vs. time is a straight line with a slope of −k.
First-Order Reactions
Definition: A first-order reaction has a rate that is directly proportional to the concentration of one reactant.
Rate Law: Rate = k[A]
Characteristics:
- The rate changes linearly with changes in reactant concentration.
- Common in simple decomposition or radioactive decay reactions.
Graphical Representation:
- A plot of the natural logarithm of reactant concentration ln[A] vs. time is a straight line with a slope of −k.
Second-Order Reactions
Definition: A second-order reaction has a rate that is proportional to either the square of the concentration of one reactant or the product of the concentrations of two reactants.
Rate Laws:
- For a single reactant: Rate = k[A]²
- For two reactants: Rate = k[A][B]
Characteristics:
- The rate increases with the square of the concentration of one reactant or the product of the concentrations of two reactants.
- Common in reactions involving bimolecular collisions.
Graphical Representation:
- For a single reactant, a plot of 1/[A] vs. time is a straight line with a slope of k.
- For two reactants, the analysis involves more complex kinetics but can also be linearized under certain conditions.
Determining the Rate Law
Determining the rate law of a reaction involves several steps and methods to understand how the reaction rate depends on the concentration of reactants. The primary techniques include the method of initial rates and integrated rate laws.
Method of Initial Rates
- Conduct Experiments: Perform a series of experiments where the initial concentrations of the reactants are varied while measuring the initial rate of the reaction.
- Compare Rates: Analyze how the initial rates change with different initial concentrations of reactants.
- Determine Reaction Orders:
- For a reaction aA + bB → cCa:
- Compare experiments where the concentration of one reactant changes while the others remain constant.
- Use the formula: \(\frac{\text{Rate}_1}{\text{Rate}_2} = \left( \frac{[A]_1}{[A]_2} \right)^m \left( \frac{[B]_1}{[B]_2} \right)^n\)
- Solve for the reaction orders m and n.
- For a reaction aA + bB → cCa:
Integrated Rate Laws
Integrated rate laws provide a way to determine reaction order by analyzing concentration vs. time data. Each reaction order has a specific integrated rate law that relates the concentration of reactants to time.
Zero-Order Reactions
For zero-order reactions, the rate is independent of the concentration of the reactant:
[A]ₜ = [A]₀ − kt
First-Order Reactions
For first-order reactions, the rate is directly proportional to the concentration of the reactant:
ln[A]ₜ = ln[A]₀ − kt
Second-Order Reactions
For second-order reactions, the rate is proportional to the square of the concentration of the reactant:
\(\frac{1}{[A]_t} = \frac{1}{[A]_0} + kt[A]t1=[A]01+kt\)
Units of the Rate Constant (k)
| Reaction Order | Rate Law Example | Units of k |
|---|---|---|
| Zero-Order | Rate = k | M/s |
| First-Order | Rate = k[A] | s⁻¹ |
| Second-Order | Rate=k[A]² | M⁻¹ s⁻¹ |
Determining Reaction Order
Determining the reaction order involves analyzing how the rate of reaction depends on the concentration of reactants. The methods include:
Method of Initial Rates
- Perform experiments with varying initial concentrations of reactants.
- Measure initial rates of the reaction.
- Compare how initial rates change with different concentrations to determine reaction orders.
Half-Life (t1/2)
| Reaction Order | Half-Life (t1/2) |
|---|---|
| Zero-Order | t1/2 = \(\frac{[A]_0}{2k}\) |
| First-Order | t1/2 = \(\frac{0.693}{k}\) |
| Second-Order | t1/2 = \(\frac{1}{k[A]_0}\) |
Temperature and Rate Constant
The rate constant kkk is temperature-dependent and can be described by the Arrhenius equation:
\(\text{k} = \text {A} e^{-\frac{E_a}{RT}}\)
Where:
- k: Rate constant
- A: Pre-exponential factor (frequency of collisions)
- Eₐ: Activation energy
- R: Universal gas constant (8.314 J/mol·K)
- T: Temperature in Kelvin