pH and pKₐ

Learning Objectives

By studying the topic of pH and pKₐ for the AP Chemistry exam, you should be able to understand and apply the concepts of pH and pKₐ , including their definitions and mathematical relationships. You should learn to calculate pH from hydrogen ion concentration and vice versa, understand the significance of the pH scale, and interpret the strength of acids and bases using pKₐ values. Mastery of the Henderson-Hasselbalch equation is essential for calculating the pH of buffer solutions and understanding the equilibrium between acids and their conjugate bases. Additionally, you should be able to solve problems involving acid-base titrations, analyze titration curves, and predict the behavior of buffer systems in various chemical contexts.

Introduction

pH measures the acidity or basicity of a solution, defined as the negative logarithm of the hydrogen ion concentration [H⁺], with low pH indicating high acidity and high pH indicating high basicity. pKₐ is the negative logarithm of the acid dissociation constant (Kₐ), indicating acid strength; a lower pKₐ means a stronger acid that dissociates more in water. Understanding pH and pKₐ helps predict the behavior of acids and bases in chemical reactions and biological systems.

pH

What is pH?

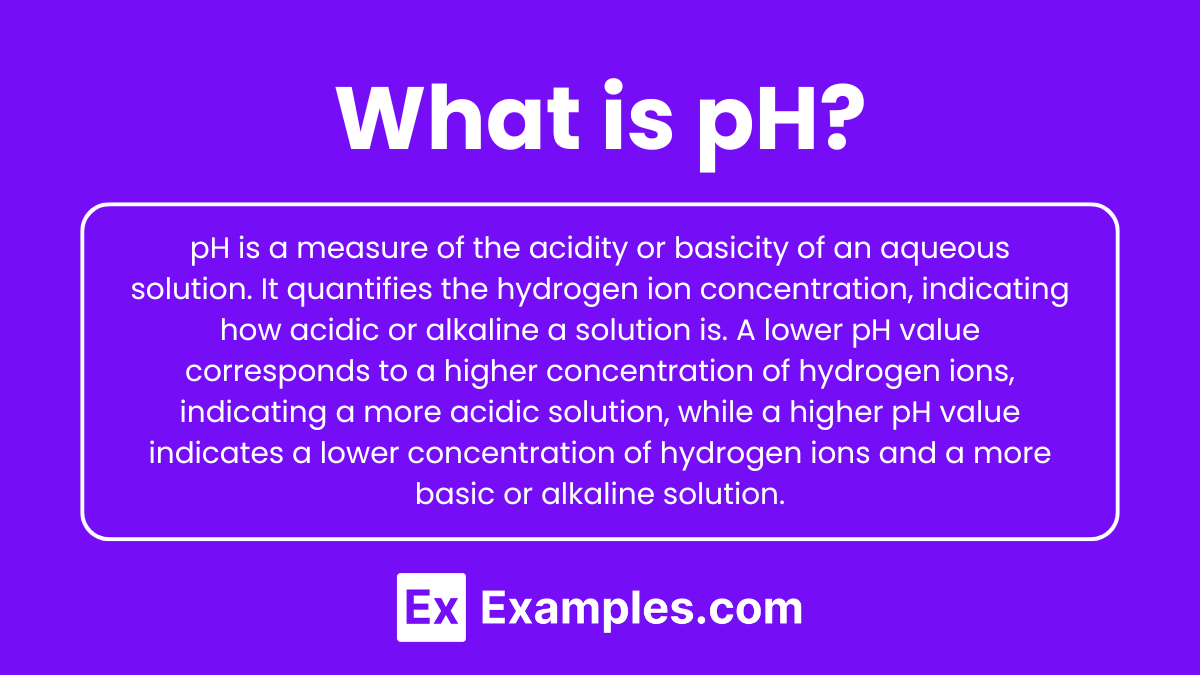
pH is a measure of the acidity or basicity of an aqueous solution. It quantifies the hydrogen ion concentration, indicating how acidic or alkaline a solution is. A lower pH value corresponds to a higher concentration of hydrogen ions, indicating a more acidic solution, while a higher pH value indicates a lower concentration of hydrogen ions and a more basic or alkaline solution.

pH Scale

The pH scale is a logarithmic scale that ranges from 0 to 14 and is used to specify the acidity or basicity of an aqueous solution. It provides a measure of the hydrogen ion concentration in a solution.

Key Points on the pH Scale

• Acidic Solutions: pH < 7
  • These solutions have a higher concentration of hydrogen ions (H⁺).
  • Examples: Lemon juice (pH ~2), Vinegar (pH ~2.4), Stomach acid (pH ~1.5-3.5).
• Neutral Solutions: pH = 7
  • Pure water is neutral with equal concentrations of hydrogen ions (H⁺) and hydroxide ions (OH⁻).
  • Example: Pure water (pH 7).
• Basic (Alkaline) Solutions: pH > 7
  • These solutions have a lower concentration of hydrogen ions (H⁺) and higher concentration of hydroxide ions (OH⁻).
  • Examples: Baking soda solution (pH ~8.3), Soap solution (pH ~9-10), Bleach (pH ~12.5).

Visualization of the pH Scale

pH Value	Description	Example
0	Very Strong Acid	Battery acid
1	Strong Acid	Hydrochloric acid
2	Strong Acid	Lemon juice
3	Moderate Acid	Vinegar
4	Weak Acid	Tomato juice
5	Weak Acid	Black coffee
6	Very Weak Acid	Milk
7	Neutral	Pure water
8	Very Weak Base	Sea water
9	Weak Base	Baking soda solution
10	Weak Base	Great Salt Lake
11	Moderate Base	Ammonia solution
12	Strong Base	Soapy water
13	Strong Base	Bleach
14	Very Strong Base	Liquid drain cleaner

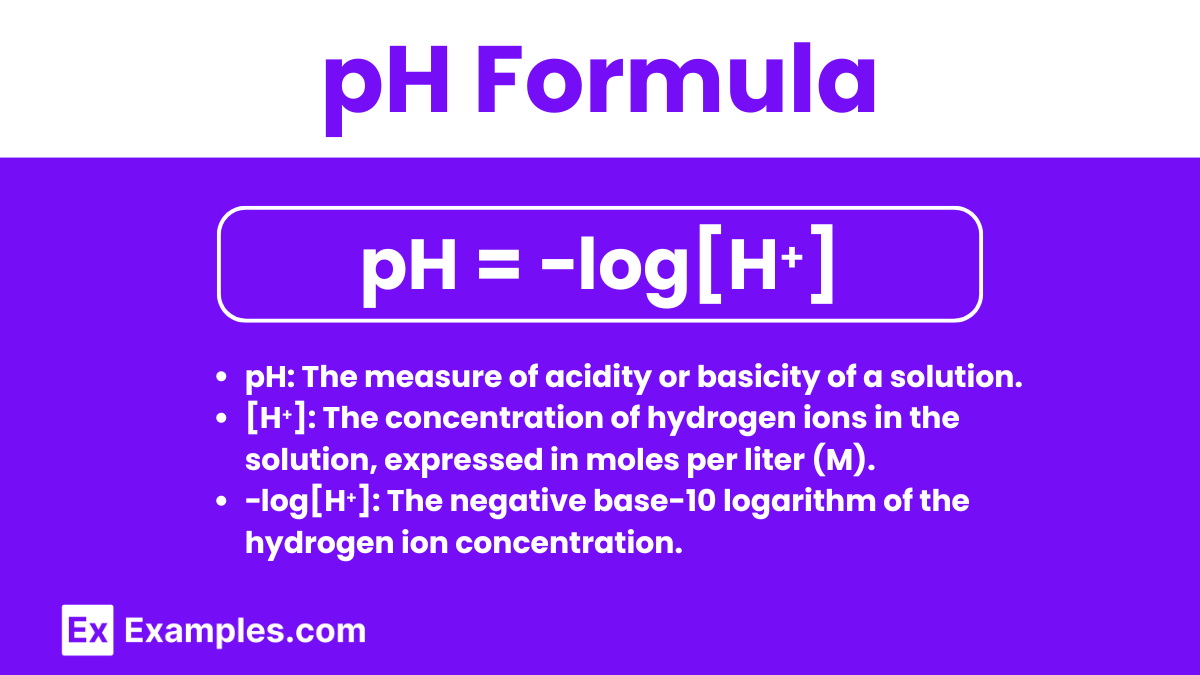
pH Formula

The pH formula is used to calculate the pH of an aqueous solution based on the concentration of hydrogen ions ([H⁺]) present. The formula is:

pH = −log⁡[H⁺]

• pH: The measure of acidity or basicity of a solution.
• [H⁺]: The concentration of hydrogen ions in the solution, expressed in moles per liter (M).
• −log⁡[H⁺]: The negative base-10 logarithm of the hydrogen ion concentration.

pKₐ

What is pKₐ?

pKₐ is a measure of the strength of an acid, indicating the degree to which an acid dissociates in water. It represents the negative base-10 logarithm of the acid dissociation constant (Kₐ). A lower pKₐ value signifies a stronger acid that dissociates more completely in solution, while a higher pKₐ value indicates a weaker acid with less dissociation.

pKₐ Formula

The pKa formula is used to express the acid dissociation constant (Ka) in logarithmic terms. The formula is:

pKₐ = −log⁡Kₐ

• pKₐ: The negative base-10 logarithm of the acid dissociation constant.
• Kₐ: The acid dissociation constant, which measures the strength of an acid in solution.

Significance of pKₐ

• Indicator of Acid Strength: Lower pKₐ values indicate stronger acids that dissociate more completely, while higher pKₐ values indicate weaker acids with less dissociation.
• Predicts Proton Donation: pKₐ helps determine how readily an acid donates protons (H⁺) in an aqueous solution.
• Designing Buffer Solutions: pKₐ is essential in selecting appropriate acids for buffer solutions, as buffers are most effective when the pH is close to the acid’s pKₐ.
• Determining pH: Knowing the pKₐ helps in calculating the pH of a solution, especially in systems involving weak acids and their conjugate bases.
• Understanding Chemical Equilibrium: pKₐ values assist in predicting the direction of acid-base reactions, favoring the formation of weaker acids and bases.

Examples of pKₐ

1. Hydrochloric Acid (HCl)
   • pKa: -6.3
   • Description: A very strong acid that dissociates almost completely in water.
2. Sulfuric Acid (H₂SO₄)
   • pKa₁: -3 (for the first dissociation)
   • Description: A strong acid with the first dissociation being very complete.
3. Acetic Acid (CH₃COOH)
   • pKa: 4.76
   • Description: A weak acid commonly found in vinegar, partially dissociates in water.
4. Carbonic Acid (H₂CO₃)
   • pKa₁: 6.35 (for the first dissociation)
   • Description: A weak acid formed in solution when carbon dioxide dissolves in water.
5. Ammonium Ion (NH₄⁺)
   • pKa: 9.25
   • Description: A weak acid formed from ammonia, which partially dissociates in water.

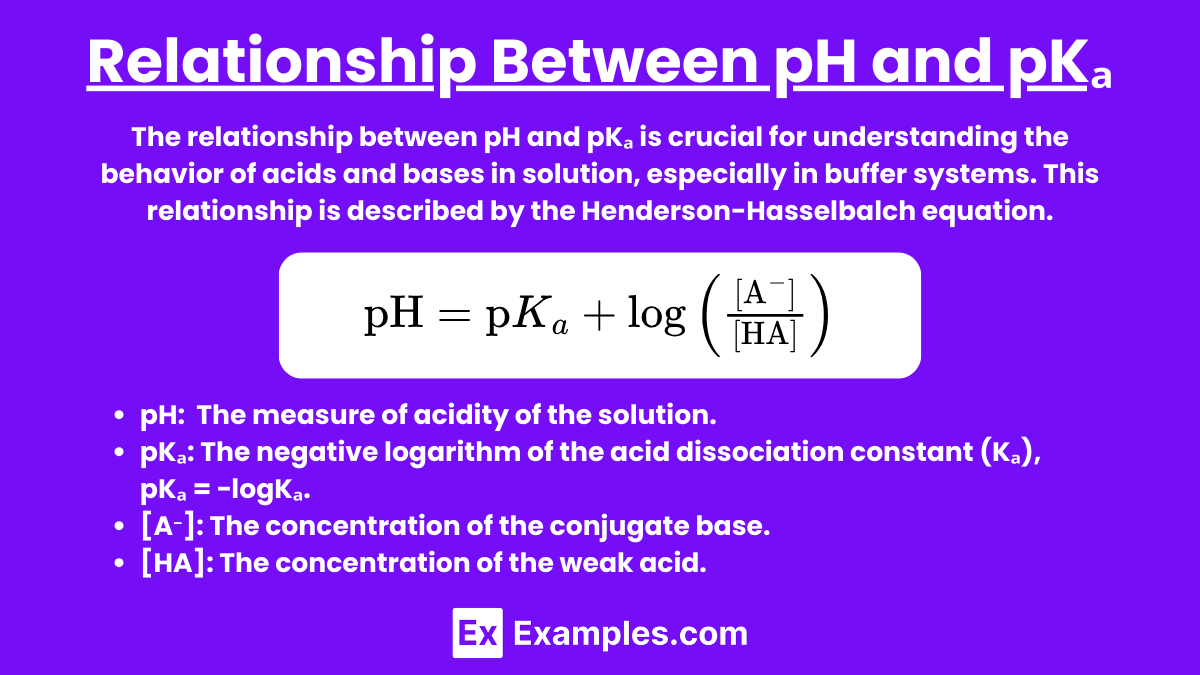
Relationship Between pH and pKₐ

The relationship between pH and pKₐ is crucial for understanding the behavior of acids and bases in solution, especially in buffer systems. This relationship is described by the Henderson-Hasselbalch equation.

Henderson-Hasselbalch Equation

The Henderson-Hasselbalch equation is used to calculate the pH of a solution containing a weak acid and its conjugate base:

\(\text{pH} = \text{pK_a} + \log \left( \frac{[\text{A}^-]}{[\text{HA}]} \right)\)

Where:

• pH is the measure of acidity or basicity of the solution.
• pKₐ is the acid dissociation constant of the weak acid.
• [A⁻] is the concentration of the conjugate base.
• [HA] is the concentration of the weak acid.

Key Points in the Relationship

• When pH = pKₐ: The concentrations of the acid (HA) and its conjugate base (A⁻) are equal. This is the point of maximum buffering capacity.
• When pH < pKₐ: The solution contains more of the protonated form (HA), indicating a more acidic environment.
• When pH > pKₐ: The solution contains more of the deprotonated form (A⁻), indicating a more basic environment.

Practical Implications

1. Buffer Solutions:
   • Buffers resist changes in pH upon the addition of small amounts of acids or bases. They work most effectively when the pH is close to the pKa of the acid in the buffer.
   • Example: A buffer made from acetic acid (pKₐ = 4.76) and sodium acetate will be most effective in the pH range of approximately 3.76 to 5.76.
2. Titration Curves:
   • During the titration of a weak acid with a strong base, the pH at the halfway point to the equivalence point equals the pKa of the weak acid.
   • Example: If you titrate acetic acid with sodium hydroxide, the pH at the halfway point of the titration curve will be around 4.76.
3. Predicting the Ionization State:
   • Knowing the pH and pKa helps predict whether an acid or base will be in its protonated or deprotonated form in a given environment.
   • Example: In a solution with a pH of 7, an acid with a pKₐ of 4.76 will primarily be in its deprotonated form (A⁻).

Calculating pH from pKₐ and Concentrations

To calculate the pH of a solution using the pKa and the concentrations of the acid and its conjugate base, follow these steps:

1. Identify the Components:
   • Determine the concentration of the acid ([HA]).
   • Determine the concentration of the conjugate base ([A⁻]).
   • Find the pKa value of the acid.
2. Use the Henderson-Hasselbalch Equation:
   • \(\text{pH} = \text{pKa} + \log \left( \frac{[\text{A}^-]}{[\text{HA}]} \right)\)
3. Substitute the Values:
   • Plug the concentrations of the conjugate base ([A⁻]) and the acid ([HA]) into the equation.
   • Plug the pKₐ value into the equation.
4. Calculate the Ratio:
   • Calculate the ratio \(\frac{[\text{A}^-]}{[\text{HA}]}\).
5. Find the Logarithm:
   • Determine the logarithm (base 10) of the ratio calculated in the previous step.
6. Add the pKₐ:
   • Add the pKₐ value to the logarithm obtained in the previous step.
7. Determine the pH:
   • The resulting value is the pH of the solution.

Applications of pH and pKₐ

• Buffer Solutions: Used in biological systems to maintain pH levels, in laboratory experiments for stable reaction conditions, and in industrial processes such as fermentation and pharmaceutical production.
• Acid-Base Titrations: Essential for chemical analysis to determine unknown concentrations, in pharmaceuticals for ensuring drug stability and efficacy, and in environmental testing to assess pollution levels in soil and water.
• Pharmaceutical Formulation: Critical for predicting drug solubility and stability, optimizing drug absorption in the body, and designing controlled-release systems for medications.
• Environmental Science: Important for monitoring water quality, managing soil pH for agriculture, and treating industrial waste to prevent environmental contamination.
• Food and Beverage Industry: Used to control pH during fermentation, preserve food by inhibiting microbial growth, and optimize the flavor and texture of food products.
• Biochemical and Molecular Biology: Regulates enzyme activity, affects protein folding by influencing amino acid ionization, and impacts the stability and hybridization of nucleic acids.
• Clinical Diagnostics: Monitors blood pH in medical conditions, analyzes urine pH for diagnosing health issues, and tailors medication management based on pH and pKa values.
• Cosmetic Industry: Ensures that skincare and haircare products have suitable pH levels, enhancing product stability and efficacy for better performance.