Profit-Maximizing Behavior in Perfectly Competitive Factor Markets

Last Updated: October 3, 2024

Notes

In AP Microeconomics, understanding profit-maximizing behavior in perfectly competitive factor markets is essential for grasping how firms make optimal input decisions. In such markets, numerous buyers and sellers interact, ensuring that no single entity can influence factor prices. Firms aim to maximize profits by equating the marginal revenue product of each factor to its respective price. This behavior ensures efficient allocation of resources, reflecting the core principles of supply and demand within the competitive framework. Mastery of this topic is crucial for excelling in the AP Microeconomics exam.

Free AP Microeconomics Practice Test

Learning Objectives

By studying “Profit-Maximizing Behavior in Perfectly Competitive Factor Markets,“ you will understand how firms determine the optimal combination of input factors to maximize profits. Specifically, you will learn to define and apply concepts such as marginal product (MP) and marginal revenue product (MRP), analyze how derived demand influences factor demand, and apply the profit-maximizing rule where MRP = factor price. Additionally, you will be able to interpret and construct factor market diagrams, distinguish between short-run and long-run factor decisions, and evaluate the effects of changes in technology and factor prices on market equilibrium. Mastery of these objectives will enhance your ability to analyze firm behavior in competitive markets effectively.

In a perfectly competitive factor market, firms are price-takers, meaning they cannot influence the price of the factor they are purchasing (e.g., labor or capital). Profit-maximizing behavior in such a market follows specific principles.

Key Concepts of Profit-maximizing behavior

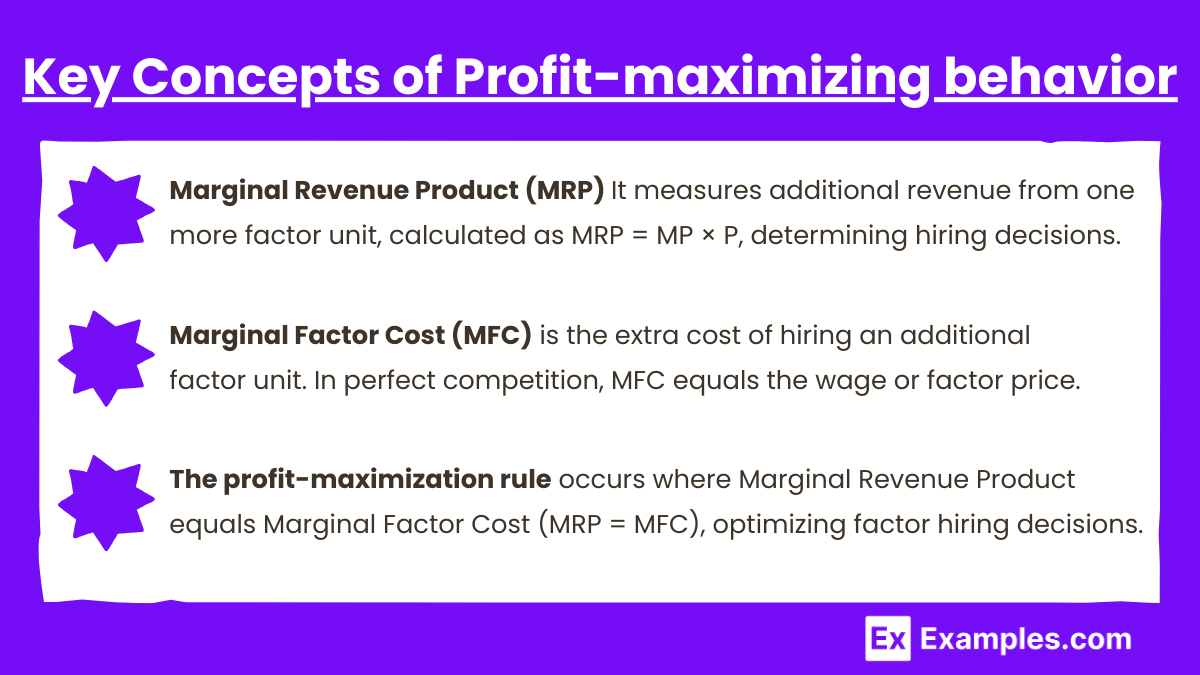
Marginal Revenue Product (MRP): The Marginal Revenue Product of a factor (e.g., labor) is the additional revenue generated from employing one more unit of that factor. It is calculated by multiplying the Marginal Product (MP) of the factor by the price of the output (MRP = MP × P). Firms will hire more of a factor until the Marginal Revenue Product equals the factor’s cost.
Marginal Factor Cost (MFC): The Marginal Factor Cost is the additional cost incurred by hiring one more unit of a factor. In a perfectly competitive factor market, the MFC is equal to the wage rate or the price of the factor. In essence, the MFC is constant and horizontal, indicating that the firm can hire as much labor as it wants at the market wage.
Profit-Maximization Rule: A firm maximizes profit in a factor market by hiring the quantity of the factor where the Marginal Revenue Product (MRP) equals the Marginal Factor Cost (MFC): MRP = MFC At this point, the additional revenue from hiring one more unit of the factor equals the additional cost, maximizing profit.

Perfect Competition in Factor Markets

For factor markets to be perfectly competitive, they must exhibit the following characteristics:

Many Buyers and Sellers: No single buyer or seller can influence the market price.
Homogeneous Factors: All units of a factor (e.g., labor) are identical in quality and characteristics.
Perfect Information: All market participants have full knowledge about prices and available factors.
Free Entry and Exit: Firms and individuals can freely enter or leave the market without significant barriers.
Factor Mobility: Factors can move freely between different uses and industries.

Graphical Representation

Demand Curve for Factors: The firm’s demand for a factor is derived from the Marginal Revenue Product curve. This curve slopes downward because of diminishing marginal returns—each additional unit of the factor contributes less to output, hence less to revenue.
Supply Curve for Factors: In a perfectly competitive market, the supply curve facing the individual firm is perfectly elastic (horizontal) at the prevailing wage or factor price.

Implications of Profit-maximizing

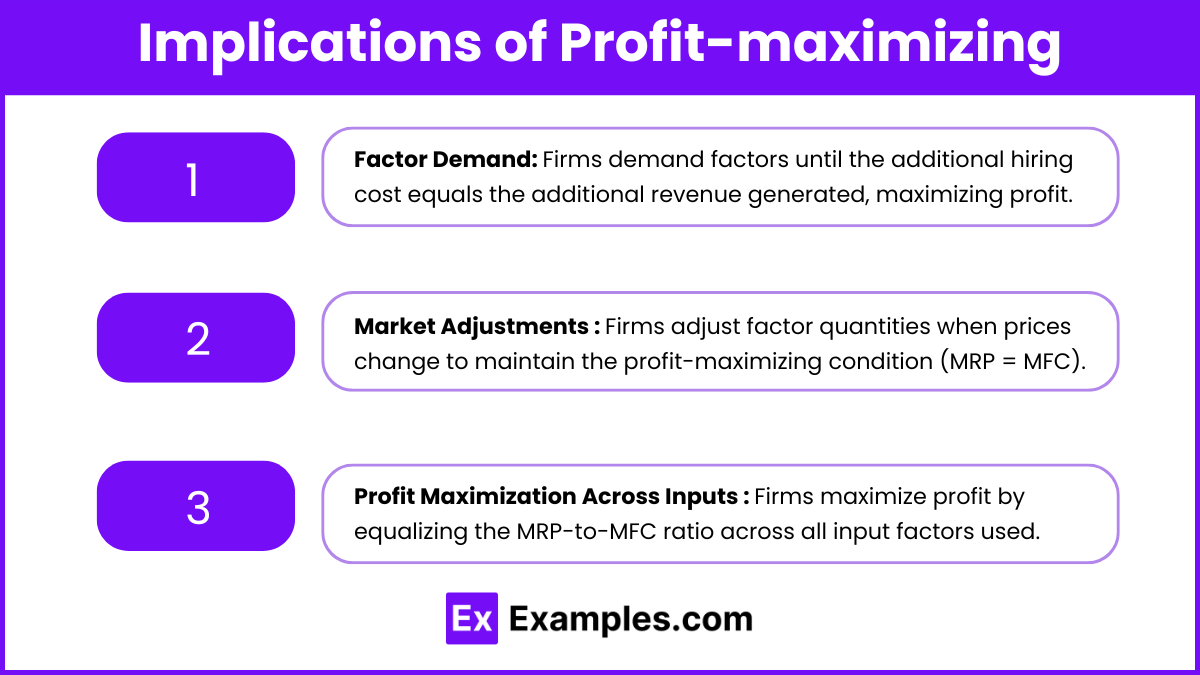
Factor Demand: Firms demand factors up to the point where the additional cost of hiring equals the additional revenue generated by hiring.
Market Adjustments: If the price of the factor changes, firms will adjust the quantity of the factor they employ to maintain the profit-maximizing condition (MRP = MFC).
Profit Maximization Across Inputs: Firms that use multiple factors (e.g., labor and capital) will allocate resources such that the ratio of Marginal Revenue Product to Marginal Factor Cost is equal across all factors, optimizing their input mix.

Applications

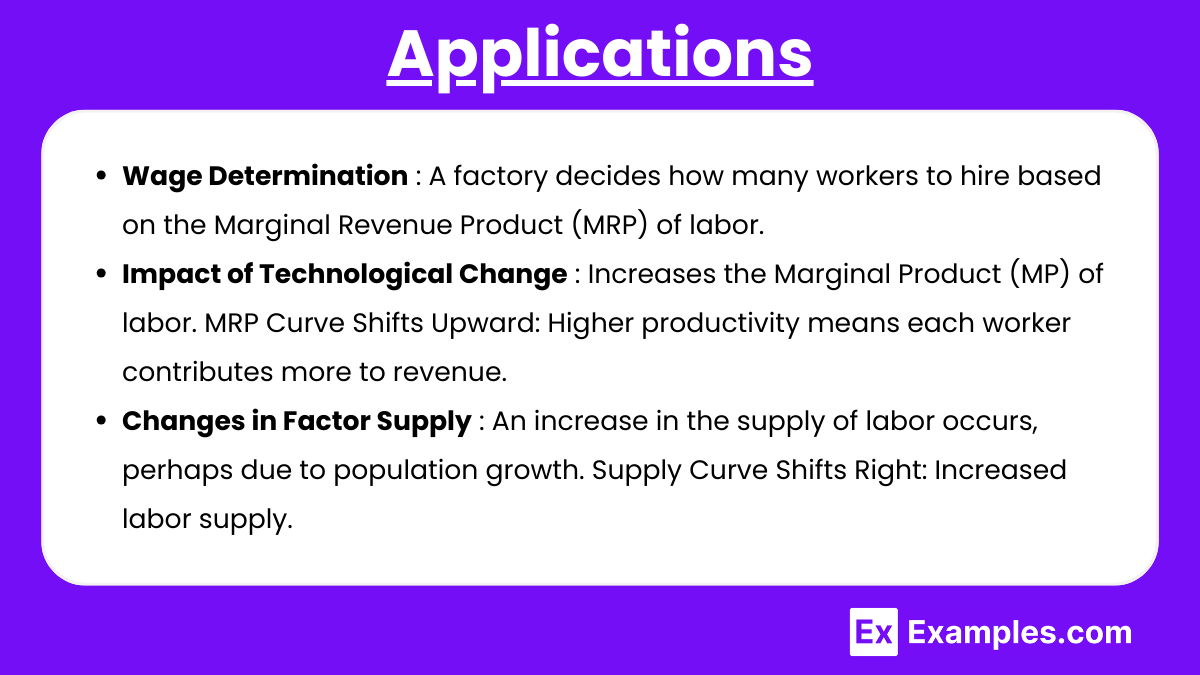
Wage Determination : A factory decides how many workers to hire based on the Marginal Revenue Product (MRP) of labor.
Impact of Technological Change :Increases the Marginal Product (MP) of labor. MRP Curve Shifts Upward: Higher productivity means each worker contributes more to revenue.
Changes in Factor Supply : An increase in the supply of labor occurs, perhaps due to population growth. Supply Curve Shifts Right: Increased labor supply.

Examples

Example 1. Wage Determination in the Fast-Food Industry

Scenario: Consider a fast-food restaurant chain operating in a perfectly competitive labor market. The firm needs to hire cashiers to manage customer orders and transactions.

Profit-Maximizing Behavior: The restaurant assesses the Marginal Revenue Product of Labor (MRP_L) for each cashier, which is the additional revenue generated by hiring one more cashier. Suppose each cashier can handle 50 transactions per day, and each transaction results in an average revenue of $5. Therefore, the MRP_L is:

MRPL = 50 transactions × $5 / transaction = $250

If the prevailing wage rate (W) in the market is $200 per day, since MRPL($250)>W($200), the restaurant hires additional cashiers. This process continues until MRPL=W, ensuring that the cost of hiring an additional cashier equals the revenue they generate, thereby maximizing profits.

Example 2. Capital Investment in the Automotive Manufacturing Sector

Scenario: An automotive manufacturer operates in a perfectly competitive capital market, deciding whether to invest in new robotic assembly lines.

Profit-Maxizing Behavior: The firm evaluates the Marginal Revenue Product of Capital (MRP_K), which is the additional revenue from investing in one more unit of capital (e.g., a robotic arm). Suppose each robotic arm increases production by 100 cars annually, and each car sells for $20,000. The MRP_K is:

MRPK = 100 cars × $20,000 /car = $2,000,000

If the cost of purchasing and maintaining a robotic arm (capital price) is $1,800,000 per year, since MRPK($2,000,000)>Capital Price($1,800,000), the manufacturer invests in additional robotic arms. The firm continues to add capital until MRPK=Capital Price, ensuring optimal use of capital resources to maximize profits.

Example 3. Land Rental Decisions in Urban Real Estate Development

Scenario: A real estate developer operates in a perfectly competitive land market, deciding how much land to lease for constructing apartment buildings in a metropolitan area.

Profit-Maximizing Behavior: The developer calculates the Marginal Revenue Product of Land (MRP_Land), which is the additional revenue generated from leasing one more acre of land. Suppose leasing an acre allows the construction of 10 apartment units, each renting for $1,000 per month. The MRP_Land is:

MRPLand = 10 units × $1,000 /unit /month × 12 months = $120,000 per acre annually

If the rental price of land is $100,000 per acre annually, since MRPLand($120,000) > Land Rental Price($100,000), the developer leases additional land. This decision continues until MRPLand = Land Rental Price, ensuring that the cost of leasing land is justified by the revenue it generates.

Example 4. Hiring Software Developers in the Technology Sector

Scenario: A tech startup operates in a perfectly competitive labor market for software developers, aiming to build a new application.

Profit-Maximizing Behavior: The startup determines the Marginal Revenue Product of Labor (MRP_L) for each software developer. Assume each developer can complete 2 projects per year, with each project generating $150,000 in revenue. The MRP_L is:

MRPL = 2 projects × $150,000 / project = $300,000

If the market wage for a software developer is $250,000 per year, since MRPL($300,000) > W($250,000), the startup hires additional developers. This hiring continues until MRPL= W, ensuring that each developer’s cost is covered by the revenue they produce, thereby maximizing the firm’s profits.

Example 5. Utilization of Raw Materials in the Textile Industry

Scenario: A textile manufacturer operates in a perfectly competitive market for raw cotton, which is a key input in producing fabrics.

Profit-Maximizing Behavior: The manufacturer assesses the Marginal Revenue Product of Cotton (MRP_Cotton), which is the additional revenue from using one more kilogram of cotton. Suppose using an extra kilogram of cotton produces 0.5 meters of fabric, and each meter sells for $40. The MRP_Cotton is:

MRPCotton = 0.5 meters × $40 /meter = $20 per kilogram

If the market price for raw cotton is $15 per kilogram, since MRPCotton($20) > Cotton Price($15), the manufacturer purchases additional cotton. This continues until MRPCotton = Cotton Price, ensuring that the cost of raw materials is justified by the revenue from the final product, thereby maximizing profits.

Multiple Choice Questions

Question 1

In a perfectly competitive labor market, a firm will hire additional units of labor until:

A) The wage rate is greater than the marginal revenue product (MRP).
B) The marginal revenue product (MRP) equals the marginal factor cost (MFC).
C) The wage rate equals the average revenue product (ARP).
D) The marginal revenue product (MRP) is greater than the marginal factor cost (MFC).

Answer: B) The marginal revenue product (MRP) equals the marginal factor cost (MFC).

Explanation: In a perfectly competitive factor market, firms maximize profit by hiring labor up to the point where the Marginal Revenue Product (MRP) of labor equals the Marginal Factor Cost (MFC), which is the wage rate in this context. The MRP is the additional revenue the firm earns from hiring one more worker, while the MFC represents the cost of hiring that worker. If MRP > MFC, the firm can increase profit by hiring more labor. If MRP < MFC, the firm would reduce its labor force to avoid losses. Thus, the profit-maximizing condition is when MRP = MFC.

Question 2

If a firm in a perfectly competitive factor market hires 10 workers, and the marginal revenue product (MRP) of the 10th worker is $50, while the wage rate (MFC) is $60, what should the firm do to maximize profit?

A) Hire more workers.
B) Hire fewer workers.
C) Keep the number of workers the same.
D) Increase the price of its output.

Answer: B) Hire fewer workers.

Explanation:

Since the marginal revenue product (MRP) of the 10th worker is less than the marginal factor cost (MFC), the firm is paying more for the labor than it is gaining in revenue from the additional worker. This means that hiring this worker is decreasing the firm’s profit. To maximize profit, the firm should reduce the number of workers it employs until MRP = MFC. In this case, it should hire fewer workers until the MRP of labor equals the wage rate.

Question 3

Which of the following describes the shape of the labor supply curve facing an individual firm in a perfectly competitive labor market?

A) Upward-sloping.
B) Downward-sloping.
C) Vertical.
D) Horizontal.

Answer: D) Horizontal.

Explanation: In a perfectly competitive labor market, the labor supply curve facing an individual firm is perfectly elastic (horizontal). This is because the firm is a “wage taker,” meaning it can hire as many workers as it wants at the prevailing market wage. The firm does not have any power to influence the wage rate, so the marginal factor cost (MFC) is constant, leading to a horizontal labor supply curve. This differs from the overall market labor supply curve, which is upward-sloping because higher wages will generally attract more workers into the labor market.