Explore Examples.com to effortlessly convert length from kilometers to miles and vice versa. Simply input your values for quick and precise results.
km to mi
Formula: Length in miles (mi) = Length in kilometers (km) × 0.621371
Kilometer:
Mile:
| kilometers | Miles |
|---|---|
| 1 | 0.621371 |
mi to km
Formula: Length in kilometers (km) = Length in miles (mi) ÷ 0.621371
Mile:
Kilometer:
| Miles | Kilometers |
|---|---|
| 1 | 1.609340 |
Length Converters to Kilometer (km)
| Meter to Kilometer | Centimeter to Kilometer | Millimeter to Kilometer |
| Micrometer to Kilometer | Mile to Kilometer | Yard to Kilometer |
| Feet to Kilometer | Inch to Kilometer | Nanometer to Kilometer |
Length Converters to Mile (mi)
| Yard to Mile | Feet to Mile | Inch to Mile |
| Nautical Mile to Mile | Kilometer to Mile | Meter to Mile |
| Centimeter to Mile | Millimeter to Mile | Micrometer to Mile |
| Nanometer to Mile |
Conversion Factors:
- Kilometers to Miles: 1 kilometer = 0.621371 miles
- Miles to Kilometers: 1 mile = 1.60934 kilometers
How to Convert Kilometers to Miles:
To convert kilometers to miles, multiply the number of kilometers by 0.621371.
Miles=Kilometers×0.621371
Example: Convert 5 kilometers to miles.
Miles=5×0.621371=3.106855 miles
How to Convert Miles to Kilometers:
To convert miles to kilometers, multiply the number of miles by 1.60934.
Kilometers=Miles×1.60934
Example: Convert 3 miles to kilometers.
Kilometers=3×1.60934=4.82802 kilometers
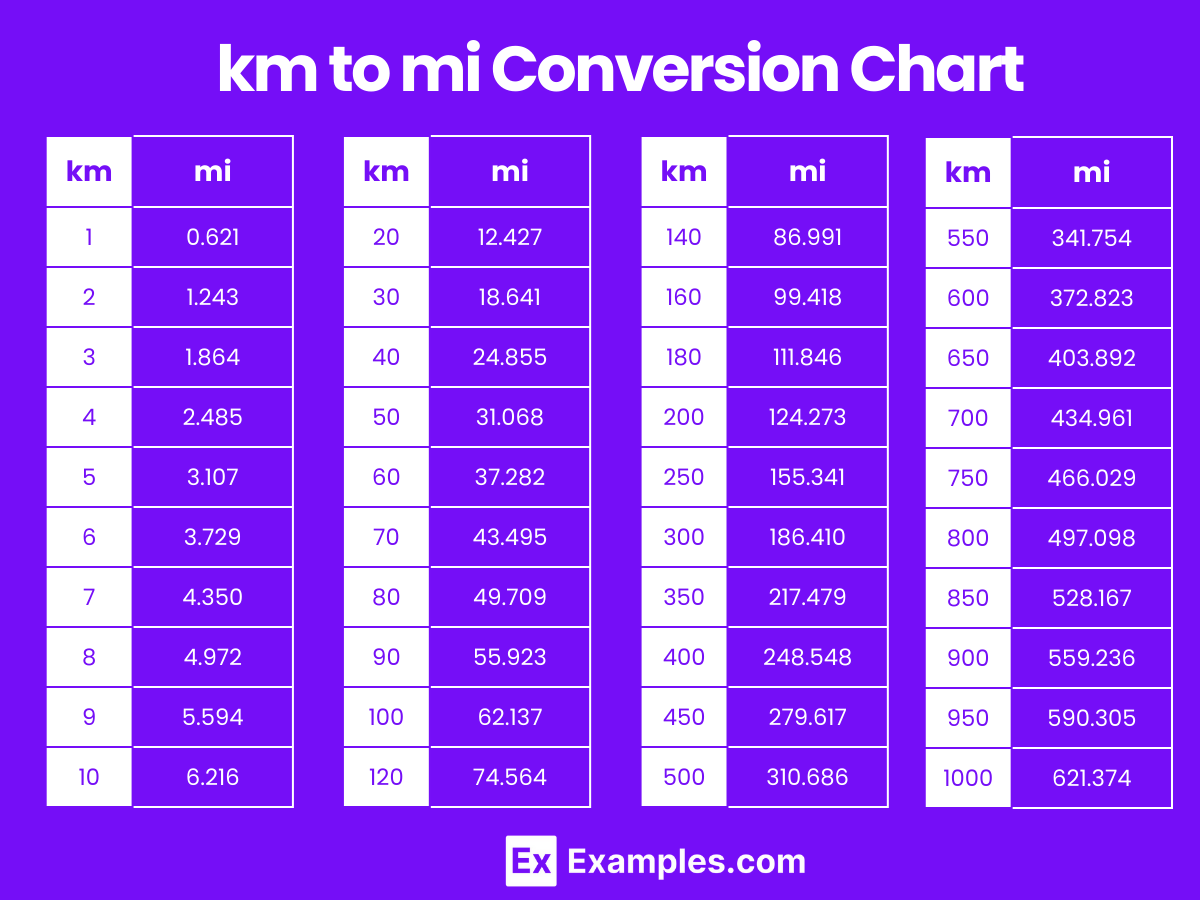
Kilometers to Miles Conversion Table
| Kilometers (km) | Miles (mi) |
|---|---|
| 1 km | 0.621 mi |
| 2 km | 1.243 mi |
| 3 km | 1.864 mi |
| 4 km | 2.485 mi |
| 5 km | 3.107 mi |
| 6 km | 3.728 mi |
| 7 km | 4.350 mi |
| 8 km | 4.971 mi |
| 9 km | 5.592 mi |
| 10 km | 6.213 mi |
| 20 km | 12.427 mi |
| 30 km | 18.641 mi |
| 40 km | 24.855 mi |
| 50 km | 31.069 mi |
| 60 km | 37.282 mi |
| 70 km | 43.496 mi |
| 80 km | 49.710 mi |
| 90 km | 55.924 mi |
| 100 km | 62.137 mi |
km to mi Conversion Chart
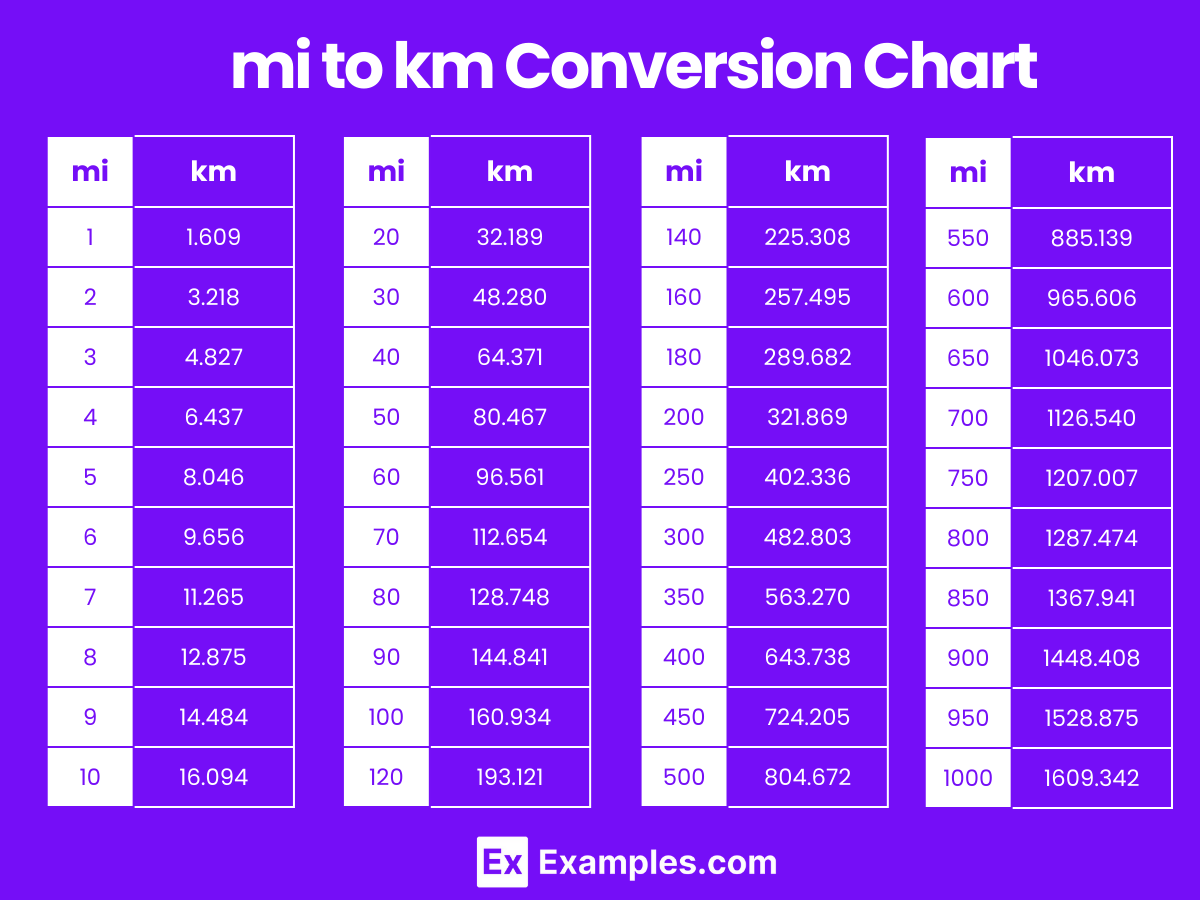
Miles to Kilometers Conversion Table
| Miles (mi) | Kilometers (km) |
|---|---|
| 1 mi | 1.609 km |
| 2 mi | 3.218 km |
| 3 mi | 4.827 km |
| 4 mi | 6.437 km |
| 5 mi | 8.046 km |
| 6 mi | 9.656 km |
| 7 mi | 11.265 km |
| 8 mi | 12.875 km |
| 9 mi | 14.484 km |
| 10 mi | 16.093 km |
| 20 mi | 32.187 km |
| 30 mi | 48.280 km |
| 40 mi | 64.374 km |
| 50 mi | 80.467 km |
| 60 mi | 96.561 km |
| 70 mi | 112.654 km |
| 80 mi | 128.748 km |
| 90 mi | 144.841 km |
| 100 mi | 160.934 km |
mi to km Conversion Chart
Difference Between Kilometers to Miles
| Aspect | Kilometers (km) | Miles (mi) |
|---|---|---|
| Unit Type | Metric system unit | Imperial system unit |
| Usage | Commonly used globally except in the USA | Primarily used in the USA, UK, and a few others |
| Conversion Factor | 1 kilometer equals approximately 0.621 miles | 1 mile equals approximately 1.609 kilometers |
| Precision | Generally used for broader, less precise measures | Often used for more precise, smaller measures |
| Contexts Used | Popular in scientific research, global travel | Common in personal travel, local distances in US |
| Decimal System | Part of the decimal-based metric system | Not based on a decimal system |
| Physical Representation | Less tangible due to larger size | More tangible and frequently visualized |
| Cultural Preference | Preferred in international and scientific contexts | Common in everyday life and literature in the US. |
1. Solved Examples on Converting Kilometers to Miles
Example 1: Convert 3 kilometers to miles.
3 km=3×0.621371 mi=1.864113 mi
Example 2: Convert 10 kilometers to miles.
10 km=10×0.621371 mi=6.21371 mi
Example 3: Convert 0.5 kilometers to miles.
0.5 km=0.5×0.621371 mi=0.310686 mi
Example 4: Convert 15 kilometers to miles.
15 km=15×0.621371 mi=9.320565 mi
Example 5: Convert 25 kilometers to miles.
25 km=25×0.621371 mi=15.534275 mi
2. Solved Examples on Converting Miles to Kilometers
Example 1: Convert 1 mile to kilometers.
1 mi=1×1.60934 km=1.60934 km
Example 2: Convert 5 miles to kilometers.
5 mi=5×1.60934 km=8.0467 km
Example 3: Convert 12 miles to kilometers.
12 mi=12×1.60934 km=19.31208 km
Example 4: Convert 0.3 miles to kilometers.
0.3 mi=0.3×1.60934 km=0.482802 km
Example 5: Convert 20 miles to kilometers.
20 mi=20×1.60934 km=32.1868 km
1. How accurate is the kilometers to miles conversion?
The conversion is quite accurate when using the standard factor of 0.621371. For most practical purposes, this level of precision is sufficient.
2. What is a common mistake to avoid when converting kilometers to miles?
A common mistake to avoid is reversing the conversion factor. Remember, the correct factor for converting kilometers to miles is 0.621371, not the other way around.
3. Can I use this conversion for scientific calculations?
Yes, the kilometers to miles conversion can be used for scientific calculations, especially in disciplines like geology and environmental science that often involve large geographic distances where both metric and imperial units are used.
4. How often do runners need to convert kilometers to miles?
Runners frequently convert kilometers to miles, especially when participating in races or using apps that track distances in a different unit than they are accustomed to.
5. How do weather reports handle kilometers and miles?
Weather reports in different countries will use the unit customary to their system; however, many online platforms provide options to display distances in either kilometers or miles.

