Contact Forces

Understanding contact forces is essential for mastering topics related to force and motion in the AP Physics exam. Contact forces occur when objects are in direct physical contact with each other. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Learning Objectives

By studying Contact Forces for the AP Physics exam, you should learn to identify and analyze different types of contact forces (normal force, friction, tension, applied force, and spring force), draw and interpret free-body diagrams, apply Newton’s laws of motion to solve problems involving contact forces, and understand the mathematical relationships governing these forces. These skills will enable you to effectively solve force and motion problems on the exam.

Definition of Contact Forces

Contact forces are forces that occur when two objects are physically touching each other. These forces arise from the interactions between the surfaces of the objects.

Types of Contact Forces

Normal Force

Definition:

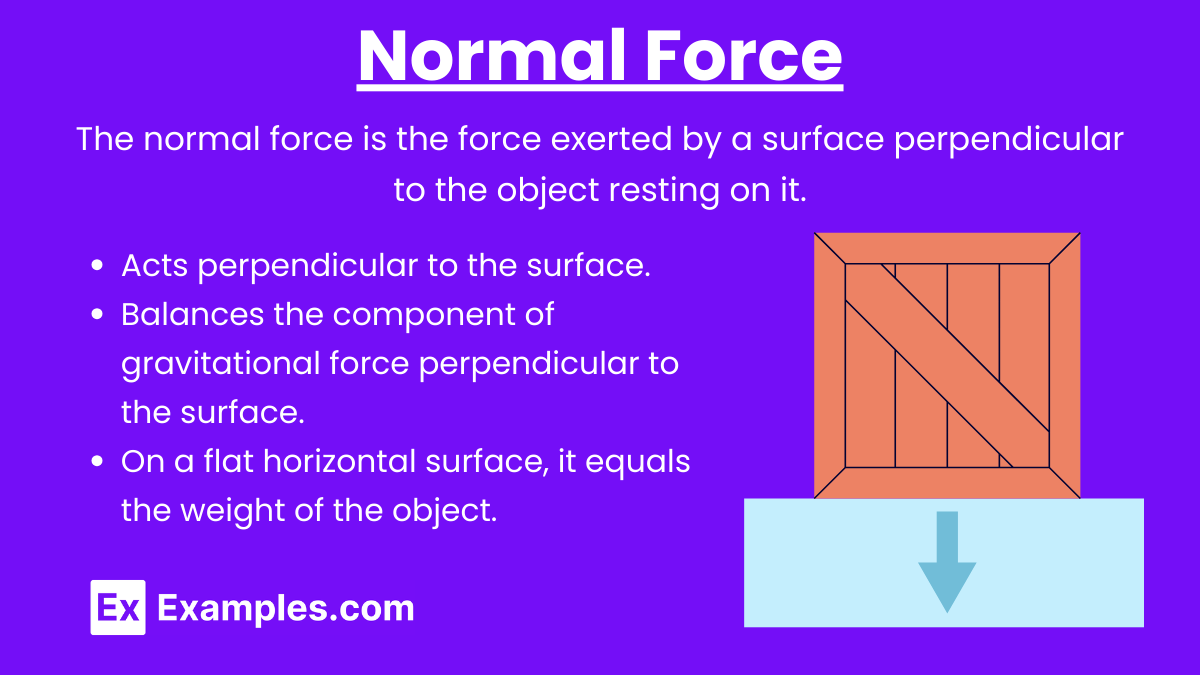
• The normal force is the force exerted by a surface perpendicular to the object resting on it.

Key Points:

• Acts perpendicular to the surface.
• Balances the component of gravitational force perpendicular to the surface.
• On a flat horizontal surface, it equals the weight of the object.

Formula:
\[ F_N = mg \cos \theta \]
where θ is the angle of the inclined surface with the horizontal.

Characteristics

• Perpendicular Direction: Always acts perpendicular to the surface.
• Reactive Force: Responds to the forces applied to the object.
• Magnitude: Depends on the weight of the object and the angle of the surface.

Importance

• Support: Prevents objects from sinking into the surface.
• Friction: The frictional force is directly proportional to the normal force.
• Equilibrium: Ensures that objects remain in equilibrium on surfaces.

Factors Affecting Normal Force

• Mass of the Object: Heavier objects exert more force on the surface, increasing the normal force.
• Surface Angle: The angle of the surface affects the distribution of forces, altering the normal force.
• External Forces: Additional forces acting on the object, such as applied forces or tension, can influence the normal force.

Friction Force

Definition:

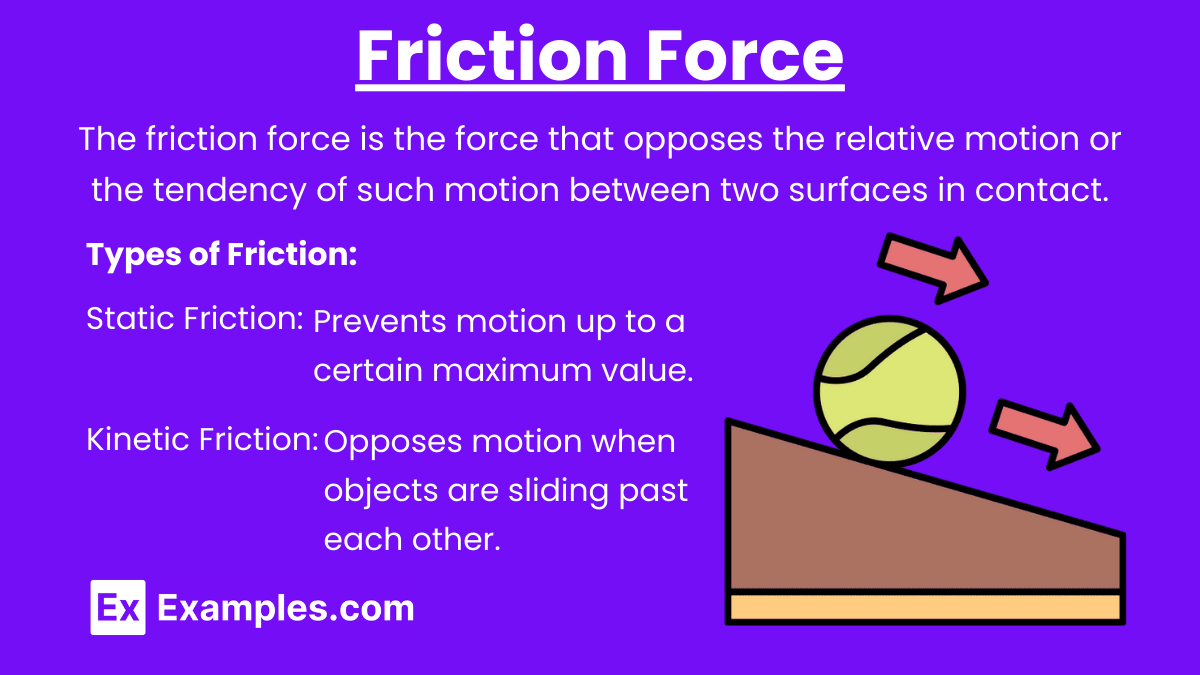
• The friction force is the force that opposes the relative motion or the tendency of such motion between two surfaces in contact.

Types of Friction:

• Static Friction: Prevents motion up to a certain maximum value.
  Formula: \[ f_s \leq \mu_s F_N \]
• Kinetic Friction: Opposes motion when objects are sliding past each other.
  Formula: \[ f_k = \mu_k F_N \]

Key Points:

• μₛ​ and μₖ are the coefficients of static and kinetic friction, respectively.

Characteristics

• Direction: Always opposes the direction of motion or intended motion.
• Magnitude: Depends on the nature of the surfaces in contact and the normal force.
• Self-Adjusting (Static Friction): Increases to match the applied force up to a maximum value.

Importance

• Motion Control: Allows walking, driving, and various other activities by providing necessary grip.
• Energy Dissipation: Converts kinetic energy into heat, leading to wear and tear.
• Stability: Helps in holding objects in place.

Factors Affecting Friction Force

• Nature of Surfaces: Rough surfaces have higher friction than smooth surfaces.
• Normal Force: Greater normal force increases friction.
• Type of Friction: Static friction is usually greater than kinetic friction for the same pair of surfaces.

Tension Force

Definition:

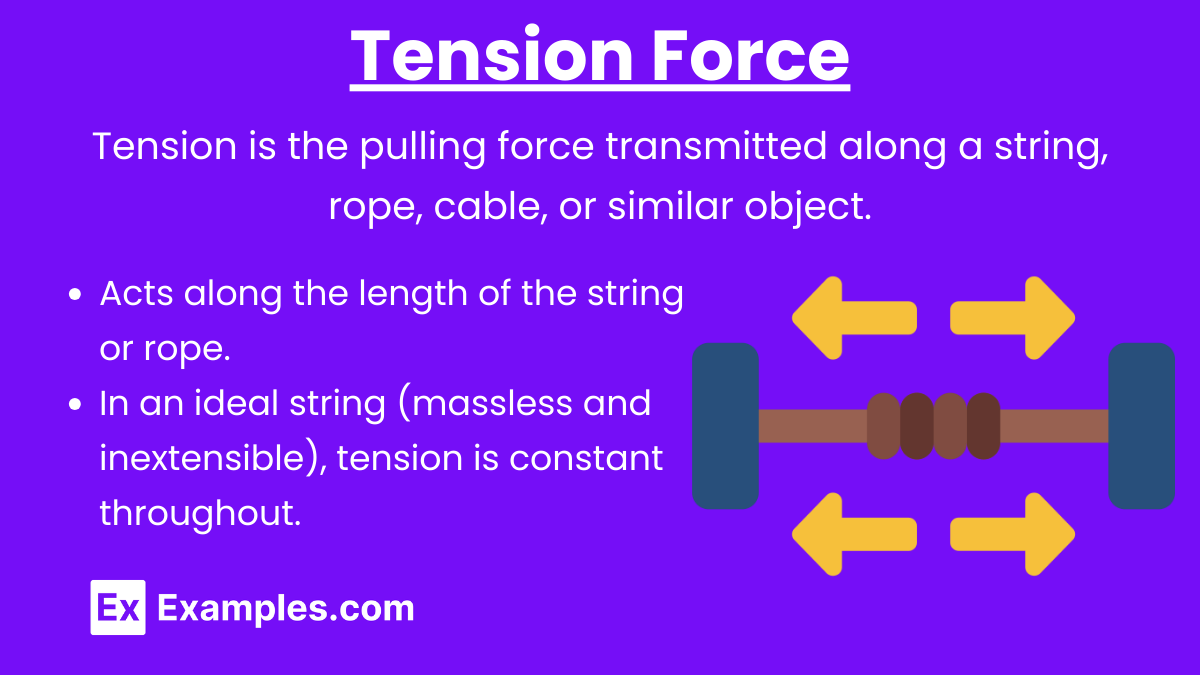
• Tension is the pulling force transmitted along a string, rope, cable, or similar object.

Key Points:

• Acts along the length of the string or rope.
• In an ideal string (massless and inextensible), tension is constant throughout.

Characteristics

• Directed along the Connector: Always acts along the length of the string, rope, or cable.
• Pulling Force: Transmits force by pulling the connected objects towards each other.
• Equal and Opposite: Acts equally and in opposite directions on the objects at both ends of the connector.

Importance

• Mechanical Systems: Essential in analyzing systems involving ropes, cables, and pulleys.
• Structural Integrity: Critical in determining the strength and stability of structures like bridges and elevators.
• Motion and Equilibrium: Plays a key role in problems involving motion, especially in systems with multiple objects.

Factors Affecting Tension Force

• Mass of the Objects: Heavier objects exert greater tension in the connecting rope.
• Angle of Inclination: The angle at which the force is applied affects the tension.
• External Forces: Additional forces such as applied forces or friction can influence the tension in the connector.

Applied Force

Definition:

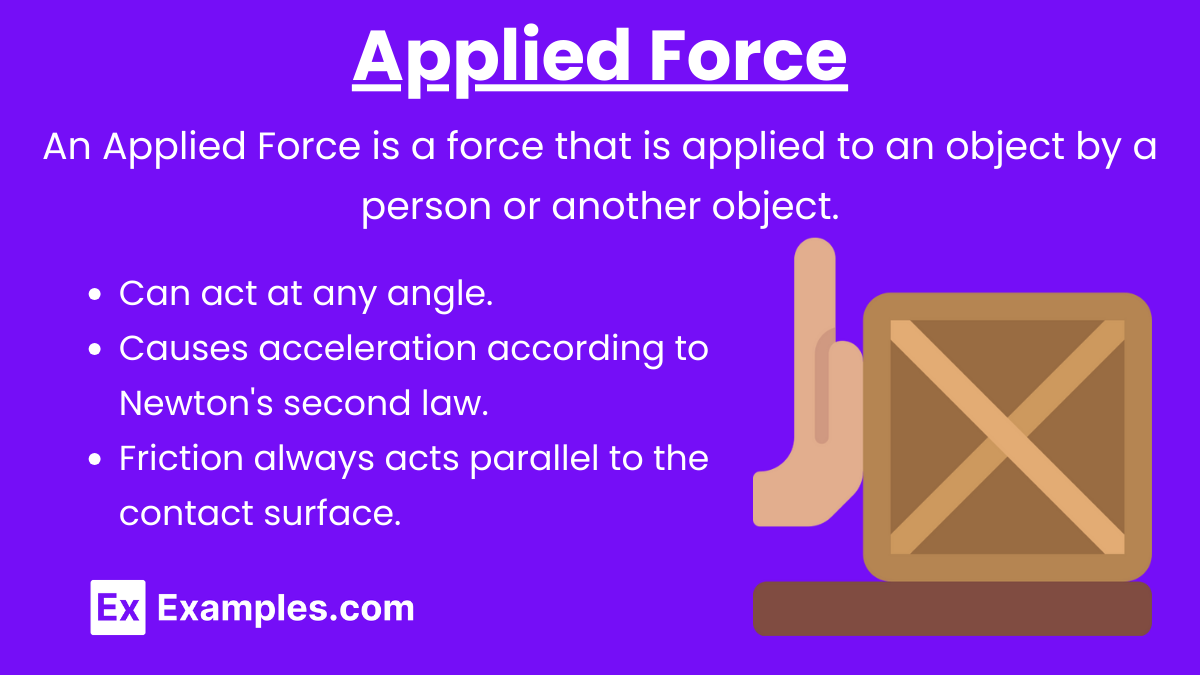
• An applied force is a force that is applied to an object by a person or another object.

Key Points:

• Can act at any angle.
• Causes acceleration according to Newton’s second law.
• Friction always acts parallel to the contact surface.

Characteristics

• Direction: Acts in the direction specified by the force application.
• Magnitude: Depends on how strong the force is applied.
• Contact Force: Requires physical contact between the source and the object.

Importance

• Movement: Causes objects to move or change their velocity.
• Direction Change: Can alter the direction of an object’s motion.
• Work and Energy: Applied force does work on the object, transferring energy.

Factors Affecting Applied Force

• Source of Force: The strength and direction of the source applying the force.
• Object’s Mass: Heavier objects require more force to achieve the same acceleration.
• Friction: The presence of friction can affect the net force and the object’s motion.

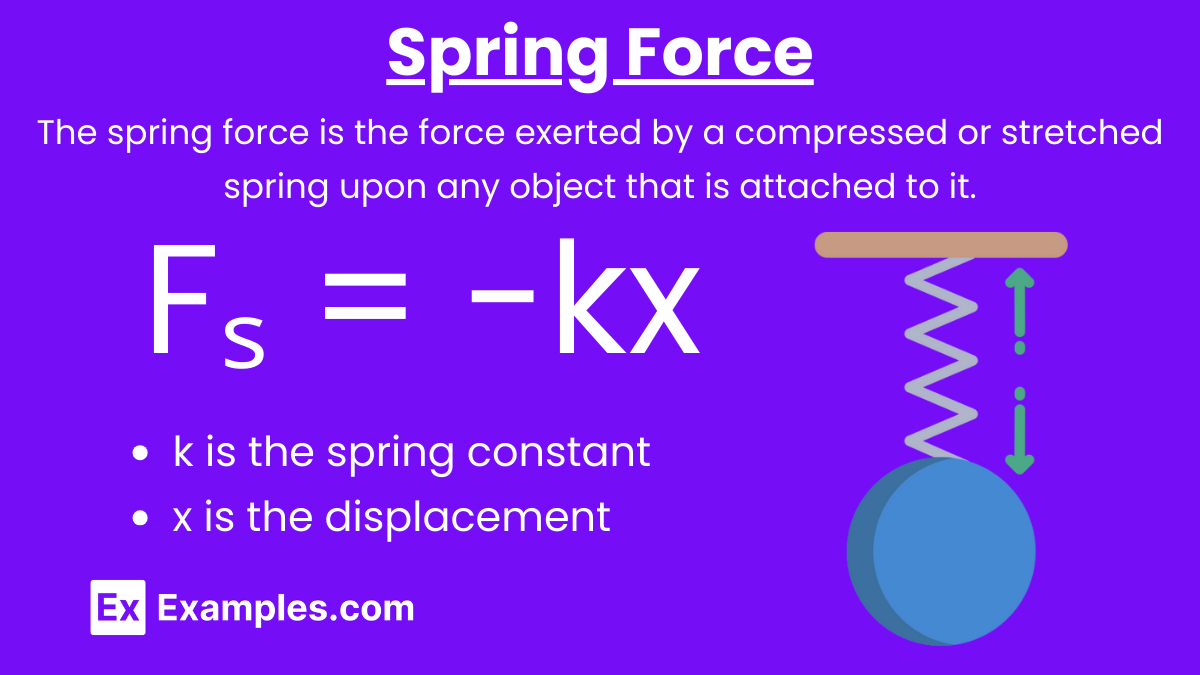
Spring Force

Definition:

• The spring force is the force exerted by a compressed or stretched spring upon any object that is attached to it.

Hooke’s Law: Fₛ = -kx
where k is the spring constant and x is the displacement from the equilibrium position.

Key Points:

• Acts in the opposite direction to the displacement.
• Restoring force that brings the object back to equilibrium.

Characteristics

• Proportional to Displacement: The force increases linearly with the increase in displacement.
• Restorative Nature: Acts to return the spring to its natural length.
• Dependent on Spring Constant: The stiffness of the spring, determined by the spring constant kkk, affects the magnitude of the force.

Importance

• Energy Storage: Springs can store potential energy when compressed or stretched.
• Mechanical Applications: Used in various mechanical devices such as suspension systems, clocks, and measuring instruments.
• Vibration Damping: Springs help in absorbing and dissipating energy in systems subject to vibrations.

Factors Affecting Spring Force

• Spring Constant k: A higher spring constant means a stiffer spring and a larger force for a given displacement.
• Displacement x: Greater displacement results in a larger force.

Analyzing Contact Forces

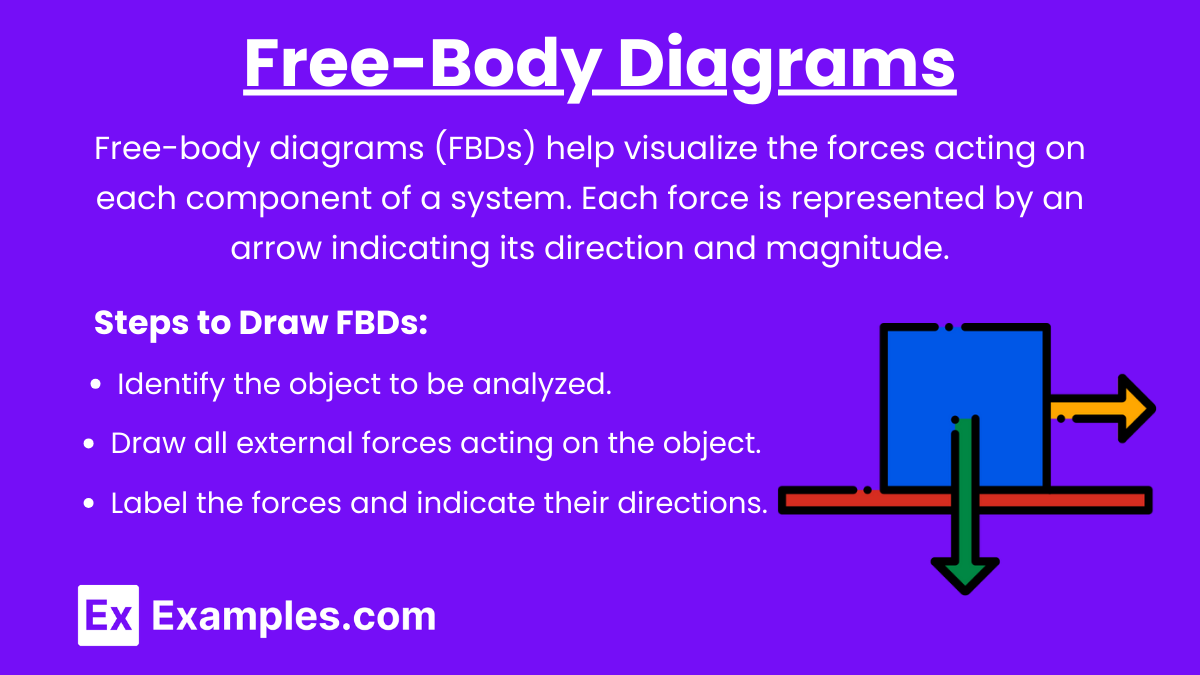
Free-Body Diagrams (FBDs)

Key Points:

• A free-body diagram is a graphical representation of the forces acting on an object.
• Each force is represented by an arrow indicating its direction and magnitude.
• Helps in setting up equations to solve for unknowns.

Steps to Draw FBDs:

1. Identify the object to be analyzed.
2. Draw all exaternal forces acting on the object
3. Label the forces and indicate their directions

Examples

Example 1: Block on an Inclined Plane

Scenario:

• A block of mass m rests on an inclined plane making an angle θ with the horizontal.

Forces Acting:

• Gravitational force: mg
• Normal force: \[ F_N = mg \cos \theta \]
• Frictional force: \[ f = \mu F_N \]

Equations:

• Parallel to the plane: \[ F_{\parallel} = mg \sin \theta – f = ma \]
• Perpendicular to the plane: \[ F_N = mg \cos \theta \]

Example 2: Two Blocks Connected by a String

Scenario:

• Two blocks of masses m​₁ and m₂ are connected by a string over a pulley.

Forces Acting:

• Gravitational force on m₁​: m₁g
• Gravitational force on m₂​: m₂​g
• Tension in the string: T

Equations:

• For m₁ ​: T−m₁​g = m₁​a
• For m₂ ​: m₂​g−T = m₂​a

Example 3: Spring-Mass System

Scenario:

• A mass m attached to a spring with spring constant k is displaced by x.

Forces Acting:

• Spring force: Fₛ ​= −kx

Equations:

• Newton’s second law: −kx=ma
• Acceleration: \[ \mathbf{a} = -\frac{k}{m} \mathbf{x} \]