Free-Body Diagrams for Objects in Uniform Circular Motion

Understanding free-body diagrams (FBDs) for objects in uniform circular motion is essential for mastering the principles of dynamics and circular motion in the AP Physics exam. These diagrams help visualize the forces acting on an object moving in a circular path with constant speed. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

In studying free-body diagrams for objects in uniform circular motion for the AP Physics exam, you will learn to identify and represent all forces acting on an object moving in a circular path. This includes understanding the role of centripetal force, recognizing different force vectors, and accurately depicting tension, friction, and gravitational forces in these scenarios. Mastery of these concepts will enable you to solve problems related to circular motion dynamics and understand the principles of forces and motion in rotational contexts.

Uniform Circular Motion

Definition: Uniform circular motion occurs when an object moves in a circular path with constant speed. Even though the speed is constant, the direction of the velocity changes continuously, resulting in an acceleration toward the center of the circle, known as centripetal acceleration.

Centripetal Acceleration (ac​): a_c = \frac{v^2}{r}

where:

• v is the tangential speed of the object,

• r is the radius of the circular path.

Key Forces in Uniform Circular Motion

1. Centripetal Force: The net force causing centripetal acceleration, always directed toward the center of the circular path.

2. Tension: Force exerted by a string or rope pulling the object toward the center.

3. Gravitational Force: Force exerted by the Earth pulling the object downward.

4. Normal Force: Force exerted by a surface perpendicular to the object.

5. Friction: Force opposing the relative motion between surfaces in contact, can act as centripetal force.

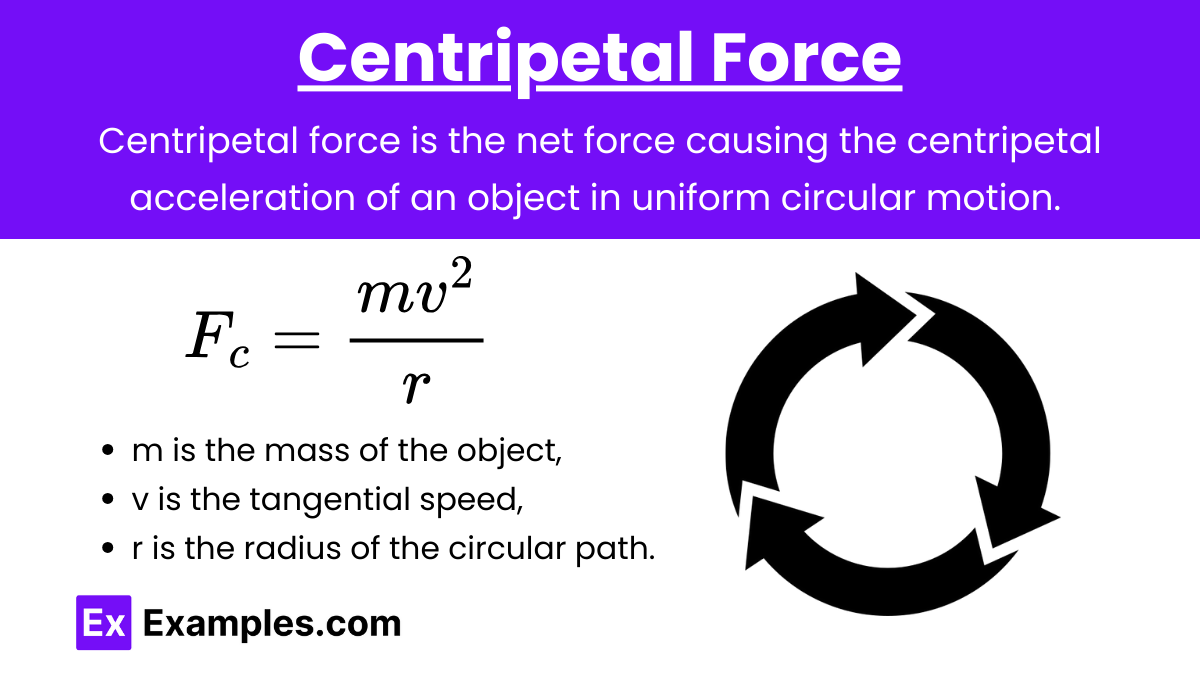
Centripetal Force

Definition: Centripetal force is the net force causing the centripetal acceleration of an object in uniform circular motion. It always points towards the center of the circular path.

Formula: F_c = \frac{mv^2}{r}

where:

• m is the mass of the object,

• v is the tangential speed,

• r is the radius of the circular path.

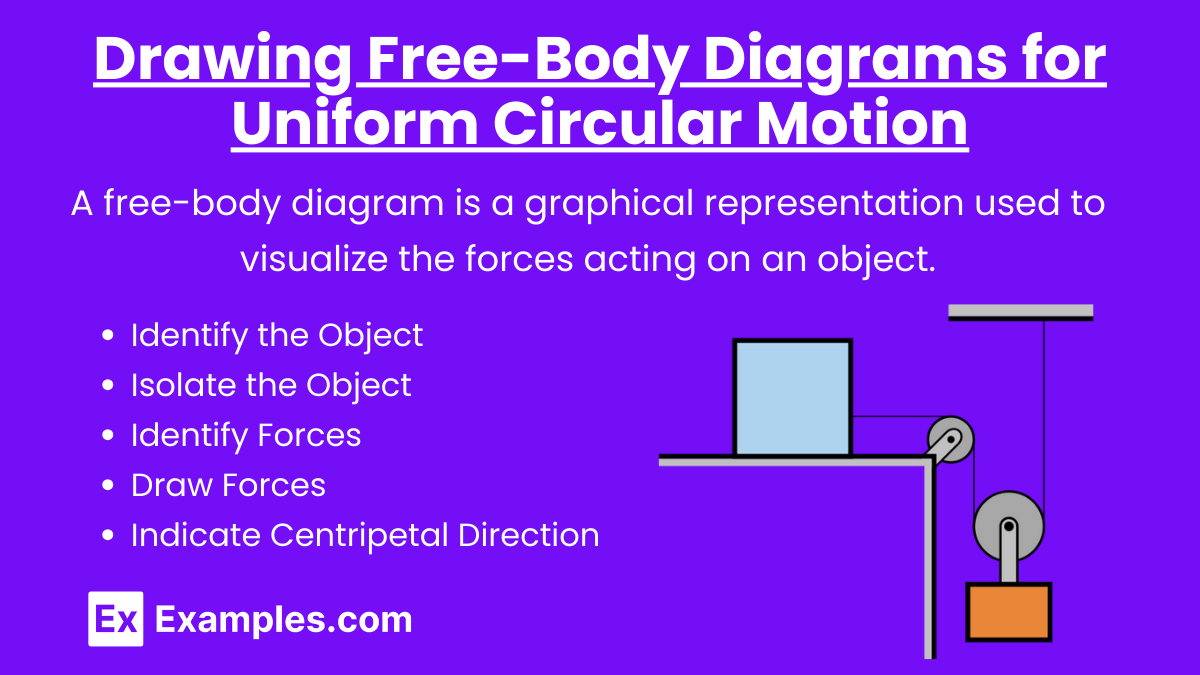
Drawing Free-Body Diagrams for Uniform Circular Motion

Free-Body Diagram (FBD): A free-body diagram is a graphical representation used to visualize the forces acting on an object. Each force is represented by an arrow pointing in the direction of the force, with the length proportional to the force's magnitude.

Steps to Draw FBDs for Circular Motion

1. Identify the Object:

   • Select the object for which the FBD is to be drawn.

2. Isolate the Object:

   • Represent the object as a dot or a simple shape.

3. Identify Forces:

   • Identify all the forces acting on the object.

4. Draw Forces:

   • Draw arrows for each force acting on the object, originating from the center of the object.

5. Indicate Centripetal Direction:

   • Indicate the direction of the centripetal force, which points towards the center of the circular path.

Common Forces in Circular Motion

• Gravitational Force (Fg​): The force of attraction between the object and the Earth.

• Normal Force (Fₙ​): The perpendicular contact force exerted by a surface on the object.

• Frictional Force (f): The force that opposes relative motion between two surfaces in contact.

• Tension Force (T): The pulling force transmitted through a string, rope, or cable.

• Centripetal Force (F꜀): The net force causing centripetal acceleration, pointing towards the center of the circular path.

Examples of Free-Body Diagrams for Circular Motion

Example 1: Object in Horizontal Circular Motion (e.g., Car on a Flat Curve)

Scenario: A car of mass m is moving in a circular path of radius r with a constant speed v on a flat horizontal road.

Forces Acting:

• Gravitational Force (Fg​=mg): Acts downward.

• Normal Force (Fₙ): Acts upward, balancing the gravitational force.

• Frictional Force (f): Provides the centripetal force, acting horizontally towards the center of the circle.

Equations: F_N = mg
f = F_c = \frac{mv^2}{r}

Example 2: Object in Vertical Circular Motion (e.g., Object in a Vertical Loop)

Scenario: An object of mass m is moving in a vertical circular loop of radius r with a constant speed v.

Forces Acting at the Top of the Loop:

• Gravitational Force (Fg​=mg): Acts downward.

• Normal Force (Fₙ): Acts downward (towards the center of the loop).

Forces Acting at the Bottom of the Loop:

• Gravitational Force (Fg​=mg): Acts downward.

• Normal Force (Fₙ): Acts upward (away from the center of the loop).

Equations at the Top of the Loop: F_c = F_N + F_g = \frac{mv^2}{r}

Equations at the Bottom of the Loop: F_c = F_N - F_g = \frac{mv^2}{r}

Example 3: Object in Circular Motion on a Banked Curve

Scenario: A car of mass m is moving on a banked curve of radius r with an angle of banking θ at a constant speed v.

Forces Acting:

• Gravitational Force (Fg​=mg): Acts downward.

• Normal Force (Fₙ​): Acts perpendicular to the surface.

• Frictional Force (f): Acts parallel to the surface, providing additional centripetal force.

Equations:

• Components of Normal Force: F_N \cos \theta = mg
  F_N \sin \theta = F_c = \frac{mv^2}{r}

• If friction is present: f = μFₙ