Gravitational Field/Acceleration due to gravity on different planets

Last Updated: November 7, 2024

Notes

Understanding the gravitational field and the acceleration due to gravity on different planets is crucial for mastering concepts related to gravity and motion in the AP Physics exam. This topic involves the concept of gravitational fields, how they vary on different planets, and the implications of these variations. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

In this topic, you will learn to calculate the gravitational field strength and acceleration due to gravity on different planets. You will understand how mass and radius affect gravity, compare gravitational acceleration on various celestial bodies, and apply Newton’s Law of Universal Gravitation. This knowledge will help you analyze gravitational forces in different planetary environments and solve related problems, preparing you for questions on the AP Physics exam.

Gravitational Field

Gravitational Field: A gravitational field is a region of space surrounding a mass where another mass experiences a force of gravitational attraction. It is a vector field, meaning it has both magnitude and direction.

Gravitational Field Strength

Gravitational Field Strength (g): The gravitational field strength at a point in space is defined as the gravitational force (F) experienced by a unit mass (m) placed at that point. It is given by:

$\mathbf{g} = \frac{\mathbf{F}}{m}$

Units: The units of gravitational field strength are newtons per kilogram (N/kg).

Direction: The direction of the gravitational field is always towards the mass that is creating the field.

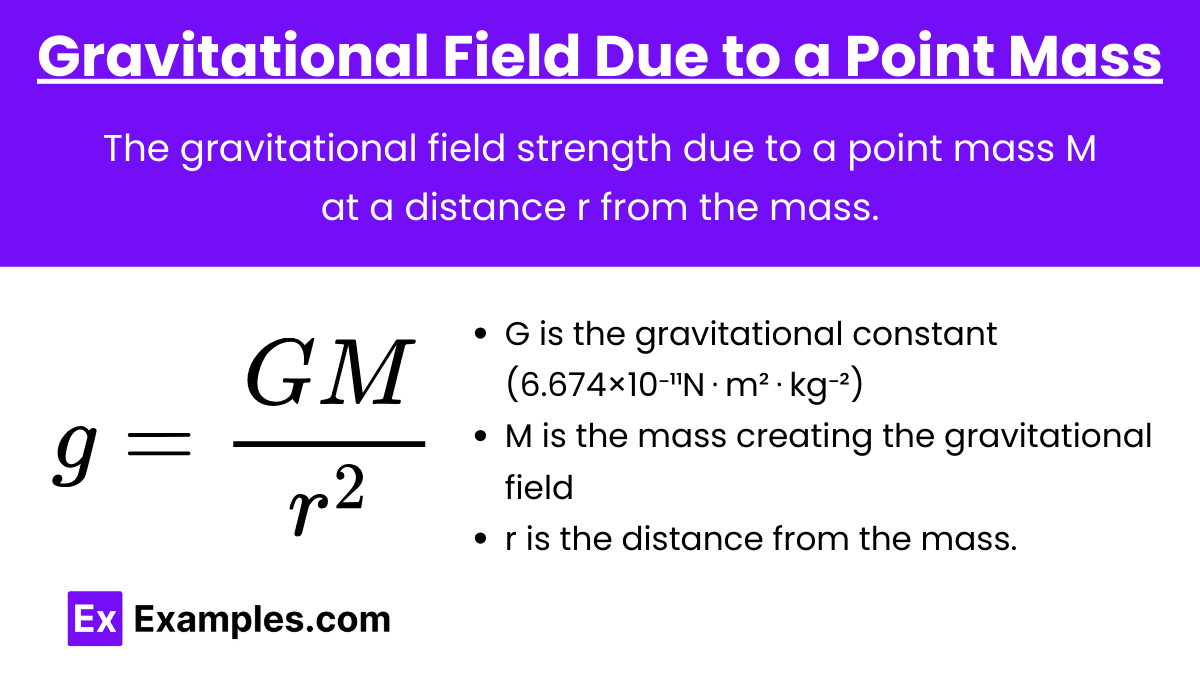
Gravitational Field Due to a Point Mass

Formula: The gravitational field strength due to a point mass M at a distance r from the mass is given by:

$F_g = \frac{G m_1 m_2}{r^2}$

where:

G is the gravitational constant (6.674×10⁻¹¹N⋅m²⋅kg⁻²)
M is the mass creating the gravitational field
r is the distance from the mass

Key Points:

The gravitational field strength decreases with the square of the distance from the mass.
The field is radially symmetric around the point mass.

Gravitational Field of Spherical Bodies

For a spherical body like a planet, the gravitational field at a distance r from its center (where r is greater than or equal to the planet’s radius R) can be treated as if all the mass were concentrated at the center.

$g = \frac{GM}{R^2}$

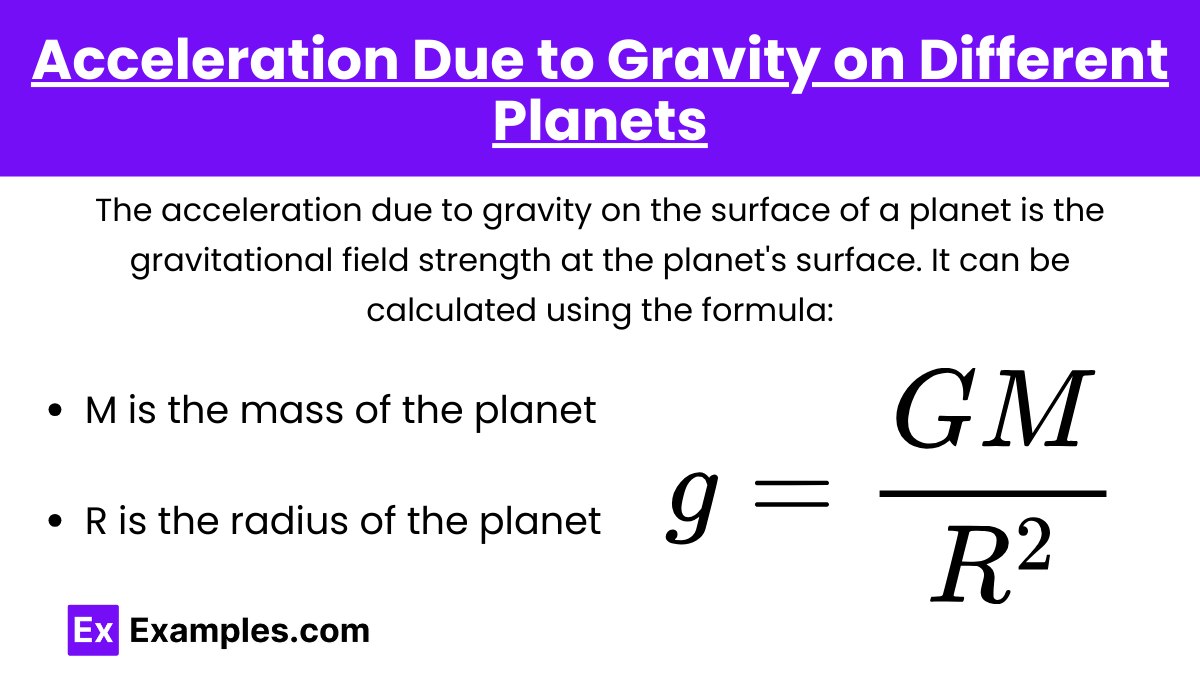
Acceleration Due to Gravity on Different Planets

Acceleration Due to Gravity (g): The acceleration due to gravity on the surface of a planet is the gravitational field strength at the planet’s surface. It can be calculated using the formula:

$g = \frac{GM}{R^2}$

where:

M is the mass of the planet
R is the radius of the planet

Factors Affecting Gravitational Acceleration

Mass of the Planet: Greater mass results in a stronger gravitational field and higher acceleration due to gravity.
Radius of the Planet: A larger radius results in a weaker gravitational field and lower acceleration due to gravity.

Gravitational Acceleration on Different Planets

Here are the gravitational accelerations on some planets in our solar system:

Earth:
Mass (Mₑₐᵣₜₕ): 5.972×10²⁴kg
Radius (Rₑₐᵣₜₕ): 6.371×10⁶m
Gravitational acceleration (g): 9.8m/s²

Moon:
Mass (Mₘₒₒₙ): 7.347×10²²kg
Radius (Rₘₒₒₙ): 1.737×10⁶m
Gravitational acceleration (g): 1.62m/s²

Mars:
Mass (Mₘₐᵣₛ): 6.417×10²³kg
Radius (Rₘₐᵣₛ): 3.390×10⁶m
Gravitational acceleration (g): 3.71m/s²

Jupiter:
Mass (Mⱼᵤₚᵢₜₑᵣ): 1.898×10²⁷kg
Radius (Rⱼᵤₚᵢₜₑᵣ): 6.991×10⁷m
Gravitational acceleration (g): 24.79m/s²

Examples and Problems

Example 1: Calculating Gravitational Field Strength

Scenario: Calculate the gravitational field strength at a distance of 10⁷m from a planet with a mass of 5×10²⁴kg.

Solution:

$\begin{aligned} g &= \frac{GM}{r^2} \\ g &= \frac{6.674 \times 10^{-11} \times 5 \times 10^{24}}{(10^7)^2} \\ g &= \frac{3.337 \times 10^{14}}{10^{14}} = 3.337 \, \text{N/kg} \end{aligned}$

Example 2: Gravitational Acceleration on a New Planet

Scenario: A newly discovered planet has a mass of 8×10²³kg and a radius of 2×10⁶m. Calculate the gravitational acceleration on the surface of this planet.

Solution:

$\begin{aligned} g &= \frac{GM}{R^2} \\ g &= \frac{6.674 \times 10^{-11} \times 8 \times 10^{23}}{(2 \times 10^6)^2} \\ g &= \frac{5.3392 \times 10^{13}}{4 \times 10^{12}} = 13.35 \, \text{m/s}^2 \end{aligned}$

Example 3: Weight on Different Planets

Scenario: An object has a mass of 10 kg. Calculate its weight on Earth, the Moon, and Jupiter.

Solution:

Weight on Earth: Wₑₐᵣₜₕ = mg = 10kg×9.8m/s² = 98N
Weight on the Moon: Wₘₒₒₙ = mg = 10kg×1.62m/s² = 16.2N
Weight on Jupiter: Wⱼᵤₚᵢₜₑᵣ = mg = 10kg×24.79m/s² = 247.9N