Inertial vs. Gravitational Mass

Understanding the concepts of inertial and gravitational mass is crucial for mastering the principles of mechanics and gravity, which are essential for the AP Physics exam. These two types of mass are fundamental to Newtonian mechanics and general relativity. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

Understand the distinction between inertial and gravitational mass. Learn how inertial mass quantifies an object's resistance to acceleration under an applied force, while gravitational mass measures the force an object experiences in a gravitational field. Explore how these concepts are experimentally equivalent due to the principle of equivalence. Apply these principles to solve problems involving Newton's laws of motion and gravitational interactions, crucial for the AP Physics exam.

Inertial Mass

Inertial Mass: Inertial mass is a measure of an object's resistance to any change in its state of motion when a force is applied. It quantifies how much an object resists acceleration when subjected to a force.

Newton’s Second Law: F = mᵢa

where:

• F is the applied force,

• mᵢ​ is the inertial mass,

• a is the acceleration.

Key Points:

• Inertial mass determines the amount of acceleration an object experiences for a given force.

• It is measured using the response of an object to a known force.

Gravitational Mass

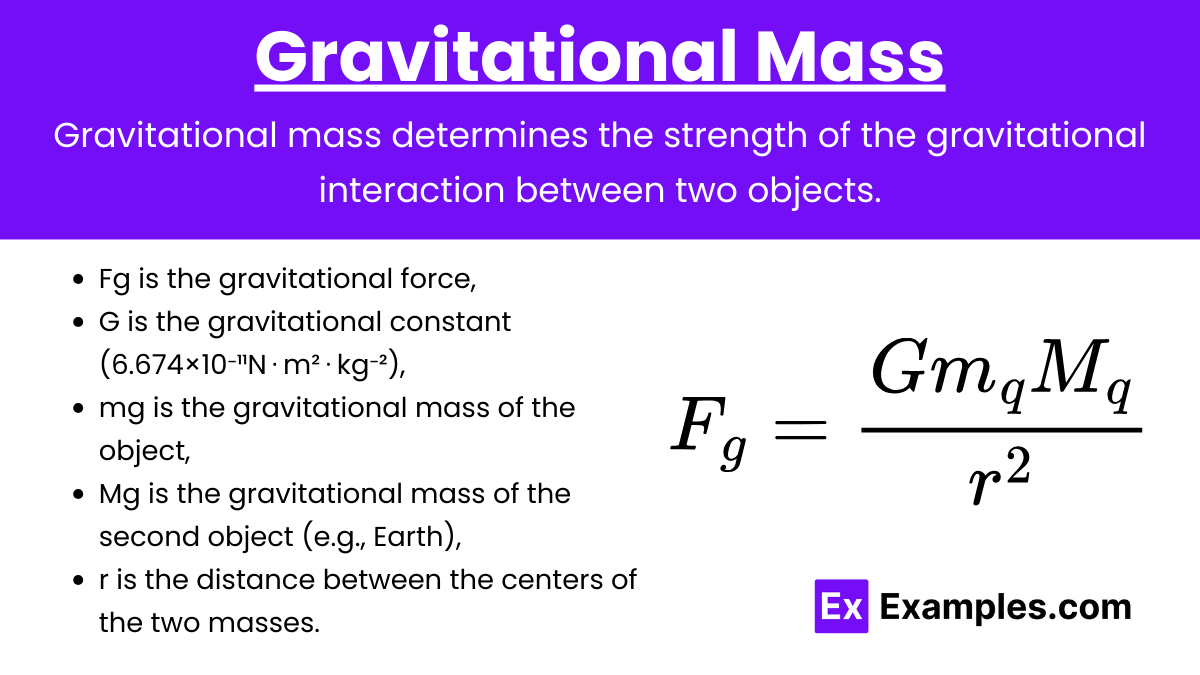
Gravitational Mass: Gravitational mass determines the strength of the gravitational interaction between two objects. It quantifies the amount of gravitational force an object experiences in a gravitational field.

Newton’s Law of Universal Gravitation: F_g = \frac{G m_q M_q}{r^2}

where:

• Fg​ is the gravitational force,

• G is the gravitational constant (6.674×10⁻¹¹N⋅m²⋅kg⁻²),

• mg​ is the gravitational mass of the object,

• Mg​ is the gravitational mass of the second object (e.g., Earth),

• r is the distance between the centers of the two masses.

Key Points:

• Gravitational mass determines the gravitational force an object experiences.

• It is measured using the gravitational interaction between objects.

Equivalence Principle

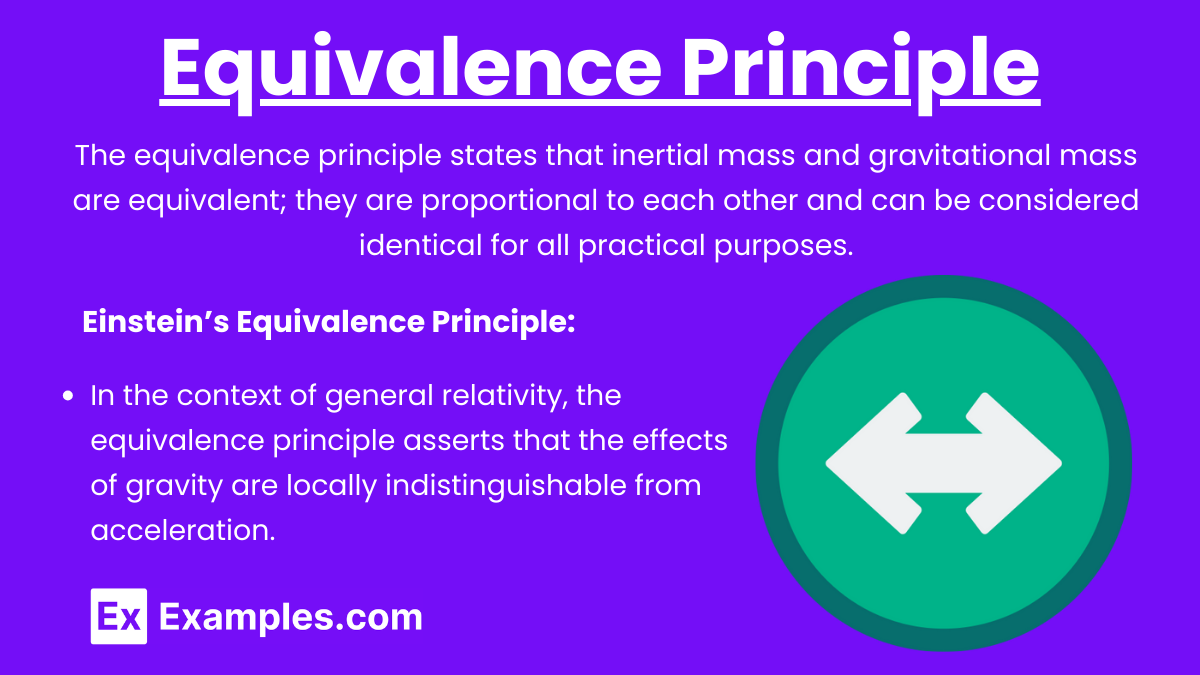
Equivalence Principle: The equivalence principle states that inertial mass and gravitational mass are equivalent; they are proportional to each other and can be considered identical for all practical purposes.

Einstein’s Equivalence Principle:

• In the context of general relativity, the equivalence principle asserts that the effects of gravity are locally indistinguishable from acceleration.

Key Points:

• All objects fall at the same rate in a given gravitational field, regardless of their masses, due to the equivalence of inertial and gravitational mass.

• This principle is fundamental to the theory of general relativity.

Measuring Inertial and Gravitational Mass

Measuring Inertial Mass

Method:

1. Apply a known force to the object.

2. Measure the acceleration of the object.

3. Calculate the inertial mass using Newton’s second law.

Example: If a force of 10 N is applied to an object and it accelerates at 2m/s², the inertial mass is: m_i = \frac{F}{a} = \frac{10 \, \text{N}}{2 \, \text{m/s}^2} = 5 \, \text{kg}

Measuring Gravitational Mass

Method:

1. Measure the gravitational force acting on the object in a known gravitational field (e.g., Earth's gravity).

2. Use the formula for gravitational force to calculate the gravitational mass.

Example: If an object weighs 49 N on Earth, where the gravitational field strength is 9.8m/s², the gravitational mass is: m_g = \frac{F_g}{g} = \frac{49 \, \text{N}}{9.8 \, \text{m/s}^2} = 5 \, \text{kg}

Applications and Implications

Newtonian Mechanics

• In Newtonian mechanics, inertial mass and gravitational mass are treated as equivalent. This equivalence simplifies the formulation of mechanics and gravitational theory.

• The equivalence allows the use of the same mass symbol (m) in both F=ma and F_g = \frac{GmM}{r^2}

General Relativity

• In general relativity, the equivalence principle implies that the motion of an object in a gravitational field is independent of its mass and composition.

• This principle leads to the concept that gravity is a curvature of spacetime caused by mass and energy.

Example 1

Comparing Inertial and Gravitational Mass

Scenario: An object has an inertial mass of 10 kg. Calculate its weight on Earth and determine its gravitational mass.

Solution: F_g = m_g g F_g = 10 \, \text{kg} \times 9.8 \, \text{m/s}^2 = 98 \, \text{N} \text{Since } F_g = 98 \, \text{N} \text{ and the gravitational field strength } g = 9.8 \, \text{m/s}^2, \text{ the gravitational mass } m_g \text{ is:} m_g = \frac{F_g}{g} = \frac{98 \, \text{N}}{9.8 \, \text{m/s}^2} = 10 \, \text{kg}

Example 2

Equivalence Principle in Free Fall

Scenario: Two objects, one with a mass of 1 kg and another with a mass of 2 kg, are dropped from the same height in a vacuum. Demonstrate that they fall at the same rate.

Explanation: Both objects experience the same gravitational field strength g=9.8m/s². According to the equivalence principle, their inertial and gravitational masses are equivalent, so they experience the same acceleration regardless of their mass.

Example 3

Calculating Gravitational Force

Scenario: Calculate the gravitational force between two 5 kg masses separated by a distance of 1 meter.

Solution: F_g = \frac{G m_1 m_2}{r^2} F_g = \frac{6.674 \times 10^{-11} \times 5 \times 5}{1^2} F_g = 1.6685 \times 10^{-9} \, \text{N}