Understanding Newton's First Law of Motion is fundamental for mastering the principles of dynamics in the AP Physics exam. This law, also known as the Law of Inertia, explains how objects behave when forces are balanced or absent. Below are detailed notes to help you achieve a high score on your AP Physics exam.
Free AP Physics 1: Algebra-Based Practice Test
Learning Objectives
By studying Newton’s First Law for the AP Physics exam, you should learn to define and explain inertia and its relationship with mass, understand the conditions for equilibrium, distinguish between static and dynamic equilibrium, and recognize balanced and unbalanced forces. You should also master drawing and interpreting free-body diagrams to visualize forces acting on objects. These skills will enable you to apply Newton’s First Law to solve problems involving motion and forces effectively, preparing you for various dynamics questions on the exam.
Definition of Newton’s First Law
Newton’s First Law of Motion: An object at rest stays at rest, and an object in motion continues in motion with the same speed and in the same direction unless acted upon by an unbalanced external force.
Inertia
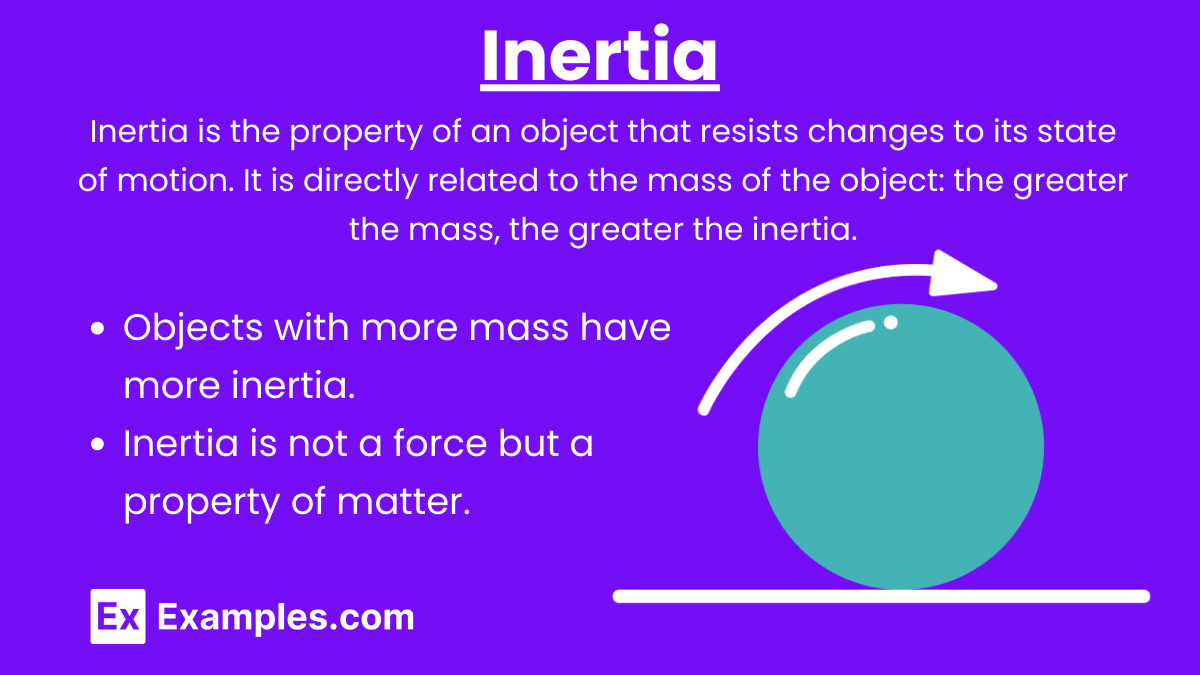
Inertia: Inertia is the property of an object that resists changes to its state of motion. It is directly related to the mass of the object: the greater the mass, the greater the inertia.
Key Points:
Objects with more mass have more inertia.
Inertia is not a force but a property of matter.
Equilibrium
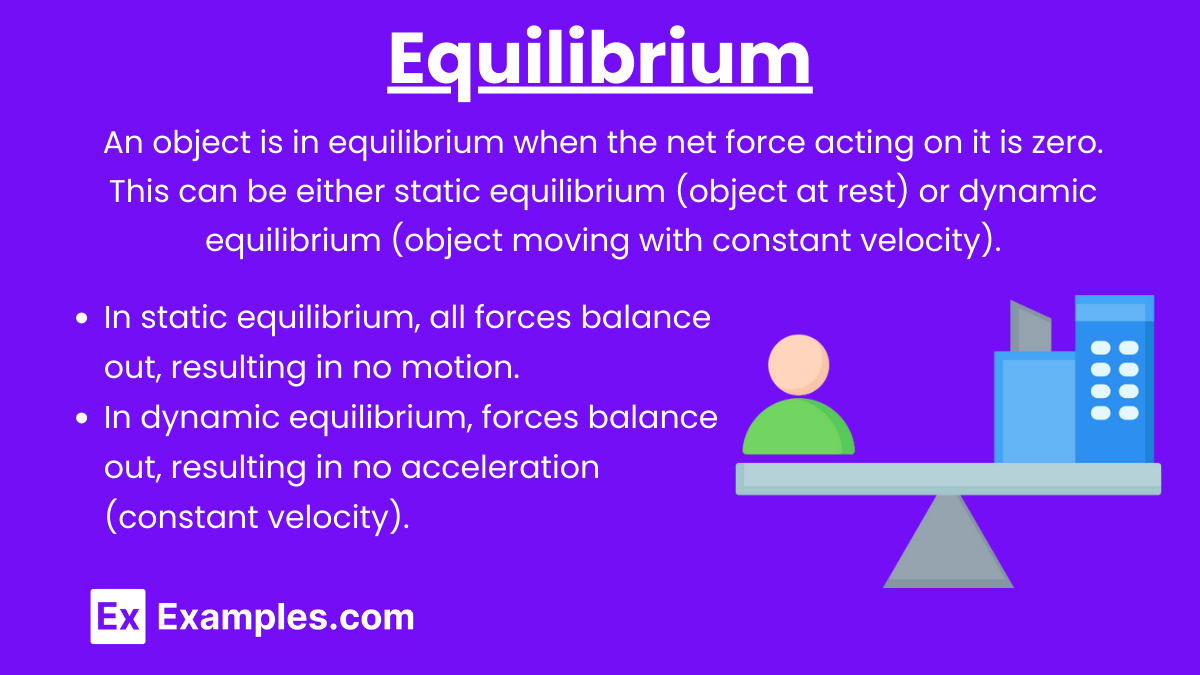
Equilibrium: An object is in equilibrium when the net force acting on it is zero. This can be either static equilibrium (object at rest) or dynamic equilibrium (object moving with constant velocity).
Key Points:
In static equilibrium, all forces balance out, resulting in no motion.
In dynamic equilibrium, forces balance out, resulting in no acceleration (constant velocity).
Applications of Newton’s First Law
Everyday Examples
A book on a table:
The book remains at rest because the forces acting on it (gravity and the normal force from the table) are balanced.
A passenger in a car:
When the car suddenly stops, the passenger continues to move forward due to inertia, illustrating the tendency to maintain motion.
Free-Body Diagrams
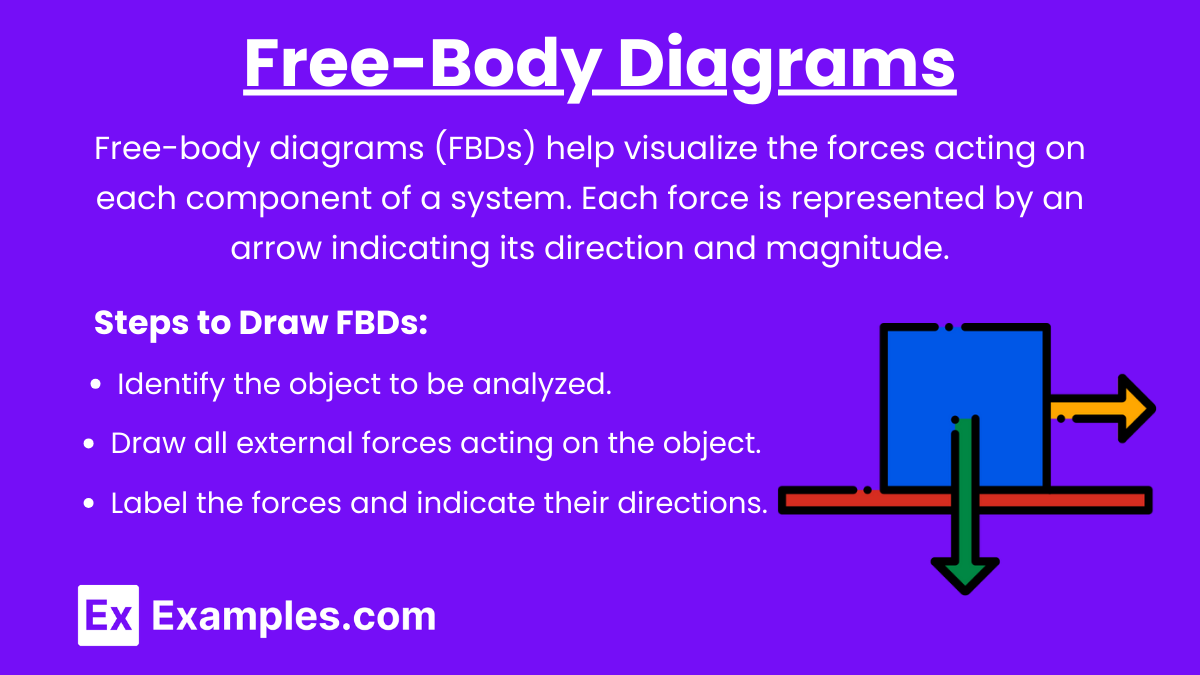
Free-Body Diagram (FBD): A free-body diagram is a graphical representation used to visualize the forces acting on an object. Each force is represented by an arrow pointing in the direction of the force.
Steps to Draw FBDs:
Identify the object to be analyzed.
Draw al, exaternal forces acting on the object.
Label each force and indicate their directions (e.g., Fg for gravitational force, Fₙ for normal force).
Forces Acting on an Object
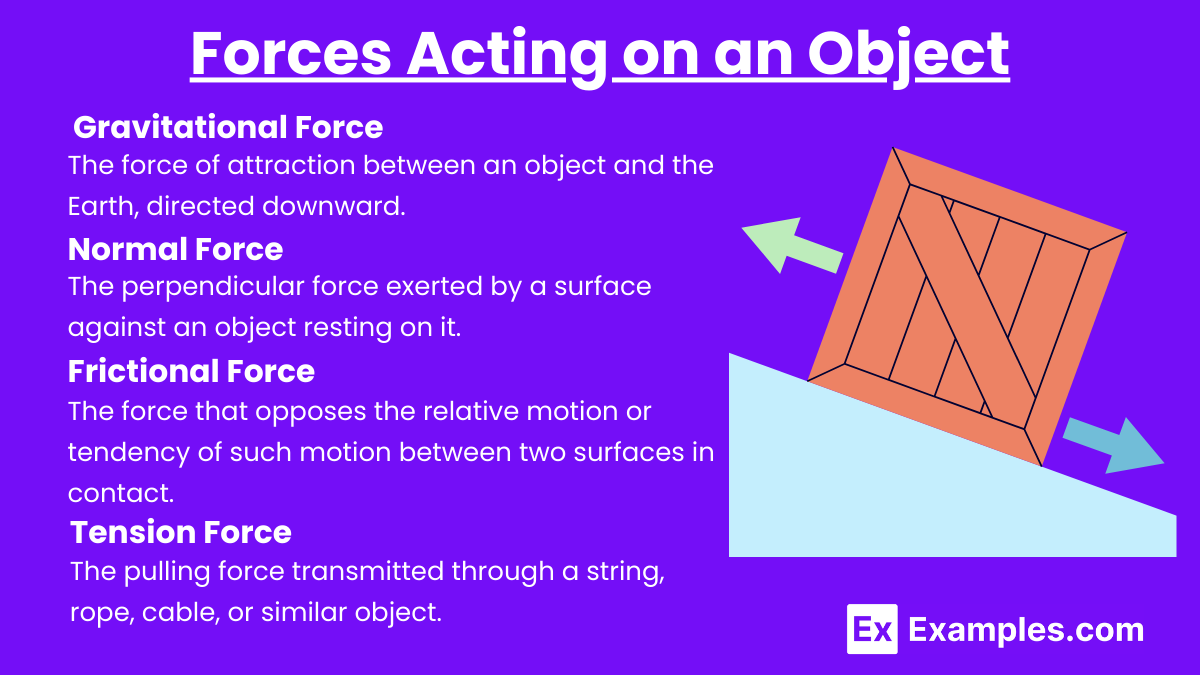
Gravitational Force
Gravitational Force: The force of attraction between an object and the Earth, directed downward.
Fg = mg
Normal Force
Normal Force: The perpendicular force exerted by a surface against an object resting on it.
Frictional Force
Frictional Force: The force that opposes the relative motion or tendency of such motion between two surfaces in contact.
Tension Force
Tension Force: The pulling force transmitted through a string, rope, cable, or similar object.
Examples and Problems
Example 1: A Block on a Horizontal Surface
Scenario: A block of mass m is placed on a horizontal surface. Identify and describe the forces acting on the block and determine if it is in equilibrium.
Forces Acting:
Gravitational force (Fg): Fg = mg
Normal force (Fₙ): Balances the gravitational force
Conclusion: The block is in static equilibrium as the net force acting on it is zero.
Example 2: A Moving Car
Scenario: A car is moving at a constant velocity on a straight road. Identify the forces acting on the car and determine if it is in equilibrium.
Forces Acting:
Gravitational force (Fg)
Normal force (Fₙ)
Applied force (Fa): Propels the car forward
Frictional force (Fբ): Opposes the motion
Conclusion: The car is in dynamic equilibrium as the net force acting on it is zero, resulting in no acceleration (constant velocity).
Example 3: Satellite Orbit
Scenario: A satellite orbits Earth in a circular path at constant speed. Identify the forces acting on the satellite and determine its state of equilibrium.
Forces Acting:
Gravitational force (Fg): Acts as the centripetal force keeping the satellite in orbit.
Conclusion: The satellite is in dynamic equilibrium, maintaining constant speed in its orbit due to the gravitational force providing the necessary centripetal force.