Newton’s Third Law and Free-Body Diagrams

Team English - Examples.com
Last Updated: November 7, 2024

Understanding Newton’s Third Law of Motion and mastering the use of Free-Body Diagrams (FBDs) are essential for excelling in AP Physics. These concepts are fundamental to analyzing forces and interactions between objects. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

Understand Newton’s Third Law of Motion, which states that for every action, there is an equal and opposite reaction. Apply this law to solve problems involving forces in various contexts, including collisions, tension, and equilibrium situations. Analyze force pairs and their interactions in different systems. Develop proficiency in identifying action-reaction pairs in complex scenarios and use this understanding to predict the behavior of objects. Prepare to tackle multiple-choice and free-response questions on Newton’s Third Law in the AP Physics exam.

Newton’s Third Law

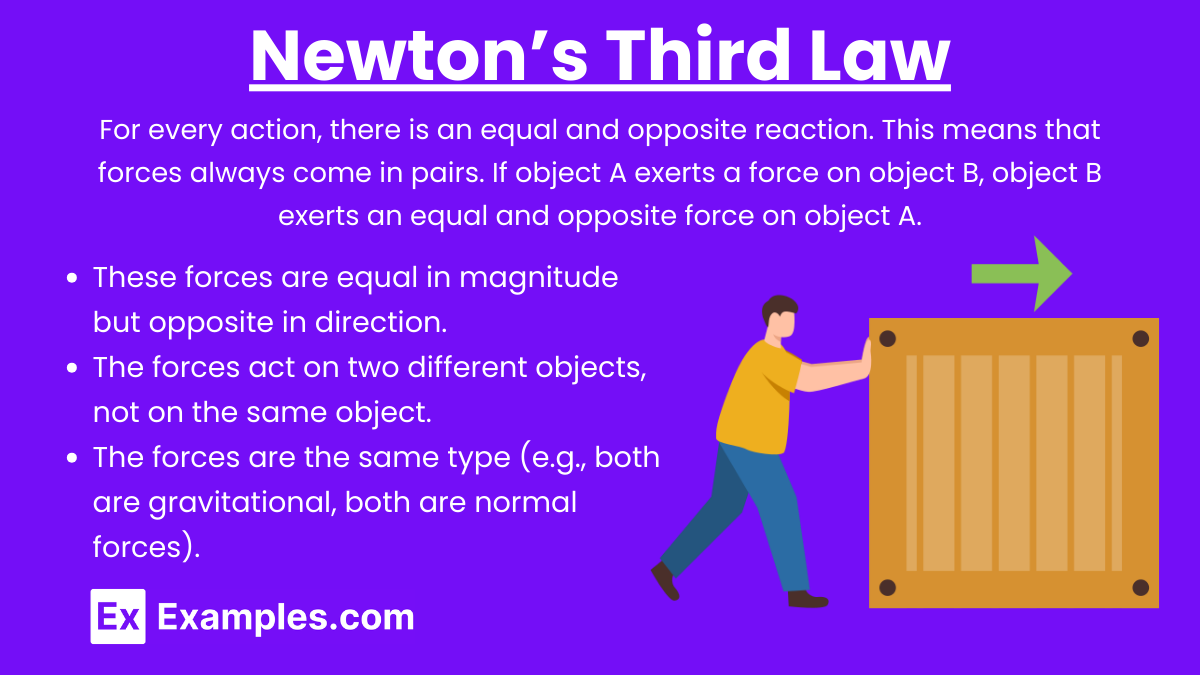
Newton’s Third Law

Newton’s Third Law of Motion: For every action, there is an equal and opposite reaction. This means that forces always come in pairs. If object A exerts a force on object B, object B exerts an equal and opposite force on object A.

Key Concepts

  • Action and Reaction Forces: These forces are equal in magnitude but opposite in direction.
  • Interaction Pairs: The forces act on two different objects, not on the same object.
  • Force Pairs: The forces are the same type (e.g., both are gravitational, both are normal forces).

Examples

  1. Walking:
    • Action: Your foot pushes backward on the ground.
    • Reaction: The ground pushes forward on your foot with an equal and opposite force, propelling you forward.
  2. Book on a Table:
    • Action: The book exerts a downward force on the table due to gravity.
    • Reaction: The table exerts an upward normal force on the book, equal in magnitude and opposite in direction.
  3. Rocket Launch:
    • Action: The rocket expels gas particles downward.
    • Reaction: The gas particles exert an equal and opposite force upward on the rocket, propelling it upward.

Applications of Newton’s Third Law

Applications of Newton's Third Law

In Engineering

  • Structural Design: Engineers design structures considering the action-reaction pairs to ensure stability.
  • Vehicle Design: Understanding action-reaction forces helps in designing efficient propulsion systems for cars, airplanes, and rockets.

In Daily Life

  • Sports: Athletes use the ground reaction force for activities like jumping and sprinting.
  • Tools: Tools like hammers and wrenches rely on action-reaction forces to function effectively.

Free-Body Diagrams (FBDs)

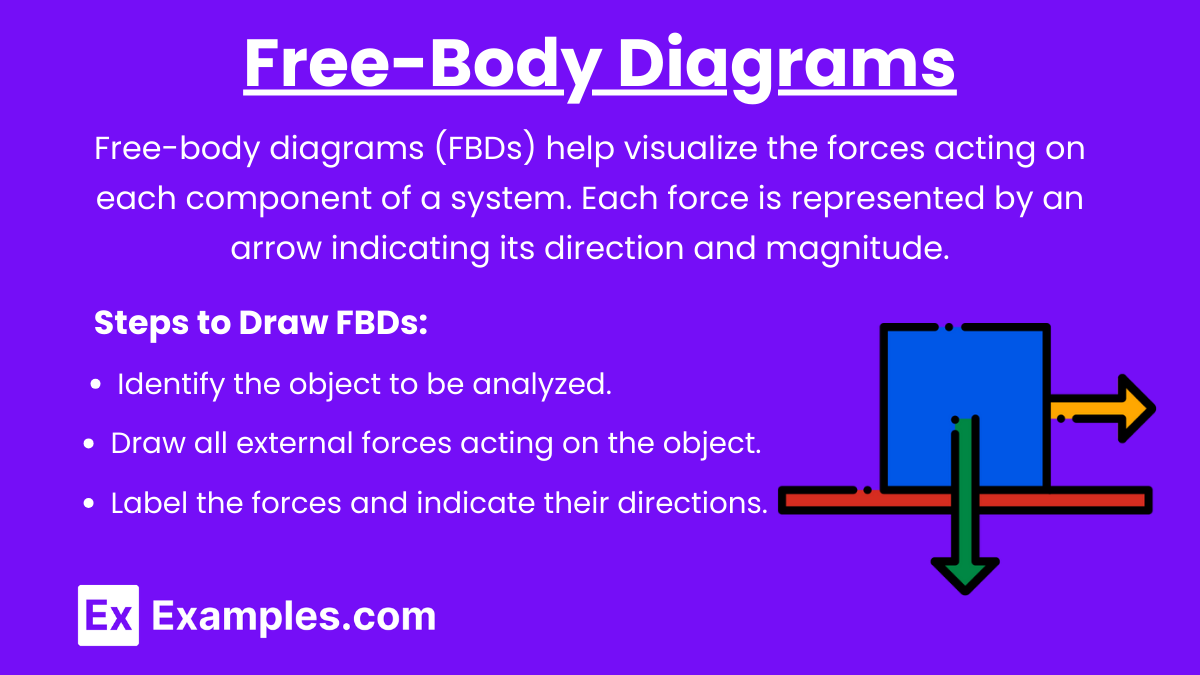
Free-Body Diagrams

Free-Body Diagram: A free-body diagram is a graphical representation used to visualize the forces acting on a single object. Each force is represented by an arrow pointing in the direction of the force, with the length proportional to the force’s magnitude.

Steps to Draw FBDs

  1. Identify the Object:
    • Select the object for which the FBD is to be drawn.
  2. Isolate the Object:
    • Represent the object as a dot or a simple shape.
  3. Draw the Forces:
    • Draw arrows for all forces acting on the object, originating from the center of the object. Label each force appropriately (e.g., Fg for gravitational force, Fₙ​ for normal force).
  4. Indicate the Direction:
    • Ensure the arrows point in the correct direction of the forces.
  5. Check for Completeness:
    • Ensure all forces acting on the object are included.

Common Forces in FBDs

  • Gravitational Force (Weight): The force due to gravity acting downward, Fg = mg.
  • Normal Force: The perpendicular force exerted by a surface on an object in contact with it.
  • Frictional Force: The force that opposes the relative motion between two surfaces in contact.
  • Tension Force: The pulling force transmitted through a string, rope, cable, or similar object.
  • Applied Force: Any external force applied to the object.

Example 1: Block on a Horizontal Surface

Scenario: A block of mass m is resting on a horizontal surface.

Forces Acting:

  • Gravitational force (Fg​): Fg​=mg
  • Normal force (Fₙ​): Balances the gravitational force, Fₙ​ = mg

Free-Body Diagram:

    \[ \begin{array}{c} \uparrow \\ F_N \\ \square \\ F_g \\ \downarrow \end{array} \]

Example 2: Block on an Inclined Plane

Scenario: A block of mass m is placed on an inclined plane making an angle θ with the horizontal.

Forces Acting:

  • Gravitational force (Fg​): Fg​ = mg
  • Normal force (Fₙ​): Perpendicular to the surface
  • Frictional force (Fբ): Opposes motion parallel to the surface

Components of Gravitational Force:

  • Parallel component:

        \[ F_{g\parallel} = mg \sin \theta \]

  • Perpendicular component:

        \[ F_{g\perp} = mg \cos \theta \]

Free-Body Diagram:

    \[ \begin{array}{c} \uparrow \\ F_N \\ \nearrow \\ F_{g\perp} \\ \searrow \\ \square \\ F_{g\parallel} \\ \downarrow \end{array} \]

​Example 3: Hanging Object

Scenario: An object of mass m is hanging from a rope.

Forces Acting:

  • Gravitational force (Fg​): Fg​ = mg
  • Tension force (T): Upward force exerted by the rope, T = mg

Free-Body Diagram:

    \[\begin{array}{c}\uparrow \\T \\\square \\F_g \\\downarrow\end{array}\]