Open and Closed Systems: Momentum
Understanding the concepts of open and closed systems and how momentum is conserved or transferred within these systems is crucial for mastering the principles of mechanics and achieving a high score on the AP Physics exam. Below are detailed notes to help you excel in this topic.
Learning Objectives
For the AP Physics exam, you should understand the concepts of open and closed systems in relation to momentum. Learn how momentum is conserved in closed systems and how external force impact open systems. Be able to apply the principle of conservation of momentum to solve problems involving collisions and explosions. Distinguish between isolated and non-isolated systems and analyze momentum changes within these contexts. Master equations and solve practice problems to reinforce these principles effectively.
Definitions
System
System: A system is a defined collection of objects that interact with each other. In physics, analyzing a system helps understand how various forces and interactions affect the motion and energy of objects within the system.
Open System
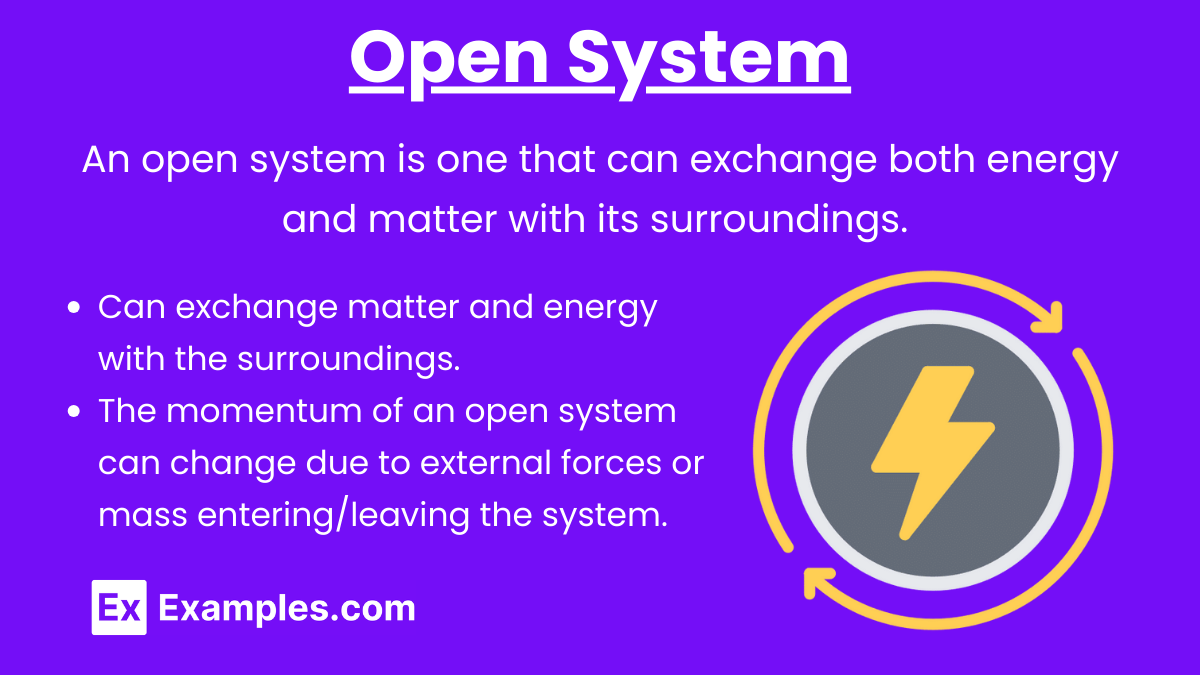
Open System: An open system is one that can exchange both energy and matter with its surroundings. This means that forces and mass can enter or leave the system, affecting its total momentum and energy.
Key Points:
- Can exchange matter and energy with the surroundings.
- The momentum of an open system can change due to external forces or mass entering/leaving the system.
Closed System
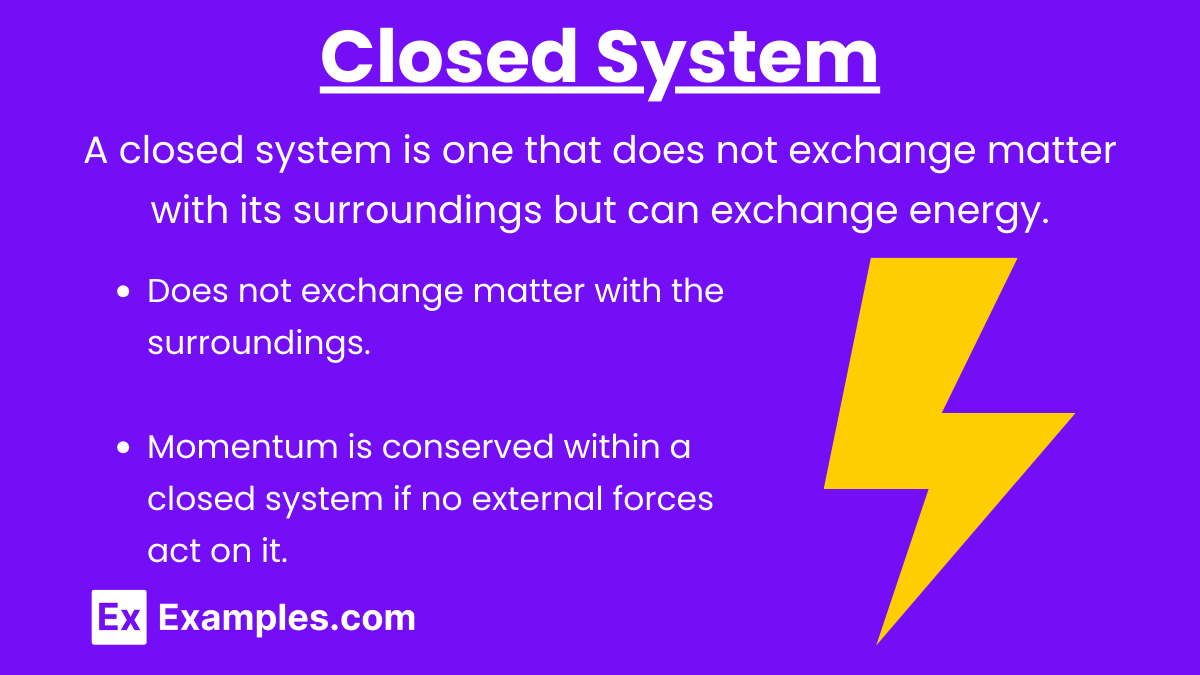
Closed System: A closed system is one that does not exchange matter with its surroundings but can exchange energy. For momentum considerations, a perfectly closed system is often considered isolated, meaning it does not exchange either matter or energy with its surroundings.
Key Points:
- Does not exchange matter with the surroundings.
- Momentum is conserved within a closed system if no external forces act on it.
Momentum
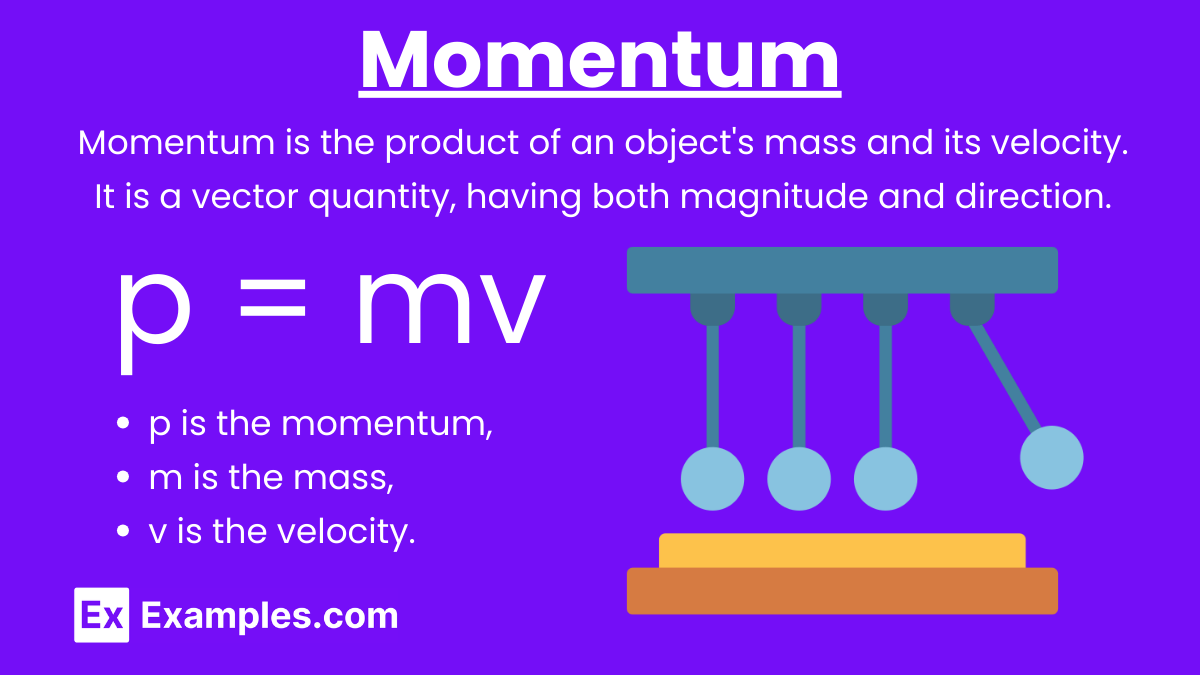
Momentum: Momentum is the product of an object’s mass and its velocity. It is a vector quantity, having both magnitude and direction.
Formula: p = mv
where:
- p is the momentum,
- m is the mass,
- v is the velocity.
Conservation of Momentum: The total momentum of a closed system remains constant if no external forces act on it. This is known as the law of conservation of momentum.
Formula: \[ \mathbf{p}_{\text{initial}} = \mathbf{p}_{\text{final}} \]
Applications
- Collisions:
- Inelastic Collisions: Objects stick together after collision.
- Elastic Collisions: Objects bounce off each other with no loss in total kinetic energy.
- Explosions:
- Objects move apart with equal and opposite momentum.
Analyzing Systems
Closed System (Isolated System)
In a closed system, the total momentum before any interaction is equal to the total momentum after the interaction, provided no external forces act on the system.
Example 1: Collision of Two Objects
- Two objects collide in a closed system.
- Initial momenta: p₁ + p₂
- Final momenta: p₁′ + p₂′
- Conservation of momentum: p₁+p₂ = p₁′+p₂′
Example Calculation: Two ice skaters push off from each other. Skater A has a mass of 50 kg and moves at 2 m/s, while skater B has a mass of 70 kg and moves at 1.43 m/s in the opposite direction. Calculate the initial total momentum and show it equals the final total momentum.
- Initial total momentum: 0 (both skaters are initially at rest)
- Final momentum of skater A: 50kg×2m/s = 100kg⋅m/s
- Final momentum of skater B: 70kg×(−1.43)m/s = −100.1kg⋅m/s
- Total final momentum: 100kg⋅m/s+(−100.1kg⋅m/s) = −0.1kg⋅m/s
(approximately conserved, considering minor calculation error)
Open System
In an open system, momentum can change due to external forces or the addition/removal of mass.
Example 2: Rocket Propulsion
- A rocket expels gas out of its engine to propel forward.
- The system (rocket + expelled gas) is open because mass (gas) leaves the system.
- Momentum change is described by the thrust force exerted by the expelled gas.
Example Calculation: A rocket with an initial mass of 1000 kg expels 50 kg of gas at a velocity of 200 m/s relative to the rocket. Calculate the change in momentum.
Momentum of expelled gas: 50kg×200m/s = 10000kg⋅m/s
The rocket gains an equal and opposite momentum: −10000kg⋅m/s
Conservation of Momentum in Collisions
Elastic Collisions
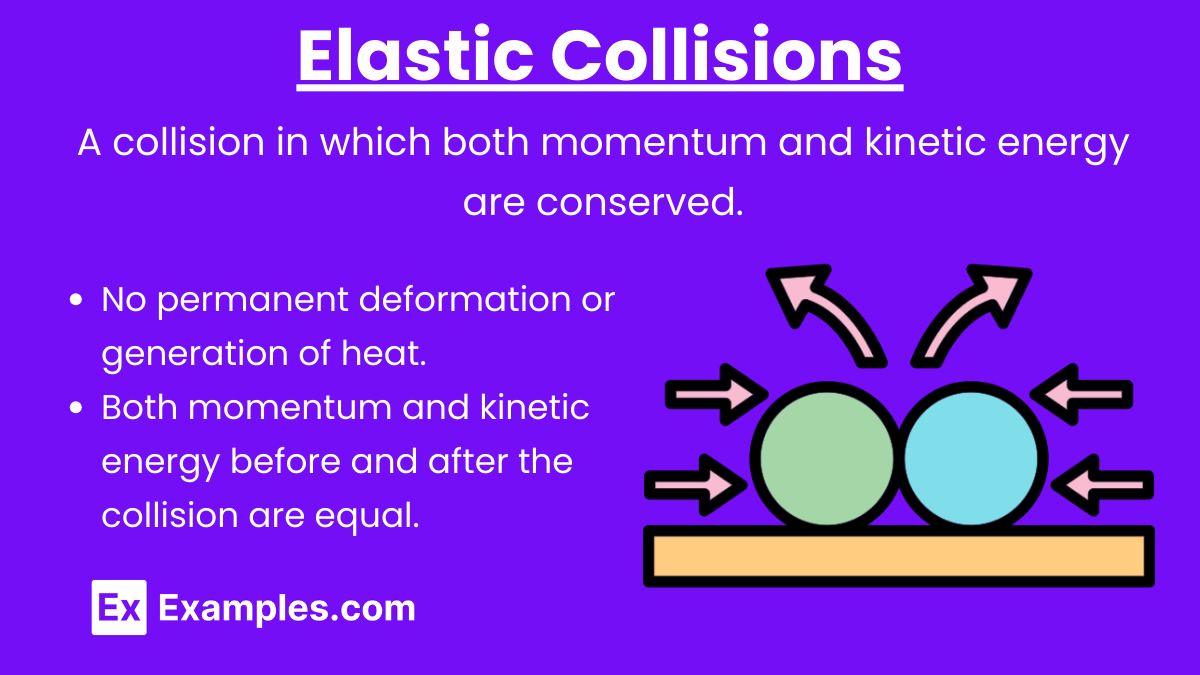
Definition: In an elastic collision, both momentum and kinetic energy are conserved. The objects collide and rebound without lasting deformation or heat generation.
Conservation of momentum: \[ m_1 v_1 + m_2 v_2 = m_1 v_1′ + m_2 v_2′ \]
Conservation of kinetic energy: \[ \frac{1}{2} m_1 v_1^2 + \frac{1}{2} m_2 v_2^2 = \frac{1}{2} m_1 v_1’^2 + \frac{1}{2} m_2 v_2’^2 \]
Inelastic Collisions
Definition: In an inelastic collision, momentum is conserved, but kinetic energy is not. The objects may stick together or deform, generating heat or sound.
Key Points:
- No permanent deformation or generation of heat.
- Both momentum and kinetic energy before and after the collision are equal.
Equations:
- Conservation of momentum: \[ m_1 v_1 + m_2 v_2 = (m_1 + m_2) v’ \]
- Kinetic energy is not conserved: \[ \frac{1}{2} m_1 v_1^2 + \frac{1}{2} m_2 v_2^2 \neq \frac{1}{2} (m_1 + m_2) v’^2 \]
Inelastic Collisions
Definition: A Inelastic collision is a special case of inelastic collision where the colliding objects stick together after the collision.
Key Points:
- Objects may stick together after collision (perfectly inelastic collision).
- Momentum is conserved, but kinetic energy is not.
Equations:
- Conservation of momentum: \[ m_1 v_1 + m_2 v_2 = (m_1 + m_2) v’ \]
- Objects move together with a common velocity after collision.
Examples and Problems
Example 1: Inelastic Collision
Scenario: Two cars, one of mass 1500 kg traveling at 20 m/s and the other of mass 1000 kg at rest, collide and stick together. Calculate their final velocity.
Solution:
m₁ = 1500kg,
v₁ = 20m/s
m₂ = 1000kg,
v₂ = 0m/s
Using conservation of momentum:
\[ \begin{aligned} m_1 v_1 + m_2 v_2 &= (m_1 + m_2) v’ \\ (1500 \, \text{kg} \times 20 \, \text{m/s}) + (1000 \, \text{kg} \times 0) &= (1500 \, \text{kg} + 1000 \, \text{kg}) v’ \\ 30000 \, \text{kg} \cdot \text{m/s} &= 2500 \, \text{kg} \cdot v’ \\ v’ &= \frac{30000 \, \text{kg} \cdot \text{m/s}}{2500 \, \text{kg}} \\ v’ &= 12 \, \text{m/s} \end{aligned} \]
Example 2: Elastic Collision
Scenario: A 0.2 kg ball traveling at 3 m/s collides elastically with a 0.3 kg ball at rest. Calculate their velocities after the collision.
Solution: Using conservation of momentum and kinetic energy:
m₁ = 0.2kg,
v₁ = 3m/s
m₂ = 0.3kg,
v₂ = 0m/s
Momentum conservation:
\[ \begin{aligned} m_1 v_1 + m_2 v_2 &= m_1 v_1′ + m_2 v_2′ \\ 0.2 \times 3 + 0.3 \times 0 &= 0.2 v_1′ + 0.3 v_2′ \\ 0.6 &= 0.2 v_1′ + 0.3 v_2′ \end{aligned} \]
Kinetic energy conservation:
\[ \begin{aligned} &\frac{1}{2} m_1 v_1^2 + \frac{1}{2} m_2 v_2^2 = \frac{1}{2} m_1 v_1’^2 + \frac{1}{2} m_2 v_2’^2 \\ &\frac{1}{2} \times 0.2 \times 3^2 + \frac{1}{2} \times 0.3 \times 0^2 = \frac{1}{2} \times 0.2 \times v_1’^2 + \frac{1}{2} \times 0.3 \times v_2’^2 \\ &0.9 = 0.1 v_1’^2 + 0.15 v_2’^2 \end{aligned} \]
Solving these two equations simultaneously yields:
v₁′ = 0.6m/s
v₂′ = 2.4m/s
Example 3: Explosion
Scenario: A stationary 10 kg bomb explodes into two pieces, 4 kg and 6 kg, with the 4 kg piece moving at 15 m/s. Calculate the velocity of the 6 kg piece.
Solution: Using conservation of momentum:
\[ \begin{align*} m_1 &= 4 \, \text{kg}, \, \mathbf{v}_1 = 15 \, \text{m/s} \\ m_2 &= 6 \, \text{kg}, \, \mathbf{v}_2 = ? \\ 0 &= m_1 \mathbf{v}_1 + m_2 \mathbf{v}_2 \\ 0 &= 4 \times 15 + 6 \mathbf{v}_2 \\ 0 &= 60 + 6 \mathbf{v}_2 \\ \mathbf{v}_2 &= -10 \, \text{m/s} \end{align*} \]