Period of Simple Harmonic Oscillators

Last Updated: November 7, 2024

Notes

Understanding the period of simple harmonic oscillators (SHOs) is crucial for mastering oscillatory motion concepts in the AP Physics exam. This topic involves the study of systems that undergo periodic motion, such as springs and pendulums. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

In the topic of the Period of Simple Harmonic Oscillators for the AP Physics exam, you should learn to: define and understand simple harmonic motion (SHM), derive the formulas for the period of oscillation of mass-spring systems and pendulums, calculate the period using given parameters, and understand the physical factors affecting the period. Additionally, you should be able to apply these concepts to solve related problems, including those involving energy conservation and damping effects.

Simple Harmonic Motion (SHM)

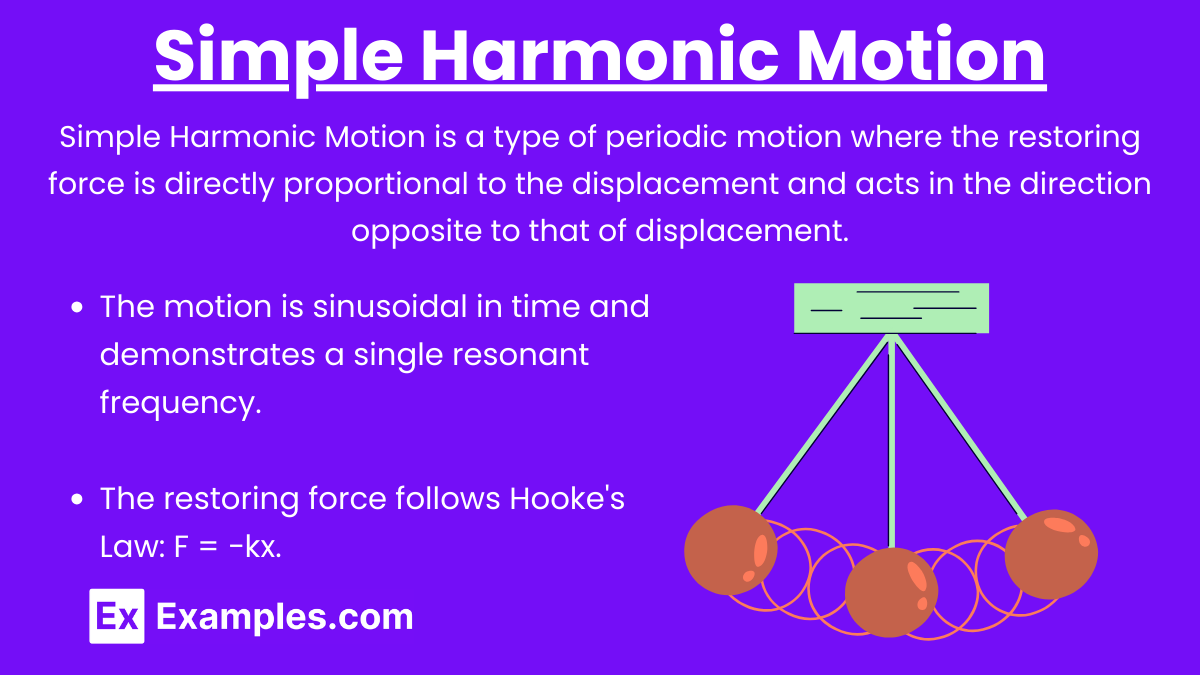
Simple Harmonic Motion: Simple Harmonic Motion is a type of periodic motion where the restoring force is directly proportional to the displacement and acts in the direction opposite to that of displacement.

Key Points:

The motion is sinusoidal in time and demonstrates a single resonant frequency.
The restoring force follows Hooke’s Law: F = −kx.

Key Characteristics:

Restoring Force: The force that brings the system back to its equilibrium position.
Displacement: The distance from the equilibrium position.
Periodic Motion: The motion repeats in a regular cycle.

Period of a Simple Harmonic Oscillator

The period of an oscillating system is the time it takes to complete one full cycle of motion.

Mass-Spring System

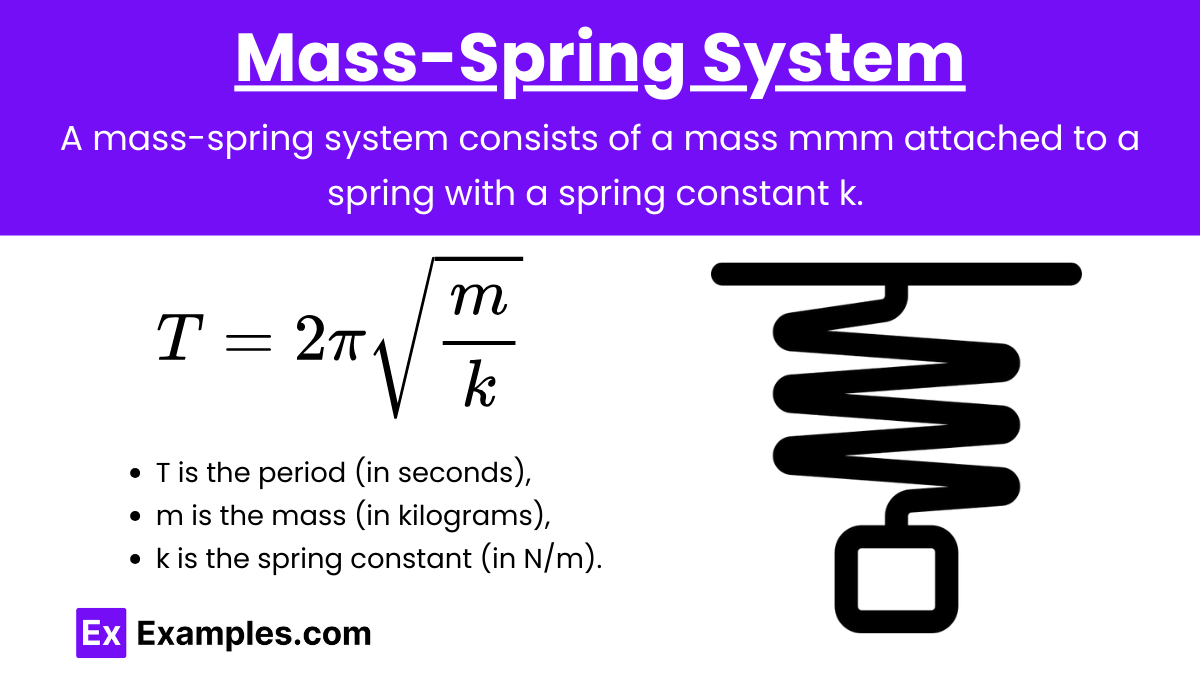
Mass-Spring System: A mass-spring system consists of a mass m attached to a spring with a spring constant k. The system exhibits SHM when displaced from its equilibrium position.

Formula for the Period (T):

$T = 2\pi \sqrt{\frac{m}{k}}$

where:

T is the period (in seconds),
m is the mass (in kilograms),
k is the spring constant (in N/m).

Key Points:

The period is independent of the amplitude of oscillation.
The period increases with increasing mass.
The period decreases with increasing spring constant.

Simple Pendulum

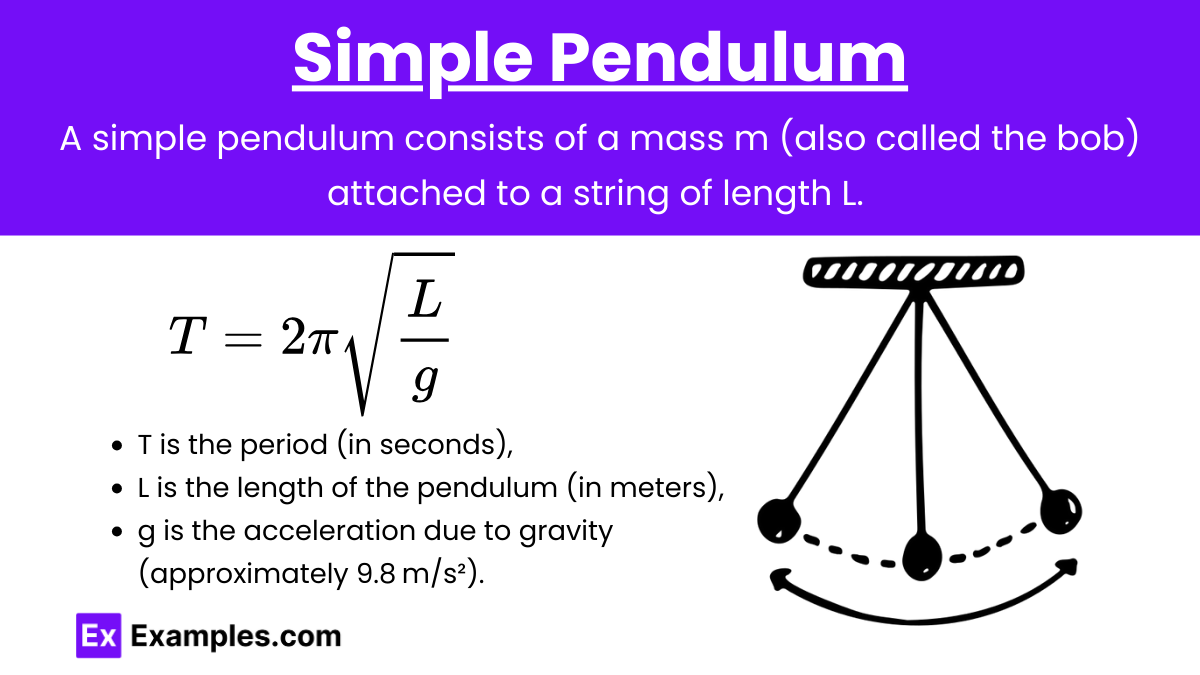
Simple Pendulum: A simple pendulum consists of a mass m (also called the bob) attached to a string of length L. The pendulum exhibits SHM for small angular displacements.

Formula for the Period (T):

$T = 2\pi \sqrt{\frac{L}{g}}$

where:

T is the period (in seconds),
L is the length of the pendulum (in meters),
g is the acceleration due to gravity (approximately 9.8 m/s²).

Key Points:

The period is independent of the mass of the bob.
The period increases with increasing length.
The period is independent of the amplitude for small oscillations.

Energy in Simple Harmonic Motion

Total Mechanical Energy: The total mechanical energy in SHM is the sum of the kinetic energy and potential energy and remains constant if there is no damping.

Mass-Spring System

Potential Energy (U):

$U = \frac{1}{2} k x^2$

Kinetic Energy (K):

$K = \frac{1}{2}mv^2$

Total Energy (E):

$E = \frac{1}{2}kA^2$

where:

A is the amplitude of oscillation.

Simple Pendulum

Potential Energy (U): U = mgh

where h is the height relative to the lowest point.

Kinetic Energy (K):

$K = \frac{1}{2}mv^2$

Total Energy (E):

$E = mgL(1 - \cos \theta)$

where:

θ is the angular displacement,
For small angles,
$\cos \theta \approx 1 - \frac{\theta^2}{2}, \text{ making } E \approx mgL \frac{\theta^2}{2}$
.

Examples and Problems

Example 1: Period of a Mass-Spring System

Scenario: A mass of 2 kg is attached to a spring with a spring constant of 50 N/m. Calculate the period of oscillation.

Solution:

$T = 2\pi \sqrt{\frac{m}{k}}$

$T = 2\pi \sqrt{\frac{2}{50}}$

$T = 2\pi \sqrt{0.04}$

$T = 2\pi \times 0.2$

$T \approx 1.26 \, \text{s}$

Example 2: Period of a Simple Pendulum

Scenario: A simple pendulum has a length of 1.5 meters. Calculate the period of oscillation.

Solution:

$T = 2\pi \sqrt{\frac{L}{g}}$

$T = 2\pi \sqrt{\frac{1.5}{9.8}}$

$T = 2\pi \sqrt{0.153}$

$T \approx 2\pi \times 0.391$

$T \approx 2.46 \, \text{s}$

Example 3: Energy in a Mass-Spring System

Scenario: A mass-spring system with a spring constant of 25 N/m and a mass of 1 kg oscillates with an amplitude of 0.5 meters. Calculate the total mechanical energy.

Solution:

$E = \frac{1}{2}kA^2$

$E = \frac{1}{2} \times 25 \times (0.5)^2$

$E = \frac{1}{2} \times 25 \times 0.25$

$E = 3.125 \, \text{J}$

Example 4: Vertical Spring

Scenario: A 3 kg mass hangs vertically from a spring with a spring constant of 120 N/m. Determine the period of oscillation.

Solution:

$T = 2\pi \sqrt{\frac{m}{k}}$

$T = 2\pi \sqrt{\frac{3 \, \text{kg}}{120 \, \text{N/m}}}$

$T = 2\pi \sqrt{0.025}$

$T \approx 2\pi \times 0.158$

$T \approx 0.99 \, \text{s}$

Example 5: Short Pendulum

Scenario: A pendulum with a length of 0.25 meters oscillates. Calculate its period.

Solution:

$T = 2\pi \sqrt{\frac{L}{g}}$

$T = 2\pi \sqrt{\frac{0.25 \, \text{m}}{9.8 \, \text{m/s}^2}}$

$T = 2\pi \sqrt{0.0255}$

$T \approx 2\pi \times 0.16$

$T \approx 1.01 \, \text{s}$

Applications of Period of Simple Harmonic Oscillators

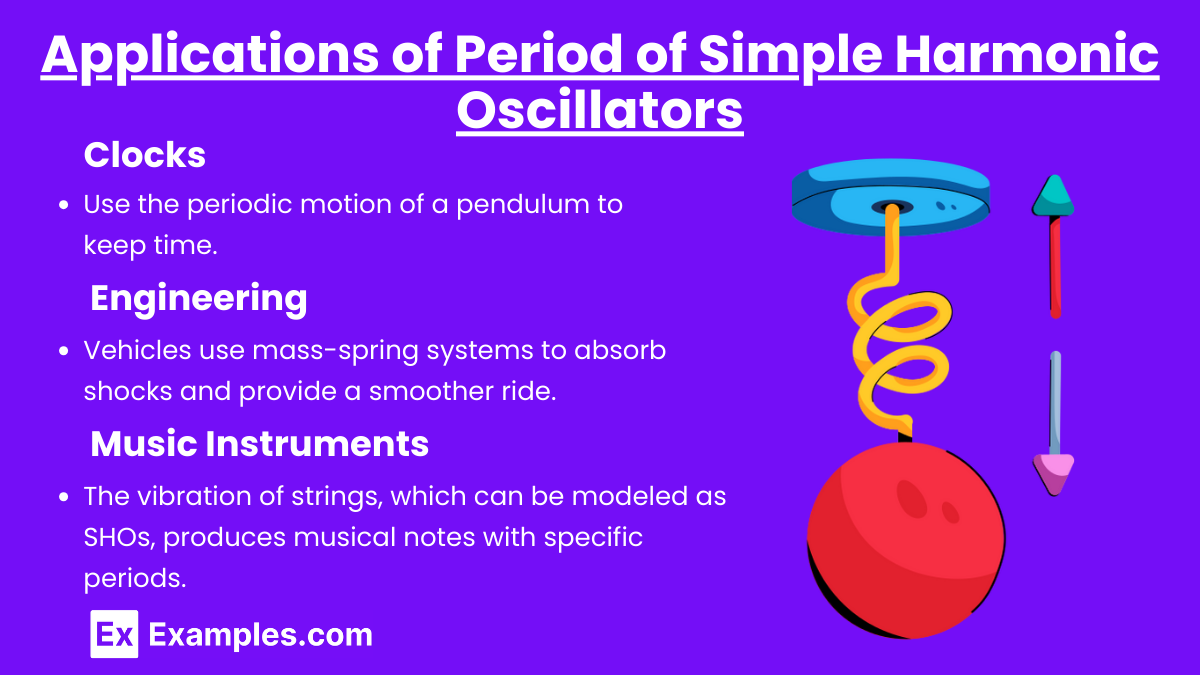
1. Clocks

Pendulum Clocks: Use the periodic motion of a pendulum to keep time.
Quartz Watches: Use the vibrations of a quartz crystal (acting as a SHO) to maintain accurate time.

2. Engineering

Vibration Analysis: Understanding the period of SHO helps in designing buildings and structures to withstand oscillations and vibrations.
Suspension Systems: Vehicles use mass-spring systems to absorb shocks and provide a smoother ride.

3. Music Instruments

String Instruments: The vibration of strings, which can be modeled as SHOs, produces musical notes with specific periods.
Wind Instruments: Air columns in wind instruments behave like SHOs, creating sound waves with periodic oscillations.

Practice Problems

Question 1: What is the period of a simple harmonic oscillator with a mass m and a spring constant k?

$A. \quad 2\pi \sqrt{\frac{m}{k}}$

$B. \quad 2\pi \sqrt{\frac{k}{m}}$

$C. \quad \frac{1}{2}\pi \sqrt{\frac{m}{k}}$

$D. \quad \frac{1}{2}\pi \sqrt{\frac{k}{m}}$

Answer: A

Explanation: The period T of a simple harmonic oscillator, such as a mass-spring system, is given by the formula:

$T = 2\pi \sqrt{\frac{m}{k}}$

This formula shows that the period depends on the mass m of the object and the spring constant k. The correct answer is A.

Question 2: Which of the following will result in an increase in the period of a simple harmonic oscillator?

A. Increasing the spring constant

B. Decreasing the spring constant

C. Decreasing the mass

D. None of the above

Answer: B

Explanation: The period T of a simple harmonic oscillator is given by:

$T = 2\pi \sqrt{\frac{m}{k}}$

From this equation, we see that the period is directly proportional to the square root of the mass m and inversely proportional to the square root of the spring constant k. Therefore, decreasing the spring constant k will result in an increase in the period T. The correct answer is B.

Question 3: For a pendulum of length L and acceleration due to gravity g, what is the formula for the period?

$A. \; 2\pi \sqrt{\frac{g}{L}}$

$B. \; 2\pi \sqrt{\frac{L}{g}}$

$C. \; \frac{1}{2\pi} \sqrt{\frac{g}{L}}$

$D. \; \frac{1}{2\pi} \sqrt{\frac{L}{g}}$

Answer: B

Explanation: The period T of a simple pendulum is given by the formula:

$T = 2\pi \sqrt{\frac{g}{L}}$

where L is the length of the pendulum and g is the acceleration due to gravity. This equation shows that the period depends on the length of the pendulum and the gravitational acceleration. The correct answer is B.