Position, Velocity, and Acceleration

Understanding the concepts of position, velocity, and acceleration is crucial for excelling in AP Physics. These concepts form the foundation of kinematics, the study of motion without considering its causes. Below are detailed notes to help you master these topics and achieve a high score on your AP Physics exam.

Learning Objectives

By studying position, velocity, and acceleration for the AP Physics exam, you should learn to define and distinguish between these fundamental kinematic quantities, interpret and analyze position-time, velocity-time, and acceleration-time graphs, derive equations of motion for objects under constant acceleration, solve problems involving linear motion, and understand the vector nature of these quantities. Mastering these objectives will enable you to analyze real-world motion scenarios and apply kinematic principles effectively in various contexts.

Formulas

Position

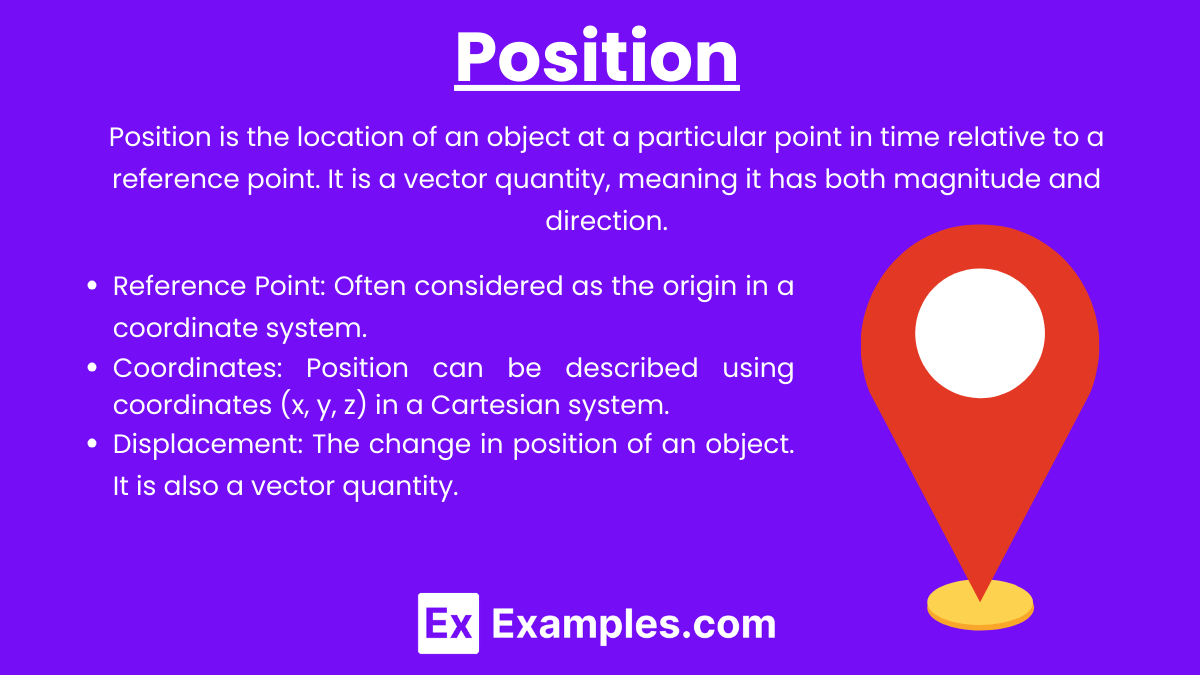
Definition: Position is the location of an object at a particular point in time relative to a reference point. It is a vector quantity, meaning it has both magnitude and direction.

Key Points:

• Reference Point: Often considered as the origin in a coordinate system.
• Coordinates: Position can be described using coordinates (x, y, z) in a Cartesian system.
• Displacement: The change in position of an object. It is also a vector quantity.

Formula:
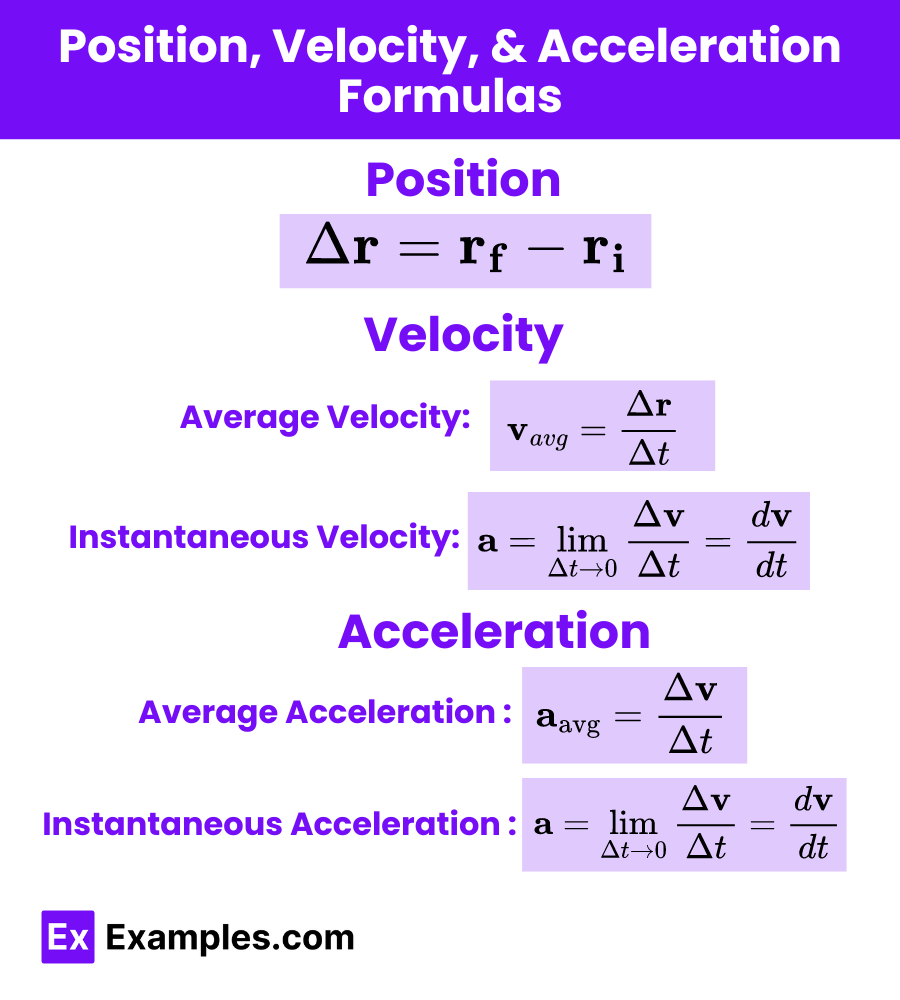
\[\Delta \mathbf{r} = \mathbf{r_f} – \mathbf{r_i} \]
where rբ is the final position and rᵢ​ is the initial position.

Velocity

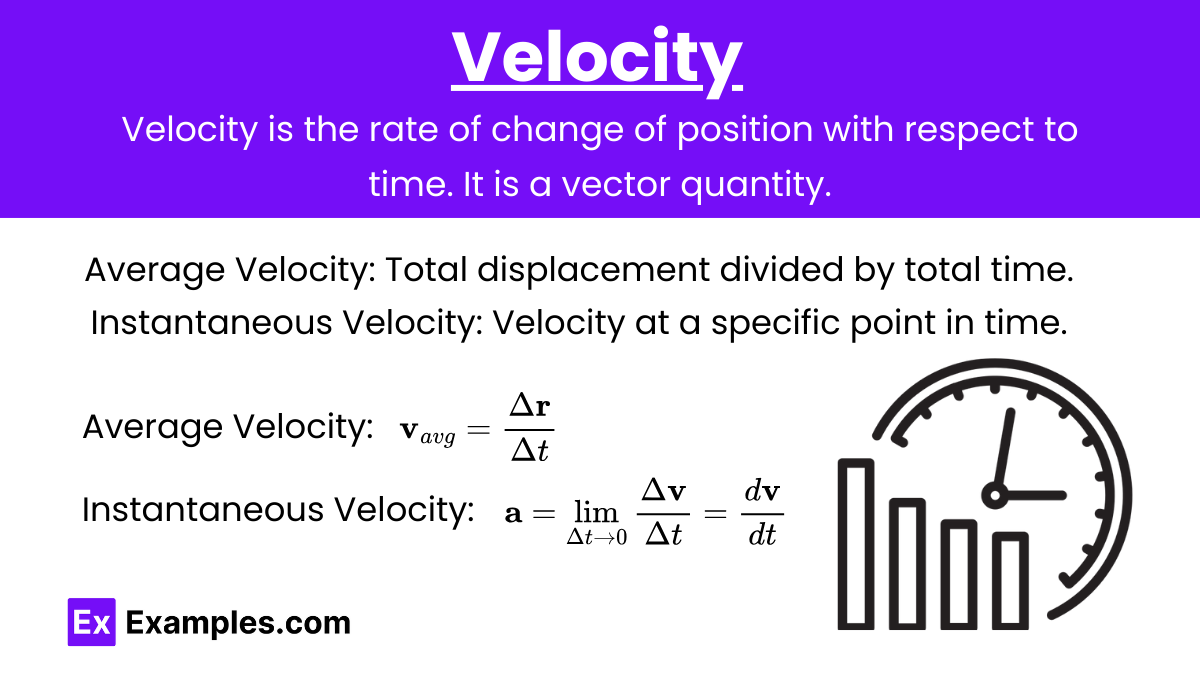
Definition: Velocity is the rate of change of position with respect to time. It is a vector quantity.

Key Points:

• Average Velocity: Total displacement divided by total time.
• Instantaneous Velocity: Velocity at a specific point in time.

Formulas:

• Average Velocity: \[ \mathbf{v}_{avg} = \frac{\Delta \mathbf{r}}{\Delta t} \]
  
• Instantaneous Velocity:
  \[ \mathbf{a} = \lim_{\Delta t \to 0} \frac{\Delta \mathbf{v}}{\Delta t} = \frac{d\mathbf{v}}{dt} \]

Graphical Interpretation:

• Slope of the position-time graph gives the velocity.

Acceleration

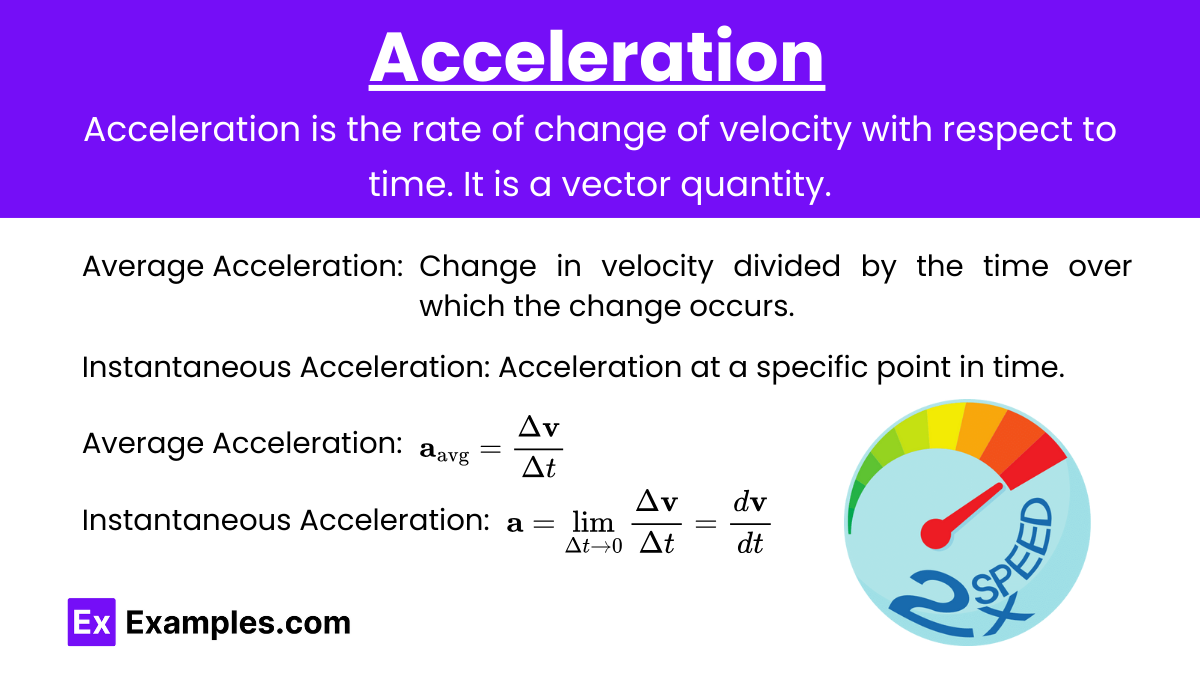
Definition: Acceleration is the rate of change of velocity with respect to time. It is a vector quantity.

Key Points:

• Average Acceleration: Change in velocity divided by the time over which the change occurs.
• Instantaneous Acceleration: Acceleration at a specific point in time.

Formulas:

• Average Acceleration: \[ \mathbf{a_{\text{avg}}} = \frac{\Delta \mathbf{v}}{\Delta t} \]
• Instantaneous Acceleration:
  \[ \mathbf{a} = \lim_{\Delta t \to 0} \frac{\Delta \mathbf{v}}{\Delta t} = \frac{d\mathbf{v}}{dt} \]

Graphical Interpretation:

• Slope of the velocity-time graph gives the acceleration.

Relationship Between Position, Velocity, and Acceleration

These quantities are interconnected through calculus:

• Velocity from Position: \[ \mathbf{v} = \frac{d\mathbf{r}}{dt} \]
• Acceleration from Velocity: \[ \mathbf{a} = \frac{d\mathbf{v}}{dt} \]
• Position from Velocity: \[ \mathbf{r}(t) = \mathbf{r_0} + \int_{0}^{t} \mathbf{v}(t’) \, dt’ \]
• Velocity from Acceleration: \[ \mathbf{v}(t) = \mathbf{v_0} + \int_{0}^{t} \mathbf{a}(t’) \, dt’ \]

Examples

Example 1: Constant Velocity

An object moves with a constant velocity of 5 m/s to the east.

• Position-Time Equation: \[x(t) = x_0 + vt\]
• Velocity: 5 m/s (constant)
• Acceleration: 0 m/s²

Example 2: Constant Acceleration

An object starts from rest and accelerates at 2 m/s² to the north.

• Position-Time Equation: \[y(t) = y_0 + v_0 t + \frac{1}{2} at^2\]
• Velocity-Time Equation: \[v(t) = v_0 + at\]

If y₀ ​= 0 and v₀ ​= 0:
\[
y(t) = \frac{1}{2}(2)t^2 = t^2
\]
\[ v(t) = 2t \]

Example 3: Free Fall

An object is dropped from a height with an initial velocity of 0. Acceleration due to gravity is g=9.8m/s2.

• Position-Time Equation: \[y(t) = y_0 + v_0 t + \frac{1}{2} gt^2\]
• Velocity-Time Equation: \[v(t) = v_0 + gt\]

For y₀​ = 0 and v₀ = 0:
\[
y(t) = \frac{1}{2} (9.8) t^2 = 4.9t^2
\]
\[ v(t) = 9.8t \]