Rotational Kinematics
Understanding rotational kinematics is essential for mastering topics related to rotational motion and dynamics, which are crucial for the AP Physics exam. This topic involves analyzing the motion of rotating objects using angular quantities. Below are detailed notes to help you achieve a high score on your AP Physics exam.
Learning Objectives
By studying rotational kinematics for the AP Physics exam, you should understand the concepts of angular displacement, angular velocity, and angular acceleration. You should be able to apply rotational kinematic equations to solve problems involving the rotational motion of rigid bodies. Additionally, you should grasp the relationship between linear and angular quantities, and analyze rotational motion using graphical methods. Understanding these principles will help in interpreting physical situations involving rotating systems and predicting their behavior under various conditions.
Angular Quantities
Angular Displacement (θ)
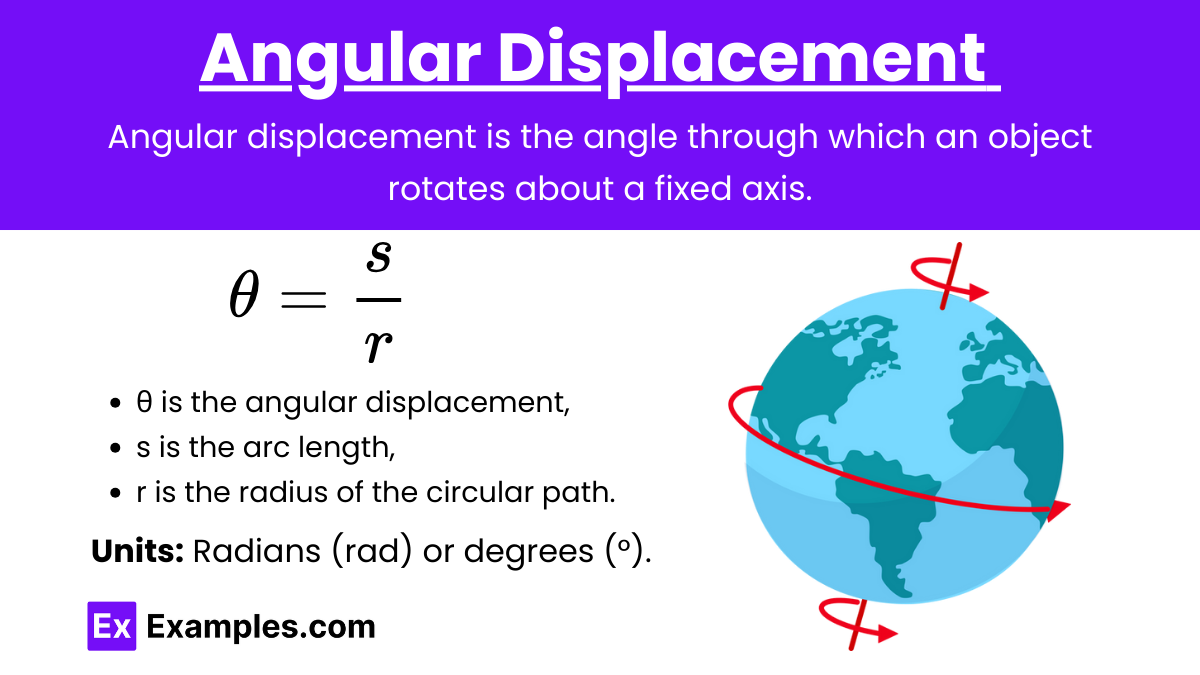
Definition: Angular displacement is the angle through which an object rotates about a fixed axis. It is the rotational analog of linear displacement.
Units: Radians (rad) or degrees (°).
Formula: \[ \theta = \frac{s}{r} \]
where:
- θ is the angular displacement,
- s is the arc length,
- r is the radius of the circular path.
Angular Velocity (ω)
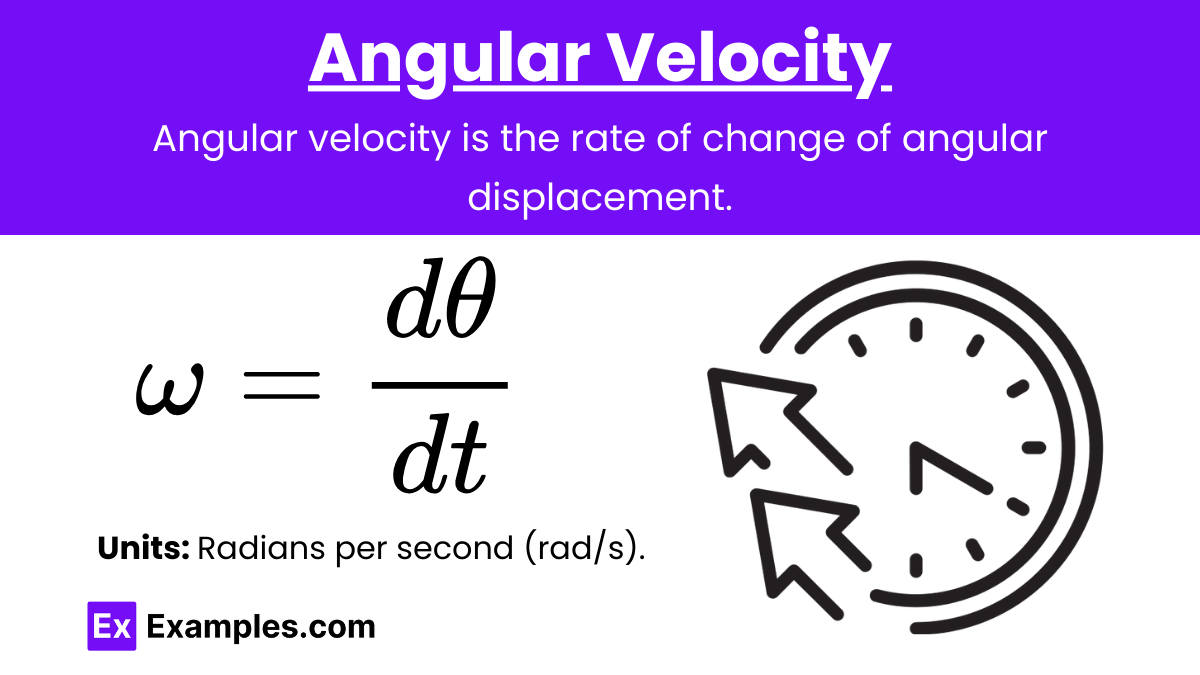
Definition: Angular velocity is the rate of change of angular displacement. It is the rotational analog of linear velocity.
Units: Radians per second (rad/s).
Formula: \[ \omega = \frac{d\theta}{dt} \]
Average Angular Velocity: \[ \omega_{\text{avg}} = \frac{\Delta \theta}{\Delta t} \]
where:
- ω is the angular velocity,
- Δθ is the change in angular displacement,
- Δt is the change in time.
Angular Acceleration (α)
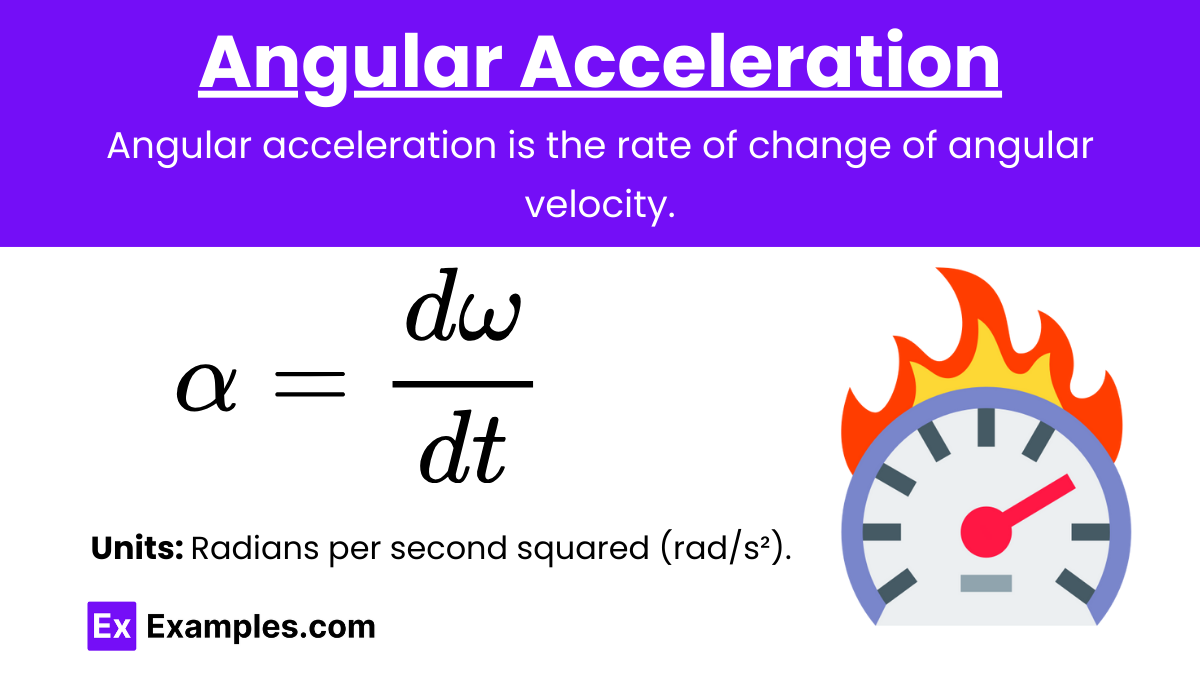
Definition: Angular acceleration is the rate of change of angular velocity. It is the rotational analog of linear acceleration.
Units: Radians per second squared (rad/s²).
Formula: \[ \alpha = \frac{d\omega}{dt} \]
Average Angular Acceleration: \[ \alpha_{\text{avg}} = \frac{\Delta \omega}{\Delta t} \]
where:
- α is the angular acceleration,
- Δω is the change in angular velocity,
- Δt is the change in time.
Kinematic Equations for Rotational Motion
The equations for rotational motion are analogous to the kinematic equations for linear motion. They describe the relationships between angular displacement, angular velocity, angular acceleration, and time.
Angular Displacement with Constant Angular Acceleration
\[ \theta = \theta_0 + \omega_0 t + \frac{1}{2} \alpha t^2 \]
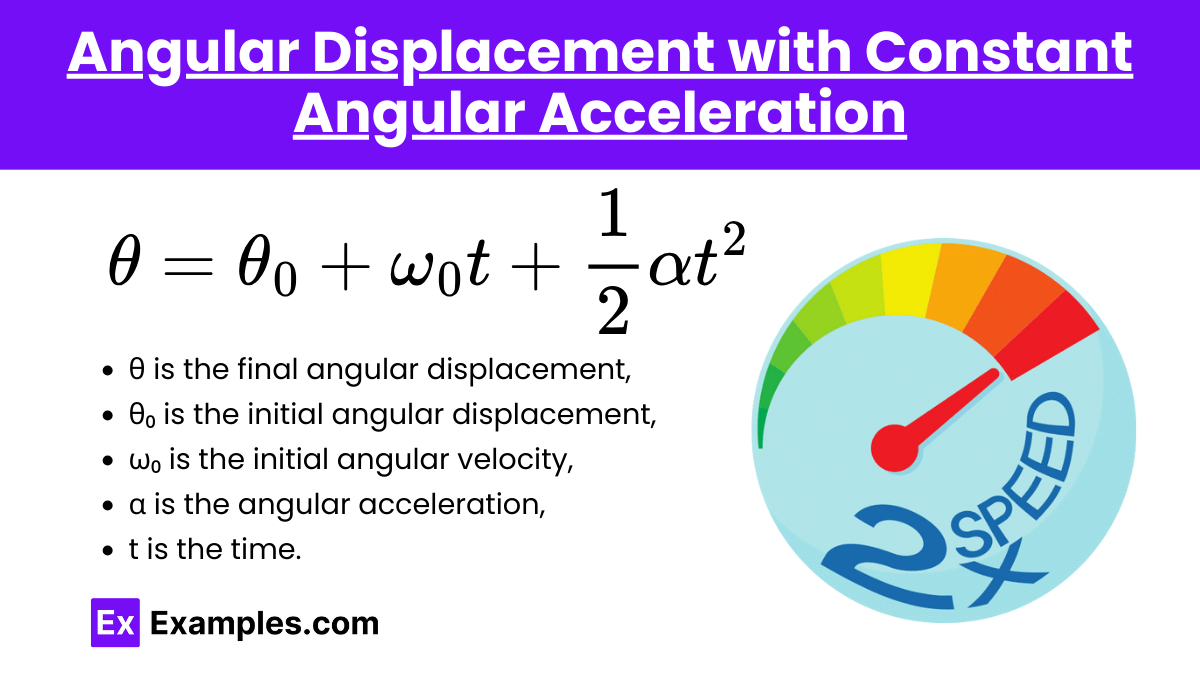
where:
- θ is the final angular displacement,
- θ₀ is the initial angular displacement,
- ω₀ is the initial angular velocity,
- α is the angular acceleration,
- t is the time.
Angular Velocity with Constant Angular Acceleration
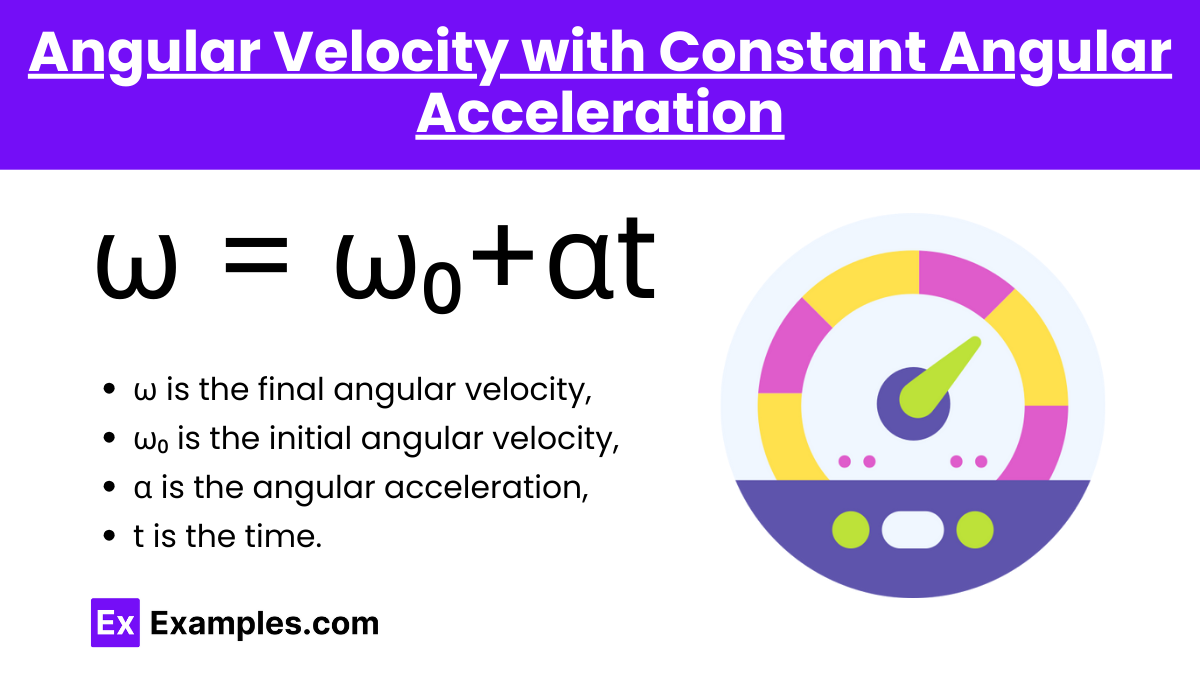
ω = ω₀+αt
where:
- ω is the final angular velocity,
- ω₀ is the initial angular velocity,
- α is the angular acceleration,
- t is the time.
Angular Velocity and Angular Displacement
\[ \omega^2 = \omega_0^2 + 2 \alpha (\theta – \theta_0) \]
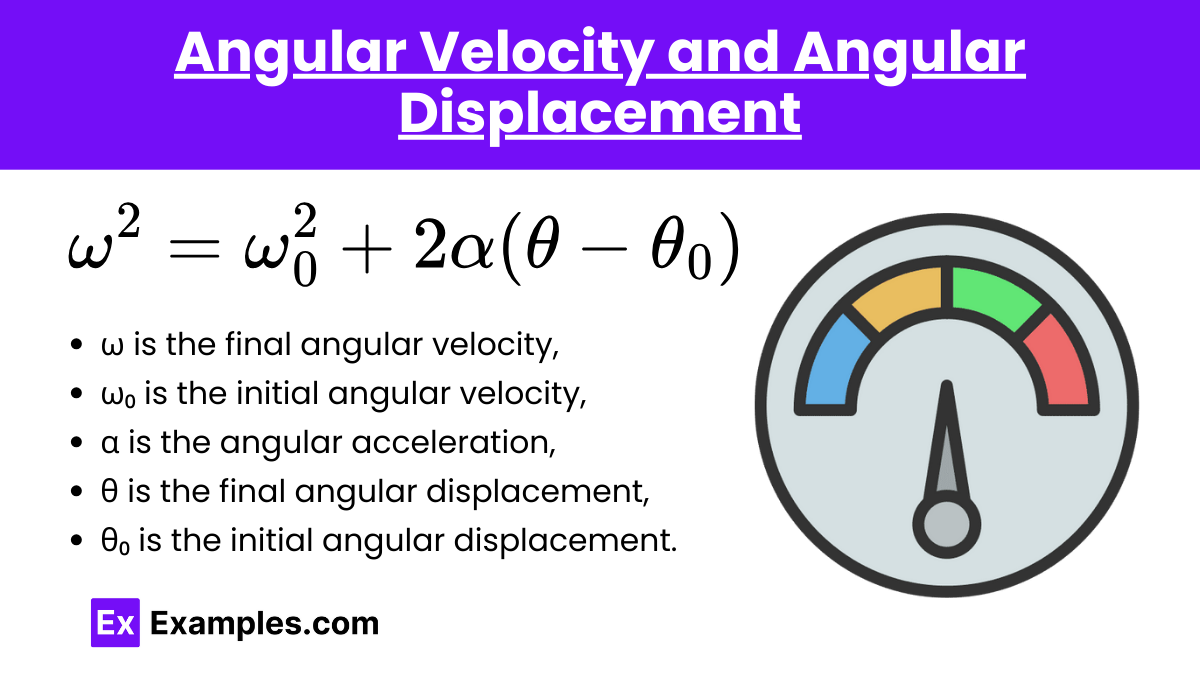
where:
- ω is the final angular velocity,
- ω₀ is the initial angular velocity,
- α is the angular acceleration,
- θ is the final angular displacement,
- θ₀ is the initial angular displacement.
Average Angular Velocity
\[ \omega_{\text{avg}} = \frac{\omega + \omega_0}{2} \]
where:
- \[ \omega_{\text{avg}}\] is the average angular velocity,
- ω is the final angular velocity,
- ω₀ is the initial angular velocity.
Relationship Between Linear and Angular Quantities
Linear and Angular Displacement
s = rθ
where:
- s is the linear displacement,
- r is the radius of the circular path,
- θ is the angular displacement.
Linear and Angular Velocity
v = rω
where:
- v is the linear velocity,
- r is the radius of the circular path,
- ω is the angular velocity.
Linear and Angular Acceleration
a = rα
where:
- a is the linear acceleration,
- r is the radius of the circular path,
- α is the angular acceleration.
Example 1
Rotating Disk
Scenario: A disk starts from rest and accelerates uniformly at 2rad/s². Calculate the angular velocity and angular displacement after 5 seconds.
Solution: \[ \text{Using } \omega = \omega_0 + \alpha t: \] \[ \omega = 0 + 2 \times 5 = 10 \, \text{rad/s} \] \[ \text{Using } \theta = \theta_0 + \omega_0 t + \frac{1}{2} \alpha t^2: \] \[ \theta = 0 + 0 \times 5 + \frac{1}{2} \times 2 \times 5^2 = 25 \, \text{rad} \]
Example 2
Ferris Wheel
Scenario: A Ferris wheel with a radius of 10 meters rotates at a constant angular velocity of 0.5rad/s². Calculate the linear velocity of a seat on the Ferris wheel.
Solution: \[ \text{Using } v = r\omega: \] \[ v = 10 \times 0.5 = 5 \, \text{m/s} \]
Example 3
Angular to Linear Acceleration
Scenario: A point on the rim of a rotating wheel with a radius of 0.5 meters experiences an angular acceleration of 4rad/s². Calculate the linear acceleration of the point.
Solution: \[ \text{Using } a = r\alpha: \] \[ a = 0.5 \times 4 = 2 \, \text{m/s}^2 \]
Example 4
Relationship Between Linear and Angular Quantities
Scenario: A car tire has a radius of 0.3 meters and rotates at an angular velocity of 10 rad/s. Calculate the linear speed of a point on the edge of the tire.
Solution: v =rω = 0.3m×10rad/s = 3m/s
Example 5
Angular Displacement
Scenario: A wheel rotates from an angular position of 0 radians to π radians in 2 seconds. Calculate the angular displacement.
Solution: θ = θբ−θᵢ = π−0 =πradians
Practice Problems
Question 1
A wheel starts from rest and has an angular acceleration of 2rad/s². What is its angular velocity after 5 seconds?
A) 5rad/s
B) 10rad/s
C) 15rad/s
D) 20rad/s
Answer:
D) 20rad/s
Explanation:
The angular velocity (ω) can be found using the equation of motion for rotational kinematics: ω=ω₀+αt
Where:
- ω₀ is the initial angular velocity (which is 0 since the wheel starts from rest),
- α is the angular acceleration (2rad/s²),
- t is the time (5 seconds).
Substituting the given values: ω = 0+(2rad/s²×5s) = 10rad/s
Thus, the angular velocity after 5 seconds is 10rad/s.
Question 2
A flywheel rotating at 60rad/s is brought to rest with a constant angular deceleration of 5rad/s². How much time does it take to stop?
A) 8 seconds
B) 10 seconds
C) 12 seconds
D) 15 seconds
Answer:
B) 12 seconds
Explanation:
The time (t) required to bring the flywheel to rest can be calculated using the following equation: ω=ω₀+αt
Here, ω is the final angular velocity (which is 0 since the wheel stops), ω₀ is the initial angular velocity (60rad/s), and α is the angular deceleration (−5rad/s²).
Rearranging the equation to solve for t: 0 = 60rad/s+(−5rad/s²)⋅t
5t=60
\[ t = \frac{60}{5} = 12 \, \text{seconds} \]
Thus, it takes 12 seconds for the flywheel to stop.
Question 3
A disc with a radius of 0.5m undergoes an angular displacement of 4πrad. What is the linear distance traveled by a point on the edge of the disc?
A) 2m
B) 4m
C) 6m
D) 8m
Answer:
D) 8m
Explanation:
The linear distance (s) traveled by a point on the edge of the disc can be found using the relationship between angular displacement (θ) and linear distance: s=rθ
Where:
- r is the radius of the disc (0.5m),
- θ is the angular displacement (4πrad).
Substituting the given values: s = 0.5m × 4πrad
s = 2πm
Approximating π ≈ 3.14:
s ≈ 2×3.14 = 6.28m
Thus, the linear distance traveled by a point on the edge of the disc is approximately 8m.