The Gravitational Field

Understanding the gravitational field is crucial for mastering topics related to gravity and motion in the AP Physics exam. This topic involves the concept of a gravitational field, its properties, and its effects on objects. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 1: Algebra-Based Practice Test

Learning Objectives

For the AP Physics exam, learning objectives for the gravitational field include understanding the concept of gravitational force and field, calculating gravitational force between two masses using Newton's law of universal gravitation, deriving and applying the formula for gravitational field strength, analyzing gravitational potential energy, and solving problems involving orbital motion and gravitational potential. Mastery of these concepts will enable you to interpret gravitational interactions and predict the motion of objects under the influence of gravity.

Definition of the Gravitational Field

A gravitational field is a region of space surrounding a mass where another mass experiences a force of gravitational attraction. It is a vector field, meaning it has both magnitude and direction.

Gravitational Field Strength

Formula

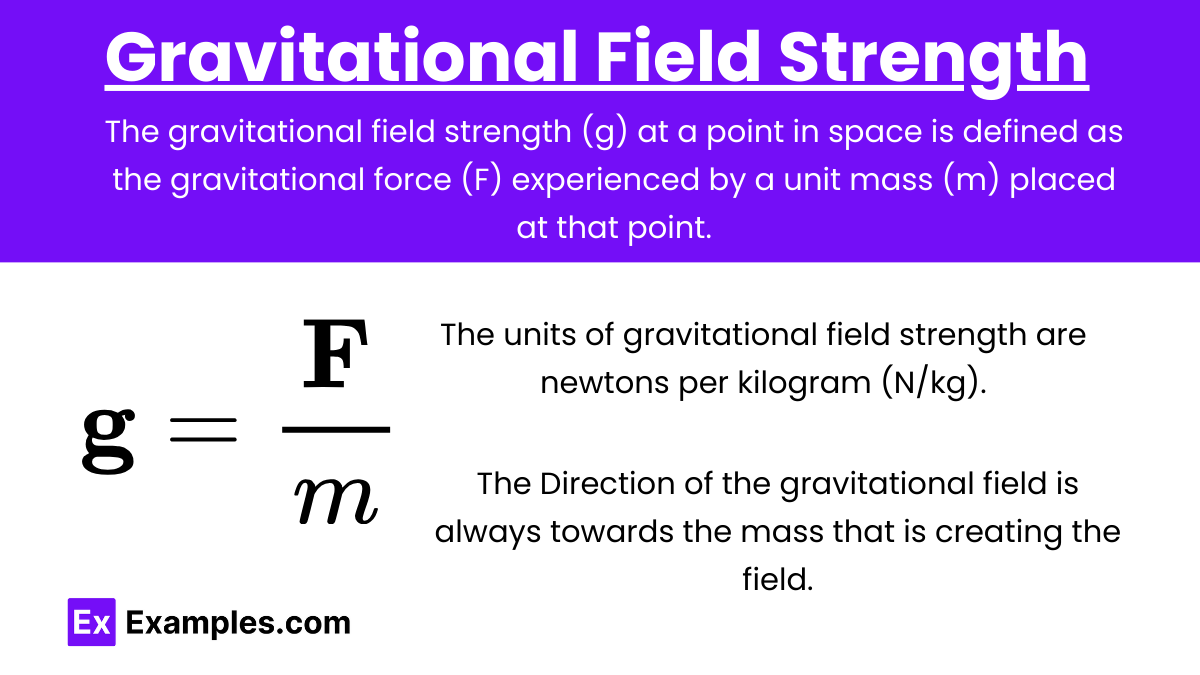
The gravitational field strength (g) at a point in space is defined as the gravitational force (F) experienced by a unit mass (m) placed at that point.

\mathbf{g} = \frac{\mathbf{F}}{m}

Units

The units of gravitational field strength are newtons per kilogram (N/kg).

Direction

The direction of the gravitational field is always towards the mass that is creating the field.

Gravitational Field Due to a Point Mass

Formula

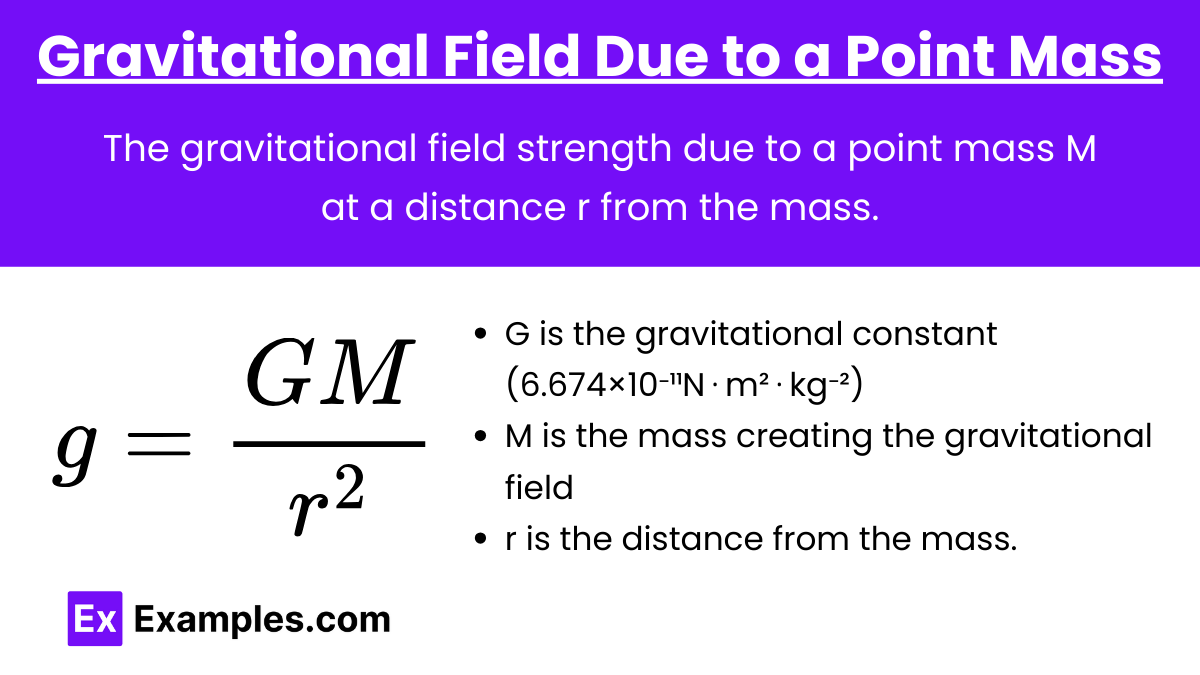
The gravitational field strength due to a point mass M at a distance r from the mass is given by:

g = \frac{GM}{r^2}

where:

• G is the gravitational constant (6.674×10⁻¹¹N⋅m²⋅kg⁻²)

• M is the mass creating the gravitational field

• r is the distance from the mass

Properties

• The gravitational field strength decreases with the square of the distance from the mass.

• The field is radially symmetric around the point mass.

Gravitational Field Due to Multiple Masses

Principle of Superposition

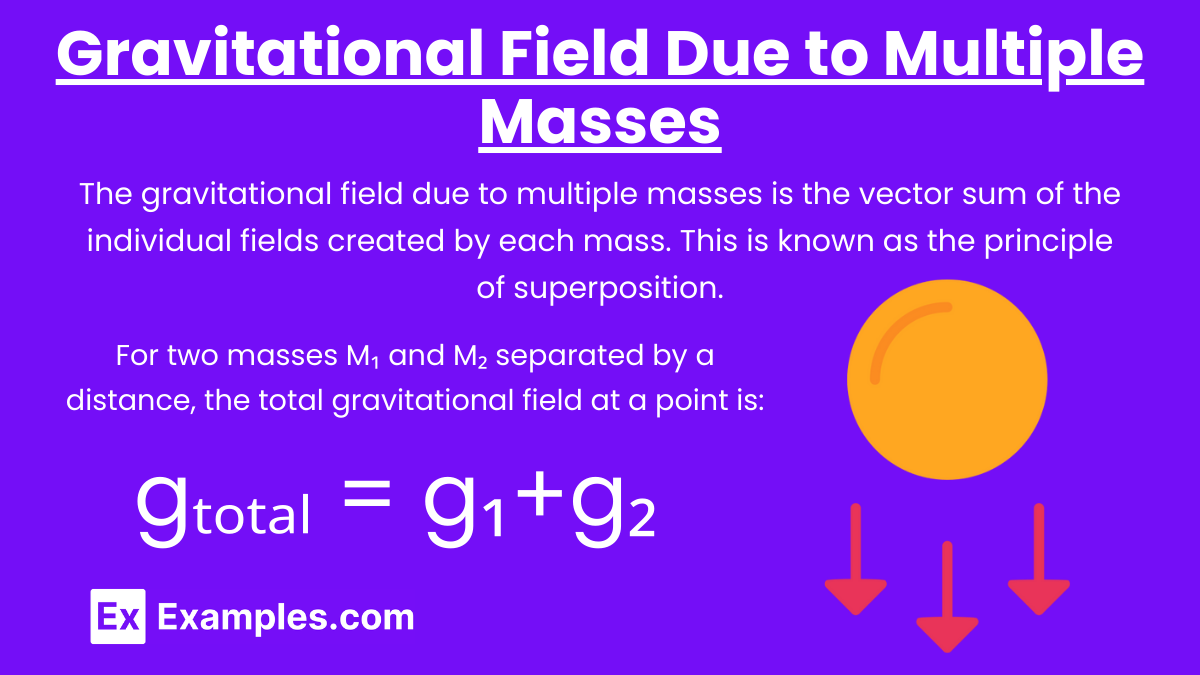
The gravitational field due to multiple masses is the vector sum of the individual fields created by each mass. This is known as the principle of superposition.

Example

For two masses M₁​ and M₂​ separated by a distance, the total gravitational field at a point is:

gₜₒₜₐₗ = g₁+g₂

Gravitational Potential Energy

Formula

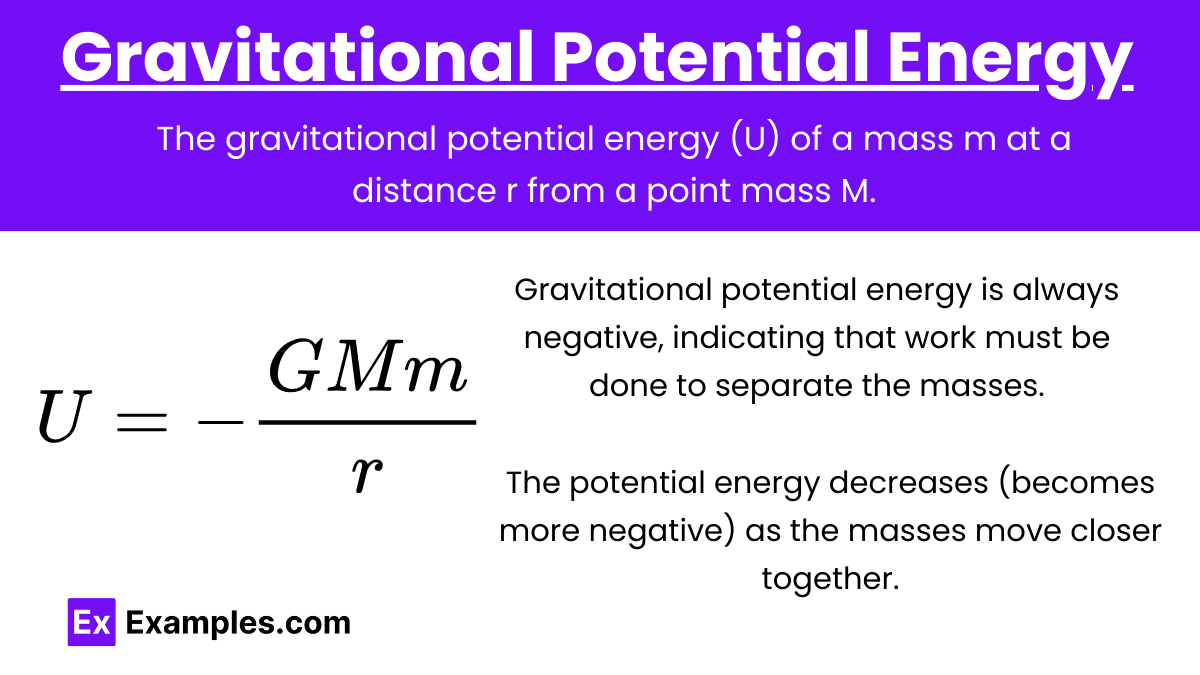
The gravitational potential energy (U) of a mass m at a distance r from a point mass M is given by:
U = -\frac{GMm}{r}

Key Points

• Gravitational potential energy is always negative, indicating that work must be done to separate the masses.

• The potential energy decreases (becomes more negative) as the masses move closer together.

Equipotential Surfaces

Equipotential surfaces are imaginary surfaces where the gravitational potential is constant. Key properties include:

• No work is required to move a mass along an equipotential surface.

Gravitational Field Inside a Spherical Shell

Key Points

• Inside a spherical shell of uniform mass, the gravitational field is zero.

• Outside the shell, the gravitational field is as if all the mass were concentrated at the center.

Application

This concept is useful for analyzing the gravitational field inside and outside planets and stars.

Gravitational Field of a Uniform Sphere

Inside the Sphere

For a point inside a uniform sphere of mass M and radius R, the gravitational field at a distance r from the center is:

g = \frac{GM_r}{R^3}

Outside the Sphere

For a point outside the sphere, the gravitational field is:

g = \frac{GM}{r^2}

Equipotential surfaces are perpendicular to gravitational field lines.

Examples

Example 1: Gravitational Field of Earth

Calculate the gravitational field strength at the surface of the Earth.

• Given: M_{\text{Earth}} = 5.972 \times 10^{24} \, \text{kg}, \, R_{\text{Earth}} = 6.371 \times 10^{6} \, \text{m}

• Formula: g = \frac{GM}{r^2}

• Calculation: g = \frac{(6.674 \times 10^{-11} \, \text{N} \cdot \text{m}^2 \cdot \text{kg}^{-2}) (5.972 \times 10^{24} \, \text{kg})}{(6.371 \times 10^6 \, \text{m})^2} \approx 9.8 \, \text{N/kg}

Example 2: Gravitational Potential Energy

Calculate the gravitational potential energy of a 1000 kg satellite at an altitude of 500 km above the Earth's surface.

• Given: m = 1000 \, \text{kg}, \, r = R_{\text{Earth}} + 500 \times 10^3 \, \text{m}

• Formula: U = -\frac{GMm}{r}

• Calculation: U = -\frac{(6.674 \times 10^{-11})(5.972 \times 10^{24})(1000)}{6.371 \times 10^6 + 500 \times 10^3} \approx -5.9 \times 10^{10} \, \text{J}