Buoyancy

Last Updated: September 24, 2024

Notes

Understanding buoyancy is crucial for mastering the principles of fluid mechanics and achieving a high score on the AP Physics exam. Buoyancy deals with the forces acting on objects submerged in fluids. Below are detailed notes on buoyancy along with five examples to help you excel in this topic.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

Understand the principle of buoyancy, including Archimedes’ principle, and apply it to solve problems involving objects submerged in fluids. Learn to calculate buoyant force and determine whether objects float or sink based on their density relative to the fluid. Explore the relationship between fluid displacement and buoyant force. Develop proficiency in solving related AP Physics exam problems, such as determining the apparent weight of objects in fluids and analyzing equilibrium conditions for floating objects.

Buoyancy

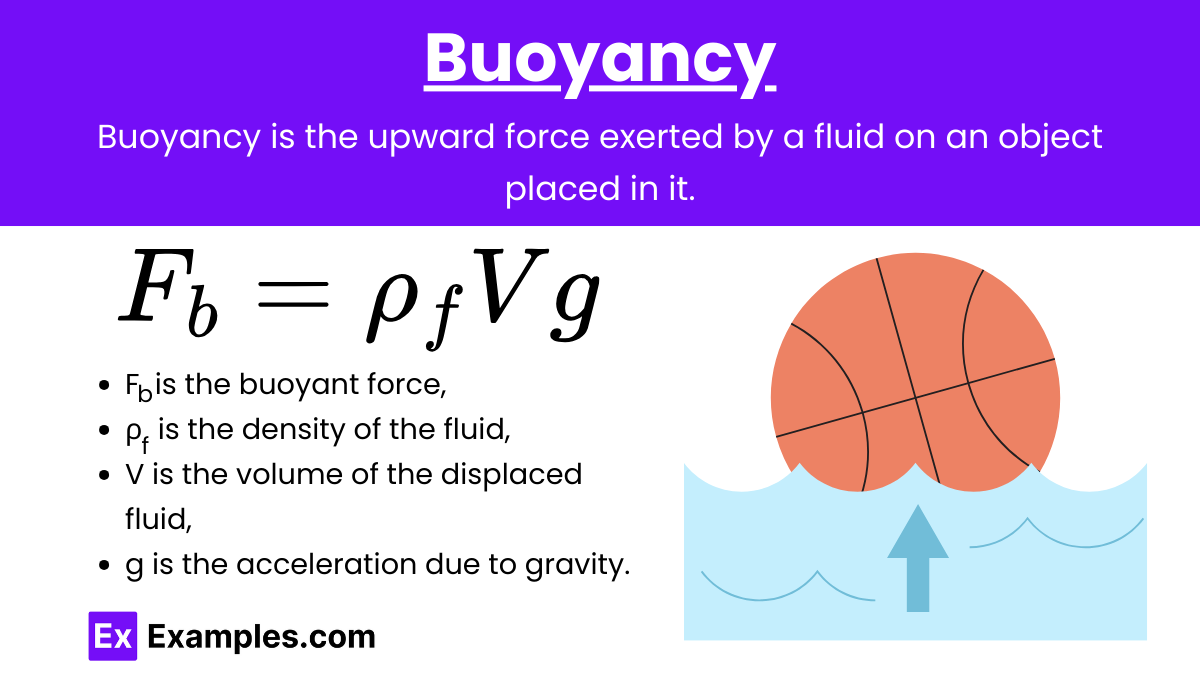
Buoyancy: Buoyancy is the upward force exerted by a fluid on an object placed in it. This force opposes the weight of the object and is responsible for making objects float or sink.

Archimedes’ Principle: An object submerged in a fluid experiences an upward buoyant force equal to the weight of the fluid displaced by the object.

Buoyant Force ( Fb):

$F_b = \rho_f V_f g$

where:

Fb is the buoyant force,
ρ is the density of the fluid,
V is the volume of the fluid displaced,
g is the acceleration due to gravity.

Key Points:

If the buoyant force is greater than the object’s weight, the object floats.
If the buoyant force is less than the object’s weight, the object sinks.
If the buoyant force equals the object’s weight, the object remains neutrally buoyant.

Factors Affecting Buoyancy

Density of the Fluid: The greater the density of the fluid, the greater the buoyant force.
Volume of the Object: The larger the volume of the object, the more fluid it displaces, leading to a greater buoyant force.
Density of the Object: Objects with density less than the fluid will float, while those with higher density will sink.

Applications of Buoyancy

Ships and Submarines: Designed to float by displacing water equal to their weight.
Hot Air Balloons: Float because hot air inside the balloon is less dense than the surrounding cooler air.
Hydrometers: Measure the density of liquids by floating at different levels based on fluid density.

Examples of Buoyancy

Example 1: Floating Object

Scenario: A 2 kg wooden block with a density of 400 kg/m³ is placed in water (density 1000 kg/m³). Determine if the block will float or sink and calculate the buoyant force.

Solution:

$\text{Volume of the block } (V_{\text{block}}) = \frac{\text{mass}}{\text{density}} = \frac{2 \, \text{kg}}{400 \, \text{kg/m}^3} = 0.005 \, \text{m}^3$

$\text{Since the block is less dense than water, it will float. To find the buoyant force:}$

$F_b = \rho_f V_f g = (1000 \, \text{kg/m}^3)(0.005 \, \text{m}^3)(9.8 \, \text{m/s}^2) = 49 \, \text{N}$

Example 2: Sinking Object

Scenario: A 1 kg metal cube with a density of 8000 kg/m³ is placed in water. Determine if the cube will float or sink and calculate the buoyant force.

Solution:

$\text{Volume of the cube } (V_{\text{cube}}) = \frac{\text{mass}}{\text{density}} = \frac{1 \, \text{kg}}{8000 \, \text{kg/m}^3} = 0.000125 \, \text{m}^3$

Since the cube is denser than water, it will sink. To find the buoyant force:

$F_b = \rho_f V_f g = (1000 \, \text{kg/m}^3)(0.000125 \, \text{m}^3)(9.8 \, \text{m/s}^2) = 1.225 \, \text{N}$

Example 3: Neutrally Buoyant Object

Scenario: A scuba diver’s equipment allows them to have the same density as seawater (density 1025 kg/m³). Calculate the volume of seawater displaced if the diver’s mass is 75 kg.

Solution:

$V_f = \frac{\text{mass}}{\text{density}} = \frac{75 \, \text{kg}}{1025 \, \text{kg/m}^3} = 0.07317 \, \text{m}^3$

Since the diver’s density matches the seawater’s density, they are neutrally buoyant and displace their own volume of water.

Example 4: Partially Submerged Object

Scenario: A 5 kg cylindrical log with a density of 600 kg/m³ is floating in freshwater. Calculate the fraction of the log’s volume that is submerged.

Solution:

$\frac{\text{density of log}}{\text{density of water}} = \frac{\rho_{\text{log}}}{\rho_{\text{water}}} = \frac{600 \, \text{kg/m}^3}{1000 \, \text{kg/m}^3} = 0.6$

So, 60% of the log’s volume is submerged.

Example 5: Buoyancy in Different Fluids

Scenario: A 10 kg object has a volume of 0.02 m³. Calculate the buoyant force on the object when it is submerged in oil (density 850 kg/m³) and in seawater (density 1025 kg/m³).

Solution: In Oil:

$F_b = \rho_{\text{oil}} V_f g = (850 \, \text{kg/m}^3)(0.02 \, \text{m}^3)(9.8 \, \text{m/s}^2) = 166.6 \, \text{N}$

In Seawater:

$F_b = \rho_{\text{seawater}} V_f g = (1025 \, \text{kg/m}^3)(0.02 \, \text{m}^3)(9.8 \, \text{m/s}^2) = 200.5 \, \text{N}$

The object experiences a greater buoyant force in seawater than in oil.

Practice Problems

Question 1:

An object is fully submerged in water and experiences a buoyant force equal to its weight. What will happen to the object?

A) It will sink.
B) It will float just below the water surface.
C) It will rise to the surface and float.
D) It will remain at its current depth.

Answer: D) It will remain at its current depth.

Explanation:

The buoyant force acting on a submerged object is given by Archimedes’ principle, which states that the buoyant force is equal to the weight of the fluid displaced by the object. When the buoyant force equals the weight of the object, the forces are balanced, and the object remains in equilibrium. Since the forces are equal and opposite, the object will remain at its current depth without rising or sinking.

Question 2:

A piece of wood floats on water with 70% of its volume submerged. What is the density of the wood?

A) 0.3 times the density of water
B) 0.5 times the density of water
C) 0.7 times the density of water
D) 1.0 times the density of water

Answer: C) 0.7 times the density of water

Explanation:

The principle of flotation states that an object floats in a fluid if its density is less than the density of the fluid. The fraction of the object’s volume submerged is equal to the ratio of the object’s density to the fluid’s density.

Let ρwood be the density of the wood and ρwater be the density of water.
Given that 70% of the wood’s volume is submerged:

$\frac{\rho_{\text{wood}}}{\rho_{\text{water}}} = 0.7$

Thus, the density of the wood is 0.7 times the density of water.

Question 3:

A solid sphere made of material with density 800 kg/m³ is dropped into a tank of liquid with density 1000 kg/m³. What will happen to the sphere?

A) It will sink to the bottom.
B) It will float on the surface.
C) It will remain submerged at a constant depth.
D) It will oscillate up and down.

Answer: B) It will float on the surface.

Explanation:

The buoyant force acting on an object in a fluid depends on the density of the fluid and the volume of the fluid displaced by the object. If the density of the object is less than the density of the fluid, the object will float.

Given:

$\text{Density of the sphere } (\rho_{\text{sphere}}) = 800 \, \text{kg/m}^3$

$\text{Density of the liquid } (\rho_{\text{liquid}}) = 1000 \, \text{kg/m}^3$

Since ρₛₚₕₑᵣₑ<ρliquid, the sphere will experience a buoyant force greater than its weight, causing it to float on the surface of the liquid.

Thus, the correct answer is that the sphere will float on the surface.