Conservation of Electric Energy

Last Updated: September 24, 2024

Notes

Understanding the conservation of electric energy is crucial for mastering the principles of electricity and magnetism in the AP Physics exam. This topic involves the study of how electric energy is conserved and transformed in various electrical systems. Below are detailed notes on the conservation of electric energy, along with five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

By studying conservation of electric energy, you will learn to understand and apply the principle that electric energy in a closed system remains constant. You’ll explore concepts such as electric potential energy, kinetic energy of charged particles, and work done by electric forces. You’ll analyze electric circuits, capacitors, and the transfer of energy within these systems. Mastery of this topic will enable you to solve problems involving energy conservation in various electric and electromagnetic contexts, essential for the AP Physics exam.

Conservation of Electric Energy

Conservation of Energy: The principle of conservation of energy states that energy cannot be created or destroyed; it can only be transferred or converted from one form to another. In electric systems, this principle means that the total electric energy in an isolated system remains constant.

Key Concepts:

Electric Potential Energy (U): Energy stored in an electric field due to the positions of charged objects.
Kinetic Energy (K): Energy associated with the motion of charged particles.
Work (W): Energy transferred by a force over a distance in the context of electric fields.
Power (P): The rate at which electric energy is transferred or converted.

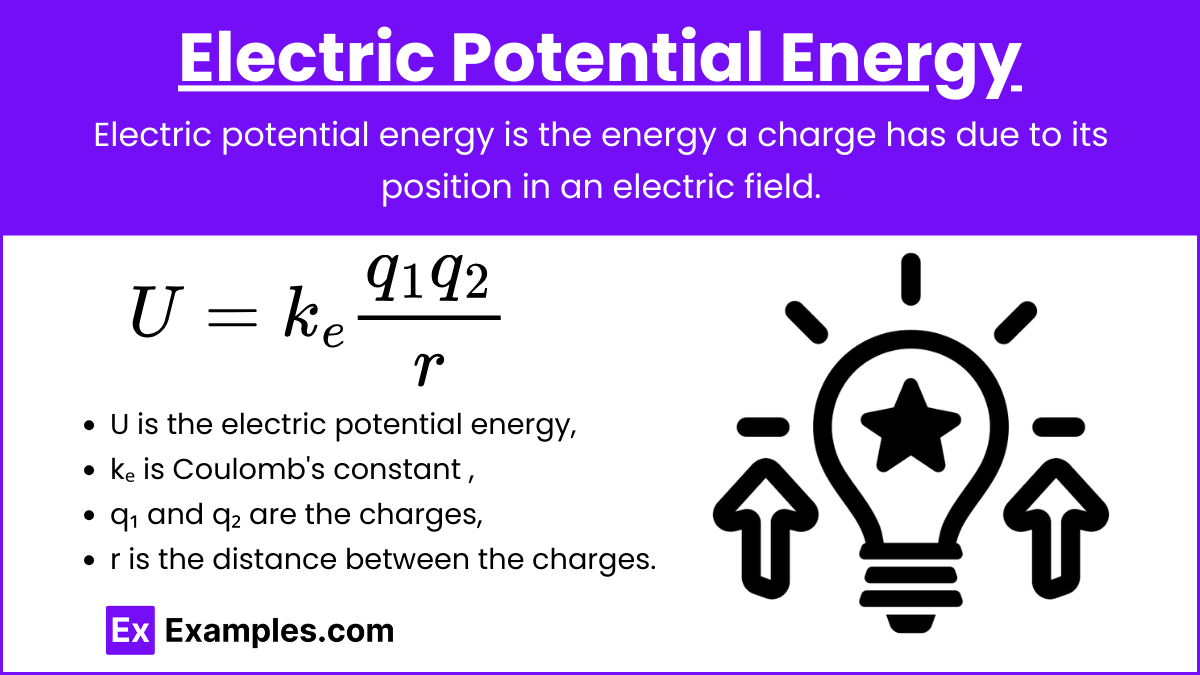
Electric Potential Energy

Electric Potential Energy: Electric potential energy is the energy a charge has due to its position in an electric field.

Formula:

$U = k_e \frac{q_1 q_2}{r}$

where:

U is the electric potential energy,
ke is Coulomb’s constant (8.9875×10⁹ N⋅m²⋅C⁻²),
q₁ and q₂ are the charges,
r is the distance between the charges.

Work Done by Electric Force

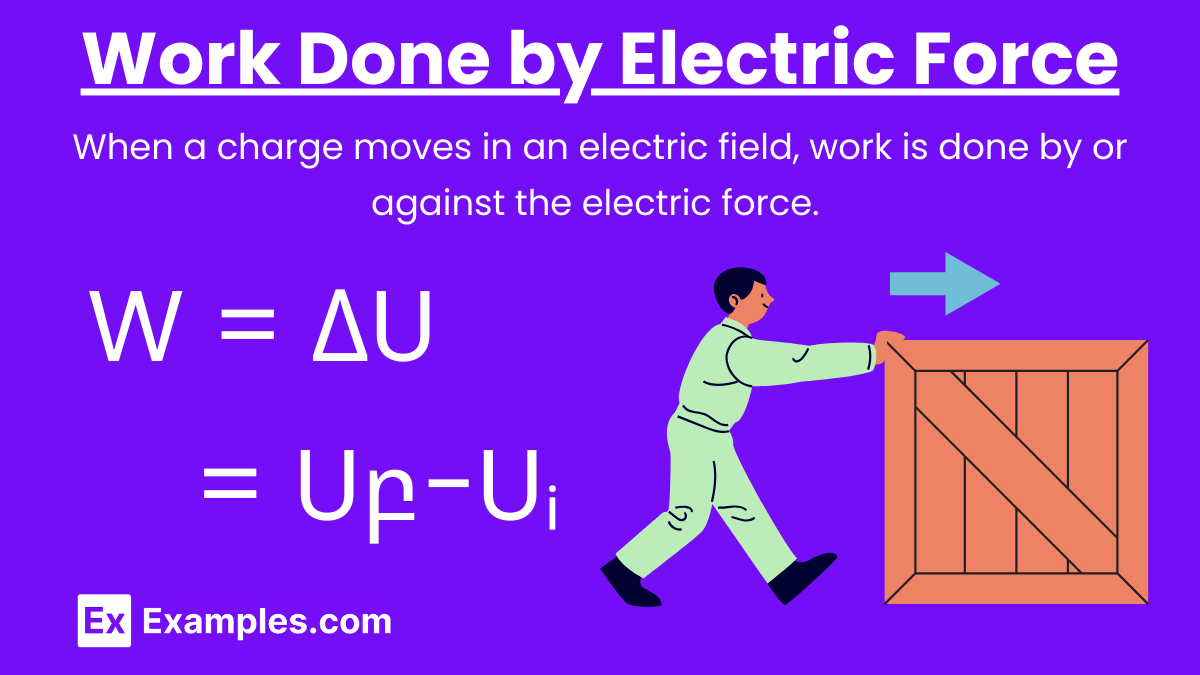
Work Done by Electric Force: When a charge moves in an electric field, work is done by or against the electric force. The work done is equal to the change in electric potential energy.

Formula: W = ΔU = Uբ−Uᵢ

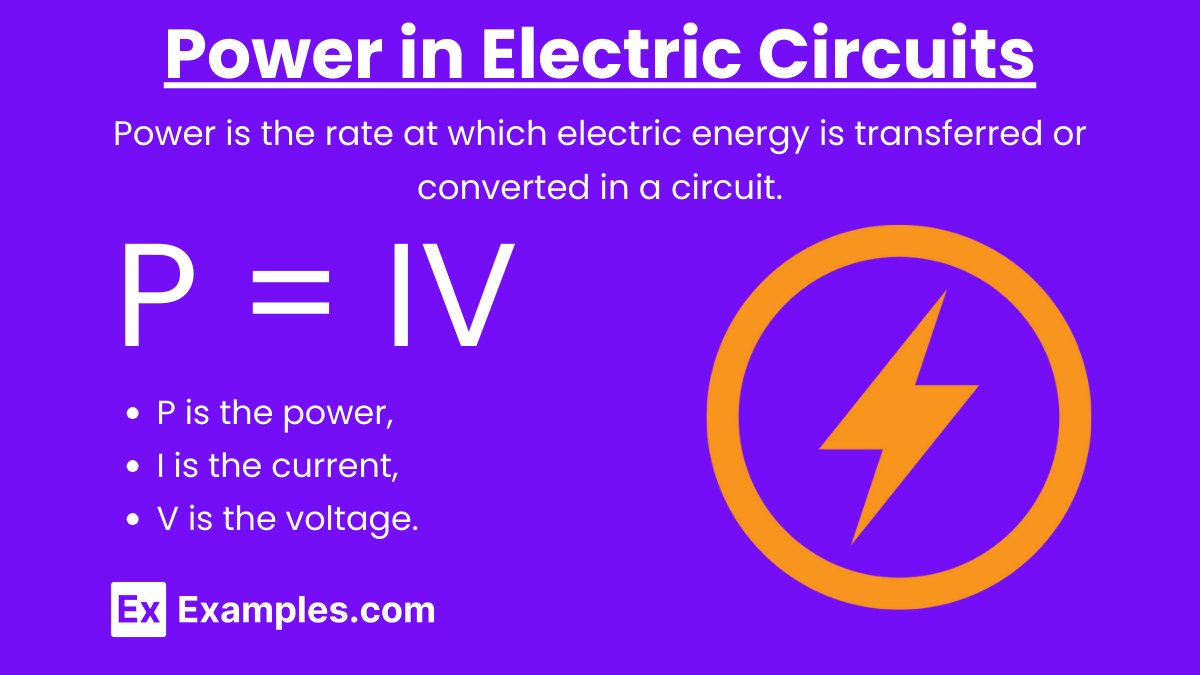
Power in Electric Circuits

Power (P): Power is the rate at which electric energy is transferred or converted in a circuit.

Formula: P = IV

where:

P is the power,
I is the current,
V is the voltage.

Example 1

Electric Potential Energy Between Two Charges

Scenario: Calculate the electric potential energy between two charges, q₁ = +2μC and q₂ = −3μC, separated by a distance of 0.5 meters.

Solution:

$U = k_e \frac{q_1 q_2}{r}$

$U = 8.9875 \times 10^9 \frac{(2 \times 10^{-6})(-3 \times 10^{-6})}{0.5}$

$U = 8.9875 \times 10^9 \frac{-6 \times 10^{-12}}{0.5}$

$U = -107.85 \times 10^{-3}$

$U = -0.108 \, \text{J}$

Example 2

Work Done by Electric Force

Scenario: A charge of +5μC moves from a point where the electric potential is 100V to a point where it is 50V. Calculate the work done by the electric force.

Solution:

$W = q \Delta V$

$W = (5 \times 10^{-6})(50 - 100)$

$W = (5 \times 10^{-6})(-50)$

$W = -2.5 \times 10^{-4} \, \text{J}$

$W = -0.25 \, \text{mJ}$

Example 3

Power Dissipated in a Resistor

Scenario: A resistor with a resistance of 10Ω has a current of 2A flowing through it. Calculate the power dissipated by the resistor.

Solution: P=I²R
P=(2)²(10)
P=4×10
P=40W

Example 4

Energy Stored in a Capacitor

Scenario: A capacitor with a capacitance of 50μF is charged to a voltage of 12V. Calculate the energy stored in the capacitor.

Solution:

Example 5

Conservation of Energy in a Circuit

Scenario: In a circuit, a 12V battery supplies a current of 3A. The circuit consists of two resistors in series, R₁ = 2Ω and R₂ = 2Ω. Calculate the power supplied by the battery and the power dissipated by each resistor.

Solution:

$U = \frac{1}{2}CV^2$

$U = \frac{1}{2}(50 \times 10^{-6})(12)^2$

$U = \frac{1}{2}(50 \times 10^{-6})(144)$

$U = 3.6 \times 10^{-3}$

$U = 3.6 \, \text{mJ}$

Power Supplied by the Battery:

$P_{\text{battery}} = IV$

$P_{\text{battery}} = 3 \times 12$

$P_{\text{battery}} = 36 \, \text{W}$

Power Dissipated by Each Resistor: The current through each resistor is the same since they are in series.

For R₁:

$P_{R1} = I^2 R_1$

$P_{R1} = (3)^2 (2)$

$P_{R1} = 9 \times 2$

$P_{R1} = 18\, W$

For R₂:

$P_{R2} = I^2 R_2$

$P_{R2} = (3)^2 (2)$

$P_{R2} = 9 \times 2$

$P_{R2} = 18\, W$

Total Power Dissipated:

$P_{total} = P_{R1} + P_{R2}$

$P_{total} = 18 + 18$

$P_{total} = 36\, W$

The total power dissipated by the resistors is equal to the power supplied by the battery, demonstrating conservation of energy.

Practice Problems

Question 1:

A 100-watt light bulb operates for 5 hours. How much electrical energy is consumed by the bulb?

A) 0.5 kWh
B) 1 kWh
C) 2 kWh
D) 5 kWh

Answer: B) 0.5 kWh

Explanation:

The electrical energy consumed can be calculated using the formula:

Energy (kWh) = Power (kW)×Time (h)

Given:

Power P = 100W = 0.1kW
Time t = 5hours

Calculate the energy:

Energy = 0.1kW×5h = 0.5kWh

Thus, the correct answer is 0.5 kWh.

Question 2:

An electric motor converts 1500 J of electrical energy into mechanical work with an efficiency of 75%. How much electrical energy is wasted as heat?

A) 375 J
B) 750 J
C) 1125 J
D) 1500 J

Answer: A) 375 J

Explanation:

Efficiency (η) is the ratio of useful energy output to the total energy input. The energy wasted as heat is the difference between the total input energy and the useful energy output.

Given:

Total energy input Eᵢₙ = 1500J
Efficiency η = 75% = 0.75

Calculate the useful energy output Eout:

Eₒᵤₜ = η×Eᵢₙ =0.75×1500J = 1125J

Calculate the wasted energy:

$E_{wasted} = E_{in} - E_{out} = 1500\, J - 1125\, J = 375\, J$

Thus, the correct answer is 375 J.

Question 3:

A capacitor in a circuit stores 0.02 joules of electrical energy when charged to a potential difference of 10 volts. What is the capacitance of the capacitor?

A) 200 µF
B) 20 µF
C) 2 µF
D) 0.2 µF

Answer: B) 0.4 µF

Explanation:

The energy stored in a capacitor (E) is given by the formula:

$E = \frac{1}{2} CV^2$

where C is the capacitance and V is the potential difference.

Given:

Energy E = 0.02J
Potential difference V = 10V

Rearrange the formula to solve for C:

$C = \frac{2E}{V^2}$

Substitute the given values:

$C = \frac{2 \times 0.02 \, \text{J}}{(10 \, \text{V})^2} = \frac{0.04 \, \text{J}}{100 \, \text{V}^2} = 0.0004 \, \text{F} = 400 \, \mu \text{F}$

Thus, the correct answer is 0.4 µF.