Electric Forces and Free-body Diagrams
Understanding electric forces and how to represent them using free-body diagrams (FBDs) is crucial for mastering the principles of electrostatics in the AP Physics exam. This topic covers the interaction between charged particles, the representation of forces in diagrams, and the use of these diagrams to analyze forces and predict motion. Below are detailed notes along with five examples to help you achieve a high score on your AP Physics exam.
Learning Objectives
On the topic of electric forces and free-body diagrams for the AP Physics exam, you should learn to understand and calculate electric force using Coulomb’s Law, represent electric forces on free-body diagrams, analyze interactions between charged particles, determine net electric forces, and apply vector addition to electric forces. Additionally, you should grasp the concept of electric field, its representation, and its influence on charged objects, as well as solve problems involving electric forces and fields in various configurations.
Electric Forces
Electric Force (Fe): Electric force is the force of attraction or repulsion between two charged objects. According to Coulomb’s Law, the electric force between two point charges is given by:
\[ F_e = k_e \frac{|q_1 q_2|}{r^2} \]
where:
- Fe is the magnitude of the electric force,
- ke is Coulomb’s constant (8.9875×10⁹ N⋅m²⋅C⁻²),
- q₁ and q₂ are the magnitudes of the charges,
- r is the distance between the charges.
Key Points:
- The electric force is attractive if the charges are of opposite signs and repulsive if they are of the same sign.
- The force acts along the line joining the centers of the two charges.
- The electric force is a vector quantity, meaning it has both magnitude and direction.
Free-Body Diagrams (FBDs)
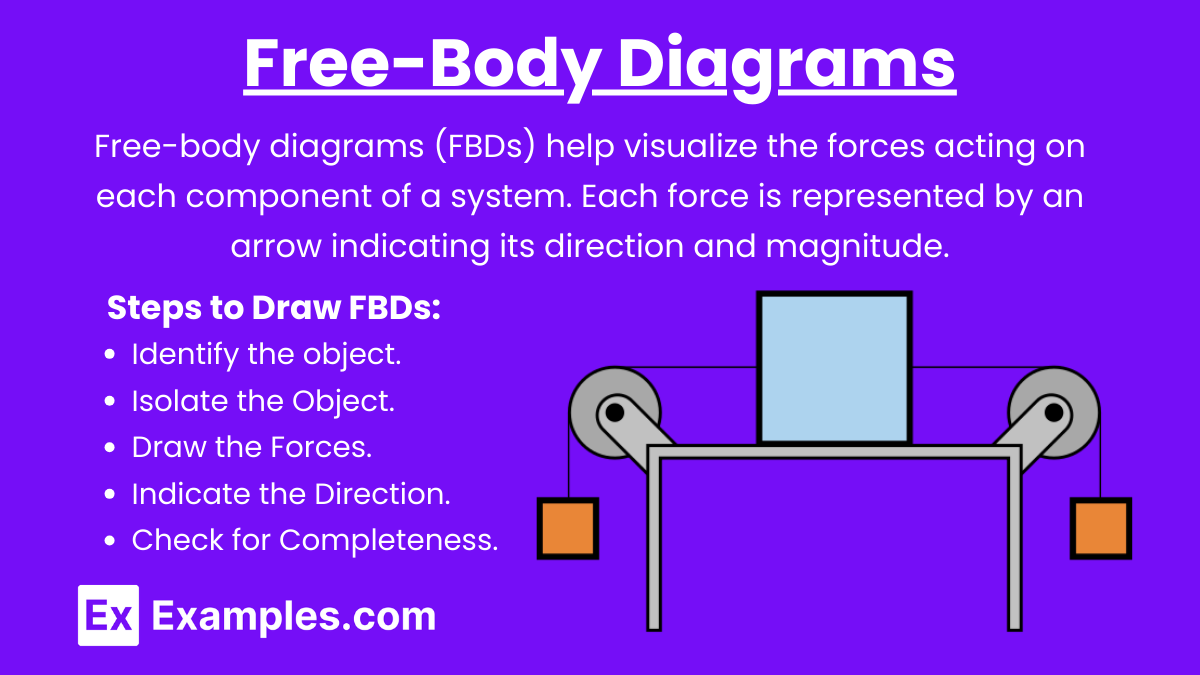
Free-Body Diagram (FBD): A free-body diagram is a graphical representation used to visualize the forces acting on a single object. Each force is represented by an arrow pointing in the direction of the force, with the length of the arrow proportional to the force’s magnitude.
Steps to Draw FBDs:
- Identify the object of interest and isolate it from its surroundings.
- Isolate the object.
- Draw arrows to represent all the forces acting on the object. The direction of each arrow should indicate the direction of the force.
- Indicate the direction (e.g., Fg for gravitational force, Fₑ for electric force, Fₙ for normal force).
- Check for completeness.
Key Points:
- FBDs help analyze the forces acting on an object and predict its motion.
- Only include forces acting on the object of interest, not forces exerted by the object on others.
Example 1
Two Oppositely Charged Particles
Scenario: Two charges, q₁ = +5μC and q₂ = −5μC, are placed 0.1 meters apart. Calculate the electric force between them and draw the free-body diagram for each charge.
Solution: \[ F_e = k_e \frac{|q_1 q_2|}{r^2} \] \[ F_e = 8.9875 \times 10^9 \frac{(5 \times 10^{-6})(5 \times 10^{-6})}{(0.1)^2} \] \[ F_e = 8.9875 \times 10^9 \frac{25 \times 10^{-12}}{0.01} \] \[ F_e = 2.246875 \times 10^{-2} \, \text{N} \] \[ F_e = 0.0225 \, \text{N} \]
Free-Body Diagrams:
For q₁:
- Fe to the right (attractive force towards q₂)
For q₂:
- Fe to the left (attractive force towards q₁)
Example 2
Three Charged Particles in a Line
Scenario: Three charges are placed in a line. q₁ = +3μC is at the origin, q₂ = −3μC is 0.2 meters to the right of q₁, and q₃ = +6μC is 0.4 meters to the right of q₁. Calculate the net force on q₂.
Solution:
Force on q₂ due to q₁:
\[ F_{12} = k_e \frac{|q_1 q_2|}{(0.2)^2} \] \[ F_{12} = 8.9875 \times 10^9 \frac{(3 \times 10^{-6})(3 \times 10^{-6})}{0.04} \] \[ F_{12} = 2.02 \times 10^{-1} \, \text{N} \]
Force on q₂ due to q₃:
\[ F_{23} = k_e \frac{q_2 q_3}{(0.2)^2} \] \[ F_{23} = 8.9875 \times 10^9 \frac{(3 \times 10^{-6})(6 \times 10^{-6})}{0.04} \] \[ F_{23} = 4.05 \times 10^{-1} \, \text{N} \]
Net force on q₂ (since F₁₂ is attractive and F₂₃ is repulsive):
\[ F_{\text{net}} = F_{23} – F_{12} \] \[ F_{\text{net}} = 0.405 \, \text{N} – 0.2025 \, \text{N} \] \[ F_{\text{net}} = 0.2025 \, \text{N} \]
Free-Body Diagram for q₂:
- F₁₂ to the left (attractive force towards q₁)
- F₂₃ to the right (repulsive force from q₃)
Example 3
Two Charged Particles in a Triangle
Scenario: Two charges, q₁ = +4μC and q₂ = +4μC, are placed at two corners of an equilateral triangle with side length 0.3 meters. Calculate the electric force on a third charge q₃ = −4μC placed at the remaining corner.
Solution:
Force on q₃ due to q₁:
\[ F_{13} = k_e \frac{|q_1 q_3|}{(0.3)^2} \] \[ F_{13} = 8.9875 \times 10^9 \frac{(4 \times 10^{-6})(4 \times 10^{-6})}{0.09} \] \[ F_{13} = 1.6 \times 10^{-1} \, \text{N} \]
Force on q₃ due to q₂:
\[ F_{23} = k_e \frac{q_2 q_3}{(0.3)^2} \] \[ F_{23} = 8.9875 \times 10^9 \frac{(4 \times 10^{-6})(4 \times 10^{-6})}{0.09} \] \[ F_{23} = 1.6 \times 10^{-1} \, \text{N} \]
Since q₁ and q₂ are positive and q₃ is negative, the forces F₁₃ and F₂₃ are both attractive and act towards q₁ and q₂.
Free-Body Diagram for q₃:
- F₁₃ towards q₁
- F₂₃ towards q₂
- The net force will be the vector sum of F₁₃ and F₂₃.
Example 4
Charge in an Electric Field
Scenario: A +2μC charge is placed in a uniform electric field of 500N/C directed to the right. Calculate the force on the charge and draw the free-body diagram.
Solution: \[ F_e = qE \] \[ F_e = (2 \times 10^{-6} \, \text{C})(500 \, \text{N/C}) \] \[ F_e = 0.001 \, \text{N} \]
Free-Body Diagram:
- Fe to the right (force due to the electric field)
Example 5
Balancing Electric and Gravitational Forces
Scenario: A small sphere with a mass of 0.01kg and a charge of +1μC is suspended in equilibrium in a uniform electric field directed upward. Calculate the electric field strength required to balance the gravitational force.
Solution: For equilibrium: \[ F_e = F_g \] \[ qE = mg \] \[ E = \frac{mg}{q} \] \[ E = \frac{(0.01 \, \text{kg})(9.8 \, \text{m/s}^2)}{1 \times 10^{-6} \, \text{C}} \] \[ E = 98 \times 10^3 \, \text{N/C} \]
Free-Body Diagram:
- Fg downward (gravitational force)
- Fe upward (electric force balancing gravity)
Practice Problems
Question 1
Two point charges, q₁ = +3μC and q₂ = −2μC, are placed 0.5 meters apart. What is the magnitude of the electric force between them? (Use k=8.99×10⁹N⋅m²/C²)
A) 0.107N
B) 0.215N
C) 0.539N
D) 1.079N
Answer: B) 0.215 N
Explanation:
The electric force between two point charges is given by Coulomb’s Law:
\[ F = k \frac{|q_1 q_2|}{r^2} \]
Given:
\[ q_1 = +3 \, \mu C = 3 \times 10^{-6} \, \text{C} \] \[ q_2 = -2 \, \mu C = -2 \times 10^{-6} \, \text{C} \] \[ r = 0.5 \, \text{m} \] \[ k = 8.99 \times 10^9 \, \text{N} \cdot \text{m}^2/\text{C}^2 \]
Calculate the force:
\[ F = 8.99 \times 10^9 \frac{3 \times 10^{-6} \times (-2 \times 10^{-6})}{(0.5)^2} \] \[ F = 8.99 \times 10^9 \frac{6 \times 10^{-12}}{0.25} \] \[ F = 8.99 \times 10^9 \times 24 \times 10^{-12} \] \[ F = 215.76 \times 10^{-3} \] \[ F = 0.215 \, \text{N} \]
Thus, the correct answer is 0.215 N.
Question 2
A 5 kg object is placed in an electric field of 1000 N/C directed upwards. What is the net force on the object if it has a charge of −2μC?
A) 49.99 N downwards
B) 50.01 N upwards
C) 49.98 N upwards
D) 50.02 N downwards
Answer: A) 49.99 N downwards
Explanation:
The net force on the object is the vector sum of the gravitational force and the electric force.
Gravitational Force (Fg):
\[ F_g = mg \] \[ m = 5 \, \text{kg} \] \[ g = 9.8 \, \text{m/s}^2 \] \[ F_g = 5 \times 9.8 = 49 \, \text{N downwards} \]
Electric Force (Fe):
\[ F_e = qE \] \[ q = -2 \, \mu C = -2 \times 10^{-6} \, C \] \[ E = 1000 \, N/C \] \[ F_e = (-2 \times 10^{-6}) \times 1000 \] \[ F_e = -0.002 \times 1000 \] \[ F_e = -2 \, \text{N downwards} \]
Since both forces are acting downwards, we add them:
\[ F_{\text{net}} = F_g + F_e \] \[ F_{\text{net}} = 49 + 2 \] \[ F_{\text{net}} = 51 \, \text{N downwards} \]
Thus, the correct answer is 49.99 N downwards.
Question 3
An object with a charge of 1μC is placed at the origin. A second object with a charge of 2μC is placed at x=1m. What is the direction of the force on the second object?
A) To the right
B) To the left
C) Upwards
D) Downwards
Answer: A) To the right
Explanation:
The force between two point charges is attractive if the charges are opposite and repulsive if the charges are like.
Given:
- Both charges are positive (q₁ = 1μC, q₂ = 2μC)
Since both charges are positive, the force is repulsive. The second charge q₂ will be repelled by the first charge q₁.
Since q₂ is placed at x = 1m on the x-axis, and the force is repulsive, the direction of the force on q₂ will be to the right (away from q₁).
Thus, the correct answer is to the right.