)
Energy in Modern Physics (energy in radioactive decay and E=mc²)
Energy in modern physics, particularly in radioactive decay and Einstein’s E=mc² equation, is fundamental to understanding nuclear processes. In AP Physics, students explore how unstable atomic nuclei release energy during alpha, beta, and gamma decay, and learn to calculate the energy involved. Additionally, the equation E=mc² illustrates the profound relationship between mass and energy, highlighting its significance in nuclear reactions and various applications in physics.
Learning Objectives
Focus on understanding the types and processes of radioactive decay (alpha, beta, and gamma decay), the energy released during these decays, and how to calculate it. Additionally, grasp the significance of Einstein’s equation E=mc², including its implications for energy-mass equivalence and applications in nuclear reactions. Be able to solve problems related to these concepts and explain their relevance in modern physics.
Energy in Modern Physics
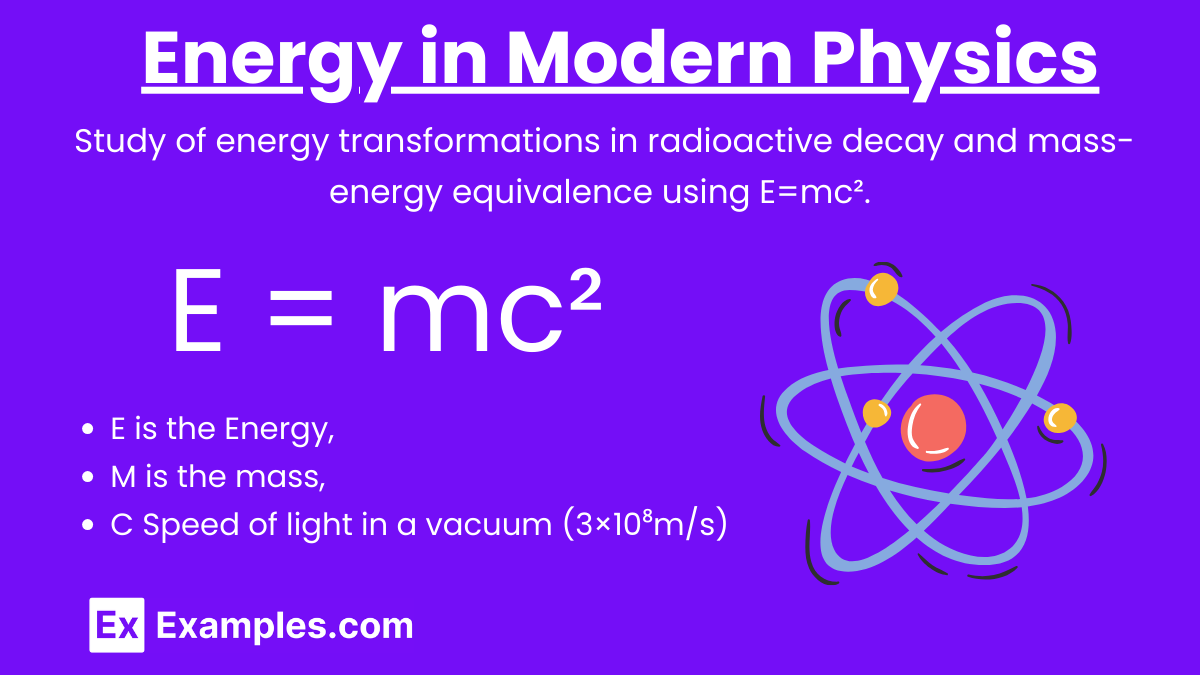
Energy plays a crucial role in modern physics, especially in the realms of radioactive decay and the famous equation E = mc² derived by Albert Einstein. These concepts form the bedrock of our understanding of nuclear physics and energy-mass equivalence.
Energy in Radioactive Decay
Radioactive decay involves the transformation of an unstable atomic nucleus into a more stable one. This process releases energy in the form of radiation. There are several types of radioactive decay, including alpha, beta, and gamma decay, each with distinct energy implications.
Alpha Decay
- Definition: An alpha particle (2 protons and 2 neutrons) is emitted from the nucleus.
- Energy Release: The energy released in alpha decay is typically around 4-8 MeV (Mega Electron Volts).
Example:
![\[ \text{U}^{238}_{92} \rightarrow \text{Th}^{234}_{90} + \text{He}^{4}_{2} + \text{Energy} \]](https://www.examples.com/wp-content/ql-cache/quicklatex.com-7ed499ade477553998c5256b73237117_l3.png "Rendered by QuickLaTeX.com")
Beta Decay
Definition: A beta particle (electron or positron) is emitted.
Types:
Beta-minus (β−) decay: A neutron transforms into a proton, an electron, and an antineutrino.
Beta-plus (β+) decay: A proton transforms into a neutron, a positron, and a neutrino.
Energy Release: The energy released can range from a few keV (kilo Electron Volts) to several MeV.
Example:
![\[ \text{C}^{14}_{6} \rightarrow \text{N}^{14}_{7} + \beta^- + \overline{\nu}_e + \text{Energy} \]](https://www.examples.com/wp-content/ql-cache/quicklatex.com-5272591dcf63ed77436f8519e181644d_l3.png "Rendered by QuickLaTeX.com")
Gamma Decay
- Definition: The emission of gamma rays (high-energy photons) from a nucleus.
- Energy Release: The energy of gamma rays typically ranges from keV to MeV.
- Example:
![\[ \text{Co}^{60}_{27} \rightarrow \text{Ni}^{60}_{28} + \gamma + \text{Energy} \]](https://www.examples.com/wp-content/ql-cache/quicklatex.com-6ba74425610fdec293864e718ba9c3b1_l3.png "Rendered by QuickLaTeX.com")
Energy Released in Radioactive Decay
- Binding Energy: The energy that holds the nucleus together. It is released during decay.
- Calculation: The energy released in decay can be calculated using the difference in binding energy before and after the decay.
Energy-Mass Equivalence: E=mc²
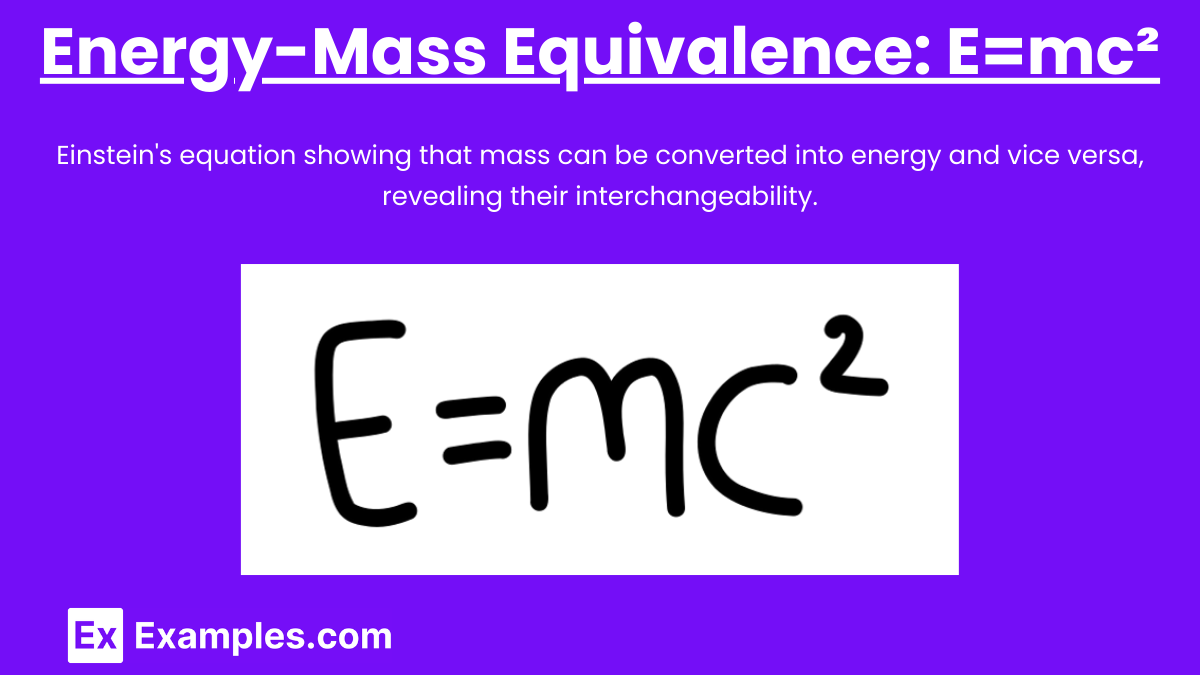
Albert Einstein’s equation E=mc² is a cornerstone of modern physics. It expresses the equivalence of mass and energy, indicating that mass can be converted into energy and vice versa.
Understanding E=mc²
- E: Energy
- m: Mass
- c: Speed of light in a vacuum (3×10⁸m/s)
Implications of E=mc²
- Energy from Mass: Even a small amount of mass can be converted into a tremendous amount of energy. For example, 1 gram of matter can theoretically produce 9×10¹³ joules of energy.
- Nuclear Reactions: In nuclear fission and fusion, mass is converted into energy. This principle underlies the energy produced by nuclear power plants and atomic bombs.
Applications of E=mc²
- Nuclear Power: Energy produced in nuclear reactors comes from the conversion of mass into energy through fission reactions.
- Particle Physics: Particle accelerators convert kinetic energy into mass to create new particles.
- Cosmology: The energy-mass equivalence plays a role in the dynamics of the universe, such as in the processes occurring in stars.
Example Calculation
Consider a nuclear reaction where 0.001 kg of mass is converted entirely into energy. Using E=mc²:
E=0.001 kg×(3×10⁸ m/s)²=9×10¹³ J
Examples of Energy in Modern Physics
- Uranium-238 Alpha Decay:
- Uranium-238 decays into Thorium-234 by emitting an alpha particle, releasing approximately 4.27 MeV of energy.
- Carbon-14 Beta Decay:
- Carbon-14 decays into Nitrogen-14 through beta-minus decay, emitting an electron and an antineutrino, with an energy release of about 0.156 MeV.
- Cobalt-60 Gamma Decay:
- Cobalt-60 decays into Nickel-60, emitting gamma rays with energies of 1.17 MeV and 1.33 MeV.
- Nuclear Fission of Uranium-235:
- In nuclear reactors, Uranium-235 undergoes fission when struck by a neutron, splitting into smaller nuclei and releasing about 200 MeV of energy.
- Mass-Energy Conversion in Particle Accelerators:
- High-energy particle collisions in accelerators, like those at CERN, convert kinetic energy into mass, creating new particles as predicted by E=mc².
Practice Test Questions On Conservation of Energy in Modern Physics
Question 1
Which type of radioactive decay involves the emission of a helium nucleus?
A) Beta-minus decay
B) Beta-plus decay
C) Alpha decay
D) Gamma decay
Answer: C) Alpha decay
Explanation: Alpha decay involves the emission of an alpha particle, which is essentially a helium nucleus consisting of 2 protons and 2 neutrons. This type of decay decreases the mass number of the parent nucleus by 4 and the atomic number by 2, resulting in a new element.
Question 2
What is the energy released when 0.001 kg of mass is completely converted into energy using the equation E=mc²?
A) 3×10⁵J
B) 3×10⁸J
C) 9×10¹⁰J
D) 9×10¹³J
Answer: D) 9×10¹³J
Explanation: Using the equation E=mc², where m is mass and c is the speed of light (3×10⁸ m/s)
E=0.001 kg×(3×10⁸ m/s)²=9×10¹³J
This shows the immense amount of energy that can be released from a small amount of mass.
Question 3
In the context of E=mc², which of the following statements is correct?
A) Energy can be created from nothing.
B) Mass and energy are interchangeable and can be converted into each other.
C) Only mass can be converted into energy, not vice versa.
D) E=mc² only applies to chemical reactions.
Answer: B) Mass and energy are interchangeable and can be converted into each other.
Explanation: The equation E=mc², derived by Albert Einstein, indicates that mass and energy are equivalent and can be transformed into each other. This principle is fundamental in nuclear physics and explains phenomena such as the energy released in nuclear reactions and the creation of particles in high-energy physics experiments.