Fluids and Free-Body Diagrams
Understanding fluids and how to represent forces using free-body diagrams is essential for mastering principles in AP Physics. This topic involves fluid statics and dynamics, as well as the use of free-body diagrams to analyze forces. Below are detailed notes to help you achieve a high score on your AP Physics exam.
Learning Objectives
In studying Fluids and Free-Body Diagrams for the AP Physics exam, you will learn to analyze fluid statics and dynamics, including concepts like buoyancy, pressure, and fluid flow. You will master the use of free-body diagrams to visualize forces acting on objects, applying Newton’s laws to solve problems involving equilibrium and motion. Key topics include Bernoulli’s principle, Archimedes’ principle, and the continuity equation, enhancing your problem-solving skills and conceptual understanding of fluid behavior and force analysis.
Fluids
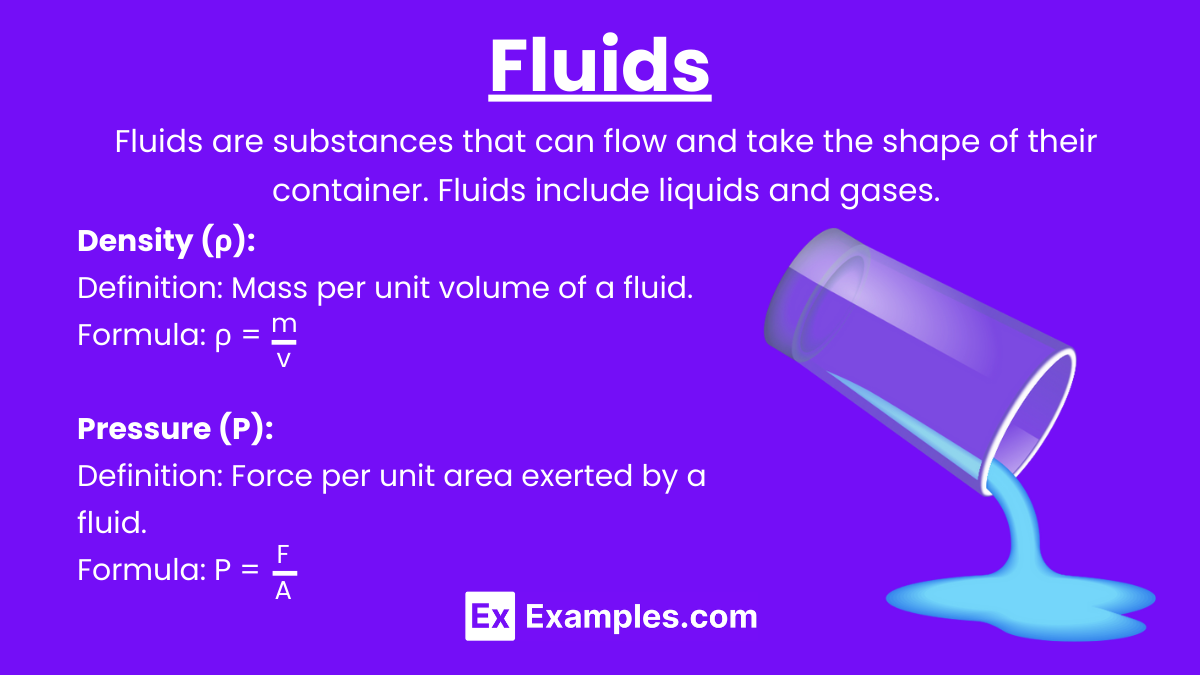
Fluids: Fluids are substances that can flow and take the shape of their container. Fluids include liquids and gases.
Properties of Fluids
- Density (ρ):
- Definition: Mass per unit volume of a fluid.
- Formula: \[ \rho = \frac{m}{V} \]
- Units: kg/m³
- Pressure (P):
- Definition: Force per unit area exerted by a fluid.
- Formula: \[ P = \frac{F}{A} \]
- Units: N/m² or Pascal (Pa)
Fluid Statics
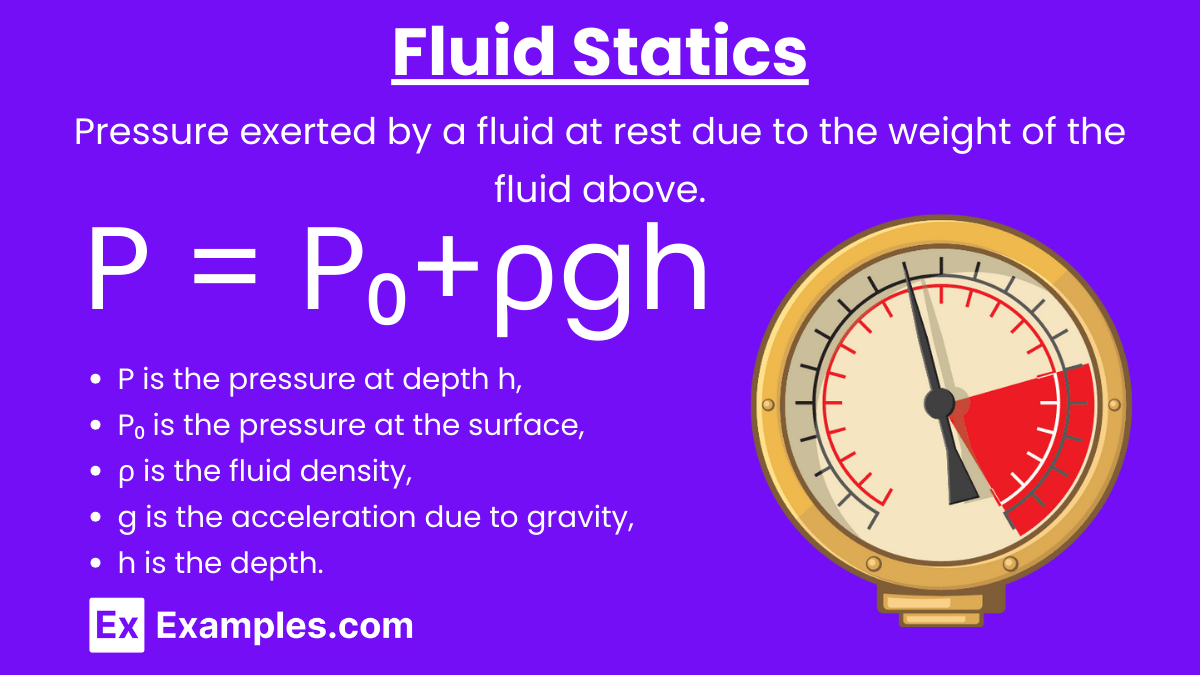
Hydrostatic Pressure: Pressure exerted by a fluid at rest due to the weight of the fluid above.
Formula: P = P₀+ρgh
where:
- P is the pressure at depth h,
- P₀ is the pressure at the surface,
- ρ is the fluid density,
- g is the acceleration due to gravity,
- h is the depth.
Key Points:
- Pressure increases with depth in a fluid.
- At any given depth, pressure is the same in all directions.
Pascal’s Principle: A change in pressure applied to an enclosed fluid is transmitted undiminished to all portions of the fluid and to the walls of its container.
Fluid Dynamics
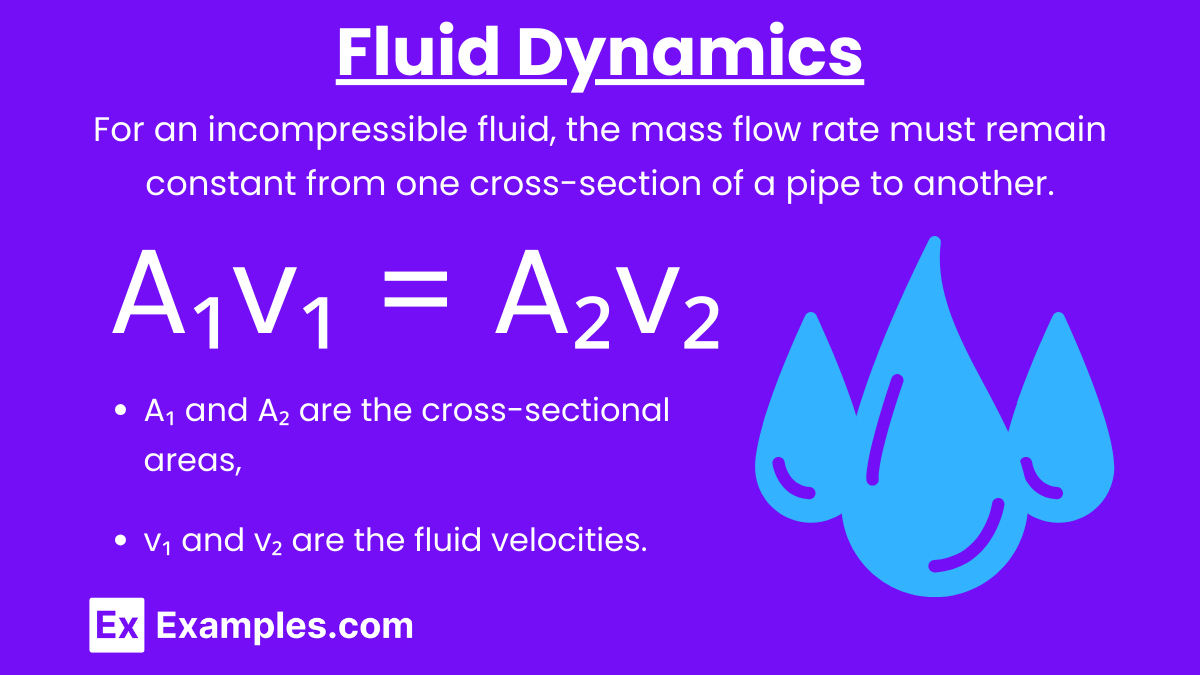
Continuity Equation: For an incompressible fluid, the mass flow rate must remain constant from one cross-section of a pipe to another.
Formula: A₁v₁ = A₂v₂
where:
- A₁ and A₂ are the cross-sectional areas,
- v₁ and v₂ are the fluid velocities.
Bernoulli’s Equation: Describes the conservation of energy in a flowing fluid.
Formula: \[ P + \frac{1}{2} \rho v^2 + \rho gh = \text{constant} \]
where:
- P is the fluid pressure,
- ρ is the fluid density,
- v is the fluid velocity,
- g is the acceleration due to gravity,
- h is the height above a reference point.
Key Points:
- High fluid speed corresponds to low pressure.
- Low fluid speed corresponds to high pressure.
Free-Body Diagrams (FBDs)
Free-Body Diagram: A graphical representation used to visualize the forces acting on an object. Each force is represented by an arrow pointing in the direction of the force.
Steps to Draw FBDs
- Identify the Object:
- Select the object to be analyzed.
- Isolate the Object:
- Represent the object as a dot or a simple shape.
- Draw the Forces:
- Draw arrows for all forces acting on the object, with lengths proportional to the magnitudes of the forces.
- Label each force (e.g., Fg for gravitational force, Fₙ for normal force).
- Check for Completeness:
- Ensure all forces acting on the object are included.
Common Forces in FBDs
- Gravitational Force (Fg):
- The force due to gravity acting downward.
- Formula: Fg = mg
- Normal Force (Fₙ):
- The perpendicular force exerted by a surface against an object in contact with it.
- Frictional Force (Fբ):
- The force that opposes the relative motion or tendency of such motion between two surfaces in contact.
- Tension Force (T):
- The pulling force transmitted through a string, rope, cable, or similar object.
- Buoyant Force (FB):
- The upward force exerted by a fluid on an immersed or floating object.
- Formula: \[ F_B = \rho V g \]
Examples
Example 1: Block in Water
Scenario: A block of mass 2 kg is submerged in water. Draw the free-body diagram and calculate the buoyant force.
Solution:
- Forces Acting:Gravitational force: Fg = mg = 2kg×9.8m/s² = 19.6N
- Buoyant force: \[ F_B = \rho V g \]
Since FB = Fg: - FB = 19.6N
FBD:
- Arrow down for Fg (19.6 N)
- Arrow up for FB (19.6 N)
Example 2: Flow Rate in a Pipe
Scenario: Water flows through a pipe with a cross-sectional area of 0.1 m² at a speed of 2 m/s. The pipe narrows to an area of 0.05 m². Find the speed of water in the narrow section.
Solution: \[ A_1 v_1 = A_2 v_2 \] \[ 0.1 \, \text{m}^2 \times 2 \, \text{m/s} = 0.05 \, \text{m}^2 \times v_2 \] \[ v_2 = \frac{0.2}{0.05} \] \[ v_2 = 4 \, \text{m/s} \]
Example 3: Object on an Inclined Plane
Scenario: A 5 kg object is on an inclined plane making a 30° angle with the horizontal. Draw the free-body diagram and calculate the components of the gravitational force parallel and perpendicular to the plane.
Solution:
- Gravitational Force: Fg = mg = 5kg×9.8m/s² = 49N
- Parallel Component: F∥ = Fgsinθ = 49N×sin30° = 24.5N
- Perpendicular Component: F⊥= Fgcosθ = 49N×cos30° = 42.4N
FBD:
- Arrow down for Fg (49 N)
- Arrow along the incline for F∥ (24.5 N)
- Arrow perpendicular to the incline for F⊥ (42.4 N)
Example 4: Buoyant Force on a Submerged Object
Problem:
A cube of wood with a density of 500 kg/m³ and a volume of 0.1 m³ is fully submerged in water. Calculate the buoyant force acting on the cube.
Solution:
- Given:
Density of wood, ρwood = 500kg/m³
Volume of wood, V=0.1m³
Density of water, ρwater=1000kg/m³ - Buoyant Force, Fb:
\[ F_b = \rho_{\text{water}} \times V \times g \] \[ F_b = 1000 \, \text{kg/m}^3 \times 0.1 \, \text{m}^3 \times 9.8 \, \text{m/s}^2 \] \[ F_b = 980 \, \text{N} \]
Example 5: Pressure at a Depth in a Fluid
Problem:
Calculate the pressure at a depth of 5 meters below the surface of a lake. Assume the density of water is 1000 kg/m³ and the atmospheric pressure at the surface is 101,325 Pa.
Solution:
- Given:
Depth, h=5m
Density of water, ρ=1000kg/m³
Gravitational acceleration, g=9.8m/s²
Atmospheric pressure, Patm=101325Pa - Pressure at depth, P:
\[ P = P_{\text{atm}} + \rho \times g \times h \] \[ P = 101325 \, \text{Pa} + 1000 \, \text{kg/m}^3 \times 9.8 \, \text{m/s}^2 \times 5 \, \text{m} \] \[ P = 101325 \, \text{Pa} + 49000 \, \text{Pa} \] \[ P = 150325 \, \text{Pa} \]
Practice Problems
Question 1:
A cube of wood with a side length of 0.1 meters is floating in water. The density of the wood is 600 kg/m³, and the density of water is 1000 kg/m³. What fraction of the cube’s volume is submerged in the water?
A) 0.6
B) 0.75
C) 0.4
D) 0.8
Answer: A) 0.6
Explanation:
The fraction of the volume of the wood submerged in water can be found using the principle of buoyancy, which states that the buoyant force equals the weight of the displaced fluid. The fraction submerged (Vs/V) is given by the ratio of the density of the wood (ρwood) to the density of water (ρwater).
\[ \frac{V_s}{V} = \frac{\rho_{\text{wood}}}{\rho_{\text{water}}} \] \[\text{Given:}\] \[ \rho_{\text{wood}} = 600 \, \text{kg/m}^3 \] \[ \rho_{\text{water}} = 1000 \, \text{kg/m}^3 \] \[\text{Calculate the fraction submerged:}\] \[ \frac{V_s}{V} = \frac{600 \, \text{kg/m}^3}{1000 \, \text{kg/m}^3} = 0.6 \] \[\text{Thus, the correct answer is 0.6.}\]
Question 2:
A cylindrical tank is filled with water to a height of 2 meters. What is the pressure at the bottom of the tank? (Assume the density of water is 1000 kg/m³ and gravitational acceleration g is 9.8 m/s².)
A) 980 Pa
B) 1960 Pa
C) 4900 Pa
D) 19600 Pa
Answer: D) 19600 Pa
Explanation:
The pressure at the bottom of a fluid column is given by the hydrostatic pressure formula:
P = ρgh
where P is the pressure, ρ is the density of the fluid, g is the acceleration due to gravity, and h is the height of the fluid column.
Given:
- ρ=1000kg/m³
- g=9.8m/s²
- h=2m
Calculate the pressure:
P = 1000kg/m³×9.8m/s²×2m = 19600Pa
Thus, the correct answer is 19600 Pa.
Question 3:
A block of metal weighing 50 N is submerged in water. The buoyant force acting on the block is 30 N. What is the net force acting on the block, and in which direction?
A) 20 N, upward
B) 80 N, downward
C) 20 N, downward
D) 50 N, upward
Answer: C) 20 N, downward
Explanation:
To determine the net force acting on the block, we need to consider both the weight of the block and the buoyant force. The net force (Fₙₑₜ) is given by the difference between the weight (W) and the buoyant force (Fb).
Given:
- Weight W = 50N
- Buoyant force Fb = 30N
Calculate the net force:
Fₙₑₜ = W−Fb = 50N−30N = 20N
Since the weight is greater than the buoyant force, the net force is downward.
Thus, the correct answer is 20 N, downward.