Heat and Energy Transfer
Understanding heat and energy transfer is essential for mastering the principles of thermodynamics and achieving a high score on the AP Physics exam. This topic covers the mechanisms of heat transfer, the laws governing energy transfer, and practical applications. Below are detailed notes on heat and energy transfer, along with five examples to help you excel in this topic.
Learning Objectives
In the topic of Heat and Energy Transfer for the AP Physics exam, you should learn the principles of thermal energy, mechanisms of heat transfer (conduction, convection, and radiation), and the laws of thermodynamics. Understand how to calculate heat transfer, analyze thermal equilibrium, and solve problems involving specific heat, latent heat, and calorimetry. Grasp the concept of entropy and its implications for energy efficiency. Master the application of these concepts in real-world scenarios and experimental contexts.
Mechanisms of Heat Transfer
1. Conduction
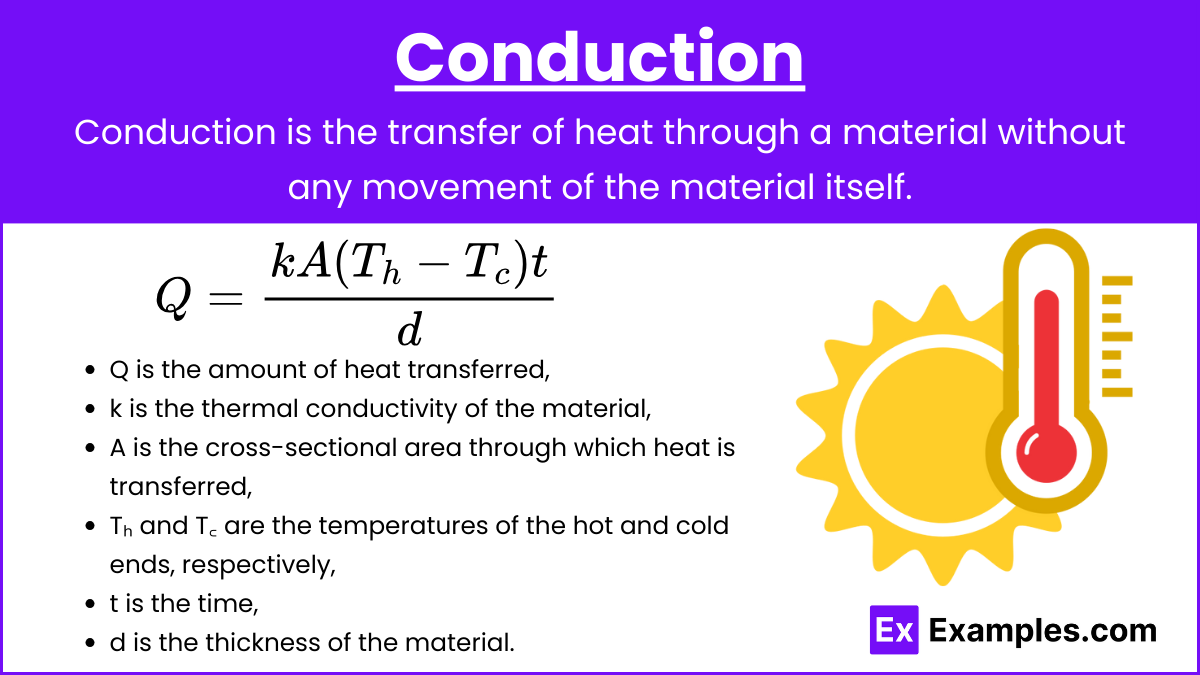
Conduction: Conduction is the transfer of heat through a material without any movement of the material itself. It occurs due to the collision and diffusion of molecules and free electrons.
Fourier’s Law of Heat Conduction: \[ Q = \frac{kA(T_h – T_c)t}{d} \]
where:
- Q is the amount of heat transferred,
- k is the thermal conductivity of the material,
- A is the cross-sectional area through which heat is transferred,
- Tₕ and T꜀ are the temperatures of the hot and cold ends, respectively,
- t is the time,
- d is the thickness of the material.
2. Convection
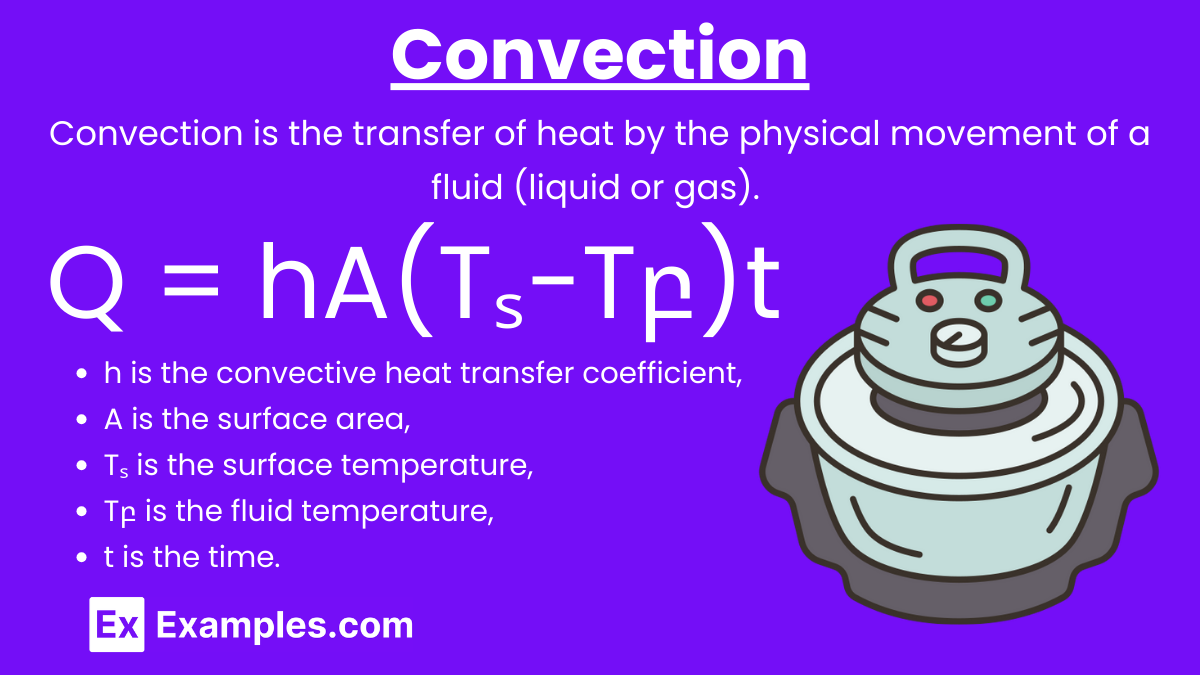
Convection: Convection is the transfer of heat by the physical movement of a fluid (liquid or gas). It can be natural (caused by buoyancy forces) or forced (caused by external means such as a pump or fan).
Convective Heat Transfer Equation: Q = hA(Tₛ−Tբ)t
where:
- h is the convective heat transfer coefficient,
- A is the surface area,
- Tₛ is the surface temperature,
- Tբ is the fluid temperature,
- t is the time.
3. Radiation
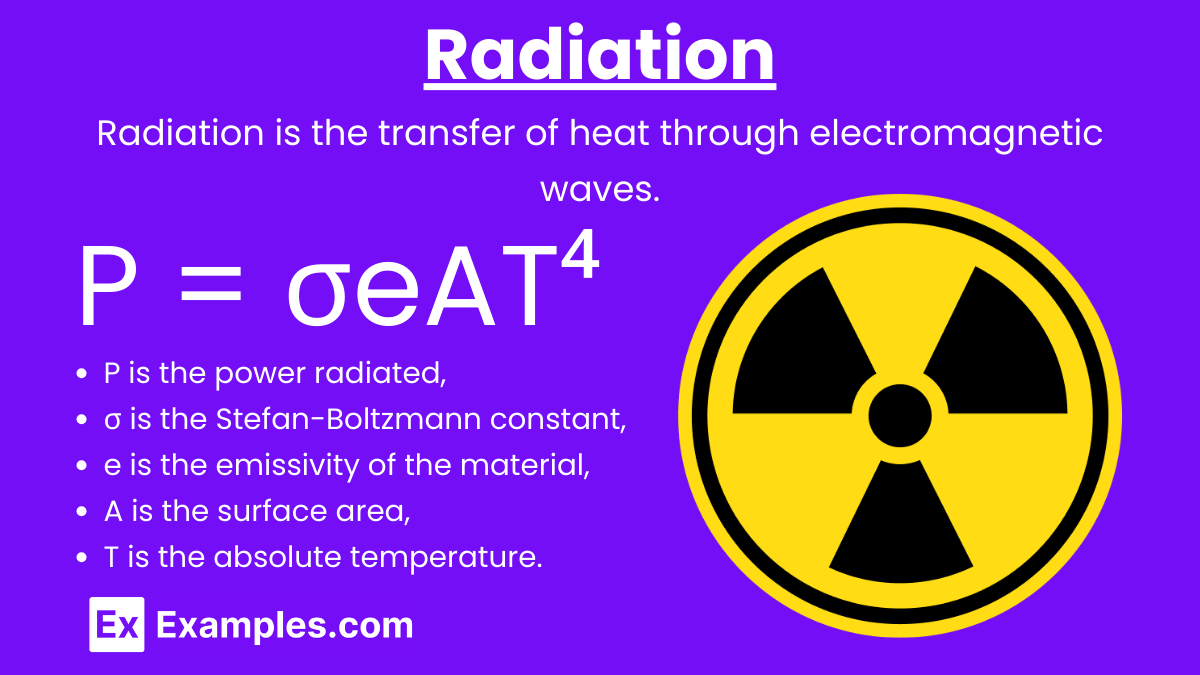
Radiation: Radiation is the transfer of heat through electromagnetic waves. It does not require a medium and can occur in a vacuum.
Stefan-Boltzmann Law: P = σeAT⁴
where:
- P is the power radiated,
- σ is the Stefan-Boltzmann constant (5.67×10⁻⁸ W/m²K⁴),
- e is the emissivity of the material,
- A is the surface area,
- T is the absolute temperature.
Laws Governing Heat Transfer
1. First Law of Thermodynamics
First Law of Thermodynamics: Energy cannot be created or destroyed, only transferred or converted from one form to another. For a thermodynamic system, the change in internal energy (ΔU) is equal to the heat added to the system (Q) minus the work done by the system (W).
ΔU = Q−W
2. Second Law of Thermodynamics
Second Law of Thermodynamics: Heat naturally flows from a hotter object to a cooler one and not in the reverse direction. It also states that the entropy of an isolated system always increases.
Examples of Heat and Energy Transfer
Example 1: Conduction Through a Metal Rod
Scenario: A metal rod with a thermal conductivity of 200W/m⋅K, a length of 0.5m, and a cross-sectional area of 0.01m² has its ends maintained at temperatures of 100⁰C and 0⁰C. Calculate the amount of heat transferred through the rod in 10 minutes.
Solution: \[ Q = \frac{kA(T_h – T_c)t}{d} \] \[ Q = \frac{200 \times 0.01 \times (100 – 0) \times (10 \times 60)}{0.5} \] \[ Q = \frac{200 \times 0.01 \times 100 \times 600}{0.5} \] \[ Q = 240,000 \, \text{J} \]
Example 2: Natural Convection
Scenario: A hot plate with a surface area of 0.5m² is maintained at 120⁰C in air at 25⁰C. If the convective heat transfer coefficient is 10W/m²K, calculate the heat transferred by convection in 1 hour.
Solution: \[ Q = hA(T_s – T_f)t \] \[ Q = 10 \times 0.5 \times (120 – 25) \times 3600 \] \[ Q = 10 \times 0.5 \times 95 \times 3600 \] \[ Q = 1,710,000 \, \text{J} \]
Example 3: Radiation from a Hot Surface
Scenario: A blackbody surface with an area of 0.2m² is at a temperature of 500K. Calculate the power radiated from the surface.
Solution: \[ P = \sigma e A T^4 \] \[ \text{For a blackbody, } e = 1: \] \[ P = 5.67 \times 10^{-8} \times 1 \times 0.2 \times 500^4 \] \[ P = 5.67 \times 10^{-8} \times 0.2 \times 6.25 \times 10^7 \] \[ P = 5.67 \times 10^{-8} \times 1.25 \times 10^7 \] \[ P = 7.0875 \, \text{kW} \]
Example 4: First Law of Thermodynamics
Scenario: A gas in a piston-cylinder device receives 1500J of heat and does 500J of work. Calculate the change in internal energy of the gas.
Solution: ΔU = Q−W
ΔU = 1500J−500J
ΔU = 1000J
Example 5: Heat Transfer in a Car Radiator
Scenario: The radiator of a car with a surface area of 1.5m² cools hot water from 90⁰C to 60⁰C. If the heat transfer coefficient is 30W/m²K, calculate the heat transferred in 20 minutes.
Solution: \[ Q = hA(T_s – T_f)t \] \[ Q = 30 \times 1.5 \times (90 – 60) \times (20 \times 60) \] \[ Q = 30 \times 1.5 \times 30 \times 1200 \] \[ Q = 1,620,000 \, J \]
Practice Problems
Question 1:
A metal rod is 1 meter long and has a cross-sectional area of 0.01m². One end of the rod is kept at 100°C, and the other end is kept at 0°C. If the thermal conductivity of the metal is 50W/m⋅K, what is the rate of heat transfer through the rod?
A) 25 W
B) 50 W
C) 75 W
D) 100 W
Answer: B) 50 W
Explanation:
The rate of heat transfer Q through a rod can be calculated using the formula:
\[ Q = \frac{kA \Delta T}{L} \]
where:
- k is the thermal conductivity (50W/m⋅K)
- A is the cross-sectional area (0.01m²)
- ΔT is the temperature difference (100°C−0°C = 100°C)
- L is the length of the rod (1m)
Calculate the rate of heat transfer:
\[ Q = \frac{50 \, \text{W/m} \cdot \text{K} \times 0.01 \, \text{m}^2 \times 100 \, \text{K}}{1 \, \text{m}} = 50 \, \text{W} \]
Thus, the correct answer is 50 W.
Question 2:
A 500 g block of copper is heated from 25°C to 75°C. The specific heat capacity of copper is 0.385J/g⋅°C. How much heat energy is required?
A) 7,700 J
B) 8,750 J
C) 9,625 J
D) 10,000 J
Answer: A) 7,700 J
Explanation:
The heat energy Q required to change the temperature of a substance can be calculated using the formula:
Q = mcΔT
where:
- m is the mass of the substance (500g)
- c is the specific heat capacity (0.385J/g⋅°C)
- ΔT is the temperature change (75°C−25°C = 50°C)
Calculate the heat energy:
Q = 500g×0.385J/g⋅°C×50°C = 9,625J
Thus, the correct answer is 9,625 J.
Question 3:
Which method of heat transfer does not require a medium?
A) Conduction
B) Convection
C) Radiation
D) Conduction and Convection
Answer: C) Radiation
Explanation:
Heat transfer occurs through three main mechanisms: conduction, convection, and radiation.
- Conduction is the transfer of heat through a material by direct contact of particles and requires a medium (solid, liquid, or gas).
- Convection is the transfer of heat by the movement of fluids (liquids or gases) and requires a medium.
- Radiation is the transfer of heat through electromagnetic waves and does not require a medium. Heat can be transferred through the vacuum of space by radiation.
Therefore, radiation is the method of heat transfer that does not require a medium. The correct answer is C) Radiation.