Interference and Diffraction

Focus on mastering the concepts of interference and diffraction. Understand the conditions for constructive and destructive interference, and be able to apply formulas related to Young’s double-slit experiment. Grasp the principles of single-slit diffraction and diffraction gratings, including how to calculate fringe spacing and diffraction angles. Practice solving problems involving these phenomena to enhance your comprehension and problem-solving skills, which are crucial for success in AP Physics.

Learning Objectives

Understand the principles of interference and diffraction, including the conditions for constructive and destructive interference, and how to apply key formulas such as those from Young’s double-slit experiment and single-slit diffraction. Master the concepts of coherence, path difference, and phase difference. Be able to analyze and interpret interference and diffraction patterns, solve related problems, and understand the significance of Huygens’ Principle in wave behavior.

Interference

Interference is the phenomenon that occurs when two or more waves overlap and combine to form a new wave pattern. This can be observed with all types of waves, including sound waves, light waves, and water waves.

Types of Interference

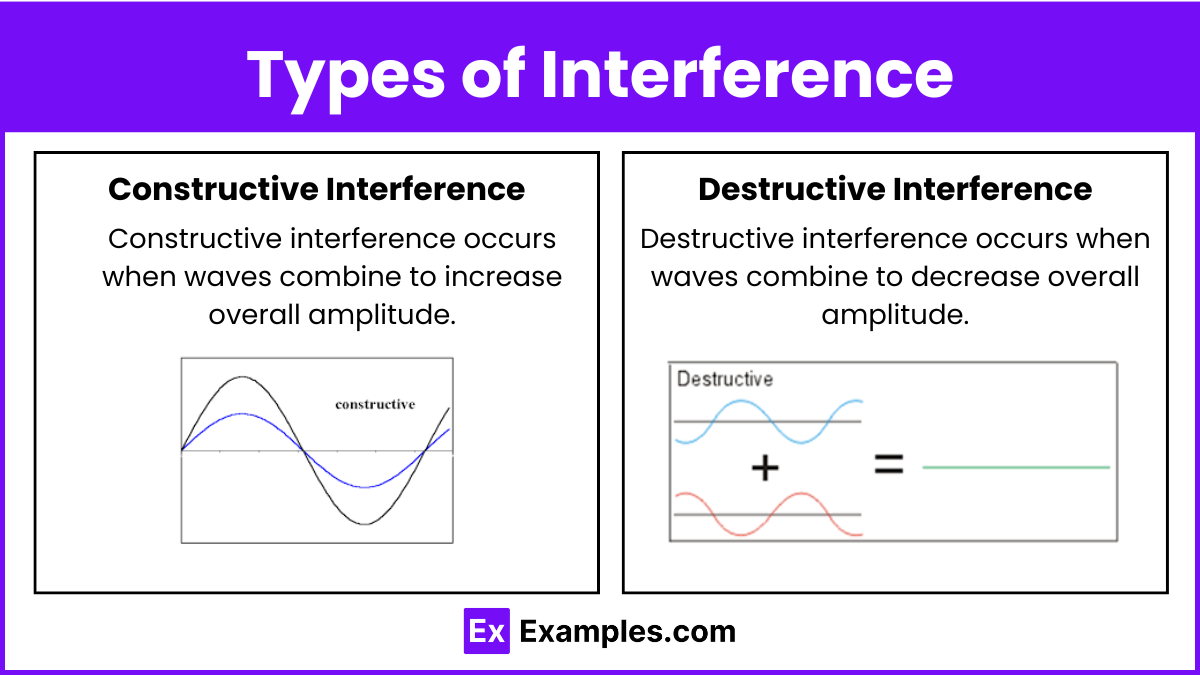
1. Constructive Interference
   • Occurs when the crests of two waves align, resulting in a wave of greater amplitude.
   • Formula: Aₜₒₜₐₗ=A1+A2
   • Example: Two in-phase light waves from coherent sources like lasers creating bright fringes.
2. Destructive Interference
   • Occurs when the crest of one wave aligns with the trough of another, resulting in a wave of reduced or zero amplitude.
   • Formula: Aₜₒₜₐₗ=A1-A2
   • Example: Noise-canceling headphones use destructive interference to reduce unwanted ambient sounds.

Conditions for Interference

• Coherent Sources: The waves must have a constant phase difference and the same frequency.
• Path Difference: The difference in the distance traveled by two waves from their respective sources to a common point.

Young’s Double-Slit Experiment

• Demonstrates the principle of interference with light.
• Setup: Light from a coherent source (like a laser) passes through two closely spaced slits.
• Result: An interference pattern of bright and dark fringes on a screen.

Key Formulas

Constructive Interference (Bright Fringes):

dsin⁡θ=mλ

Where d is the distance between the slits, θ is the angle of the fringe, m is the order of the fringe, and λ is the wavelength of the light.

Destructive Interference (Dark Fringes):

   

Fringe Spacing:

   

where L is the distance from the slits to the screen.

Diffraction

Diffraction is the bending of waves around the edges of an obstacle or aperture. It becomes significant when the size of the obstacle or aperture is comparable to the wavelength of the wave.

Types of Diffraction

1. Single-Slit Diffraction
   • Occurs when waves pass through a narrow slit and spread out.
   • Central maximum is the brightest and widest part of the pattern.
   • Secondary maxima are less intense and narrower.

Key Formula

• Position of Dark Fringes:
• asin⁡θ=mλ where a is the width of the slit, and m is the order of the dark fringe.

2. Multiple-Slit Diffraction (Diffraction Grating)
   • Consists of many equally spaced slits.
   • Produces sharp and well-defined maxima.

Key Formula

• Maxima Condition:
• dsin⁡θ=nλ
• where d is the grating spacing, and nnn is the order of the maximum.

Diffraction and Interference Combined

• Double-Slit Diffraction: The pattern observed is a combination of interference and diffraction effects. The overall envelope pattern is due to single-slit diffraction, while the detailed pattern within this envelope is due to double-slit interference.

Key Concepts to Remember

• Coherence: Necessary for stable interference patterns.
• Path Difference: Determines constructive or destructive interference.
• Phase Difference: Δϕ=2πλΔx
• Superposition Principle: The resulting wave is the sum of individual waves.
• Huygens’ Principle: Every point on a wavefront is a source of secondary wavelets.

Examples of Interference and Diffraction

1. Young’s Double-Slit Experiment:
   • Demonstrates light interference by passing light through two narrow slits, producing a pattern of bright and dark fringes on a screen.
2. Noise-Canceling Headphones:
   • Use destructive interference to reduce ambient sounds by producing sound waves that are out of phase with external noise.
3. Diffraction Gratings in Spectrometers:
   • Utilize multiple slits to disperse light into its component wavelengths, creating detailed spectra for analysis.
4. CD and DVD Surfaces:
   • Reflect light in specific patterns due to the microscopic grooves, causing constructive and destructive interference that produces rainbow colors.
5. Single-Slit Diffraction with Water Waves:
   • Observed when water waves pass through a narrow opening and spread out, forming a pattern of alternating constructive and destructive interference regions.

Multiple Choice Questions on Interference and Diffraction

Question 1

In Young’s double-slit experiment, if the wavelength of light is increased, what happens to the fringe spacing on the screen? A) Increases
B) Decreases
C) Remains the same
D) Becomes zero

Answer: A) Increases

Explanation: Fringe spacing (Δy) in Young’s double-slit experiment is given by Δy=λL/d where λ is the wavelength, L is the distance from the slits to the screen, and d is the distance between the slits. If the wavelength (λ) increases, the fringe spacing (Δy) also increases, because they are directly proportional.

Question 2

Which of the following conditions is necessary for two waves to produce a stable interference pattern? A) They must have different frequencies.
B) They must be incoherent.
C) They must have the same amplitude.
D) They must have a constant phase difference.

Answer: D) They must have a constant phase difference.

Explanation: For a stable interference pattern, the waves must be coherent, meaning they have a constant phase difference and the same frequency. The same amplitude is not necessary, although it can affect the visibility of the interference pattern. Incoherent waves do not produce stable interference patterns because their phase difference varies randomly over time.

Question 3

In a single-slit diffraction experiment, what happens to the width of the central maximum if the slit width is decreased? A) Increases
B) Decreases
C) Remains the same
D) Vanishes

Answer: A) Increases

Explanation: The angular width of the central maximum in single-slit diffraction is given by θ=λa\theta = \frac{\lambda}{a}θ=aλ​, where λ\lambdaλ is the wavelength of the light, and aaa is the slit width. If the slit width aaa is decreased, the angle θ\thetaθ increases, which means the central maximum spreads out more, resulting in an increased width of the central maximum.