Magnetic systems are fundamental in physics, encompassing the study of magnetic fields, forces, and their interactions with electric currents and materials. In AP Physics, understanding magnetic systems includes exploring the behavior of magnetic fields generated by currents and permanent magnets, the forces on moving charges, and the concept of magnetic flux. Key applications include electromagnets, electric motors, and transformers, which illustrate the practical significance of magnetic principles in technology and everyday life.
Free AP Physics 2: Algebra-Based Practice Test
Learning Objectives
Understand the principles of magnetic fields, including their sources and properties. Learn how to calculate the magnetic force on moving charges and current-carrying conductors using the Lorentz force and related equations. Understand magnetic flux, Faraday's Law of Induction, and Lenz's Law. Study the applications of magnetic systems, such as electromagnets, electric motors, and transformers. Practice solving problems involving these concepts to apply your knowledge effectively.
Magnetic Systems
Magnetic systems play a crucial role in the field of physics, particularly in understanding how magnetic fields interact with electric currents and materials. The study of magnetic systems includes concepts such as magnetic fields, forces on moving charges, and the behavior of materials in magnetic fields.
Magnetic Fields
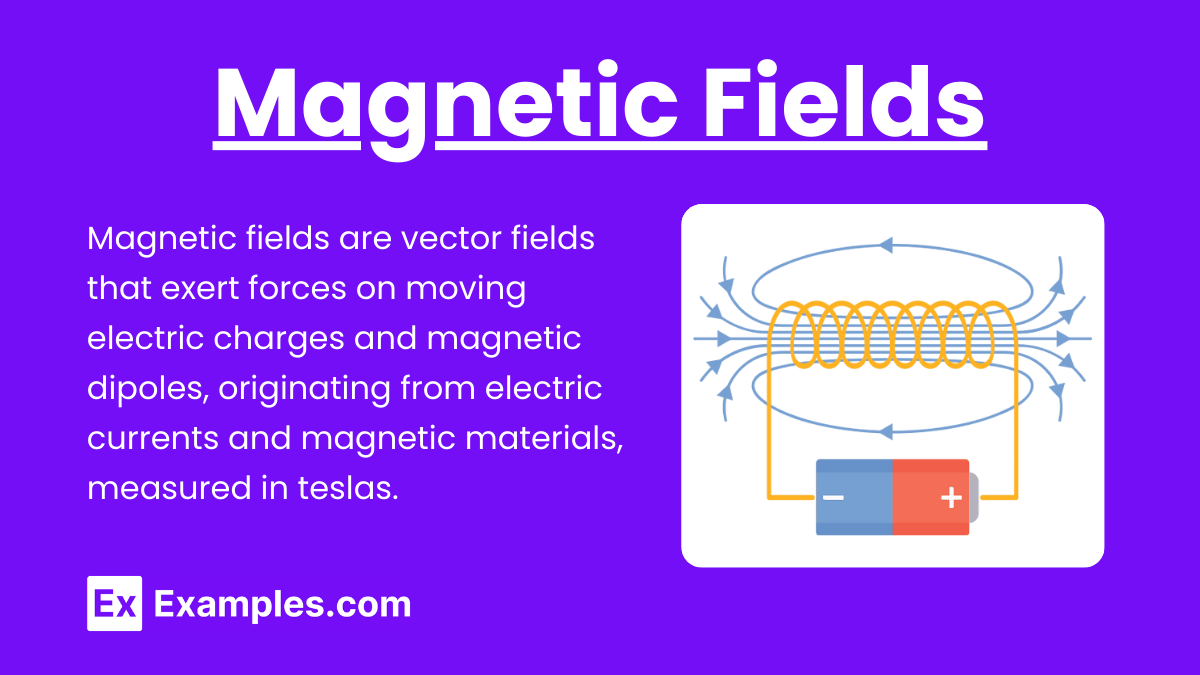
A magnetic field is a vector field that exerts a magnetic force on moving electric charges, electric currents, and magnetic dipoles. It is represented by the symbol B and is measured in teslas (T).
Properties
Direction: The direction of the magnetic field at a point is the direction of the magnetic force on a north pole placed at that point.
Strength: The strength of the magnetic field is proportional to the density of the magnetic field lines.
Sources of Magnetic Fields
Permanent Magnets: Objects that produce a magnetic field due to the alignment of magnetic domains within the material.
Electric Currents: Moving electric charges produce a magnetic field around them, described by Ampère's Law.
Magnetic Forces

Lorentz Force
The force exerted on a charged particle moving in a magnetic field is called the Lorentz force. It is given by: F=q(v×B)
Where:
F is the force on the particle.
q is the charge of the particle.
v is the velocity of the particle.
B is the magnetic field.
Force on a Current-Carrying Conductor
A current-carrying conductor in a magnetic field experiences a force given by:
F=I(L×B)
where:
I is the current.
L is the length vector of the conductor within the magnetic field.
Magnetic Flux
Magnetic flux (Φ) is a measure of the number of magnetic field lines passing through a given surface.
It is given by:
Φ=B⋅A=BAcos(θ)
where:
A is the area vector.
θ is the angle between the magnetic field and the normal to the surface.
Faraday's Law of Induction
Faraday's Law states that a change in magnetic flux through a circuit induces an electromotive force (EMF) in the circuit. It is given by:
\mathcal{E} = -\frac{d\Phi}{dt}
Applications of Magnetic Systems
Electromagnets
Electromagnets are created by running an electric current through a coil of wire, generating a magnetic field. The strength of the electromagnet can be increased by increasing the current or the number of turns in the coil.
Electric Motors
Electric motors convert electrical energy into mechanical energy using the force exerted by magnetic fields on current-carrying conductors.
Transformers
Transformers use the principles of electromagnetic induction to transfer electrical energy between two or more coils of wire, often changing the voltage in the process.
Examples of Magnetic Systems
Electromagnets: Used in devices like cranes for lifting heavy metal objects.
Electric Motors: Convert electrical energy into mechanical energy in appliances.
Transformers: Transfer electrical energy between circuits, changing voltage levels.
Magnetic Resonance Imaging (MRI): Uses strong magnetic fields for medical imaging.
Speakers and Microphones: Utilize magnetic fields to convert electrical signals to sound and vice versa.
Practice Test Questions on Magnetic Systems
Question 1: What is the direction of the force on a positive charge moving in a magnetic field?
a) Parallel to the magnetic field
b) Perpendicular to both the velocity of the charge and the magnetic field
c) Opposite to the direction of the magnetic field
d) In the direction of the velocity of the charge
Answer: b) Perpendicular to both the velocity of the charge and the magnetic field
Explanation: The force on a moving charge in a magnetic field is given by the Lorentz force law: F=q(v×B). This means the force is perpendicular to both the velocity (v) of the charge and the magnetic field (B). The direction is determined by the right-hand rule for a positive charge.
Question 2: Which law describes the relationship between the magnetic field around a closed loop and the electric current passing through the loop?
a) Faraday's Law
b) Lenz's Law
c) Biot-Savart Law
d) Ampère's Law
Answer: d) Ampère's Law
Explanation: Ampère's Law states that the integrated magnetic field around a closed loop is proportional to the electric current passing through the \oint \mathbf{B} \cdot d\mathbf{l} = \mu_0 I_{\text{enc}} . This law is fundamental in calculating the magnetic field produced by current-carrying conductors.
Question 3: What happens to the magnetic flux through a coil if the magnetic field strength is doubled and the area of the coil is halved, assuming the angle between the field and the area remains constant?
a) It remains unchanged.
b) It doubles.
c) It is halved.
d) It is quadrupled.
Answer: a) It remains unchanged.
Explanation: Magnetic flux (Φ) is given by Φ=B⋅A⋅cos(θ)where B is the magnetic field strength, A is the area, and θ is the angle between the magnetic field and the normal to the area. If B is doubled and A is halved, the product B .A remains the same, thus the magnetic flux remains unchanged.