Mass-energy equivalence, encapsulated by Einstein's famous equation ( E = mc² ), reveals the profound relationship between mass and energy. This principle indicates that mass can be converted into energy and vice versa. Understanding mass-energy equivalence is crucial for AP Physics students, as it underlies concepts in nuclear reactions, particle physics, and various practical applications such as nuclear power and medical imaging. Mastery of this topic is essential for excelling in advanced physics studies.
Free AP Physics 2: Algebra-Based Practice Test
Learning Objectives
Learning objectives for mass-energy equivalence include understanding and applying Einstein's equation E=mc², comprehending the concept of mass defect and its role in nuclear reactions, calculating the energy released in nuclear fission and fusion, and recognizing practical applications such as nuclear power and medical imaging. Additionally, students should be able to solve problems involving mass-energy conversions and explain the historical and theoretical significance of mass-energy equivalence in modern physics.
Mass-Energy Equivalence

Mass-energy equivalence is a fundamental concept in physics encapsulated by Albert Einstein's famous equation:
E=mc²
where:
E is the energy,
m is the mass,
c is the speed of light in a vacuum (≈3×10⁸m/s).
This equation indicates that mass can be converted into energy and vice versa.
Historical Context
Albert Einstein introduced the concept of mass-energy equivalence in his 1905 paper on special relativity. This breakthrough revolutionized our understanding of mass and energy, showing that they are interchangeable.
The Equation Explained
Energy (E)
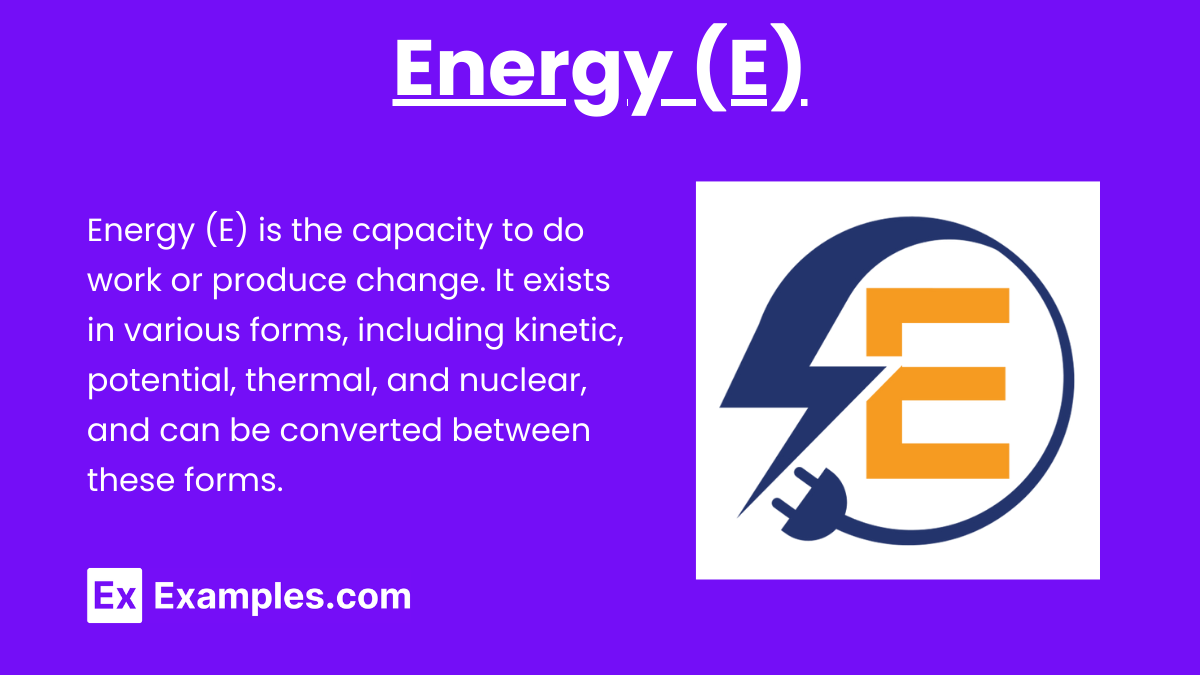
Energy is the capacity to do work. In the context of mass-energy equivalence, it refers to the intrinsic energy contained within a mass.
Mass (m)
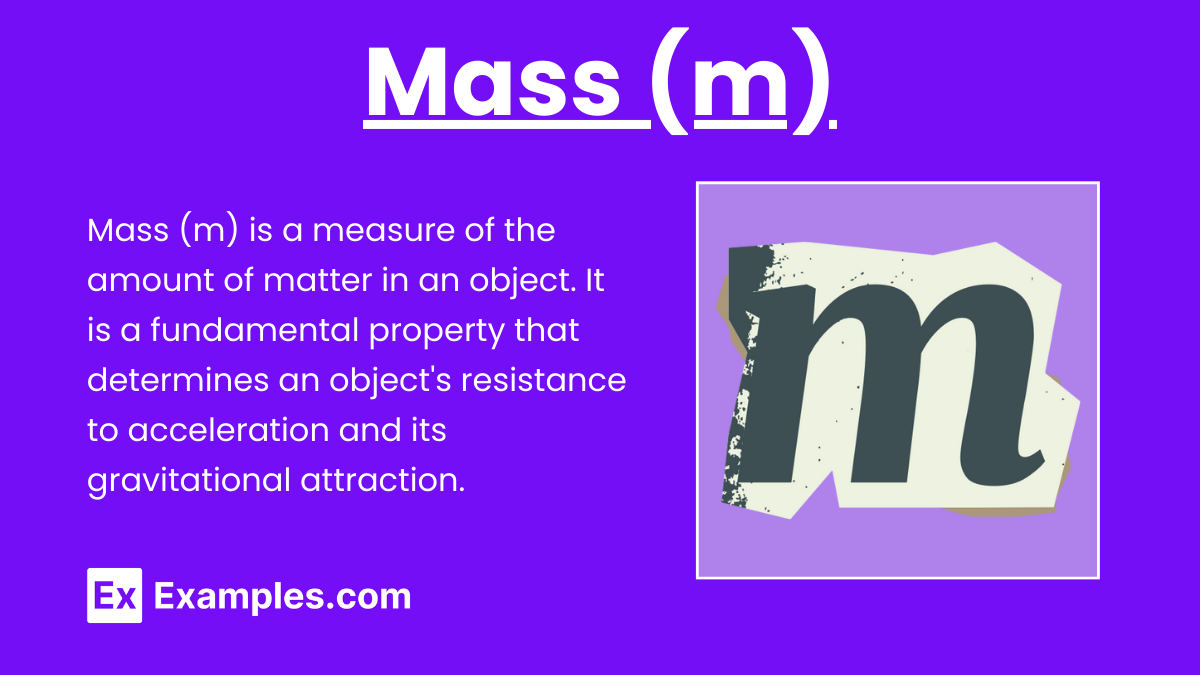
Mass is a measure of the amount of matter in an object. In Einstein's equation, it specifically refers to rest mass, which is the mass of an object when it is at rest.
Speed of Light (c)

The speed of light is a constant value, approximately 3×10⁸ meters per second. The squaring of this value in the equation emphasizes the large amount of energy produced from a relatively small amount of mass.
Implications of Mass-Energy Equivalence
Nuclear Reactions
Fission: In nuclear fission, a heavy nucleus splits into smaller nuclei, releasing energy. The loss in mass (mass defect) during this process is converted into energy.
Fusion: In nuclear fusion, light nuclei combine to form a heavier nucleus, releasing energy. Again, the mass defect is converted into energy.
Particle Physics
In particle physics, particles and antiparticles annihilate each other, converting their mass entirely into energy, often in the form of photons.
Examples of Mass-Energy Equivalence
Nuclear Fission in Power Plants: Uranium-235 nuclei split into smaller nuclei, releasing a significant amount of energy due to the mass defect.
Nuclear Fusion in the Sun: Hydrogen nuclei combine to form helium, converting some mass into energy that powers the sun and produces sunlight.
Particle-Antiparticle Annihilation: When an electron and positron collide, they annihilate each other, converting their entire mass into gamma-ray photons.
PET Scans in Medicine: Positron Emission Tomography uses positrons emitted by radioactive substances, which annihilate with electrons, converting mass into detectable energy.
Atomic Bomb Explosion: In an atomic bomb, the rapid fission of uranium or plutonium nuclei releases massive amounts of energy due to the conversion of mass into energy.
Practice Test Questions on Mass-Energy Equivalence
Question 1:
In which of the following processes is mass converted directly into energy?
A) Boiling water
B) Burning coal
C) Nuclear fusion in the sun
D) Compressing a spring
Answer: C) Nuclear fusion in the sun
Explanation: In nuclear fusion, such as the fusion of hydrogen nuclei into helium in the sun, some of the mass is converted directly into energy according to E=mc². This process releases a vast amount of energy, powering the sun and providing light and heat.
Question 2:
Which medical technology utilizes the principle of mass-energy equivalence to create detailed body images?
A) MRI (Magnetic Resonance Imaging)
B) X-rays
C) PET (Positron Emission Tomography) scans
D) Ultrasound
Answer: C) PET (Positron Emission Tomography) scans
Explanation: PET scans use positrons emitted by radioactive substances. When these positrons annihilate with electrons in the body, their mass is converted into gamma-ray photons, which are detected to create detailed images of the body’s internal structures.
Question 3:
The principle of mass-energy equivalence is directly responsible for the energy released in which of the following events?
A) Chemical reactions in a battery
B) Nuclear fission in a power plant
C) Stretching a rubber band
D) Melting ice
Answer: B) Nuclear fission in a power plant
Explanation: In nuclear fission, such as that which occurs in a power plant, the nucleus of an atom splits into smaller nuclei, and the mass defect (the difference in mass between the original nucleus and the sum of the smaller nuclei) is converted into a significant amount of energy, as described by E=mc²