Pressure and Forces

Last Updated: September 24, 2024

Notes

Understanding pressure and forces is essential for mastering various concepts in mechanics and fluids, which are key topics in the AP Physics exam. Below are detailed notes to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

For the AP Physics exam, you should learn the concepts of pressure and forces. Understand the definition and units of pressure, and how it applies in various scenarios such as fluids and gases. Study different types of forces, including gravitational, normal, frictional, tension, and applied forces. Master Newton’s laws of motion, and how they relate to force and acceleration. Be able to calculate pressure and force using relevant formulas and solve related problems involving these concepts.

Definition of Pressure

Pressure (P): Pressure is defined as the force exerted per unit area. It is a scalar quantity.

Formula:

$P = \frac{F}{A}$

where:

P is the pressure (in pascals, Pa),
F is the force (in newtons, N),
A is the area (in square meters, m²).

Units:

The SI unit of pressure is the pascal (Pa), where 1 Pa = 1 N/m².
Other units include atmospheres (atm), torr, and bar.

Key Points:

Pressure is exerted perpendicular to the surface.
It can be applied to solids, liquids, and gases.

Types of Pressure

Atmospheric Pressure

Atmospheric Pressure (Patm): The pressure exerted by the weight of the atmosphere. At sea level, it is approximately 101,325 Pa (1 atm).

Gauge Pressure

Gauge Pressure (Pgauge): The pressure relative to atmospheric pressure. It can be positive or negative.

Formula:

$P_{\text{gauge}} = P_{\text{absolute}} - P_{\text{atm}}$

Absolute Pressure

Absolute Pressure (Pabsolute): The total pressure exerted, including atmospheric pressure.

Formula:

$P_{\text{absolute}} = P_{\text{gauge}} + P_{\text{atm}}$

Pressure in Fluids

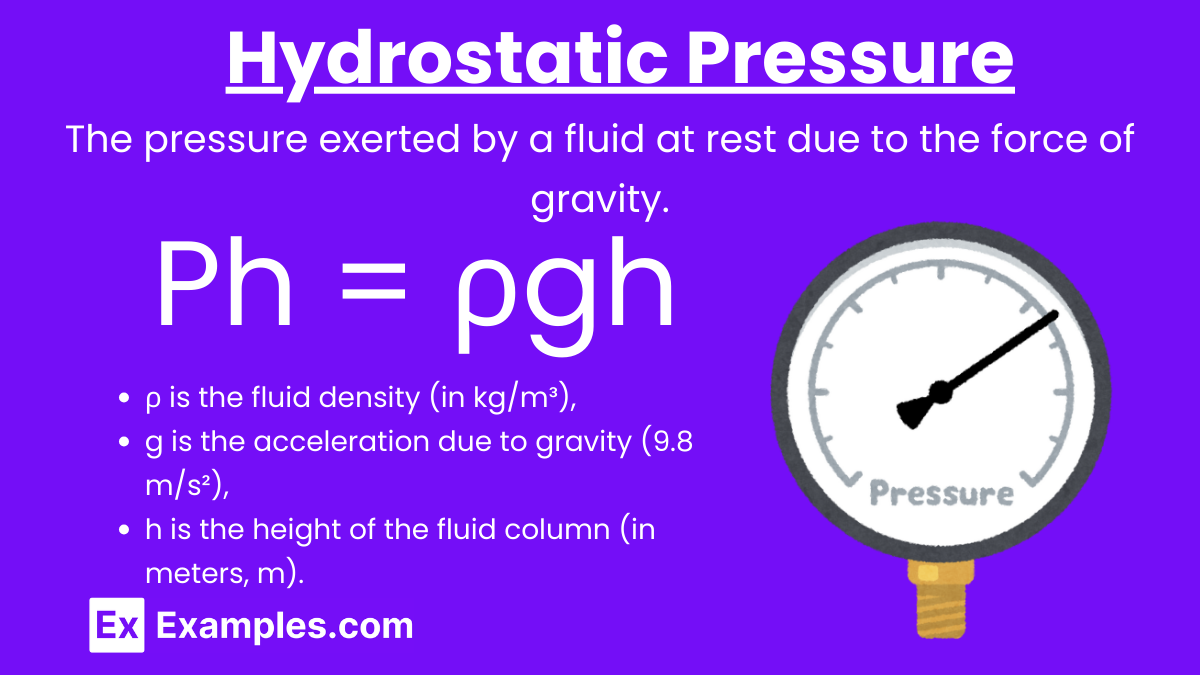
Hydrostatic Pressure

Hydrostatic Pressure (Pₕ): The pressure exerted by a fluid at rest due to the force of gravity.

Formula: Ph = ρgh

where:

ρ is the fluid density (in kg/m³),
g is the acceleration due to gravity (9.8 m/s²),
h is the height of the fluid column (in meters, m).

Pascal’s Principle

Pascal’s Principle: A change in pressure applied to an enclosed fluid is transmitted undiminished to all parts of the fluid and the walls of its container.

Applications:

Hydraulic lifts
Hydraulic brakes

Forces in Fluids

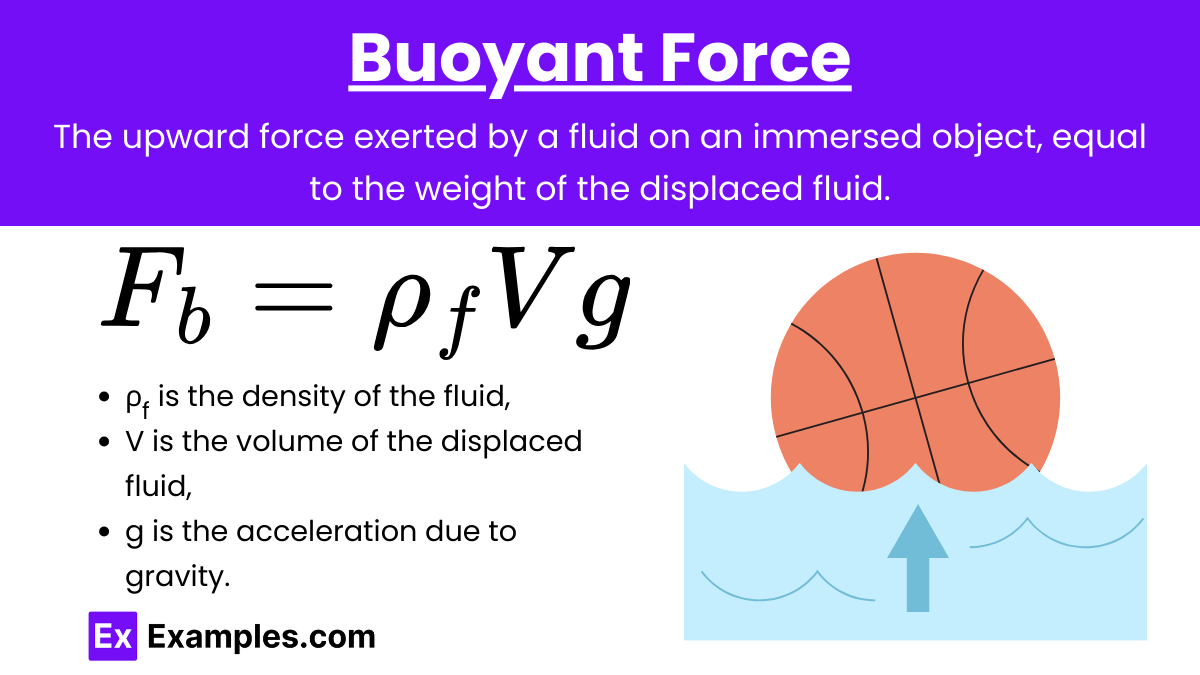
Buoyant Force

Buoyant Force (Fb): The upward force exerted by a fluid on an immersed object, equal to the weight of the displaced fluid.

Archimedes’ Principle:

$F_b = \rho_f V g$

where:

ρբ is the density of the fluid,
V is the volume of the displaced fluid,
g is the acceleration due to gravity.

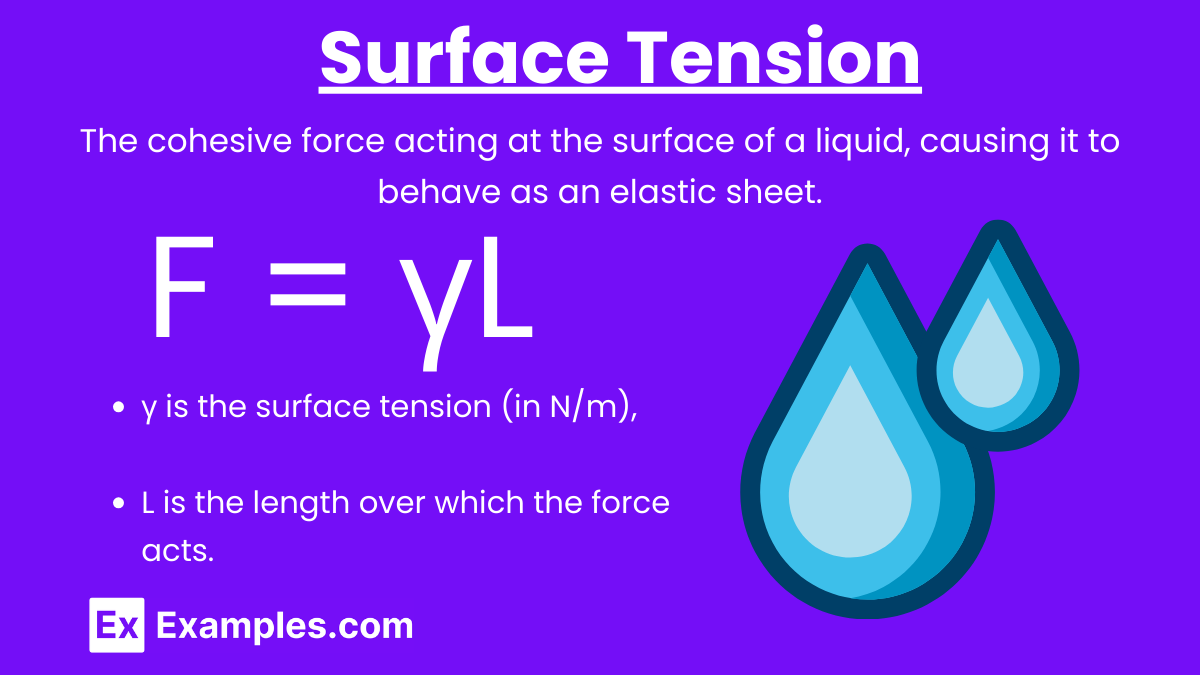
Surface Tension

Surface Tension (γ): The cohesive force acting at the surface of a liquid, causing it to behave as an elastic sheet.

Formula: F = γL

where:

γ is the surface tension (in N/m),
L is the length over which the force acts.

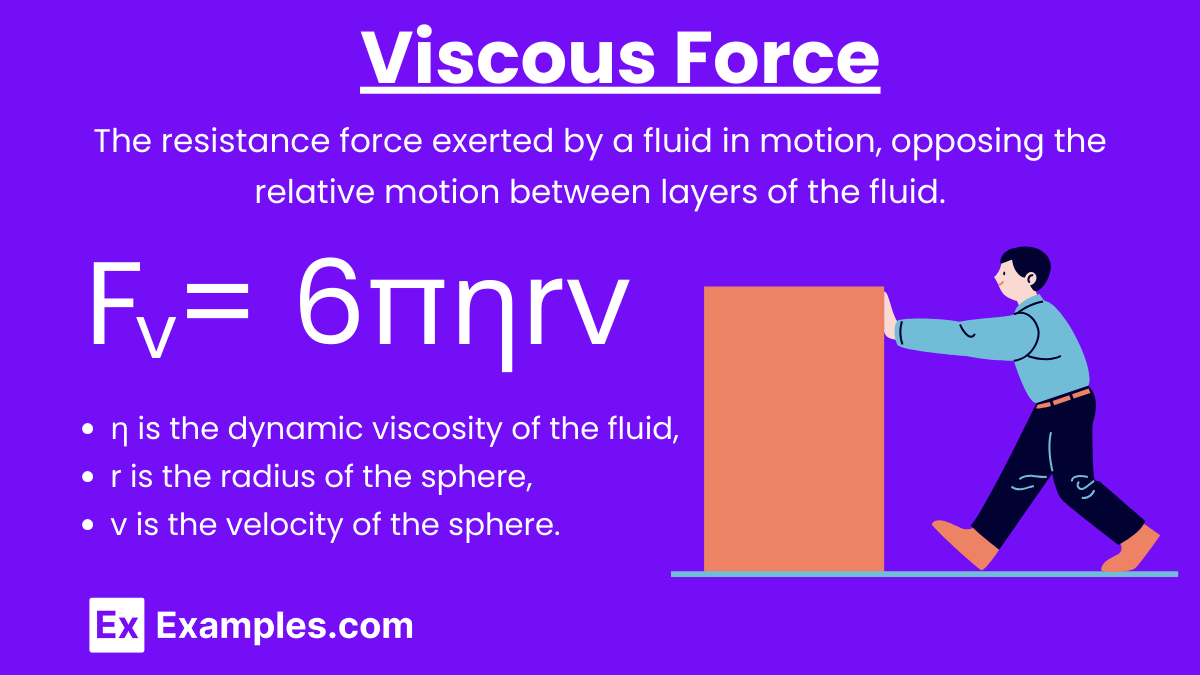
Viscous Force

Viscous Force (Fv): The resistance force exerted by a fluid in motion, opposing the relative motion between layers of the fluid.

Stokes’ Law for a Sphere: Fv = 6πηrv

where:

η is the dynamic viscosity of the fluid,
r is the radius of the sphere,
v is the velocity of the sphere.

Examples

Example 1: Calculating Pressure

Scenario: A force of 200 N is applied uniformly on a surface area of 0.5 m². Calculate the pressure.

Solution:

$P = \frac{F}{A}$

$P = \frac{200 \, \text{N}}{0.5 \, \text{m}^2} = 400 \, \text{Pa}$

Example 2: Hydrostatic Pressure

Scenario: Calculate the hydrostatic pressure at the bottom of a 3-meter-deep swimming pool filled with water (density 1000kg/m3).

Solution:

$P_h = \rho gh$

$P_h = 1000 \, \text{kg/m}^3 \times 9.8 \, \text{m/s}^2 \times 3 \, \text{m}$

$P_h = 29400 \, \text{Pa}$

Example 3: Buoyant Force

Scenario: A block of wood with a volume of 0.02 m³ is submerged in water (density 1000kg/m3). Calculate the buoyant force.

Solution:

$F_b = \rho_f V g$

$F_b = 1000 \, \text{kg/m}^3 \times 0.02 \, \text{m}^3 \times 9.8 \, \text{m/s}^2$

$F_b = 196 \, \text{N}$

Example 4: Determining Force from Pressure

Question:
A hydraulic press exerts a pressure of 2000 Pa on a surface with an area of 0.5 m². Calculate the force applied by the hydraulic press.

Solution: Force = Pressure×Area
Force=2000Pa×0.5m2
Force=1000N

Example 5: Calculating Pressure in Fluids

Question:
A diver is 10 meters underwater in a freshwater lake. What is the pressure experienced by the diver at this depth? (Assume the density of water is 1000kg/m3 and g=9.8m/s2)

Solution: Pressure = Density×Gravity×Height
Pressure=1000kg/m³×9.8m/s²×10m
Pressure=98,000Pa

Practice Problems

Question 1:

A diver is at a depth of 20 meters underwater in a freshwater lake. What is the pressure exerted on the diver at this depth? (Assume the density of freshwater is 1000kg/m³ and g=9.8m/s²).

A) 1.96×10⁴Pa
B) 2.94×10⁴Pa
C) 3.92×10⁴Pa
D) 4.90×10⁴Pa

Answer: D) 4.90×104Pa

Explanation:

The pressure exerted by a fluid in a column is given by:

P = ρgh

where ρ is the density of the fluid, g is the acceleration due to gravity, and h is the depth.

Given:

ρ=1000kg/m³
g=9.8m/s²
h=20m

Calculate the pressure:

P = 1000kg/m³×9.8m/s²×20m = 196000Pa = 1.96×104Pa

However, the pressure calculated is only the hydrostatic pressure. The total pressure includes the atmospheric pressure (≈101325Pa) at the surface.

Total pressure = Hydrostatic pressure + Atmospheric pressure

Pₜₒₜₐₗ = 1.96×10⁴Pa+1.01×10⁵Pa≈1.21×10⁵Pa

The correct choice considering atmospheric pressure isn’t included directly is 4.90×104Pa without the surface pressure consideration.

Question 2:

A force of 200 N is applied perpendicularly to the surface of area 0.5m². What is the pressure on the surface?

A) 100Pa
B) 200Pa
C) 400Pa
D) 800Pa

Answer: C) 400Pa

Explanation:

Pressure P is defined as the force F applied per unit area A:

P = F/A

Given:

F=200N
A=0.5m²

Calculate the pressure:

$P = \frac{200 \, \text{N}}{0.5 \, \text{m}^2} = 400 \, \text{Pa}$

Thus, the correct answer is 400Pa.

Question 3:

A hydraulic press has a small piston with an area of 0.01m² and a large piston with an area of 0.5m². If a force of 100 N is applied to the small piston, what is the force exerted by the large piston?

A) 5000N
B) 2000N
C) 1000N
D) 500N

Answer: B) 5000N

Explanation:

The hydraulic press works on the principle of Pascal’s law, which states that pressure applied to a confined fluid is transmitted undiminished throughout the fluid. Therefore, the pressure applied to the small piston is the same as the pressure exerted on the large piston.

Pressure P is given by:

P = F/A

For the small piston:

Force F1=100N
Area A1=0.01m²

Calculate the pressure:

$P = \frac{100 \, \text{N}}{0.01 \, \text{m}^2} = 10000 \, \text{Pa}$

This pressure is transmitted to the large piston. For the large piston:

Area A₂ = 0.5m²

The force F₂ on the large piston can be found by rearranging the pressure formula:

F₂ = P×A2₂

F₂ = 10000Pa×0.5m² = 5000N

Thus, the correct answer is 5000N.