Pressure, Thermal Equilibrium, and the Ideal Gas Law

Understanding pressure, thermal equilibrium, and the ideal gas law is crucial for mastering thermodynamics concepts in the AP Physics exam. These topics cover the behavior of gases, how they interact, and the principles governing their physical properties. Below are detailed notes and five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics 2: Algebra-Based Practice Test

Learning Objectives

In this unit, you will learn to define and calculate pressure in various contexts, understand and apply the concept of thermal equilibrium in physical systems, and use the Ideal Gas Law (PV = nRT) to relate the pressure, volume, and temperature of an ideal gas. You will also explore how these principles interconnect, solve related problems, and analyze real-world applications, ensuring a comprehensive grasp of thermodynamics for the AP Physics exam.

Pressure

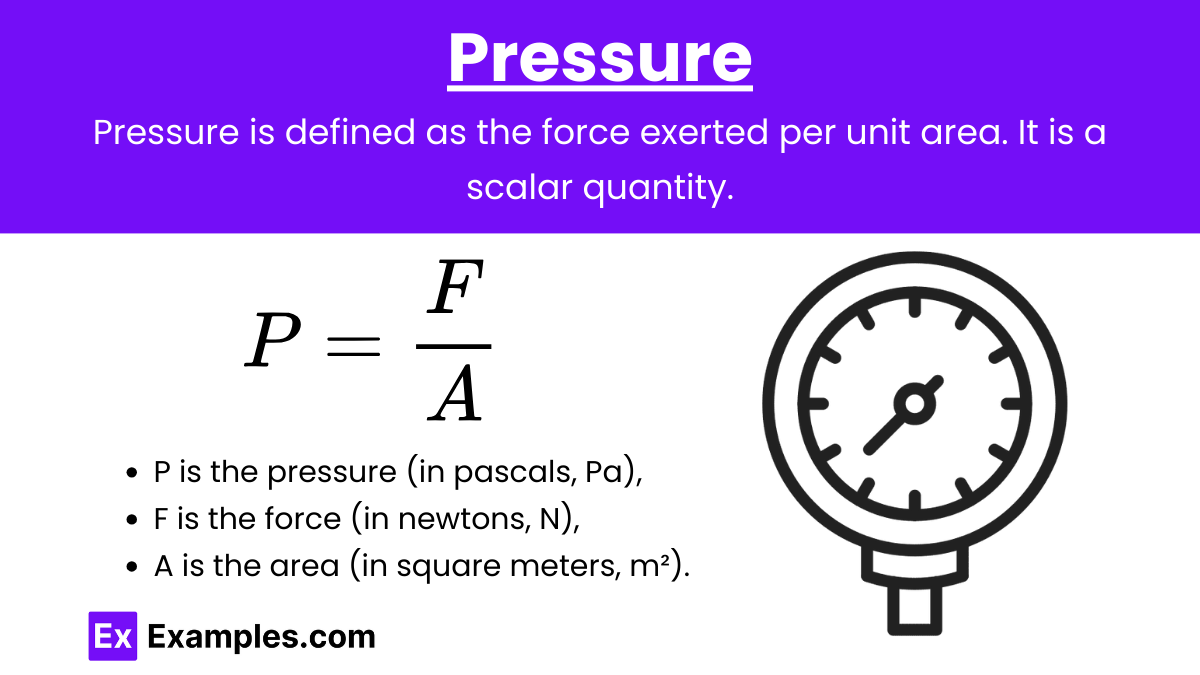
Pressure (P): Pressure is defined as the force exerted per unit area.

Formula: P = \frac{F}{A}

where:

• P is the pressure (in pascals, Pa),

• F is the force (in newtons, N),

• A is the area (in square meters, m²).

Units

• The SI unit of pressure is the Pascal (Pa), where 1 Pa = 1 N/m².

• Other units include atmospheres (atm), bars, and torr.

Characteristics

• Directly Proportional to Force: Increasing the force increases the pressure.

• Inversely Proportional to Area: Increasing the area decreases the pressure for the same force.

Key Points:

• Pressure is a scalar quantity.

• Common units of pressure include pascal (Pa), atmospheres (atm), and torr.

Thermal Equilibrium

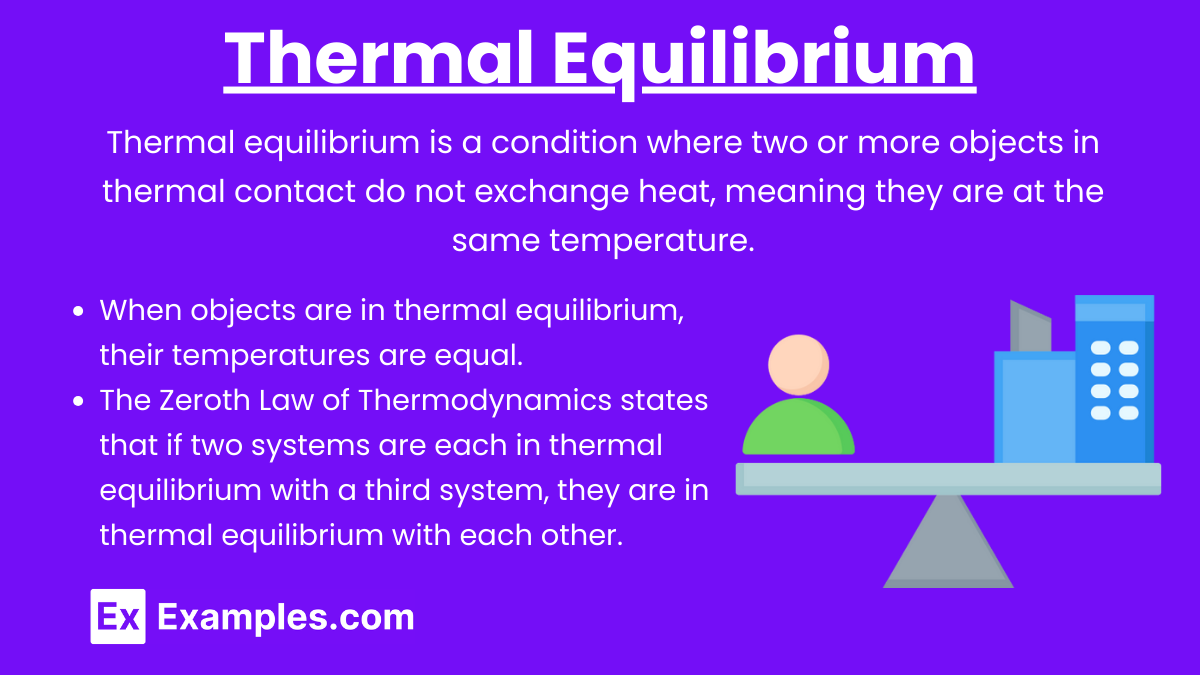
Thermal Equilibrium: Thermal equilibrium is a condition where two or more objects in thermal contact do not exchange heat, meaning they are at the same temperature.

Key Points:

• When objects are in thermal equilibrium, their temperatures are equal.

• The Zeroth Law of Thermodynamics states that if two systems are each in thermal equilibrium with a third system, they are in thermal equilibrium with each other.

Examples

• Thermometer: When a thermometer is placed in a substance, it reaches thermal equilibrium with the substance, allowing accurate temperature measurement.

• Room Temperature: Objects in a room eventually reach thermal equilibrium with the room’s air temperature.

Ideal Gas Law

Ideal Gas Law: The ideal gas law relates the pressure, volume, temperature, and amount of an ideal gas.

Formula: PV = nRT

where:

• P is the pressure (in pascals, Pa),

• V is the volume (in cubic meters, m³),

• n is the number of moles,

• R is the ideal gas constant (8.314 J/(mol\cdotpK))

• T is the temperature (in kelvin, K).

Key Points:

• The ideal gas law assumes that the gas particles do not interact and occupy no volume.

• Real gases deviate from ideal behavior at high pressures and low temperatures.

Assumptions

• Point Particles: Gas particles have negligible volume.

• No Interactions: No intermolecular forces between gas particles.

• Elastic Collisions: Collisions between gas particles and with the container walls are perfectly elastic.

Examples

Example 1: Calculating Pressure

Scenario: A force of 500 N is applied to an area of 0.25 m². Calculate the pressure exerted.

Solution: P = \frac{F}{A}
F = 500 \, \text{N}
A = 0.25 \, \text{m}^2
P = 2000 \, \text{Pa}

Example 2: Thermal Equilibrium

Scenario: A metal rod at 100°C is placed in contact with a block of ice at 0°C. After some time, the rod and ice reach thermal equilibrium. What is their final temperature?

Solution: At thermal equilibrium, the final temperature of both the rod and the ice will be the same, 0°C, assuming no other heat exchanges.

Example 3: Ideal Gas Law (Volume Calculation)

Scenario: A 2.0 mole sample of an ideal gas is at a temperature of 300 K and a pressure of 1 atm. Calculate the volume of the gas.

Solution: First, convert the pressure to pascals: 1atm = 1.01325×10⁵Pa

Use the ideal gas law: PV = nRT V = \frac{nRT}{P} V = \frac{2.0 \times 8.314 \times 300}{1.01325 \times 10^5} V \approx 0.049 \, \text{m}^3

Example 4: Calculating Temperature

Scenario: A 1.5 mole sample of an ideal gas occupies a volume of 22.4 liters at a pressure of 1 atm. Calculate the temperature of the gas.

Solution: First, convert the volume to cubic meters: 22.4L = 0.0224m³

Convert the pressure to pascals: 1atm = 1.01325×10⁵Pa

Use the ideal gas law: PV = nRT T = \frac{PV}{nR} T = \frac{(1.01325 \times 10^5) \times 0.0224}{1.5 \times 8.314} T \approx 181 \, \text{K}

Example 5: Finding the Number of Moles

Scenario: A gas is contained in a 10-liter container at a pressure of 2 atm and a temperature of 400 K. Calculate the number of moles of gas in the container.

Solution: First, convert the volume to cubic meters: 10L = 0.01m³

Convert the pressure to pascals: 2atm = 2×1.01325×10⁵Pa = 2.0265×10⁵Pa

Use the ideal gas law: PV = nRT n = \frac{PV}{RT} n = \frac{(2.0265 \times 10^5) \times 0.01}{8.314 \times 400} n \approx 0.61 \, \text{moles}

Practice Problems

Question 1:

A sealed container with a volume of 2.0 m³ holds an ideal gas at a pressure of 100 kPa and a temperature of 300 K. If the temperature is increased to 600 K, what will be the new pressure, assuming the volume remains constant?

A) 50 kPa
B) 100 kPa
C) 150 kPa
D) 200 kPa

Answer: D) 200 kPa

Explanation:

According to the Ideal Gas Law, PV=nRT. For a constant volume and amount of gas, the relationship between pressure and temperature is given by:

T₁​P₁ ​​= T₂P₂

Given:

• Initial pressure P₁​ = 100kPa

• Initial temperature T₁ ​= 300K

• Final temperature T₂​ = 600K

Solving for P₂:

\frac{100 \, \text{kPa}}{300 \, \text{K}} = \frac{P_2}{600 \, \text{K}} P_2 = \frac{100 \, \text{kPa} \times 600 \, \text{K}}{300 \, \text{K}} = 200 \, \text{kPa}

Thus, the new pressure is 200 kPa.

Question 2:

Two objects, A and B, are in thermal equilibrium. If object A has a higher specific heat capacity than object B, what can be said about their temperatures?

A) Object A is hotter than object B.
B) Object A is cooler than object B.
C) Both objects are at the same temperature.
D) The temperatures fluctuate.

Answer: C) Both objects are at the same temperature.

Explanation:

Thermal equilibrium means that two objects in contact do not exchange heat, implying they are at the same temperature. The specific heat capacity of an object does not affect the equilibrium temperature, only the amount of heat required to change its temperature.

Thus, when objects A and B are in thermal equilibrium, their temperatures are the same, regardless of their specific heat capacities.

Question 3:

A 10-liter container holds an ideal gas at a pressure of 500 kPa and a temperature of 400 K. If the gas is allowed to expand to a volume of 20 liters while the temperature remains constant, what will be the new pressure?

A) 125 kPa
B) 250 kPa
C) 500 kPa
D) 1000 kPa

Answer: B) 250 kPa

Explanation:

According to Boyle's Law for an ideal gas, when the temperature is constant, the pressure and volume have an inverse relationship:

P₁V₁​ = P₂V₂

Given:

• Initial pressure P₁​ = 500kPa

• Initial volume V₁​ = 10L

• Final volume V₂ ​= 20L

Solving for P​₂:

500 \, \text{kPa} \times 10 \, \text{L} = P_2 \times 20 \, \text{L} P_2 = \frac{500 \, \text{kPa} \times 10 \, \text{L}}{20 \, \text{L}} = 250 \, \text{kPa}

Thus, the new pressure is 250 kPa.