Current and Resistance
Current and resistance are fundamental concepts in AP Physics, essential for understanding how electric circuits operate. Electric current is the flow of charge through a conductor, while resistance is the opposition to that flow. These concepts are interconnected through Ohm’s Law, which relates current, voltage, and resistance in a circuit. Mastering these topics is crucial for analyzing various electrical systems, from simple circuits to complex networks, making them key areas of focus in AP Physics studies.
Learning Objectives
In studying Current and Resistance for the AP Physics exam, you should aim to understand the principles of electric current, including the flow of charge, and how resistance affects this flow in different materials and circuits. You should be able to apply Ohm’s Law to solve problems involving voltage, current, and resistance, analyze series and parallel circuits, and use Kirchhoff’s Laws for more complex circuit analysis. Mastery of these concepts will allow you to predict and calculate electrical behavior in various circuit configurations.
1. Electric Current
Electric current is the flow of electric charge through a conductor, typically measured in amperes (A). It represents the movement of electrons within a material.
Formula: \( I = \frac{Q}{t} \)
- I = Electric Current (Amperes, A)
- Q = Electric Charge (Coulombs, C)
- t = Time (Seconds, s)
Types of Current
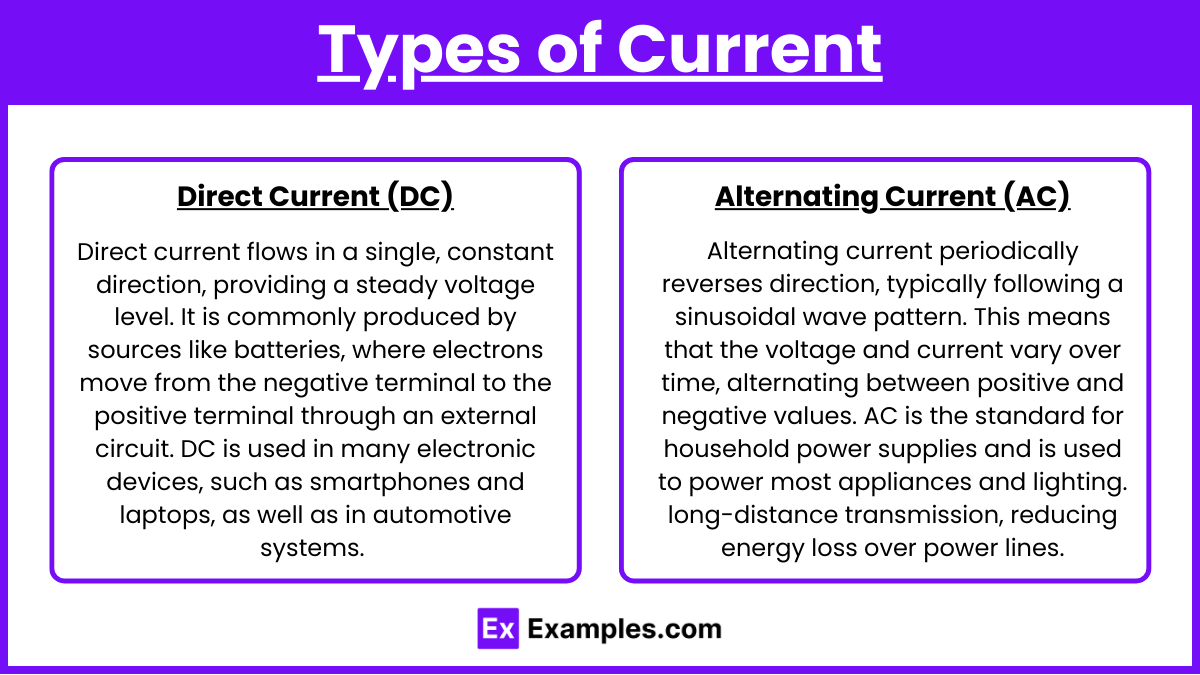
- Direct Current (DC): Direct current flows in a single, constant direction, providing a steady voltage level. It is commonly produced by sources like batteries, where electrons move from the negative terminal to the positive terminal through an external circuit. DC is used in many electronic devices, such as smartphones and laptops, as well as in automotive systems. The consistent flow of current makes DC ideal for applications where stable voltage is crucial.
- Alternating Current (AC): Alternating current periodically reverses direction, typically following a sinusoidal wave pattern. This means that the voltage and current vary over time, alternating between positive and negative values. AC is the standard for household power supplies and is used to power most appliances and lighting. The ability to easily transform AC to different voltage levels using transformers makes it efficient for long-distance transmission, reducing energy loss over power lines. AC’s versatility and efficiency make it the dominant form of electrical power distribution worldwide.
2. Resistance
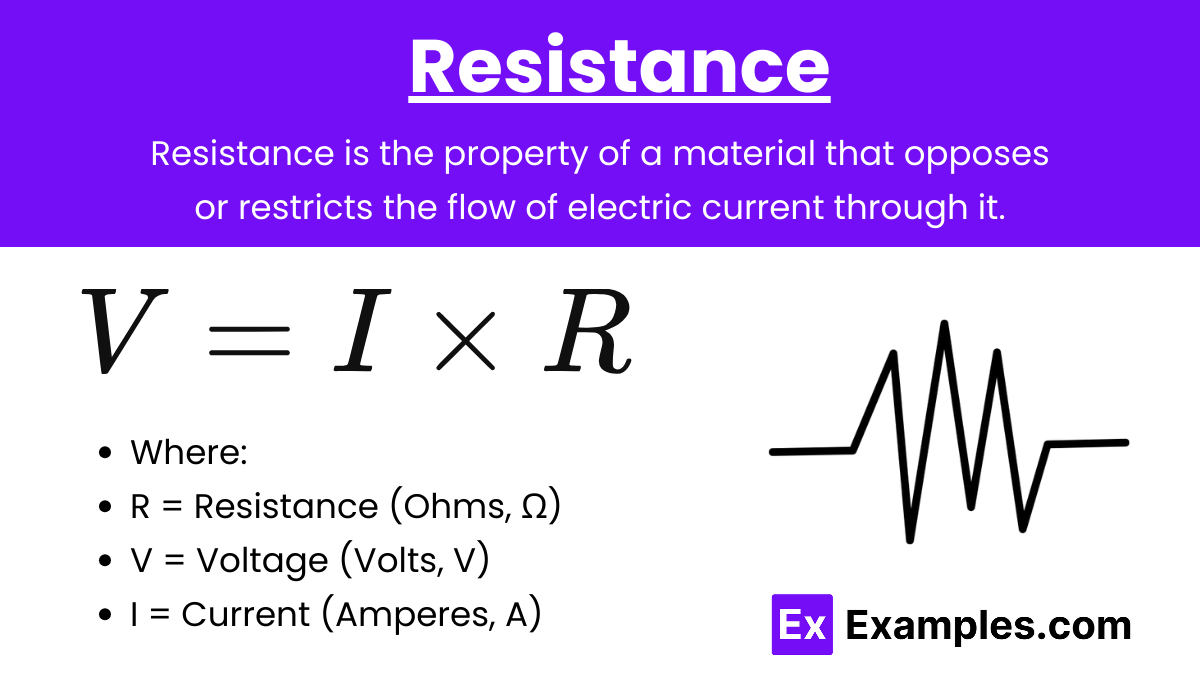
Resistance is the property of a material that opposes or restricts the flow of electric current through it. It quantifies how much the material resists the movement of electrons when a voltage is applied. The higher the resistance, the lower the current for a given voltage. It is a fundamental concept in electrical circuits and is measured in ohms (Ω), where 1Ω is defined as the resistance that allows a current of 1 ampere to flow under a potential difference of 1 volt.
Formula: \( V = I \times R \)
- R = Resistance (Ohms, Ω)
- V = Voltage (Volts, V)
- I = Current (Amperes, A)
Key Factors Affecting Resistance:
- Material: Conductors (like copper) have low resistance, while insulators (like rubber) have high resistance.
- Length of the Conductor: Resistance increases with the length of the conductor.
- Cross-sectional Area: Resistance decreases as the cross-sectional area increases.
- Temperature: For most conductors, resistance increases with temperature.
Ohm’s Law: Ohm’s Law is a fundamental principle that relates voltage (V), current (I), and resistance (R) in an electrical circuit.
\( P = I \times V \)
\( P = I^{2} \times R \)
This law is applicable to ohmic materials, where the resistance remains constant as the voltage and current vary.
3. Resistivity
Resistivity is a material-specific property that quantifies how strongly a material opposes the flow of electric current.
Formula: \( R = \rho \frac{L}{A} \)
- R = Resistance (Ohms, Ω)
- ρ (rho) = Resistivity (Ohm-meter, Ω·m)
- L = Length of the conductor (meters, m)
- A = Cross-sectional area (square meters, m²)
Key Points:
- Resistivity is constant for a given material and depends on its nature and temperature.
- Conductors have low resistivity, while insulators have high resistivity.
4. Power in Electric Circuits
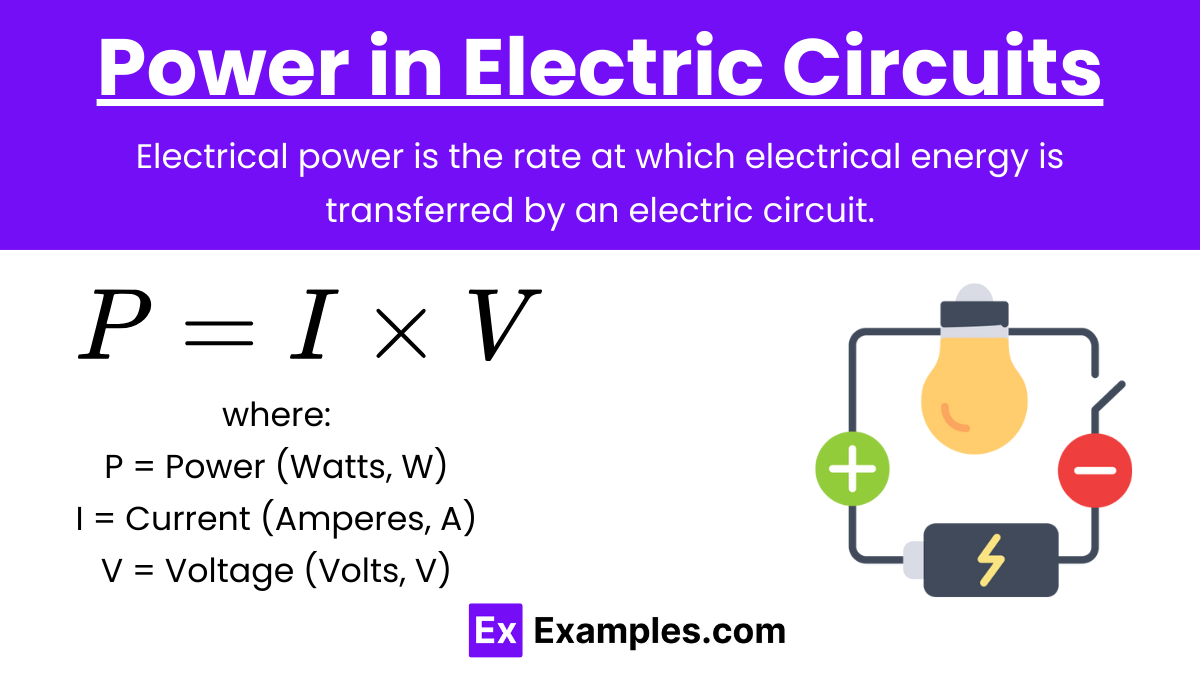
Electrical power is the rate at which electrical energy is transferred by an electric circuit.
Formula: \( P = I \times V \)
- P = Power (Watts, W)
- I = Current (Amperes, A)
- V = Voltage (Volts, V)
Alternative Formulas: Using Ohm’s Law, the power can also be expressed as:
\( P = I^{2} \times R \)
\( P = \frac{V^{2}}{R} \)
Key Concepts:
- Power Dissipation: In resistors, electrical energy is converted into heat, which is why resistors get warm when current flows through them.
- Efficiency: Not all power is converted into useful energy. Some power is always lost as heat due to resistance.
5. Series and Parallel Circuits
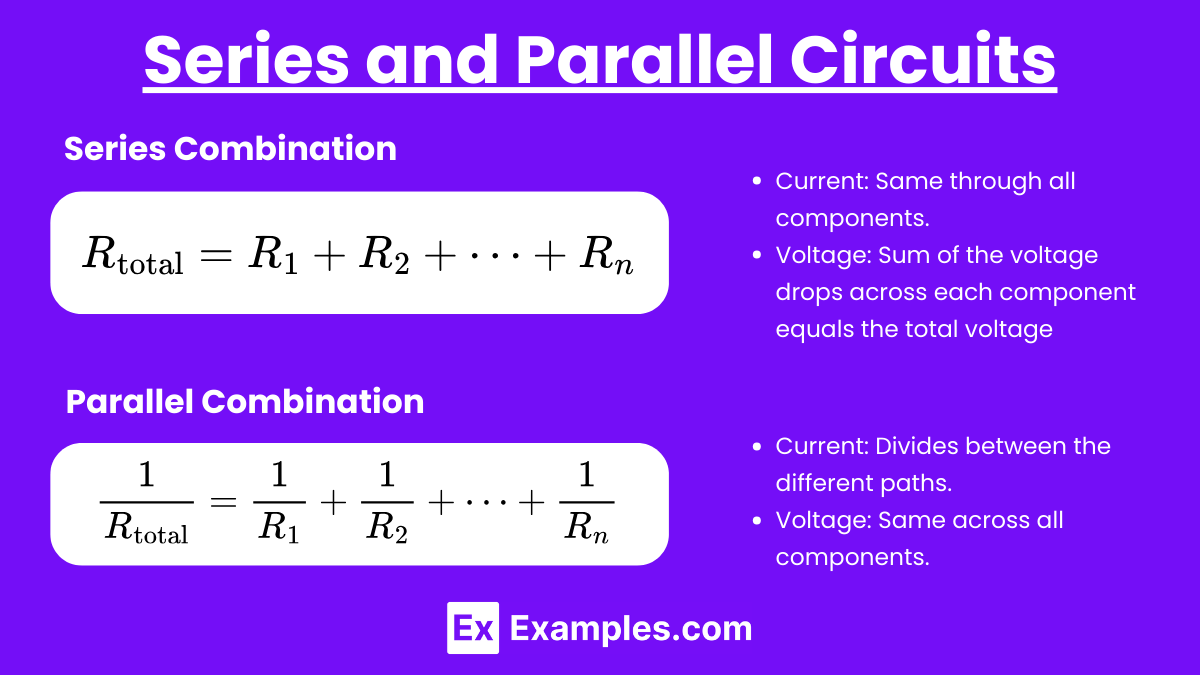
Series Circuits:
- Current: Same through all components.
- Voltage: Sum of the voltage drops across each component equals the total voltage supplied by the source.
- Resistance: Total resistance is the sum of the individual resistances.
- \( R_{\text{total}} = R_{1} + R_{2} + \cdots + R_{n} \)
Parallel Circuits:
- Current: Divides between the different paths.
- Voltage: Same across all components.
- Resistance: The reciprocal of the total resistance is the sum of the reciprocals of the individual resistances.
\( \frac{1}{R_{\text{total}}} = \frac{1}{R_{1}} + \frac{1}{R_{2}} + \cdots + \frac{1}{R_{n}} \)
Key Concepts:
- Series Circuits have higher total resistance and lower total current.
- Parallel Circuits have lower total resistance and higher total current.
6. Kirchhoff’s Laws
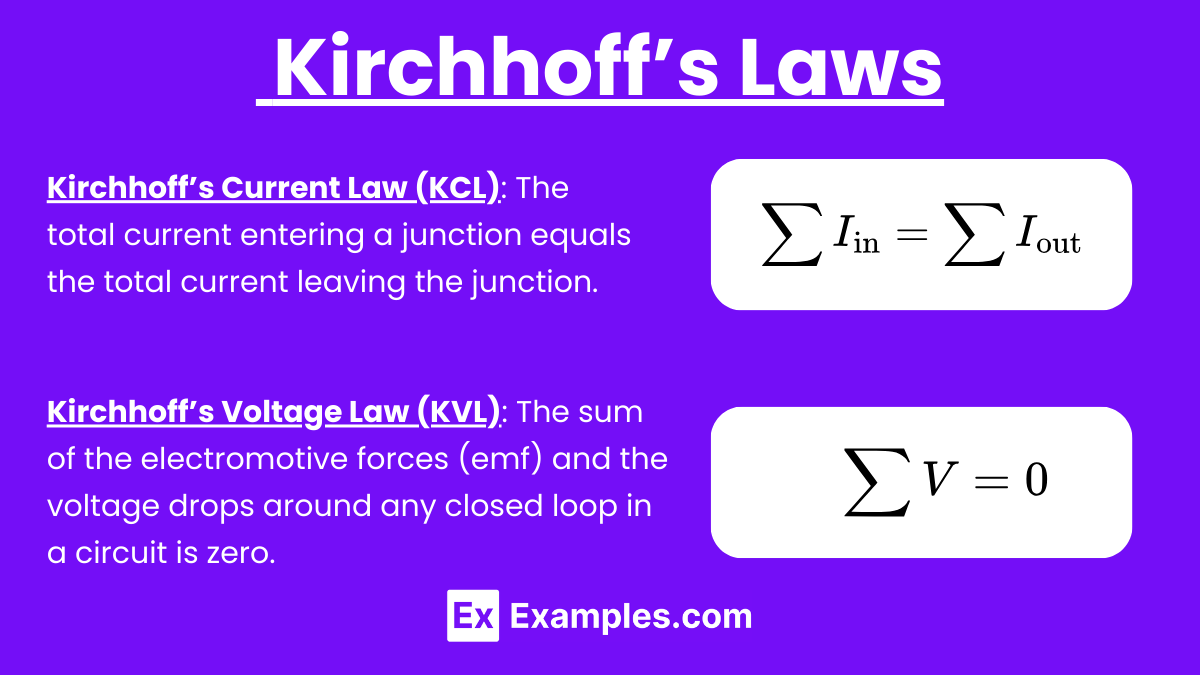
Kirchhoff’s Current Law (KCL): The total current entering a junction equals the total current leaving the junction. \( \sum I_{\text{in}} = \sum I_{\text{out}} \)
Kirchhoff’s Voltage Law (KVL): The sum of the electromotive forces (emf) and the voltage drops around any closed loop in a circuit is zero. ∑V=0
Key Applications:
- Used to analyze complex circuits.
- Essential for solving circuits with multiple loops and junctions.
Examples
Example 1: Electric Kettle Heating Element
The heating element in an electric kettle has a high resistance, which converts electrical energy into heat. When current flows through this resistive element, it heats the water.
Example 2: Resistors in a Circuit
Resistors are components designed to have a specific resistance, controlling the flow of current in a circuit. For example, in an LED circuit, a resistor limits the current to prevent the LED from burning out.
Example 3: Incandescent Light Bulb
The filament inside an incandescent light bulb is made of tungsten, a material with high resistance. When current passes through it, the resistance causes the filament to heat up and emit light.
Example 4: Electric Stove Burner
The coils of an electric stove burner are made of a resistive material. When electric current flows through the coils, the resistance generates heat, which is used for cooking.
Example 5: Car Headlights
Car headlights use a resistive filament similar to a light bulb. The resistance in the filament causes it to glow and produce light when current flows through it, illuminating the road ahead.
Multiple Choice Questions
Question 1
What happens to the current in a circuit if the resistance is doubled, assuming the voltage remains constant?
A) The current doubles
B) The current remains the same
C) The current is halved
D) The current is reduced to one-fourth
Answer: C) The current is halved
Explanation: According to Ohm’s Law, I=VR, where III is the current, V is the voltage, and R is the resistance. If the resistance R is doubled and the voltage V remains constant, the current III will decrease because current is inversely proportional to resistance. Specifically, if resistance doubles, the current is reduced to half of its original value.
Question 2
Which of the following materials is likely to have the highest electrical resistance?
A) Copper
B) Silver
C) Gold
D) Rubber
Answer: D) Rubber
Explanation: Electrical resistance is a property that opposes the flow of electric current. Conductors, such as copper, silver, and gold, have low resistance, making them good at conducting electricity. Insulators like rubber have very high resistance, which prevents the flow of electric current. Therefore, rubber is likely to have the highest electrical resistance among the given options.
Question 3
If the voltage across a resistor is tripled while the resistance remains the same, what happens to the current through the resistor?
A) The current triples
B) The current doubles
C) The current remains the same
D) The current is reduced to one-third
Answer: A) The current triples
Explanation: Again, using Ohm’s Law I=VR, where III is the current, V is the voltage, and R is the resistance. If the voltage V is tripled while the resistance R remains constant, the current III will increase proportionally to the voltage. Therefore, tripling the voltage will result in tripling the current.