Electric field and electric potential

Last Updated: September 23, 2024

Notes

Understanding electric fields and electric potential is crucial for mastering electricity and magnetism concepts in the AP Physics exam. These topics cover the behavior of charges in electric fields and the energy associated with electric potentials. Below are detailed notes on electric fields and electric potential, along with five examples to help you achieve a high score on your AP Physics exam.

Free AP Physics C: Electricity and Magnetism Practice Test

Learning Objectives

By studying Electric Field and Electric Potential, you should learn how to calculate electric field strength, understand the concept of electric potential energy, and apply Coulomb’s law. You should be able to describe the relationship between electric field and electric potential, analyze the behavior of electric charges in various configurations, and solve problems involving electric force and potential energy. Mastery of these concepts will help you understand how electric fields influence charged particles and the potential energy within electric systems.

Electric Field

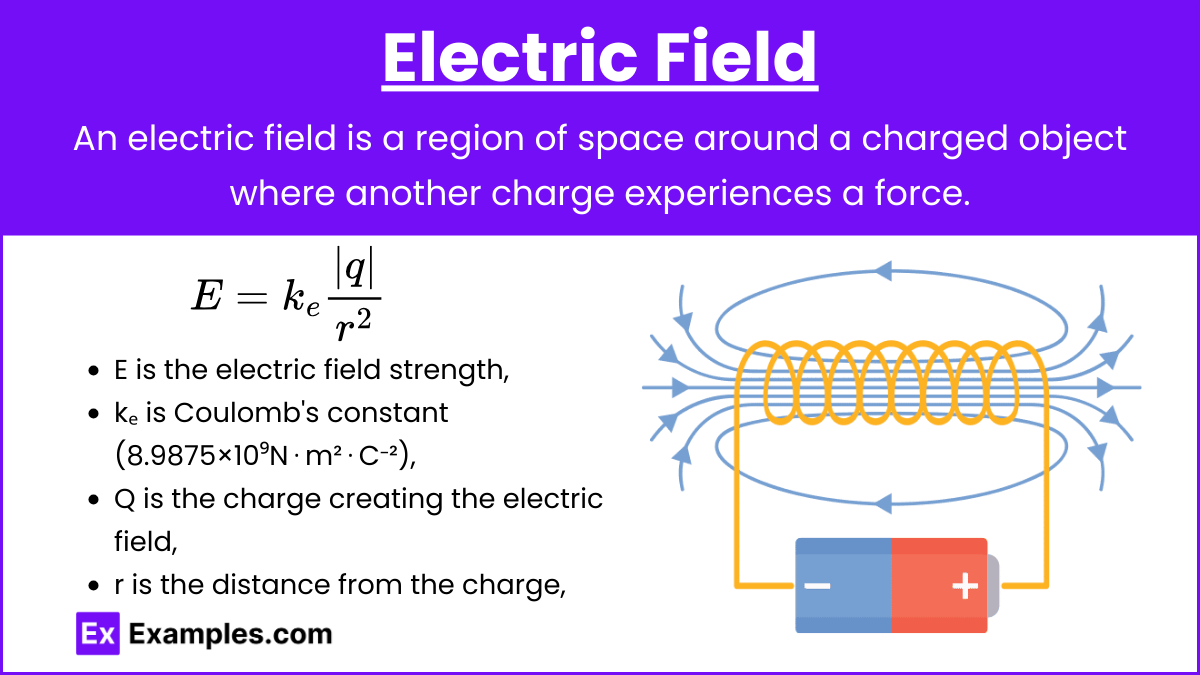
Electric Field (E): An electric field is a region around a charged object where another charged object experiences a force.

The electric field E due to a point charge q at a distance r is given by:

$E = k_e \frac{|q|}{r^2}$

where:

E is the electric field strength (in N/C),
kₑ is Coulomb’s constant (8.9875×10⁹ N⋅m²⋅C⁻²),
q is the charge creating the field (in C),
r is the distance from the charge (in m).

Key Points

The direction of the electric field is away from positive charges and towards negative charges.
The unit of electric field is Newtons per Coulomb (N/C).

Electric Potential

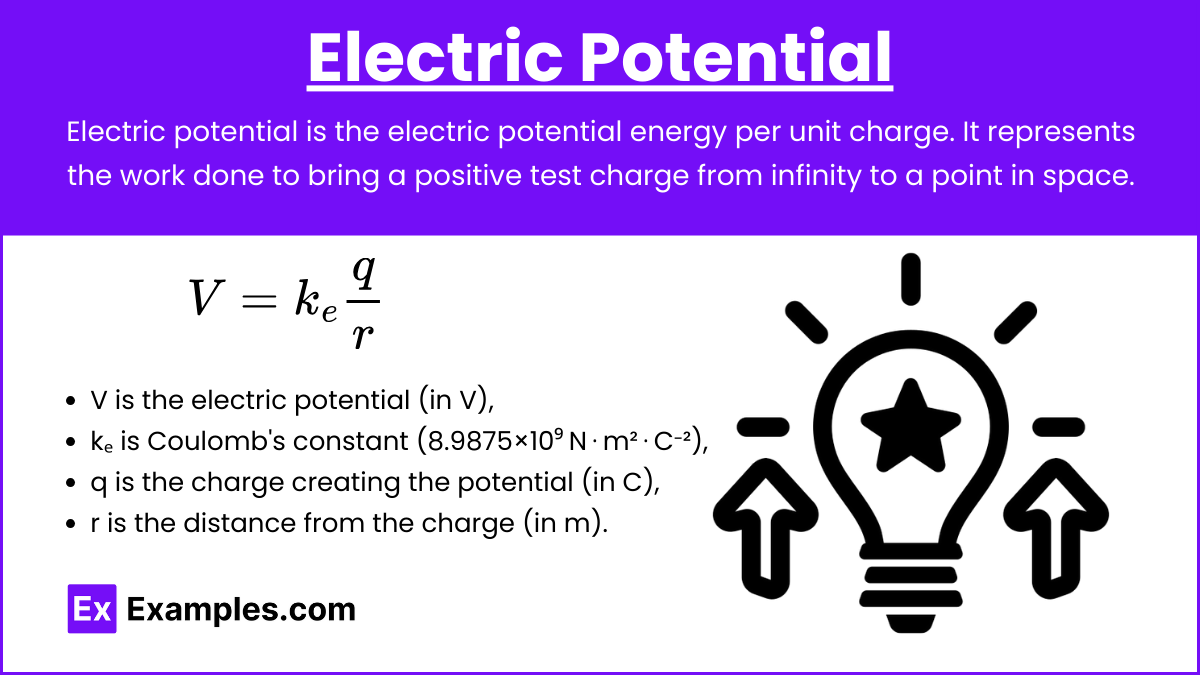
Electric Potential (V): Electric potential is the electric potential energy per unit charge. It represents the work done to bring a positive test charge from infinity to a point in space.

Formula

The electric potential V due to a point charge q at a distance r is given by:

where:

$V = k_e \frac{q}{r}$

V is the electric potential (in V),
kₑ is Coulomb’s constant (8.9875×10⁹ N⋅m²⋅C⁻²),
q is the charge creating the potential (in C),
r is the distance from the charge (in m).

Key Points

The unit of electric potential is Volts (V).
The electric potential due to multiple charges is the algebraic sum of the potentials due to individual charges.

Relationship Between Electric Field and Electric Potential

The electric field E is related to the electric potential V by the negative gradient of the potential: E = −∇V

In one dimension, this simplifies to:

$E = -\frac{dV}{dx}$

Example 1

Electric Field Due to a Point Charge

Scenario: Calculate the electric field at a point 0.2 meters away from a +5μC charge.

Solution:

$E = k_e \frac{q}{r^2}$

$E = 8.9875 \times 10^9 \frac{5 \times 10^{-6}}{(0.2)^2}$

$E = 8.9875 \times 10^9 \frac{5 \times 10^{-6}}{0.04}$

$E = 1.12 \times 10^6 \, \text{N/C}$

Example 2

Electric Potential Due to a Point Charge

Scenario: Find the electric potential at a distance of 0.3 meters from a +3μC charge.

Solution:

$V = k_e \frac{q}{r}$

$V = 8.9875 \times 10^9 \frac{3 \times 10^{-6}}{0.3}$

$V = 8.9875 \times 10^9 \times 1 \times 10^{-5}$

$V = 8.99 \times 10^4 \, \text{V}$

Example 3

Superposition of Electric Fields

Scenario: Two charges, q₁ = +2μC and q₂ = −3μC, are placed 0.5 meters apart. Calculate the electric field at a point midway between them.

Solution: Distance from each charge to the midpoint:

$r = \frac{0.5}{2} = 0.25 \, \text{m}$

Electric field due to q₁ at the midpoint:

$E_1 = k_e \frac{q}{r^2}$

$E_1 = 8.9875 \times 10^9 \frac{2 \times 10^{-6}}{(0.25)^2}$

$E_1 = 2.87 \times 10^5 \, \text{N/C}$

Electric field due to q₂ at the midpoint:

$E_2 = k_e \frac{q_2}{r^2}$

$E_2 = 8.9875 \times 10^9 \frac{3 \times 10^{-6}}{(0.25)^2}$

$E_2 = 4.31 \times 10^5 \, \text{N/C}$

Since q₁ is positive and q₂ is negative, their electric fields at the midpoint are in the same direction:

$E_{\text{net}} = E_1 + E_2$

$E_{\text{net}} = 2.87 \times 10^5 + 4.31 \times 10^5$

$E_{\text{net}} = 7.18 \times 10^5 \, \text{N/C}$

Example 4

Electric Potential Due to Multiple Charges

Scenario: Calculate the electric potential at a point 0.2 meters away from a +1μC charge and 0.3 meters away from a −2μC charge.

Solution: Electric potential due to +1μC charge:

$V_1 = k_e \frac{q_1}{r_1}$

$V_1 = 8.9875 \times 10^9 \frac{1 \times 10^{-6}}{0.2}$

$V_1 = 4.49 \times 10^4 \, \text{V}$

Electric potential due to −2μC charge:

$V_2 = k_e \frac{q_2}{r_2}$

$V_2 = 8.9875 \times 10^9 \frac{2 \times 10^{-6}}{0.3}$

$V_2 = 5.99 \times 10^4 \, \text{V}$

Net electric potential:

$V_{\text{net}} = V_1 + V_2$

$V_{\text{net}} = 4.49 \times 10^4 - 5.99 \times 10^4$

$V_{\text{net}} = -1.5 \times 10^4 \, \text{V}$

Example 5

Work Done by Electric Field

Scenario: Calculate the work done by an electric field in moving a +2μC charge from a point where the potential is 100V to a point where the potential is 50V.

Solution:

$W = q \Delta V$

$W = (2 \times 10^{-6})(50 - 100)$

$W = (2 \times 10^{-6})(-50)$

$W = -1 \times 10^{-4} \, \text{J}$

$W = -0.1 \, \text{mJ}$

Practice Problems

Question 1

Which of the following correctly describes the relationship between electric field (E) and electric potential (V)?

A) The electric field is the gradient of the electric potential.
B) The electric potential is the gradient of the electric field.
C) The electric field is perpendicular to the electric potential.
D) The electric potential and electric field are unrelated.

Answer: A) The electric field is the gradient of the electric potential.

Explanation:

The electric field (E) is related to the electric potential (V) by the following relationship:

E = −∇V

This means the electric field is the negative gradient of the electric potential. It shows how the electric potential changes in space, and the negative sign indicates that the electric field points in the direction of decreasing potential.

Question 2

A point charge q is placed at the origin. What is the electric potential V at a distance r from the charge?

$\text{A) } V = \frac{k q}{r^2}$

$\text{B) } V = \frac{k q}{r}$

$\text{C) } V = k q r$

$\text{D) } V = k q r^2$

Answer: B)

$V = \frac{k q}{r}$

Explanation:

The electric potential V due to a point charge q at a distance r from the charge is given by:

$V = \frac{k q}{r}$

where k is Coulomb’s constant (k≈8.99 × 10⁹N m²/C²). This formula shows that the potential decreases with increasing distance from the charge.

Question 3

What is the direction of the electric field at a point in space relative to the electric potential at that point?

A) The electric field points in the direction of increasing potential.
B) The electric field points in the direction of decreasing potential.
C) The electric field points perpendicular to the potential gradient.
D) The electric field direction is independent of the potential.

Answer: B) The electric field points in the direction of decreasing potential.

Explanation:

The electric field (E) is directed from regions of higher electric potential to regions of lower electric potential. This is expressed mathematically by the relation:

E = −∇V

The negative sign indicates that the electric field points in the direction of the steepest decrease in electric potential. Thus, the electric field points in the direction where the potential decreases most rapidly.