Electric Potential due to Point Charges and Uniform Fields

Last Updated: October 10, 2024

Notes

Electric potential is a crucial concept in electromagnetism, describing the work done to move a unit charge to a specific point in an electric field. In AP Physics, understanding electric potential due to point charges and uniform fields is essential for solving complex problems. It involves calculating the potential from single and multiple charges, as well as understanding potential differences in uniform fields. Mastery of these topics is key to excelling in the AP Physics exam and gaining a deep comprehension of electric forces and energy interactions.

Free AP Physics C: Electricity and Magnetism Practice Test

Learning Objectives

In studying Electric Potential due to Point Charges and Uniform Fields for the AP Physics exam, you should aim to understand the concept of electric potential and how it relates to point charges and uniform electric fields. Master the formulas for calculating electric potential due to single and multiple point charges and the potential difference in a uniform electric field. Develop the ability to apply the superposition principle and solve related problems. Grasp the physical significance of electric potential and its applications in real-world scenarios.

Electric Potential due to Point Charges and Uniform Fields

Electric potential is a fundamental concept in electromagnetism, describing the potential energy per unit charge at a point in an electric field. It is crucial for understanding electric forces and energy interactions in physics. This guide covers electric potential due to point charges and uniform electric fields, providing detailed explanations and examples to help you excel in your AP Physics exam.

Electric Potential Due to a Point Charge

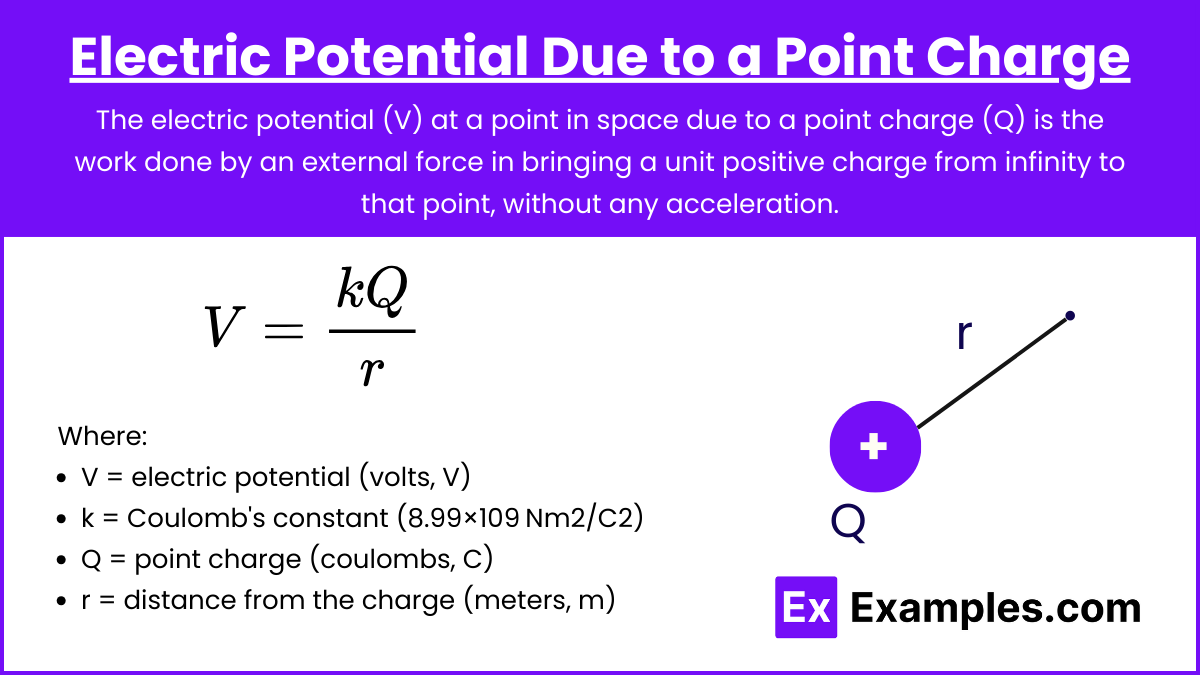
The electric potential (V) at a point in space due to a point charge (Q) is the work done by an external force in bringing a unit positive charge from infinity to that point, without any acceleration.

Formula

The electric potential V at a distance rrr from a point charge Q is given by:

$V = \frac{kQ}{r}$

where:

V = electric potential (volts, V)
k = Coulomb’s constant (8.99×10⁹ Nm²/C²)
Q = point charge (coulombs, C)
r = distance from the charge (meters, m)

Electric Potential Due to Multiple Point Charges

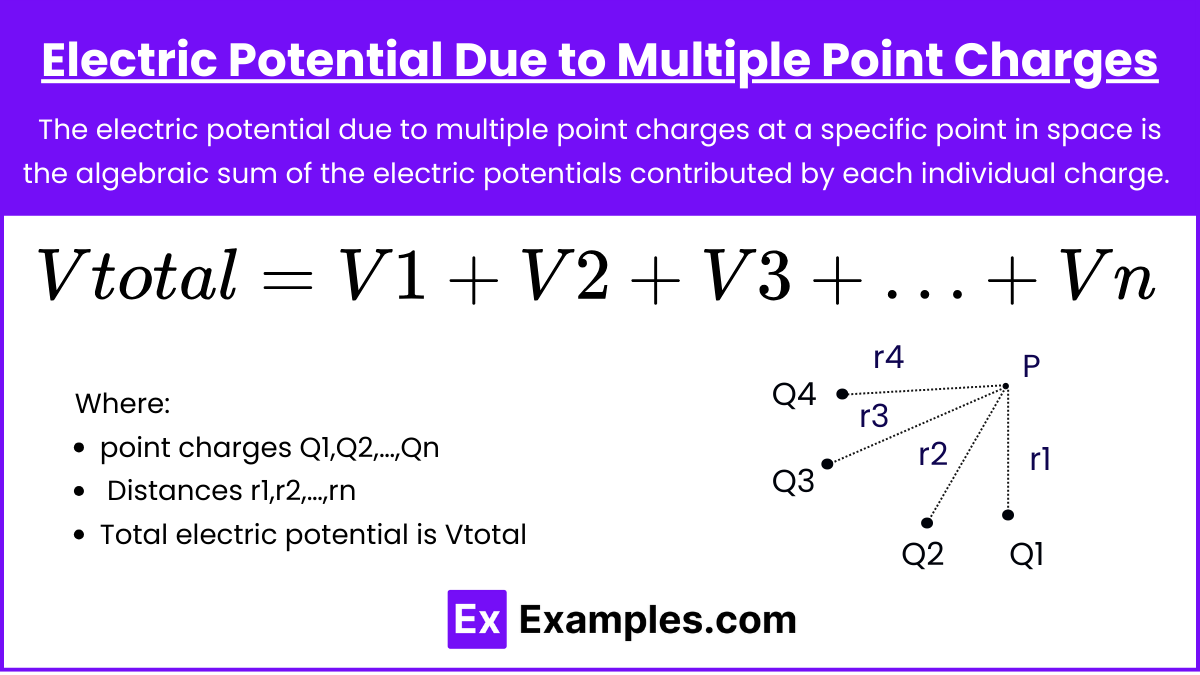
The electric potential due to multiple point charges at a specific point in space is the algebraic sum of the electric potentials contributed by each individual charge. This principle follows from the superposition principle in electromagnetism, which states that the total potential at any point is equal to the sum of the potentials due to each charge considered independently.

Superposition Principle

When dealing with multiple point charges, the total electric potential at a point is the algebraic sum of the potentials due to each charge.

For point charges Q1,Q2,…,Qn located at distances r1,r2,…,rn respectively from the point of interest, the total electric potential Vtotal is given by:

Formula

V_total = V₁ +V₂ +V₃ +…+ V_n

where V_iis the potential due to each point charge.

Electric Potential in a Uniform Electric Field

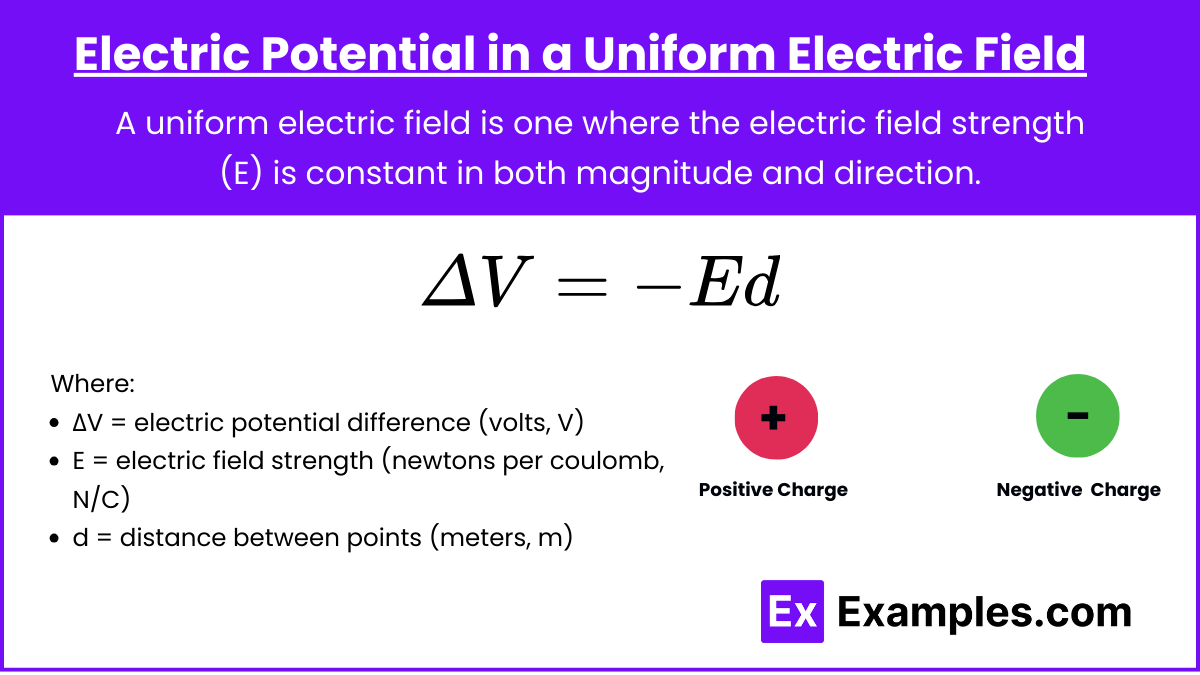
A uniform electric field is one where the electric field strength (E) is constant in both magnitude and direction. The electric potential difference (ΔV) between two points in a uniform field is related to the electric field strength and the distance (d) between the points.

Formula

ΔV = −Ed

where:

ΔV = electric potential difference (volts, V)
E = electric field strength (newtons per coulomb, N/C)
d = distance between points (meters, m)

Key Concepts and Formulas Summary

Electric Potential (V): $V = \frac{kQ}{r}$
Superposition Principle: V_total = V₁ + V₂ + V₃ +…+ V_n
Uniform Electric Field: ΔV = −Ed

Understanding electric potential due to point charges and uniform fields is essential for mastering electromagnetism in physics. By mastering these concepts and formulas, you will be well-prepared for the AP Physics exam. Practice solving problems and applying these principles to different scenarios to reinforce your knowledge and problem-solving skills.

Examples

Example 1

Electric Potential of a Single Point Charge

Problem: Calculate the electric potential at a distance of 0.3 meters from a point charge of 2 μC

Solution:

Q=2×10⁻⁶C
r=0.3 mr = 0.3
V=8.99×10⁹×2×10⁻⁶
V=5.993×10⁴ V

Example 2

Electric Potential of Multiple Point Charges

Problem: Find the electric potential at a point equidistant from two charges, Q₁=5 μC and Q2=−3 μC placed 0.4 meters apart.

Solution:

Let the point be 0.2 meters from each charge.

$V_{1} = \frac{8.99 \times 10^{9} \times 5 \times 10^{-6}}{0.2}$
$V_{1} = 224.75 \times 10^{3} \, V$
$V_{2} = \frac{8.99 \times 10^{9} \times -3 \times 10^{-6}}{0.2}$ $V_{2} = -134.85 \times 10^{3} \, V$
$V_{\text{total}} = 224.75 \times 10^{3} + \left( -134.85 \times 10^{3} \right)$
$V_{\text{total}} = 89.9 \times 10^{3} \, V$

Example 3

Potential Difference in a Uniform Electric Field

Problem: Calculate the potential difference between two points 1.5 meters apart in a uniform electric field of 200N/C.

Solution:

$E = 200 \, \text{N/C}$
$d = 1.5 \, \text{m}$
$\Delta V = -Ed$
$\Delta V = -200 \times 1.5$
$\Delta V = -300 \, \text{V}$

Example 4

Electric Potential Due to a Combination of Point Charges

Problem: Determine the electric potential at a point 0.2 meters from a 4 μC charge and 0.4 meters from a −2 μC charge.

Solution:

$V_{1} = \frac{8.99 \times 10^{9} \times 4 \times 10^{-6}}{0.2}$
$V_{1} = 179.8 \times 10^{3} \, V$
$V_{2} = \frac{8.99 \times 10^{9} \times -2 \times 10^{-6}}{0.4}$ $V_{2} = -44.95 \times 10^{3} \, V$
$V_{\text{total}} = 179.8 \times 10^{3} + \left( -44.95 \times 10^{3} \right)$
$V_{\text{total}} = 134.85 \times 10^{3} \, V$

Example 5

Electric Potential in a Non-Uniform Electric Field

Problem: Consider a point charge 3 μC located at the origin. Calculate the electric potential at a point 0.1 meters away along the x-axis and 0.2 meters away along the y-axis.

Solution:

For the x- axis :
$r = 0.1 \, \text{m}$
$V_x = \frac{8.99 \times 10^9 \times 3 \times 10^{-6}}{0.1}$
$V_x = 269.7 \times 10^3 \, \text{V}$
for the y – axis :
$r = 0.2 \, \text{m}$
$V_y = \frac{8.99 \times 10^9 \times 3 \times 10^{-6}}{0.2}$
$V_y = 134.85 \times 10^3 \, \text{V}$

These examples demonstrate various scenarios involving electric potential due to point charges and uniform fields, helping to solidify your understanding of these concepts.

Multiple Choice Questions

Question 1

Which of the following statements is true about the electric potential at a point due to a single point charge?

A) The electric potential is directly proportional to the distance from the charge.
B) The electric potential is inversely proportional to the square of the distance from the charge.
C) The electric potential is inversely proportional to the distance from the charge.
D) The electric potential is directly proportional to the square of the distance from the charge.

Answer: C

Explanation: The electric potential V at a distance rrr from a point charge Q is given by the equation V=kQ/r, where k is Coulomb’s constant. This shows that the electric potential is inversely proportional to the distance r from the charge. Thus, as the distance increases, the potential decreases, and vice versa.

Question 2

Two point charges, +Q and −Q, are placed a certain distance apart. At the midpoint between these charges, the electric potential is:

A) Zero
B) Positive
C) Negative
D) Infinite

Answer: A

Explanation: The electric potential at a point is the algebraic sum of the potentials due to individual charges. At the midpoint between two equal and opposite charges +Q and −Q, the potentials due to each charge will have the same magnitude but opposite signs. Hence, they will cancel each other out, resulting in a net electric potential of zero at the midpoint.

Question 3

In a uniform electric field, the electric potential difference between two points separated by a distance ddd is given by:

A) Ed
B) E/d
C) E²d
D) E/d²

Answer: A

Explanation: In a uniform electric field E, the potential difference ΔV between two points separated by a distance ddd in the direction of the field is given by ΔV=Ed. This is because the potential difference in a uniform field is directly proportional to both the strength of the field and the distance over which it is applied. The uniform field assumption means the field strength E is constant, and the potential difference is thus the product of E and ddd.