Electrostatics With Conductors

Last Updated: September 23, 2024

Notes

Electrostatics with conductors is a fundamental topic in AP Physics, focusing on the behavior of electric charges within conductive materials. Conductors allow free movement of electrons, leading to unique properties such as zero electric field inside and surface charge distribution in electrostatic equilibrium. This topic explores the principles governing charge distribution, induced charges, and electric fields around conductors. Understanding these concepts is crucial for mastering various applications in physics, such as capacitors and electrostatic shielding.

Free AP Physics C: Electricity and Magnetism Practice Test

Learning Objectives

In studying “Electrostatics with Conductors” for the AP Physics exam, you should aim to understand the behavior of conductors in electric fields, including charge distribution and electric field inside and outside conductors. Master the application of Gauss’s law to determine electric fields and potentials. Learn the principles of capacitors, including their construction, function, and calculations. Grasp concepts such as electrostatic shielding and induced charges. These objectives will help you apply theoretical knowledge to practical scenarios and solve related physics problems effectively.

Electrostatics deals with the study of forces, fields, and potentials arising from static charges. Conductors play a crucial role in electrostatics because they allow charges to move freely within them, which significantly influences the behavior of electric fields and potentials.

Conductors in Electrostatics

Conductors are materials that permit the free movement of electric charge. In electrostatics, the properties of conductors include:

Free Electrons: Conductors have free electrons that can move throughout the material.
Equilibrium State: In electrostatic equilibrium, the electric field inside a conductor is zero.
Surface Charge Distribution: Excess charge resides on the surface of conductors.
Equipotential Surface: The surface of a conductor in electrostatic equilibrium is an equipotential surface.

Key Concepts of Conductors

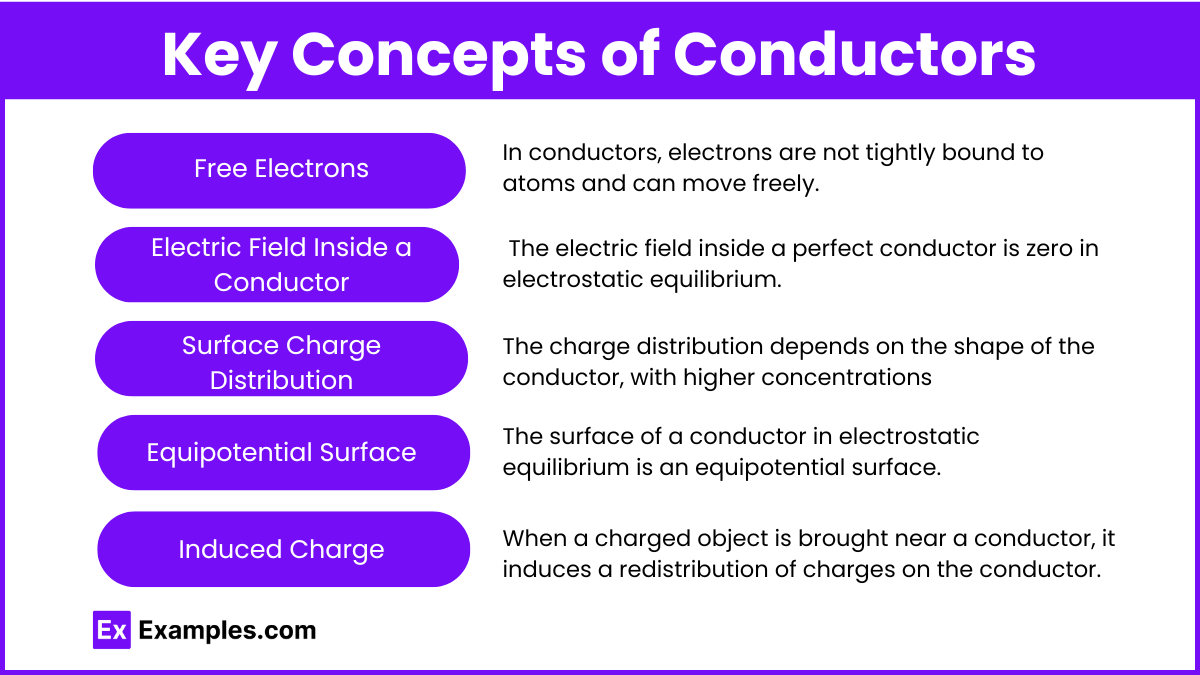
Conductors are materials that allow the free movement of electric charge, primarily electrons. Understanding the behavior of conductors in electrostatics involves several key concepts:

1. Free Electrons

Definition: In conductors, electrons are not tightly bound to atoms and can move freely.
Example: Metals like copper and aluminum have a sea of free electrons that facilitate the flow of electric current.

2. Electric Field Inside a Conductor

Property: The electric field inside a perfect conductor is zero in electrostatic equilibrium.
Reason: Free electrons rearrange themselves to cancel any internal electric field.

3. Surface Charge Distribution

Behavior: Excess charge resides on the surface of a conductor.
Distribution: The charge distribution depends on the shape of the conductor, with higher concentrations at points of sharp curvature (e.g., edges and tips).

4. Equipotential Surface

Characteristic: The surface of a conductor in electrostatic equilibrium is an equipotential surface.
Implication: No work is needed to move a charge along the surface, as the potential is the same everywhere.

5. Induced Charge

Phenomenon: When a charged object is brought near a conductor, it induces a redistribution of charges on the conductor.
Result: This creates regions of positive and negative charges on the surface, even if the conductor is initially neutral.

Applications of Electrostatics with Conductors

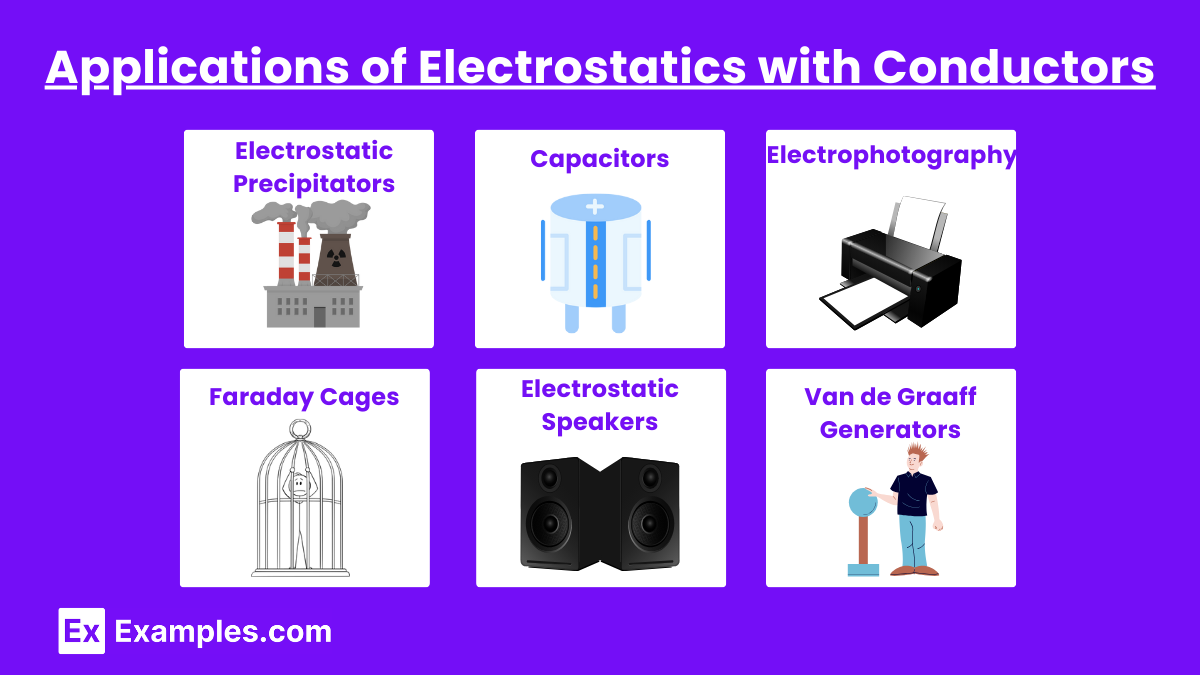
Electrostatics with conductors has several practical applications in various fields, utilizing the principles of charge distribution and electric potential. Here are some notable applications:

1. Electrostatic Precipitators

Function: Remove particulate matter from industrial emissions.
Mechanism: Charged plates attract and capture dust and smoke particles from the air, improving air quality.

2. Capacitors

Function: Store and release electrical energy.
Mechanism: Consist of two conductive plates separated by an insulating material. When connected to a voltage source, charges accumulate on the plates, creating an electric field and storing energy.

3. Electrophotography (Xerography)

Function: Used in photocopiers and laser printers.
Mechanism: A photoconductive surface is charged in darkness, and an image is projected onto it. Light exposure discharges the illuminated areas, leaving a charge pattern that attracts toner particles to form a visible image.

4. Faraday Cages

Function: Shield sensitive electronic equipment from external electric fields.
Mechanism: A conductive enclosure blocks external static and non-static electric fields by redistributing charge on its surface.

5. Electrostatic Speaker

Function: Convert electrical signals into sound.
Mechanism: An electrostatic speaker uses a thin, electrically charged diaphragm placed between two conductive plates. When an audio signal is applied to the plates, it creates an electrostatic field that causes the diaphragm to move, producing sound waves with high fidelity and low distortion.

6. Van de Graaff Generators

Function: Generate high voltages for educational demonstrations and experiments.
Mechanism: A moving belt transfers charge to a hollow metal sphere, creating a high electric potential and strong electric field.

Mathematical Analysis

Gauss’s Law

Gauss’s law is a fundamental principle that relates the electric flux through a closed surface to the charge enclosed by the surface. For conductors, Gauss’s law is often used to determine the electric field near charged surfaces.

Gauss’s law states:

$\oint \vec{E} \cdot d\vec{A} = \frac{Q_{\text{enc}}}{\epsilon_{0}}$

where E is the electric field, dA is a vector representing an infinitesimal area on the closed surface, and Q_enc is the charge enclosed by the surface.

Example: Spherical Conductor

Consider a charged spherical conductor with radius R and total charge Q. Using Gauss’s law, the electric field outside the sphere (at a distance rrr from the center) is:

$E = \frac{1}{(4 \pi \epsilon_{0})} \frac{Q}{r^{2}}$

Inside the conductor, the electric field is zero.

$E = 0 \text{ for } (r < R)$

Capacitance of a Spherical Capacitor

For a spherical capacitor consisting of two concentric spherical conductors with radii R1 (inner) and R2 (outer), the capacitance C is:

$C = 4 \pi \epsilon_{0} \frac{(R_{1} R_{2})}{(R_{2} - R_{1})}$

Important Considerations

Charge Distribution on Conductors: Charges redistribute quickly in response to external fields.
Influence of Shape: Sharp points on conductors can lead to higher charge densities and stronger local electric fields.
Potential Difference: The potential difference between two points in a conductor in electrostatic equilibrium is zero.

Examples

Example 1 : Charging by Induction

When a charged object is brought near a neutral conductor, the free electrons in the conductor are either attracted or repelled, depending on the charge of the object. This causes a redistribution of charges within the conductor, creating an induced charge on its surface.

Example 2 : Faraday Cage

A Faraday cage is a conductive enclosure that blocks external static and non-static electric fields. When an external electric field is applied, the charges in the conductor rearrange themselves to cancel the field inside, protecting the interior from external electrostatic influences.

Example 3: Grounding a Conductor

Connecting a conductor to the Earth with a conductive path allows excess charge to dissipate. This is because the Earth can accept or supply an unlimited amount of charge, effectively neutralizing the conductor.

Example 4 : Electrostatic Shielding

Placing a conductor between a region of space and an external electric field can shield that region from the field. The conductor’s free electrons move to oppose the external field, thus nullifying its effect within the shielded area.

Example 5 : Capacitors

Capacitors consist of two conductive plates separated by an insulator (dielectric). When a voltage is applied, one plate accumulates positive charge while the other accumulates an equal amount of negative charge, creating an electrostatic field in the dielectric. This allows capacitors to store electrical energy.

Multiple Choice Questions

Question 1

Which of the following statements about conductors in electrostatic equilibrium is true?

A. The electric field inside a conductor is always zero.
B. The electric field inside a conductor is always uniform.
C. The charge density inside a conductor is non-zero.
D. Excess charges reside on the surface of the conductor.

Answer: D. Excess charges reside on the surface of the conductor.

Explanation:
In electrostatic equilibrium, conductors exhibit several key properties:

Electric Field Inside a Conductor: The electric field inside a conductor is zero. This is because the free electrons within the conductor move until they reach a configuration where the internal electric field cancels any applied field.
Charge Distribution: Excess charges in a conductor move to the surface. This occurs because the like charges repel each other and spread out to minimize the repulsive forces.
Charge Density Inside a Conductor: The charge density inside a conductor is zero because any excess charge moves to the surface.

Thus, the correct statement is that excess charges reside on the surface of the conductor.

Question 2

If a hollow conducting sphere is given a net positive charge, how is the charge distributed?

A. The charge is uniformly distributed throughout the volume of the sphere.
B. The charge resides only on the inner surface of the sphere.
C. The charge resides only on the outer surface of the sphere.
D. The charge is distributed equally between the inner and outer surfaces.

Answer: C. The charge resides only on the outer surface of the sphere.

Explanation:
For a hollow conducting sphere in electrostatic equilibrium:

Inner Surface: If there are no charges inside the cavity of the sphere, there is no induced charge on the inner surface.
Outer Surface: All the net positive charge resides on the outer surface. This is because charges repel each other and move to the position where they are farthest apart, which is the outer surface of the conductor.

Therefore, the net positive charge given to the sphere will reside entirely on the outer surface.

Question 3

What happens to the electric potential inside a conductor in electrostatic equilibrium?

A. The electric potential is highest at the surface and decreases to zero inside.
B. The electric potential is lowest at the surface and increases inside.
C. The electric potential is constant throughout the conductor.
D. The electric potential varies linearly with distance from the center.

Answer: C. The electric potential is constant throughout the conductor.

Explanation:
In electrostatic equilibrium, the electric potential within a conductor is constant. This is due to the following reasons:

Movement of Charges: Free electrons within the conductor move in response to any electric field until the field is neutralized, resulting in no net movement of charge within the conductor.
Electric Field Inside a Conductor: Since the electric field inside a conductor is zero, there is no potential difference within the conductor. Hence, the potential must be the same at every point within the conductor.