Forces on Current Carrying Wires in Magnetic Fields

Learn the forces on current-carrying wires in magnetic fields is crucial in electromagnetism. When a wire carrying an electric current is placed in a magnetic field, it experiences a force due to the interaction between the current and the magnetic field. This principle, essential for AP Physics, is governed by the Lorentz Force Law. Mastering these concepts is vital for solving problems related to electric motors, measuring instruments, and other electromagnetic applications in real-world technology.

Learning Objectives

Understand the Lorentz Force Law and how it applies to current-carrying wires in magnetic fields. Learn to calculate the magnitude and direction of the force using F=I(L×B). Master the right-hand rule for determining force direction. Understand torque on current loops and its applications in devices like electric motors. Be able to solve related problems, demonstrating proficiency in these fundamental electromagnetism concepts.

Lorentz Force Law

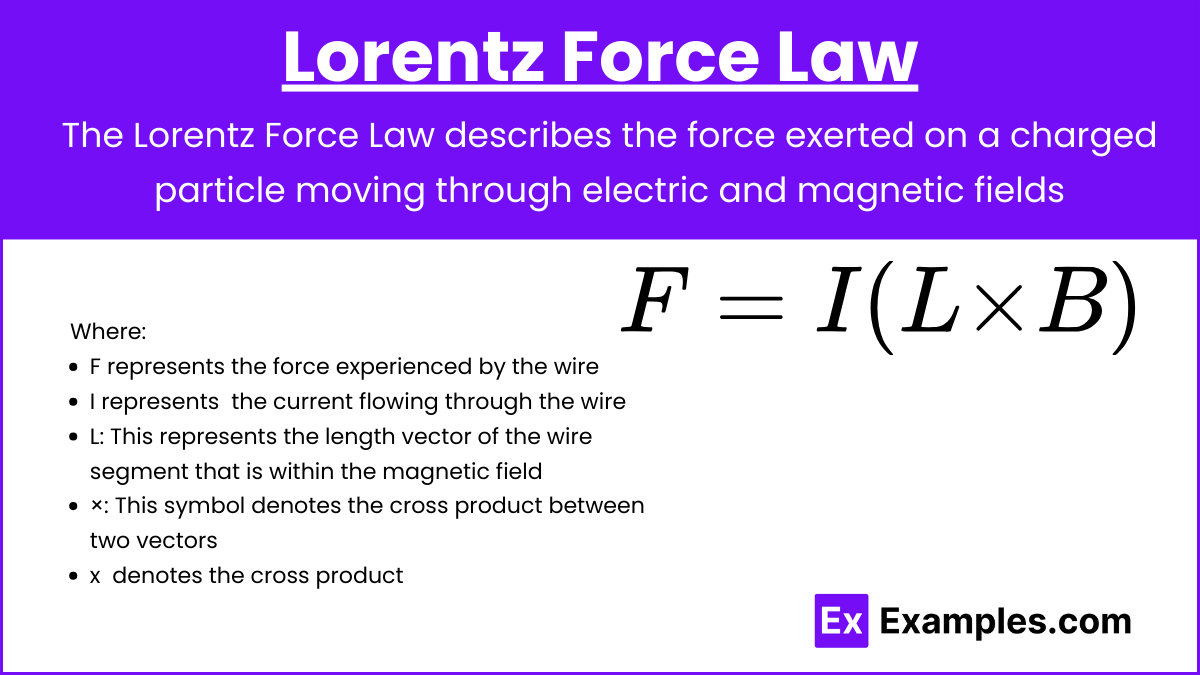
The Lorentz Force Law describes the force experienced by a charged particle moving through a magnetic field. For a current-carrying wire, this force can be expressed as:

F=I(L×B)

Where:

• F is the force on the wire
• I is the current flowing through the wire
• L is the length vector of the wire within the magnetic field
• B is the magnetic field vector
• × denotes the cross product

Direction of the Force

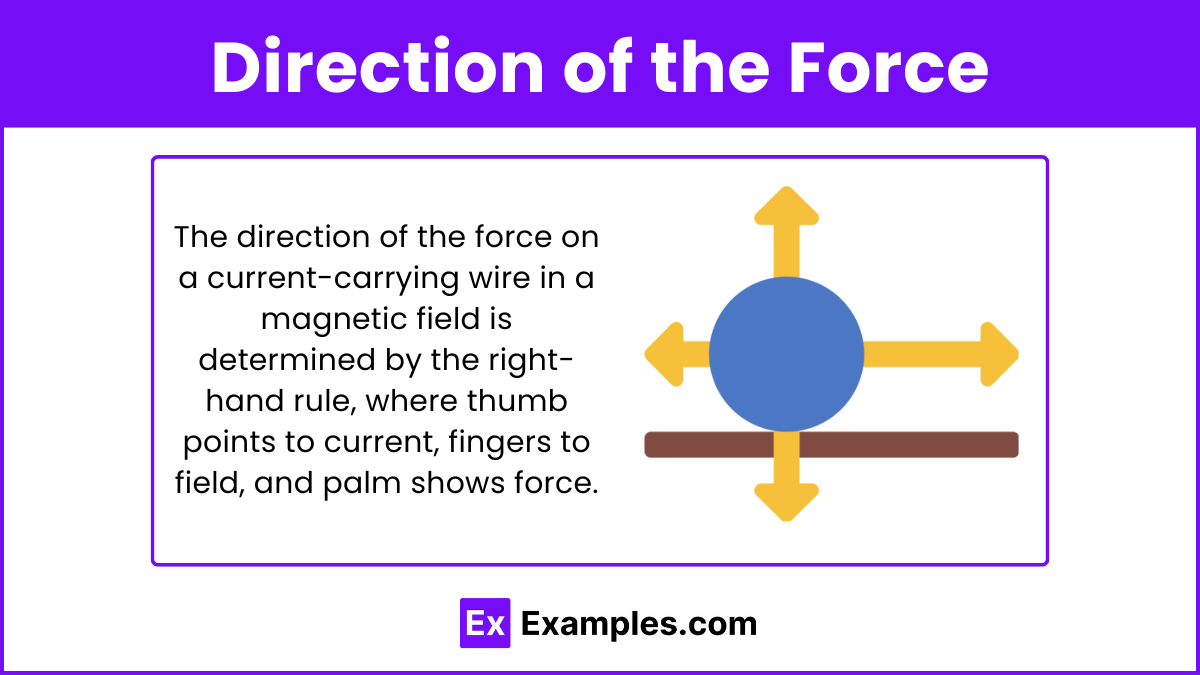
The direction of the force on the wire can be determined using the right-hand rule:

1. Point your thumb in the direction of the current (III).
2. Point your fingers in the direction of the magnetic field (B\mathbf{B}B).
3. Your palm will face in the direction of the force (F\mathbf{F}F).

Magnitude of the Force

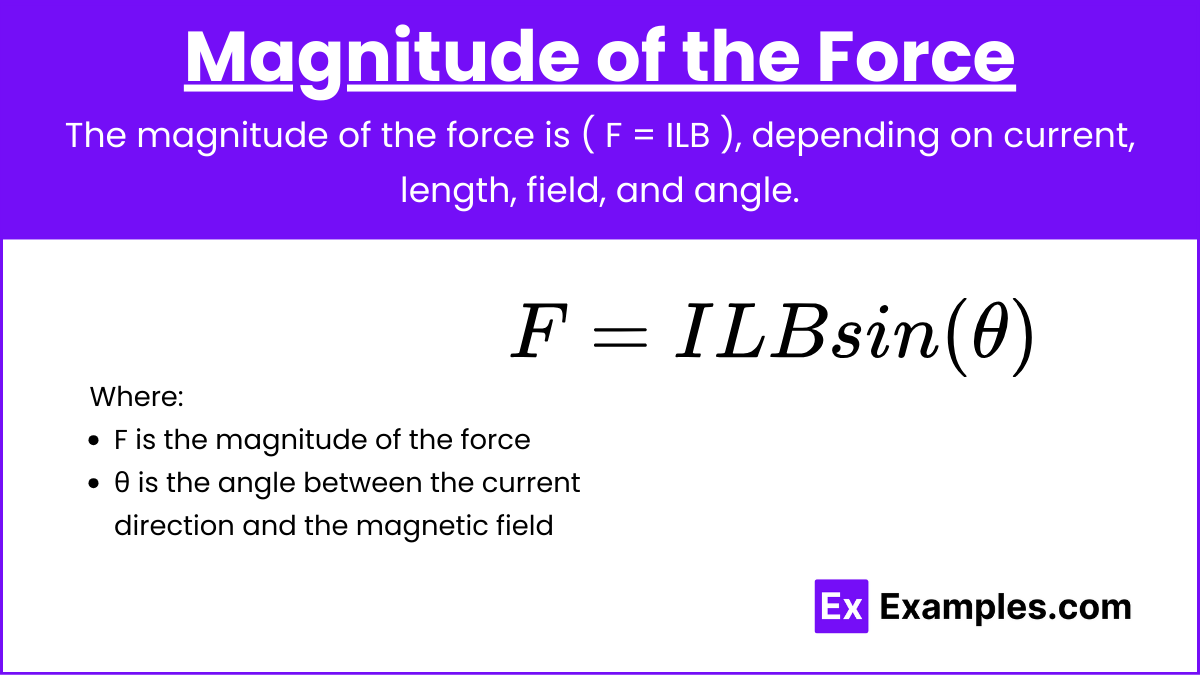
The magnitude of the force is given by:

F=ILBsin⁡(θ)

Where:

• F is the magnitude of the force
• θ is the angle between the current direction and the magnetic field

Example Problem

A wire carrying a current of 5 A is placed in a uniform magnetic field of 0.3 T. The length of the wire within the magnetic field is 0.2 m and the wire is oriented perpendicular to the magnetic field. Calculate the force on the wire.

Solution:

Since the wire is perpendicular to the magnetic field, θ=90∘ sin⁡(90∘)=1

F=ILBsin⁡(θ)

F=(5 A)(0.2 m)(0.3 T)(1)

F = 0.3N

So, the force on the wire is 0.3 N.

Magnetic Force on a Current Loop

When a current-carrying wire forms a loop, it experiences a force that can lead to torque, causing the loop to rotate. This principle is used in electric motors.

Torque on a Current Loop

The torque (τ\tauτ) on a current-carrying loop in a magnetic field is given by:

τ=nIABsin⁡(θ)

Where:

• n is the number of turns in the loop
• A is the area of the loop
• θ is the angle between the normal to the loop and the magnetic field

Example Problem

A rectangular loop with 3 turns, carrying a current of 2 A, has dimensions 0.1 m by 0.05 m and is placed in a magnetic field of 0.4 T. The plane of the loop is perpendicular to the magnetic field. Calculate the torque on the loop.

Solution:

Since the loop is perpendicular to the magnetic field, θ=90∘ and sin(90∘)=1.

A=length×width=(0.1 m)(0.05 m)=0.005 m²

τ=nIABsin⁡(θ)

τ=(3)(2 A)(0.005 m²)

τ=0.012 Nm

So, the torque on the loop is 0.012 Nm.

Applications

Electric Motors

Electric motors utilize the principle of forces on current-carrying wires in magnetic fields. By arranging loops of wire within a magnetic field and passing current through them, the resulting torque causes the loops to rotate, converting electrical energy into mechanical energy.

Measuring Instruments

Devices like galvanometers use the force on current-carrying wires to measure electric current. The deflection of a needle connected to a wire loop in a magnetic field provides a measure of the current passing through the wire.

Magnetic Levitation

Magnetic levitation (maglev) trains use powerful electromagnets and the forces between current-carrying wires and magnetic fields to achieve frictionless, high-speed transportation.

Examples of Forces on Current Carrying Wires in Magnetic Fields

1. Straight Wire in a Magnetic Field: A wire carrying a current of 10 A placed perpendicular to a 0.5 T magnetic field experiences a force calculated using F=ILB For a 1 m length, the force is 5 N.
2. Electric Motor Armature: In an electric motor, a rectangular coil with multiple loops carries current through a magnetic field, generating torque that rotates the armature, converting electrical energy to mechanical energy.
3. Galvanometer Needle Deflection: A current-carrying coil in a galvanometer placed in a magnetic field experiences a torque that causes the needle to deflect proportionally to the current.
4. Maglev Train Propulsion: Maglev trains use superconducting coils to generate currents that interact with magnetic fields, producing lift and propulsion forces, allowing frictionless high-speed travel.
5. Loudspeaker Operation: In a loudspeaker, an alternating current passes through a coil in a magnetic field, causing the coil and attached diaphragm to move, producing sound waves through force interaction.

Multiple Choice Questions on Forces on Current Carrying Wires in Magnetic Fields

Question 1

Which rule helps determine the direction of the force on a current-carrying wire in a magnetic field?

A. Left-hand rule

B. Right-hand rule

C. Ampere’s law

D. Faraday’s law

Answer: B. Right-hand rule

Explanation: The right-hand rule is used to determine the direction of the force on a current-carrying wire in a magnetic field. When you point your thumb in the direction of the current and your fingers in the direction of the magnetic field, your palm will point in the direction of the force.

Question 2

What happens to the force on a current-carrying wire if the direction of the magnetic field is reversed?

A. The force doubles.

B. The force remains the same.

C. The force is halved.

D. The force reverses direction.

Answer: D. The force reverses direction

Explanation: If the direction of the magnetic field is reversed, the direction of the force on the current-carrying wire also reverses. This is because the force direction depends on the cross product of the current direction and the magnetic field direction.

Question 3

In which scenario is the force on a current-carrying wire zero?

A. The wire is parallel to the magnetic field.

B. The wire is perpendicular to the magnetic field.

C. The current is zero.

D. Both A and C

Answer: D. Both A and C

Explanation: The force on a current-carrying wire is zero when the wire is parallel to the magnetic field, as there is no perpendicular component to create force. Additionally, if there is no current, there is no force regardless of the magnetic field direction.