The study of forces on moving charges in magnetic fields is fundamental in understanding electromagnetism. In the context of AP Physics, this topic covers the Lorentz force, the right-hand rule for direction determination, and the resulting motion, such as circular and helical paths of charged particles. Applications include particle accelerators like cyclotrons and natural phenomena like auroras. Mastery of these concepts is crucial for excelling in the AP Physics exam and for understanding broader physical principles.
Free AP Physics C: Electricity and Magnetism Practice Test
Learning Objectives
learn how to calculate the magnetic force on a moving charge using the Lorentz force equation, understand the application of the right-hand rule to determine the direction of the force, and analyze the motion of charged particles in uniform magnetic fields, including circular and helical paths. Mastery of these concepts, along with their applications in cyclotrons, synchrotrons, and natural phenomena like auroras, is essential for a comprehensive understanding of forces on moving charges in magnetic fields.
Magnetic Force on a Moving Charge
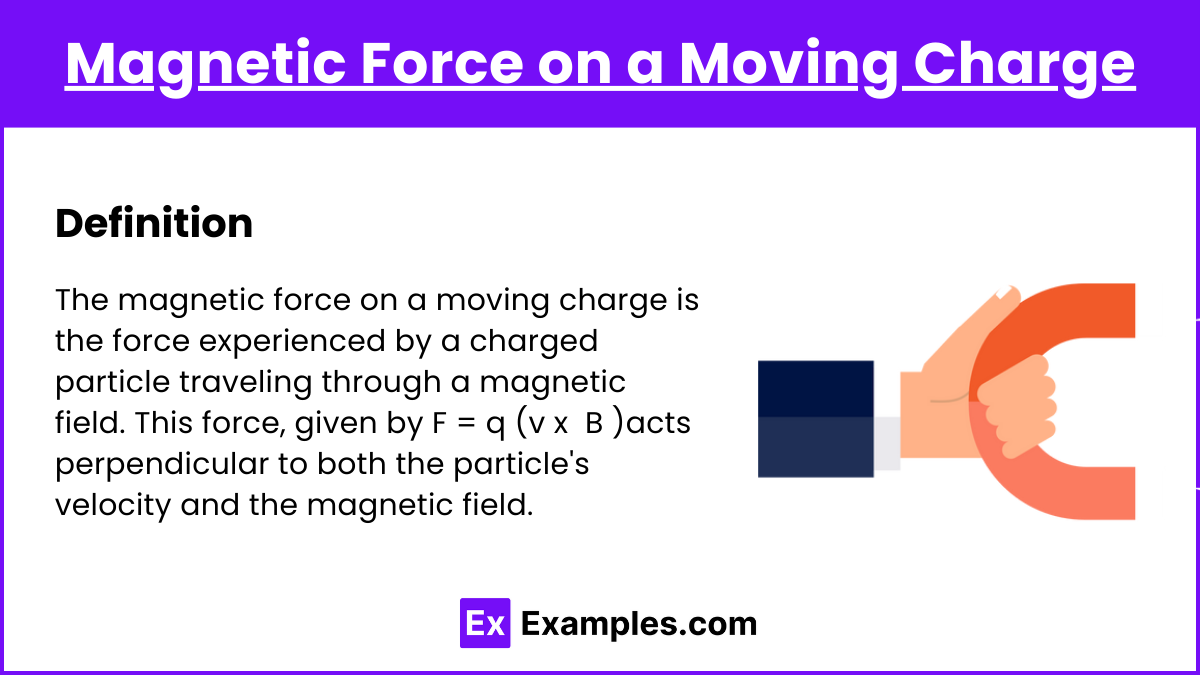
When a charged particle moves through a magnetic field, it experiences a force known as the Lorentz force. This force is perpendicular to both the velocity of the particle and the magnetic field.
Lorentz Force Formula
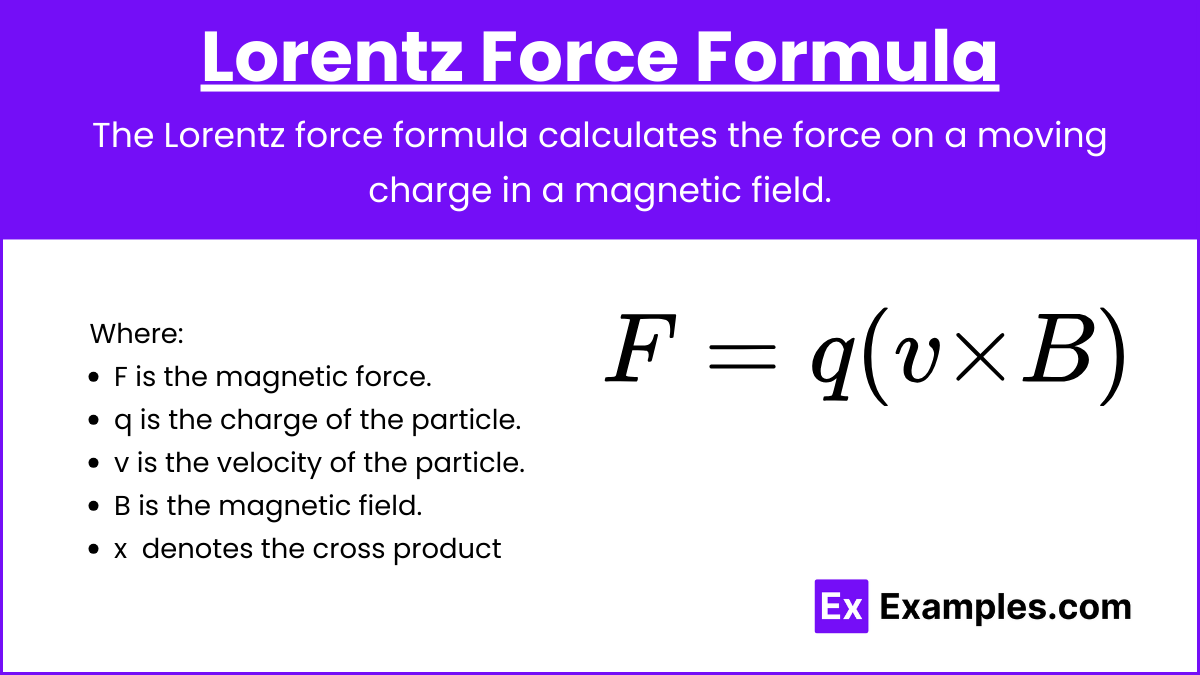
The magnitude of the force F acting on a charge q moving with velocity v in a magnetic field B is given by:
F=q(v×B)
q = charge of the particle (Coulombs, C)
v = velocity of the particle (meters per second, m/s)
B = magnetic field strength (Tesla, T)
Right-Hand Rule
To determine the direction of the magnetic force, use the right-hand rule:
Point your fingers in the direction of v (velocity).
Orient your palm so that when you curl your fingers, they point in the direction of B (magnetic field).
Your thumb points in the direction of the magnetic force F for a positive charge. For a negative charge, the force direction is opposite to your thumb.
Motion of Charged Particles
The motion of charged particles in magnetic fields can be circular, helical, or straight-line, depending on the initial conditions and field configuration.
Circular Motion in Magnetic Fields
When a charged particle moves perpendicular to a uniform magnetic field, it follows a circular path due to the centripetal force provided by the magnetic force.
Radius of Circular Path
The radius rrr of the circular path is given by:
m = mass of the particle (kilograms, kg)
v = speed of the particle (meters per second, m/s)
q = charge of the particle (Coulombs, C)
B = magnetic field strength (Tesla, T)
Period of Circular Motion
The period T of the circular motion is:
This period is independent of the particle's speed and depends only on its mass, charge, and the magnetic field strength.
Helical Motion
If the velocity of the particle has a component parallel to the magnetic field, the particle follows a helical path. The parallel component of the velocity remains constant, while the perpendicular component results in circular motion.
Examples of Forces on Moving Charges in Magnetic Fields
Electron in a Cathode Ray Tube (CRT):
Electrons are accelerated and directed using magnetic fields to create images on older television and computer screens.
Protons in a Cyclotron:
Protons are accelerated in circular paths using magnetic fields, increasing their energy for use in medical treatments or research.
Charged Particles in the Earth's Magnetosphere:
Solar wind particles interact with Earth’s magnetic field, causing them to spiral along magnetic field lines, resulting in auroras.
Electrons in a Mass Spectrometer:
Electrons are deflected by a magnetic field to separate ions based on their mass-to-charge ratio, aiding in the analysis of chemical substances.
Charged Particles in the Large Hadron Collider (LHC):
Protons and other particles are accelerated and collided at high speeds using strong magnetic fields, allowing scientists to study fundamental particles and forces.
Practice Test Questions on Forces on Moving Charges in Magnetic Fields
Question 1
When a charged particle moves perpendicular to a uniform magnetic field, what is the shape of its path?
A. Straight line
B. Circular
C. Elliptical
D. Parabolic
Answer: B. Circular
Explanation: When a charged particle moves perpendicular to a uniform magnetic field, it experiences a magnetic force that acts perpendicular to both its velocity and the magnetic field. This force acts as a centripetal force, causing the particle to move in a circular path.
Question 2
What is the primary factor that determines the direction of the force on a moving charge in a magnetic field?
A. The magnitude of the charge
B. The speed of the charge
C. The direction of the velocity and the magnetic field
D. The mass of the particle
Answer: C. The direction of the velocity and the magnetic field
Explanation: The direction of the magnetic force on a moving charge is determined by the direction of the charge's velocity and the magnetic field, according to the right-hand rule. The force is perpendicular to both the velocity of the particle and the magnetic field.
Question 3
Which application relies on the principles of charged particles moving in magnetic fields to separate ions based on their mass-to-charge ratio?
A. Cyclotron
B. Cathode Ray Tube (CRT)
C. Mass Spectrometer
D. Electric Motor
Answer: C. Mass Spectrometer
Explanation: A mass spectrometer uses magnetic fields to separate ions based on their mass-to-charge ratio. As ions move through the magnetic field, they experience a force that deflects them in a path whose curvature depends on their mass-to-charge ratio, allowing for their identification and analysis.