Gauss’s Law

Gauss’s Law, a fundamental principle in electromagnetism, relates the electric flux through a closed surface to the charge enclosed by that surface. It simplifies the calculation of electric fields in cases with high symmetry, such as spherical, cylindrical, and planar distributions. In AP Physics, understanding Gauss’s Law is crucial for solving complex electrostatics problems efficiently. This law not only helps in deriving electric field equations but also deepens the comprehension of the relationship between electric charges and field lines.

Free AP Physics C: Electricity and Magnetism Practice Test

Learning Objectives

Master the statement and mathematical formulation of Gauss’s Law, including the concepts of electric flux and permittivity of free space. Identify and choose appropriate Gaussian surfaces based on symmetry (spherical, cylindrical, and planar) to simplify electric field calculations. Apply Gauss’s Law to determine electric fields for various charge distributions such as point charges, line charges, and plane charges. Recognize how Gauss’s Law relates to Coulomb’s Law in electrostatics.

Introduction to Gauss’s Law

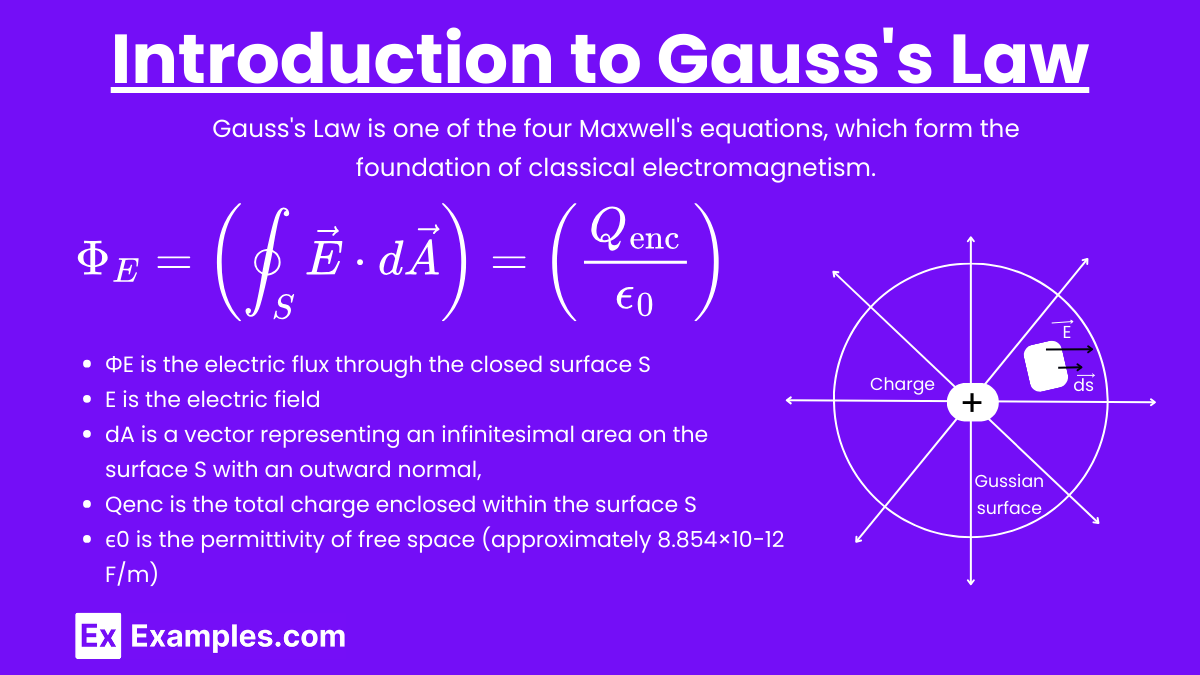
Gauss’s Law is one of the four Maxwell’s equations, which form the foundation of classical electromagnetism. It relates the electric flux through a closed surface to the charge enclosed by that surface.

Statement of Gauss’s Law

Gauss’s Law states:

\( \Phi_E = \left( \oint_S \vec{E} \cdot d\vec{A} \right) = \left( \frac{Q_{\text{enc}}}{\epsilon_0} \right) \)

Where:

ΦE is the electric flux through the closed surface S
E is the electric field
dA is a vector representing an infinitesimal area on the surface S with an outward normal,
Q_encis the total charge enclosed within the surface S
ϵ₀ is the permittivity of free space (approximately 8.854×10⁻¹² F/m).

Understanding Electric Flux

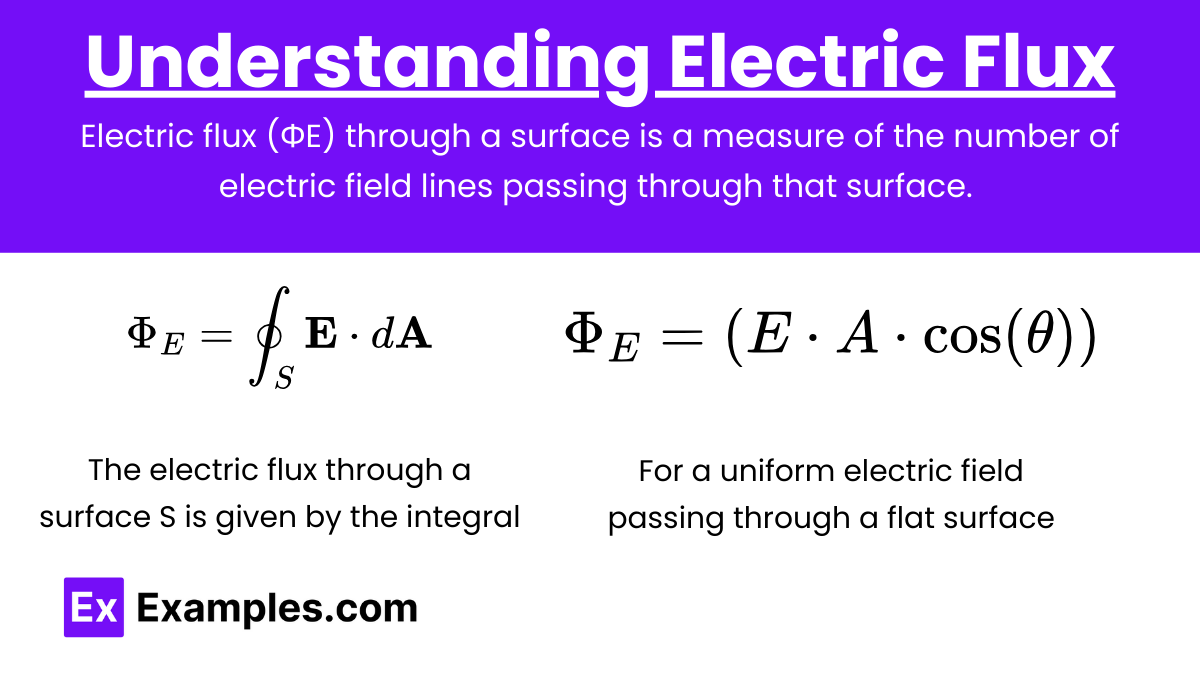
Electric flux (ΦE\Phi_EΦE) quantifies the number of electric field lines passing through a given surface. It is a measure of the electric field strength over an area.

Formula

The electric flux through a surface S is given by the integral:

\( \Phi_{E} = \oint_{S} \mathbf{E} \cdot d\mathbf{A} \)

where:

ΦE is the electric flux,
E is the electric field vector,
dA is the differential area vector on the surface S with an outward normal.

For a uniform electric field passing through a flat surface, the formula simplifies to:

\( \Phi_E = \left( E \cdot A \cdot \cos(\theta) \right) \)

where:

E is the magnitude of the electric field,
A is the area of the surface,
θ is the angle between the electric field and the normal to the surface.

Key Points About Electric Flux

It is proportional to the number of electric field lines intersecting the surface.
Positive flux indicates field lines exiting the surface, while negative flux indicates field lines entering the surface.

Applying Gauss’s Law

1. Symmetry Considerations

Gauss’s Law is particularly useful in situations with high symmetry (spherical, cylindrical, or planar symmetry). In such cases, the electric field can be assumed to be uniform over the Gaussian surface, simplifying the calculations.

2. Choosing a Gaussian Surface

To effectively apply Gauss’s Law, choose a Gaussian surface that matches the symmetry of the charge distribution. Common surfaces include:

Spherical surfaces for point charges or spherical charge distributions.
Cylindrical surfaces for line charges.
Planar surfaces for infinite plane sheets of charge.

Steps to Apply Gauss’s Law

Identify the symmetry of the charge distribution.
Choose an appropriate Gaussian surface based on the symmetry.
Calculate the electric flux through the Gaussian surface.
Determine the enclosed charge (Q_enc).
Solve for the electric field (E).

Examples

Example 1. Electric Field Due to a Point Charge

Using Gauss’s Law, the electric field E due to a point charge Q can be determined. For a spherical Gaussian surface of radius rrr centered on the charge:
\( \Phi_{E} = \oint \mathbf{E} \cdot d\mathbf{A} = \frac{Q}{\epsilon_{0}} \)
\( E \cdot 4\pi r^{2} = \frac{Q}{\epsilon_{0}} \)
\( E = \frac{Q}{4\pi \epsilon_{0} r^{2}} \)
This result matches Coulomb’s Law for the electric field of a point charge.

Example 2. Electric Field of an Infinite Line Charge

For an infinite line charge with linear charge density λ, consider a cylindrical Gaussian surface of radius r and length L:
\( \Phi_{E} = \oint \mathbf{E} \cdot d\mathbf{A} = \frac{\lambda L}{\epsilon_{0}} \)
\( E \cdot 2\pi r L = \frac{\lambda L}{\epsilon_{0}} \)
\( E = \frac{\lambda}{2\pi \epsilon_{0} r} \)
The electric field decreases with distance rrr from the line charge.

Example 3. Electric Field of an Infinite Plane Sheet of Charge

For an infinite plane sheet with surface charge density σ\sigmaσ, using a Gaussian pillbox that straddles the sheet:
\( \Phi_{E} = \oint \mathbf{E} \cdot d\mathbf{A} = \frac{\sigma A}{\epsilon_{0}} \)
Since the field is the same on both sides of the sheet:
\( 2EA = \frac{\sigma A}{\epsilon_{0}} \)
\( E = \frac{\sigma}{2\epsilon_{0}} \)
This shows that the electric field is constant and independent of the distance from the sheet.

Example 4. Electric Field Inside a Conducting Shell

For a charged conducting shell with total charge Q, the electric field inside the shell (at any point within the cavity) is zero. Using a Gaussian surface inside the conductor:
\( \Phi_{E} = \oint \mathbf{E} \cdot d\mathbf{A} = 0 \)
\( \mathbf{E} = 0 \)
This result shows that the electric field inside a conductor in electrostatic equilibrium is zero.

Example 5. Electric Field Outside a Conducting Sphere

For a conducting sphere with radius RRR and total charge Q, the electric field outside the sphere is the same as if all the charge were concentrated at the center. Using a spherical Gaussian surface of radius r where r>R
\( \Phi_{E} = \oint \mathbf{E} \cdot d\mathbf{A} = \frac{Q}{\epsilon_{0}} \)
\( E \cdot 4\pi r^{2} = \frac{Q}{\epsilon_{0}} \)
\( E = \frac{Q}{4\pi \epsilon_{0} r^{2}} \)
This is identical to the result for a point charge, demonstrating the equivalence of a spherical charge distribution to a point charge for points outside the sphere.

Multiple Choice Questions

Question 1

A point charge q is placed at the center of a spherical Gaussian surface of radius R. What is the electric flux Φ through the surface?

A) q/ϵ₀
B) q/4πϵ₀R²
C) qR/ϵ₀
D) q/R²

Answer: A) q/ϵ₀

Explanation: According to Gauss’s Law, the electric flux Φ through a closed surface is equal to the charge enclosed q divided by the permittivity of free space ϵ₀. Mathematically, this is expressed as:

Φ=qenc/ϵ₀

Since the point charge q is at the center of the spherical Gaussian surface, it is entirely enclosed by the surface. Thus, the electric flux through the surface is q/ϵ₀.

Question 2

Which of the following statements about Gauss’s Law is true?

A) Gauss’s Law can only be applied to spherical symmetry.
B) The electric flux through a closed surface depends only on the charge enclosed by that surface.
C) Gauss’s Law states that the total electric flux through any closed surface is always zero.
D) Gauss’s Law is only valid for conductors.

Answer: B) The electric flux through a closed surface depends only on the charge enclosed by that surface.

Explanation: Gauss’s Law is a general law that applies to any closed surface, not just those with spherical symmetry. It states that the electric flux through a closed surface is directly proportional to the charge enclosed within that surface and is given by:

Φ=qenc/ϵ₀

This means that the flux depends only on the enclosed charge, not on the shape or size of the surface. Therefore, statement B is true. Statements A, C, and D are incorrect as they either limit the applicability of Gauss’s Law or misrepresent its content.

Question 3

A long, straight, uniformly charged rod with linear charge density λ is enclosed by a cylindrical Gaussian surface of radius R and length L, coaxial with the rod. What is the electric flux through the cylindrical surface?

A) λL/ϵ0
B) λ²πRL/ϵ0
C) λL/2πϵ₀R
D) λ²πR²Lϵ₀

Answer: A) λL/ϵ₀

Explanation: To find the electric flux through the cylindrical Gaussian surface, we apply Gauss’s Law. The total charge q_encenclosed by the cylindrical surface is given by the linear charge density λ multiplied by the length L:

q_enc = λL

According to Gauss’s Law, the electric flux Φ\PhiΦ through the cylindrical surface is:

Φ=q_encϵ₀=λLϵ₀

Therefore, the correct answer is λLϵ₀.